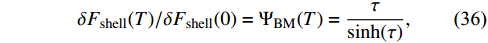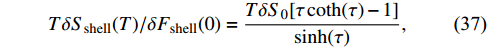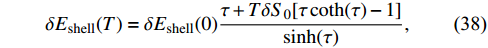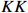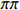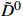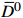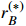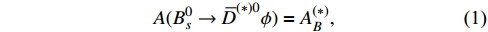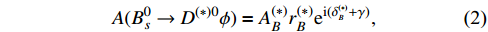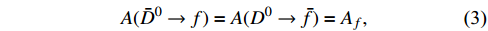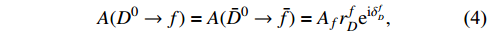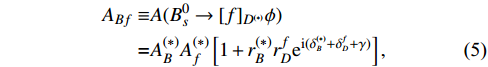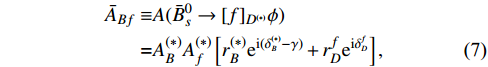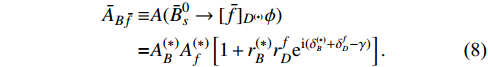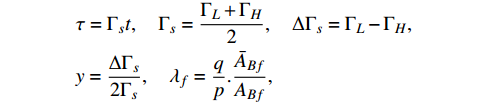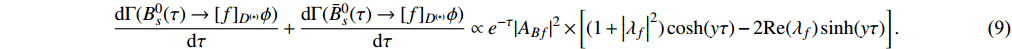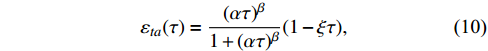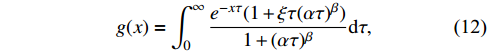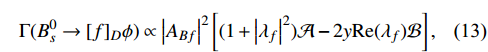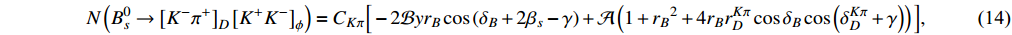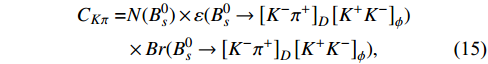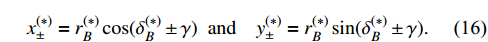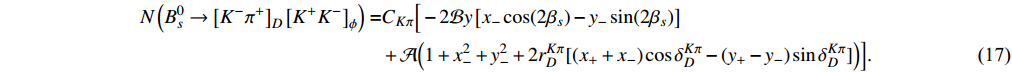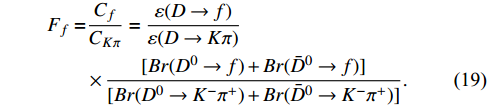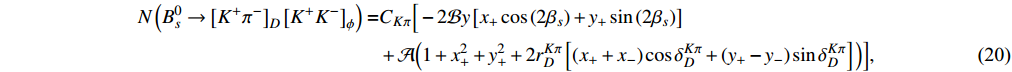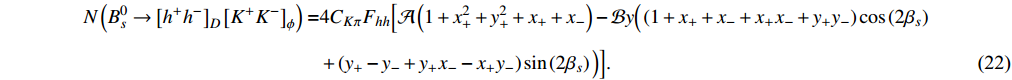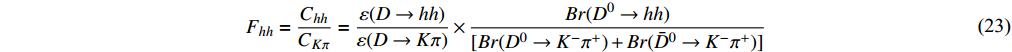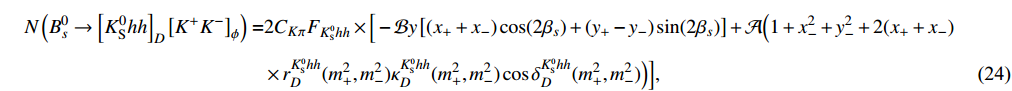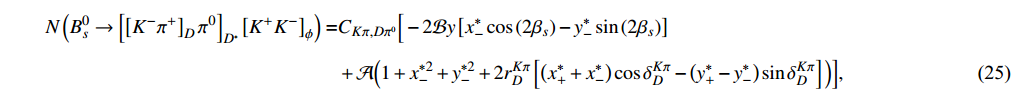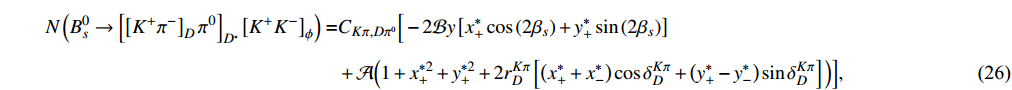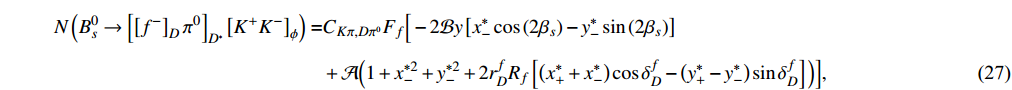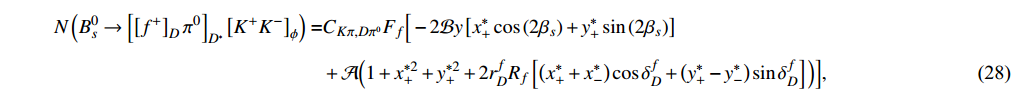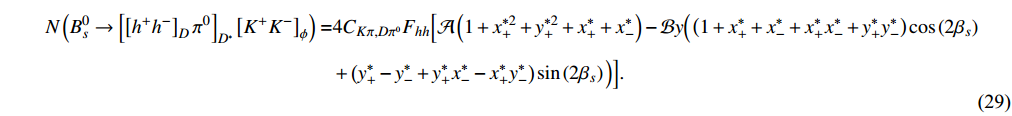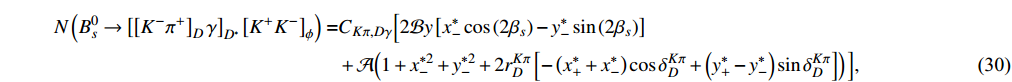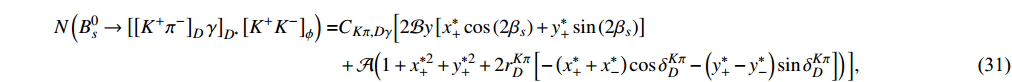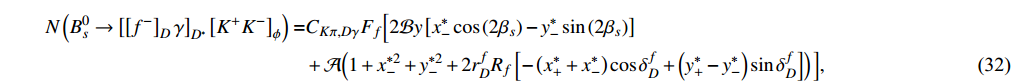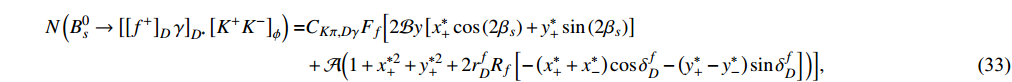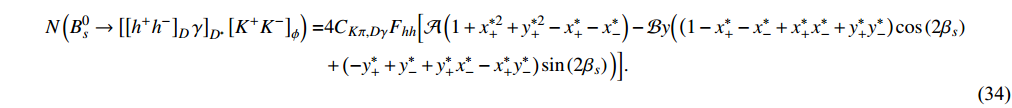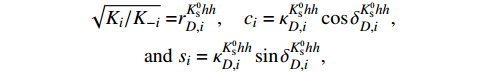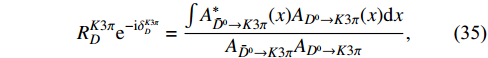-
Precision measurement of the CKM [1] angle
γ (which is defined asarg[−VudV∗ub/VcdV∗cb] ) in various B-meson decay modes is one of the main goals of flavor physics. Such measurements can be achieved by exploiting the interference of the decays that proceed via theb→cˉus andb→uˉcs tree-level amplitudes, in which the determination of the relative weak phaseγ is not affected by theoretical uncertainties.Several methods have been proposed to extract
γ [2-11]. In LHCb, the best precision is obtained by combining the measurements of many decay modes, which yieldsγ=(74.0+5.0−5.8)∘ [12]. This precision dominates the world average ofγ from tree-level decays. LHCb has presented a new measurement based on the BPGGSZ method [7] using the full Run 1 and Run 2 data. The result isγ=(68.7+5.2−5.1)∘ [13], and it constitutes the single best world measurement ofγ . A2σ difference betweenB+ andB0s results was observed since the summer of 2018. TheB0s measurement is based on a single decay mode with only Run 1 data, i.e.,B0s→D∓sK± ,① and exhibits large uncertainty [14]. In October 2020, LHCb included a similar analysis with the decayB0s→D∓sK±π+π− [15] based on Run 1 and Run 2 data, for whichγ−2βs=(42±10±4±5)∘ . The most recent LHCb combination is thereforeγ=(67±4)∘ [16], and the newB+ (B0s ) result is(64+4−5)∘ ((82+17−20)∘) . AdditionalB0s decay modes will aid in improving the measurement precision of theB0s modes and the understanding of a possible discrepancy with respect to theB+ modes. The two analyses based onB0s→D∓sK±(π+π−) use time-dependent methods and are therefore strongly reliant on the B-tagging capabilities of the LHCb experiment. It should be noted that measurements exist at LHCb forB0 mesons [17, 18], which also exhibit quite good prospects as their current average is(82+8−9)∘ . These are based on the decayB0→D0K∗0 , where theB0 is self-tagged from theK∗0→K+π− decay. As opposed to measurements withB0s , those withB0 andB+ are also accessible at Belle II [19]. The prospects of these measurements at LHCb are provided in Ref. [20]. For the modeB0s→D∓sK± , one may anticipate a precision onγ of the order of4∘ after the end of LHC Run 3 in 2025, and1∘ by 2035-2038. In combination with theB0 andB+ modes, the expected sensitivities are1.5∘ and0.35∘ . The anticipated precision provided by Belle II is1.5∘ .In this work,
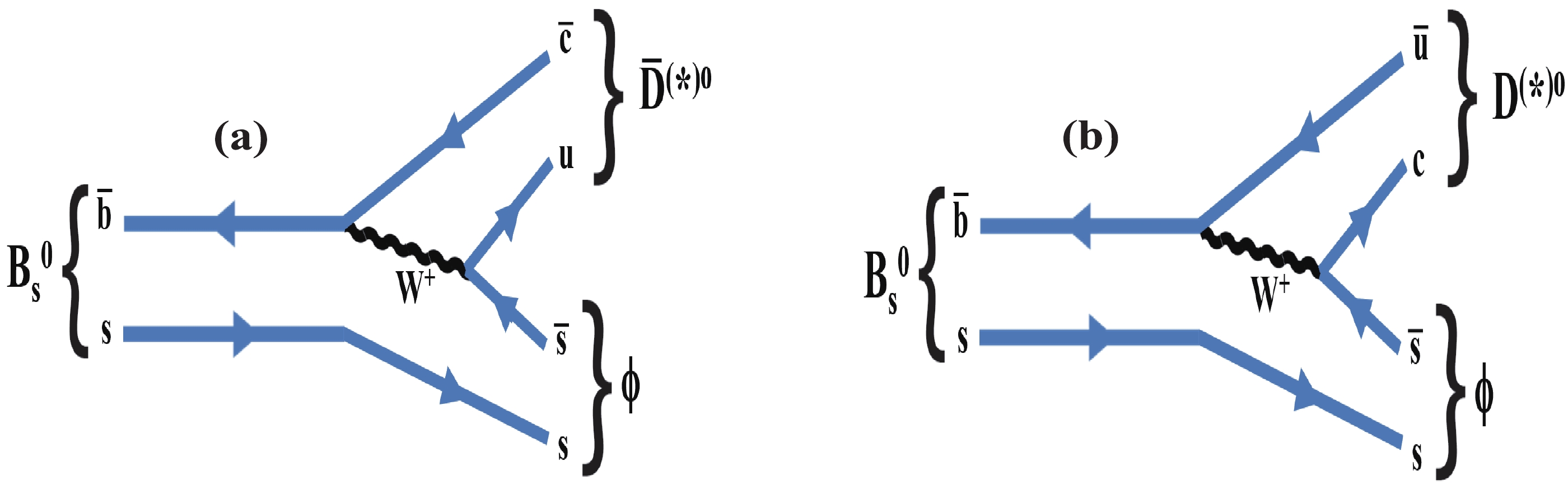
B0s→¯D(∗)0ϕ decays, the observations of which were published by the LHCb experiment in 2013 [21] and 2018 [22], are used to determineγ . A novel method presented in Ref. [22] also demonstrated the feasibility of measuringB0s→¯D∗0ϕ decays with a high purity. A partial reconstruction method is used for the¯D∗0 meson [23]. A time-integrated method [24-26] was investigated, in which it was shown that information regarding CP violation is preserved in the untagged rate ofB0s→˜D(∗)0ϕ (or ofB0→˜D0K0S ), and that if a sufficient number of different D-meson final states is included in the analysis, this decay alone can be used to measureγ in principle. The sensitivity toγ is expected to be much better with the case of theB0s→˜D(∗)0ϕ decay than forB0→˜D0K0S , as it is proportional to the decay width differencey=ΔΓ/2Γ defined in Section II and is equal to(6.3±0.3) % forB0s mesons and(0.05±0.50) % forB0 [27]. The sensitivity toγ from theB0s→˜D(∗)0ϕ modes arises from the interference between two colour-suppressed diagrams, as illustrated in Fig. 1. The relatively large expected value of the ratio of theˉb→ˉucˉs andˉb→ˉcuˉs tree-level amplitudes (20%−40 %; see Section IVA) serves as additional motivation for measuringγ inB0s→˜D(∗)0ϕ decays. In this study, five neutral D-meson decay modes, namelyKπ ,K3π ,Kππ0 ,KK , andππ , are included, the event yields of which are estimated using realistic assumptions based on measurements from LHCb [22, 28, 29]. We justify the choice of these decays and also discuss the case of the two decay modes˜D0→K0Sπ+π− andK0SK+K− in Section III.In Section II, the notations and the selection of the D-meson decay final states are introduced. In Section III, the expected signal yields and their uncertainties are presented. In Section IV, the sensitivity that can be achieved using solely these decays is presented, and further improvements are briefly discussed. In Section V, the future expected precision on
γ withB0s→¯D(∗)0ϕ at LHCb is discussed for the dataset available after LHC Run 3 by 2025 and after a possible second upgrade of LHCb by 2038. Finally, conclusions are presented in Section VI. -
Following the formalism introduced in Refs. [24-26], we define the amplitudes
A(B0s→¯D(∗)0ϕ)=A(∗)B,
(1) A(B0s→D(∗)0ϕ)=A(∗)Br(∗)Bei(δ(∗)B+γ),
(2) where
A(∗)B andr(∗)B are the magnitude of theB0s decay amplitude and amplitude magnitude ratio between the suppressed over the favoredB0s decay modes, respectively, whereasδ(∗)B andγ are the strong and weak phases, respectively. Neglecting mixing and CP violation in the D decays (see, for example, Refs. [30, 31]), the amplitudes into the final state f (denoted below as[f]D ) and its CP conjugateˉf are defined asA(ˉD0→f)=A(D0→ˉf)=Af,
(3) A(D0→f)=A(ˉD0→ˉf)=AfrfDeiδfD,
(4) where
δfD andrfD are the strong phase difference and relative magnitude, respectively, between theD0→f andˉD0→f decay amplitudes.The amplitudes of the full decay chains are given by
ABf≡A(B0s→[f]D(∗)ϕ)=A(∗)BA(∗)f[1+r(∗)BrfDei(δ(∗)B+δfD+γ)],
(5) ABˉf≡A(B0s→[ˉf]D(∗)ϕ)=A(∗)BA(∗)f[r(∗)Bei(δ(∗)B+γ)+rfDeiδfD].
(6) The amplitudes of the CP-conjugate decays are obtained by changing the sign of the weak phase
γ ˉABf≡A(ˉB0s→[f]D(∗)ϕ)=A(∗)BA(∗)f[r(∗)Bei(δ(∗)B−γ)+rfDeiδfD],
(7) ˉABˉf≡A(ˉB0s→[ˉf]D(∗)ϕ)=A(∗)BA(∗)f[1+r(∗)BrfDei(δ(∗)B+δfD−γ)].
(8) Using the standard notations
τ=Γst,Γs=ΓL+ΓH2,ΔΓs=ΓL−ΓH,y=ΔΓs2Γs,λf=qp.ˉABfABf,
and assuming that
|q/p|=1 (|q/p|=1.0003±0.0014 [32]), the untagged decay rate for the decayB0s/ˉB0s→[f]D(∗)ϕ is obtained by Eq. (10) of Ref. [33]:dΓ(B0s(τ)→[f]D(∗)ϕ)dτ+dΓ(ˉB0s(τ)→[f]D(∗)ϕ)dτ∝e−τ|ABf|2×[(1+|λf|2)cosh(yτ)−2Re(λf)sinh(yτ)].
(9) -
Experimentally, owing to the trigger and selection requirements as well as inefficiencies in the reconstruction, the decay time distribution is affected by the acceptance effects. The acceptance correction has been estimated from pseudoexperiments based on a related publication by the LHCb collaboration [34]. It is described by an empirical acceptance function
εta(τ)=(ατ)β1+(ατ)β(1−ξτ),
(10) with
α=1.5 ,β=2.5 , andξ=0.01 .Taking into account this effect, the time-integrated untagged decay rate is
Γ(˜B0s→[f]D(∗)ϕ)=∫∞0[dΓ(B0s(τ)→[f]D(∗)ϕ)dτ+dΓ(ˉB0s(τ)→[f]D(∗)ϕ)dτ]εta(τ)dτ.
(11) By defining the function
g(x)=∫∞0e−xτ(1+ξτ(ατ)β)1+(ατ)βdτ,
(12) and using Eq. (9), one obtains
Γ(B0s→[f]Dϕ)∝|ABf|2[(1+|λf|2)A−2yRe(λf)B],
(13) where
A=1−[g(1−y)+g(1+y)]/2 andB=1−[g(1−y)− g(1+y)]/2y . Withy=(0.128±0.009)/2 for theB0s meson [27], one obtainsA=0.488±0.005 andB=0.773±0.008 . Examples of decay-time acceptance distributions are displayed in Fig. 2. -
The D-meson decays are reconstructed in quasi flavor-specific modes
f−(≡f)=K−π+ ,K−3π , andK−π+π0 ; their CP-conjugate modesf+(≡ˉf)=K+π− ,K+3π , andK+π−π0 ; and CP-eigenstate modesfCP=K+K− andπ+π− .In the following, we introduce the weak phase
βs that is defined asβs=arg(−VtsV∗tbVcsV∗cb) . From Eqs. (5), (7), and (13) and withλf=e2iβsˉABfABf , for a given number of untaggedB0s mesons produced in thepp collisions at the LHCb interaction point,N(B0s) , we can compute the number ofB0s→ˉD0ϕ decays with the D meson decaying into the final statef− . For the reference decay modef−≡K−π+ , we obtainN(B0s→[K−π+]D[K+K−]ϕ)=CKπ[−2ByrBcos(δB+2βs−γ)+A(1+rB2+4rBrKπDcosδBcos(δKπD+γ))],
(14) in which the terms proportional to
(rKπD)2≪1 andyrKπD≪1 have been neglected (rKπD=5.90+0.34−0.25 % [27]). The best approximation for the scale factorCKπ isCKπ=N(B0s)×ε(B0s→[K−π+]D[K+K−]ϕ)×Br(B0s→[K−π+]D[K+K−]ϕ),
(15) where
ε(B0s→[K−π+]D[K+K−]ϕ) is the global detection efficiency of this decay mode, andBr(B0s→[K−π+]D [K+K−]ϕ) is its branching fraction. The value of the scale factorCKπ is estimated from the LHCb Run 1 data [22], the averagefs/fd of the b-hadron production fraction ratio measured by LHCb [35], and the different branching fractions [32].For better numerical behavior, we use the Cartesian coordinate parameterization
x(∗)±=r(∗)Bcos(δ(∗)B±γ) andy(∗)±=r(∗)Bsin(δ(∗)B±γ).
(16) Subsequently, Eq. (14) becomes
N(B0s→[K−π+]D[K+K−]ϕ)=CKπ[−2By[x−cos(2βs)−y−sin(2βs)]+A(1+x2−+y2−+2rKπD[(x++x−)cosδKπD−(y+−y−)sinδKπD])].
(17) For three and four body final states
K3π andKππ0 , multiple interfering amplitudes exist; therefore, their amplitudes and phasesδfD vary across the decay phase space. However, an analysis that is integrated over the phase space can be performed in a very similar manner to two body decays with the inclusion of an additional parameter, namely the so-called coherence factorRfD , which has been measured in previous experiments [36]. The strong phase differenceδfD is then treated as an effective phase that is averaged over all amplitudes. For these modes, we obtain an expression similar to (17):N(B0s→[f−]D[K+K−]ϕ)=CKπFf[−2By[x−cos(2βs)−y−sin(2βs)]+A(1+x2−+y2−+2rfDRfD[(x++x−)cosδfD−(y+−y−)sinδfD])],
(18) where
Ff is the scale factor of the f decay relative to theKπ decay and depends on the ratios of detection efficiencies and branching fractions of the corresponding modesFf=CfCKπ=ε(D→f)ε(D→Kπ)×[Br(D0→f)+Br(ˉD0→f)][Br(D0→K−π+)+Br(ˉD0→K−π+)].
(19) The value of
Ff for the different modes used in this study is determined from the LHCb measurements in theB±→DK± andB±→Dπ± modes, with two or four-body D decays [28, 29].The time-integrated untagged decay rate for
B0s→[ˉf]Dϕ is given by Eq. (13) by substitutingABf→ˉABˉf andλf→ˉλˉf=λ−1f=e−2iβs(ABˉf/ˉABˉf) , which is equivalent to the changeβs→−βs andγ→−γ (i.e.,x±→x∓ andy±→y∓ ). Therefore, the observables areN(B0s→[K+π−]D[K+K−]ϕ)=CKπ[−2By[x+cos(2βs)+y+sin(2βs)]+A(1+x2++y2++2rKπD[(x++x−)cosδKπD+(y+−y−)sinδKπD])],
(20) and for the modes
f+≡K+3π ,K+π−π0 N(B0s→[f+]D[K+K−]ϕ)=CKπFf[−2By[x+cos(2βs)+y+sin(2βs)]+A(1+x2++y2++2rfDRf[(x++x−)cosδfD+(y+−y−)sinδfD])].
(21) Obviously, any significant asymmetries on the yield of the observable corresponding to Eq. (17) with respect to Eq. (20), or Eq. (18) with respect to Eq. (21), are a clear signature for CP violation.
For the CP-eigenstate modes
D→h+h−(h≡K,π) , we haverD=1 andδD=0 . By following the same approach as that for quasi flavor-specific modes, the observables can be written asN(B0s→[h+h−]D[K+K−]ϕ)=4CKπFhh[A(1+x2++y2++x++x−)−By((1+x++x−+x+x−+y+y−)cos(2βs)+(y+−y−+y+x−−x+y−)sin(2βs))].
(22) Analogous to
Ff ,Fhh is defined asFhh=ChhCKπ=ε(D→hh)ε(D→Kπ)×Br(D0→hh)[Br(D0→K−π+)+Br(ˉD0→K−π+)]
(23) and their values are determined in the same manner as
Ff .For the modes
K0Sπ+π− andK0SK+K− (i.e.,K0Shh ), we obtainN(B0s→[K0Shh]D[K+K−]ϕ)=2CKπFK0Shh×[−By[(x++x−)cos(2βs)+(y+−y−)sin(2βs)]+A(1+x2−+y2−+2(x++x−)×rK0ShhD(m2+,m2−)κK0ShhD(m2+,m2−)cosδK0ShhD(m2+,m2−))],
(24) where
FK0Shh is defined as in Eq. (23). The strong parametersrK0ShhD(m2+,m2−) ,κK0ShhD(m2+,m2−) , andcosδK0ShhD(m2+,m2−) vary over the Dalitz plot(m2+,m2−)≡(m2(K0Sπ+), m2(K0Sπ−)) and are defined in Section III. -
For the
D∗0 decays, we consider the two modesD∗0→D0π0 andD∗0→D0γ , where theD0 mesons are reconstructed, as in the above, in quasi flavor-specific modesKπ ,K3π , andKππ0 as well as CP-eigenstate modesππ andKK . As demonstrated in Ref. [37], the formalism for the cascadeB0s→ˉD∗0ϕ,ˉD∗0→ˉD0π0 is similar to that ofB0s→ˉD0ϕ . Therefore, the relevant observables can be written similarly to Eqs. (17), (18), (20), (21), and (22), by substitutingCKπ→CKπ,Dπ0 ,rB→r∗B , andδB→δ∗B (x±→x∗± andy±→y∗± ):N(B0s→[[K−π+]Dπ0]D∗[K+K−]ϕ)=CKπ,Dπ0[−2By[x∗−cos(2βs)−y∗−sin(2βs)]+A(1+x∗2−+y∗2−+2rKπD[(x∗++x∗−)cosδKπD−(y∗+−y∗−)sinδKπD])],
(25) N(B0s→[[K+π−]Dπ0]D∗[K+K−]ϕ)=CKπ,Dπ0[−2By[x∗+cos(2βs)+y∗+sin(2βs)]+A(1+x∗2++y∗2++2rKπD[(x∗++x∗−)cosδKπD+(y∗+−y∗−)sinδKπD])],
(26) N(B0s→[[f−]Dπ0]D∗[K+K−]ϕ)=CKπ,Dπ0Ff[−2By[x∗−cos(2βs)−y∗−sin(2βs)]+A(1+x∗2−+y∗2−+2rfDRf[(x∗++x∗−)cosδfD−(y∗+−y∗−)sinδfD])],
(27) N(B0s→[[f+]Dπ0]D∗[K+K−]ϕ)=CKπ,Dπ0Ff[−2By[x∗+cos(2βs)+y∗+sin(2βs)]+A(1+x∗2++y∗2++2rfDRf[(x∗++x∗−)cosδfD+(y∗+−y∗−)sinδfD])],
(28) N(B0s→[[h+h−]Dπ0]D∗[K+K−]ϕ)=4CKπ,Dπ0Fhh[A(1+x∗2++y∗2++x∗++x∗−)−By((1+x∗++x∗−+x∗+x∗−+y∗+y∗−)cos(2βs)+(y∗+−y∗−+y∗+x∗−−x∗+y∗−)sin(2βs))].
(29) In the case of
D∗0→D0γ , the formalism is very similar, except that there is an effective strong phase shift ofπ with respect toD∗0→D0π0 [37]. The observables can be derived from the previous ones by substitutingCKπ,Dπ0→CKπ,Dγ andδ∗B→δ∗B+π (i.e.x∗±→−x∗± andy∗±→−y∗± ):N(B0s→[[K−π+]Dγ]D∗[K+K−]ϕ)=CKπ,Dγ[2By[x∗−cos(2βs)−y∗−sin(2βs)]+A(1+x∗2−+y∗2−+2rKπD[−(x∗++x∗−)cosδKπD+(y∗+−y∗−)sinδKπD])],
(30) N(B0s→[[K+π−]Dγ]D∗[K+K−]ϕ)=CKπ,Dγ[2By[x∗+cos(2βs)+y∗+sin(2βs)]+A(1+x∗2++y∗2++2rKπD[−(x∗++x∗−)cosδKπD−(y∗+−y∗−)sinδKπD])],
(31) N(B0s→[[f−]Dγ]D∗[K+K−]ϕ)=CKπ,DγFf[2By[x∗−cos(2βs)−y∗−sin(2βs)]+A(1+x∗2−+y∗2−+2rfDRf[−(x∗++x∗−)cosδfD+(y∗+−y∗−)sinδfD])],
(32) N(B0s→[[f+]Dγ]D∗[K+K−]ϕ)=CKπ,DγFf[2By[x∗+cos(2βs)+y∗+sin(2βs)]+A(1+x∗2++y∗2++2rfDRf[−(x∗++x∗−)cosδfD−(y∗+−y∗−)sinδfD])],
(33) N(B0s→[[h+h−]Dγ]D∗[K+K−]ϕ)=4CKπ,DγFhh[A(1+x∗2++y∗2+−x∗+−x∗−)−By((1−x∗+−x∗−+x∗+x∗−+y∗+y∗−)cos(2βs)+(−y∗++y∗−+y∗+x∗−−x∗+y∗−)sin(2βs))].
(34) CKπ,Dπ0 andCKπ,Dγ are determined in the same manner asCKπ , i.e., from the LHCb Run 1 data [22] and taking into account the fraction of longitudinal polarization in the decayB0s→D∗0ϕ ,fL=(73±15±4) % [22], and the branching fractionsBr(ˉD∗0→ˉD0π0) andBr(ˉD∗0→ˉD0γ) [32]. -
The LHCb collaboration has measured the yields of the
B0s→˜D(∗)0ϕ and˜D0→Kπ modes using Run 1 data, corresponding to an integrated luminosity of 3fb−1 (Ref. [22]). Taking into account the cross-section differences among different center-of-mass energies, the equivalent integrated luminosities in different data over several years from LHCb are summarized in Table 1. The corresponding expected yields of the˜D0 meson decaying into other modes are also estimated according to Refs. [28], [29], and [41]. The scaled results are listed in Table 2, where the longitudinal polarization fractionfL=(73±15±4) % [22] ofB0s→˜D∗0ϕ is considered so that the CP eigenvalue of the final state is well defined and is similar to that of theB0s→˜D0ϕ mode.Years/Run √s /TeV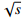
Int. lum./fb−1 Cross section Equiv. 7 TeV data 2011 7 1.1 σ2011=38.9μb 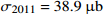
1.1 2012 8 2.1 1.17×σ2011 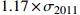
2.4 Run 1 − 3.2 − 3.5 2015 to 2018 (Run 2) 13 5.9 2.00×σ2011 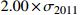
11.8 Total − 9.1 − 15.3 Expect. yield (Run 1 only) B0s→˜D0(Kπ)ϕ 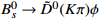
577 (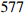
132±13 [22])
B0s→˜D0(K3π)ϕ 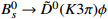
218 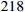
B0s→˜D0(Kππ0)ϕ 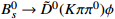
58 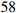
B0s→˜D0(KK)ϕ 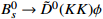
82 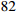
B0s→˜D0(ππ)ϕ 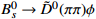
24 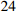
B0s→˜D0(K0Sππ)ϕ 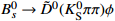
54 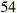
B0s→˜D0(K0SKK)ϕ 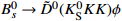
8 
B0s→˜D∗0ϕ mode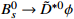
D0π0 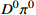
D0γ 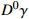
B0s→˜D∗0(Kπ)ϕ 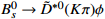
337 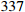
184 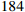
(119 [22]) B0s→˜D∗0(K3π)ϕ 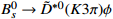
127 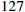
69 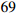
B0s→˜D∗0(Kππ0)ϕ 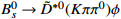
34 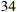
18 
B0s→˜D∗0(KK)ϕ 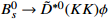
48 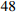
26 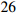
B0s→˜D∗0(ππ)ϕ 
14 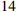
8 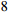
Table 2. Expected yield of each mode for
9.1fb−1 (Run 1 and Run 2 data). The expected yields for theB0s→˜D∗0ϕ sub-modes are scaled by the longitudinal fraction of polarizationfL=(73±15) %. To be scaled by 6.3 (90) for prospects after 2025 (2038) (see Section V).Several additional parameters are used in the sensitivity study, as indicated in Table 3. Most of these originate from D decays, and the scale factors F are calculated by using the data from Refs. [28] and [29], as well as the branching fractions from PDG [32].
Parameter Value −2 βS [mrad]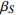
−36.86±0.82 [42]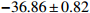
y=ΔΓs/2Γs (%)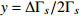
6.40±0.45 [27]
rKπD (%)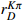
5.90+0.34−0.25 [27]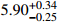
δKπD [deg]
188.9+8.2−8.9 [27]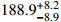
rK3πD (%)
5.49±0.06 [36]
RK3πD (%)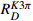
43+17−13 [36]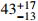
δK3πD [deg]
128+28−17 [36]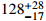
rKππ0D (%)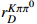
4.47±0.12 [36]
RKππ0D (%)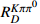
81±6 [36]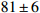
δKππ0D [deg]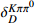
198+14−15 [36]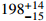
Scale factor (w.r.t. Kπ )
(Stat. uncertainty only) FK3π (%)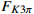
37.8±0.1 [28]
FKππ0 (%)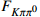
10.0±0.1 [29]
FKK (%)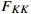
14.2±0.1 [28]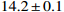
Fππ (%)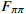
4.2±0.1 [28]
Table 3. Other external parameters used in sensitivity study. The scale factors F are also listed.
The expected numbers of signal events are also calculated from the full expressions provided in Sections IIB and IIC by using the detailed branching fraction derivations explained in Ref. [22], as well as scaling by the LHCb Run 1 and Run 2 integrated luminosities, as listed in Table 1. The obtained normalization factors
CKπ ,CKπ,Dπ0 , andCKπ,Dγ are608±67 ,347±56 , and189±31 , respectively. To compute the uncertainty on the normalization factors, we assume that it is possible to improve the global uncertainty on the measurement of the branching fraction of the decay modesB0s→¯D(∗)0ϕ , and of the polarization of the modeB0s→¯D∗0ϕ , by a factor of 2 when adding the LHCb data from Run 2 [22]. The values of the three normalization factors are in good agreement with the yields listed in Table 2.The number of expected event yields and the value of the coherence factor
RD listed in Tables 2 and 3 justify a posteriori our choice of performing the sensitivity study onγ with the D-meson decay modesKπ ,K3π ,Kππ0 ,KK andππ . By definition, the value ofRD is 1 for two-body decays andrD=1 for CP-eigenstates, whereas forK3π ,RD is approximately43 % and larger, namely81 %, forKππ0 . A largerRD results in stronger sensitivity toγ . According to Eqs. (20), (21), and (22), it is clear that the largest sensitivity toγ is expected to originate from the orderedD0 decay modes:KK ,ππ ,Kπ ,Kππ0 , andK3π , for the same number of selected events. Therefore, even with lower yields, the modesKππ0 andππ should be of interest; this is discussed in Section IV I.Returning to the modes
D0→K0Sππ andK0SKK , the scale factors areFK0Sππ=(9.3±0.1) % andFK0SKK= (1.4±0.1) % [41]. The strong parametersrK0ShhD(m2+,m2−) ,κK0ShhD(m2+,m2−) , andcosδK0ShhD(m2+,m2−) can be defined according the effective method presented in Ref. [43], by using quantum-correlated˜D0 decays, in which the phase space(m2+, m2−) is divided intoN tailored regions or “bins” [44], such that in the bin of index i,√Ki/K−i=rK0ShhD,i,ci=κK0ShhD,icosδK0ShhD,i, and si=κK0ShhD,isinδK0ShhD,i,
where
δK0ShhD,i is the strong phase difference andκK0ShhD,i is the coherence factor. A recent publication by the BES-III collaboration [45] combines its data with the results of CLEO-c [44], while applying the same technique to obtain the values of theci ,si , andK±i parameters varying with the phase space. The binning schemes are symmetric with respect to the diagonal in the Dalitz plot(m2+, m2−) (i.e.±i ). These results have also been compared to an amplitude model from the B-factories BaBar and Belle [46]. When porting the result between the BES-III/CLEO-c combination, obtained using quantum correlated˜D0 decays, and LHCb forB0s→˜D(∗)0ϕ measurements, care should be taken with the bin conventions so that there may be a minus sign in the phase (which only affectssi ). The expected yield listed in Table 2 for the modeD0→K0Sππ is 54 events, whereas it is 8 events for the decayD0→K0SKK . Although the binning scheme in the latter case is only2×2 , its expected yield is definitely too small to be considered further. ForD0→K0Sππ , the binned method of Refs. [44, 45] splits the selected˜D0 events over2×8 bins, such that with Run 1 and Run 2, only approximately three events may populate each bin. This is the reason that, although the related observable is presented in Eq. (24), we decide not include that mode in the sensitivity study. This choice could eventually be revisited after Run 3, when approximately 340B0s→D0(K0Sππ)ϕ events should be available, at which point approximately 20 events may populate each bin. -
The sensitivity study consists of testing and measuring the value of the unfolded
γ ,r(∗)B , andδ(∗)B parameters and their expected resolution, after having computed the values of the observables according to various initial configurations and given the external inputs for the other involved physics parameters or associated experimental observables. To achieve this, a procedure involving globalχ2 fit based on the CKMfitter package [47] has been established to generate pseudoexperiments and to fit samples ofB0s→˜D(∗)0ϕ events.This section is organized as follows: In Section IVA, we explain the various configurations that we tested for the nuisance strong parameters
r(∗)B , andδ(∗)B , as well as the value ofγ . In Section IVB, we explain how the pseudoexperiments have been generated. In Section IVC, the first step of the method is illustrated with one- and two-dimensional (1-D and 2-D) p-value profiles for theγ ,r(∗)B , andδ(∗)B parameters. Before showing how theγ ,r(∗)B , andδ(∗)B parameters are unfolded from the generated pseudoexperiments in Section IVF, we discuss the stability of the former 1-D p-value profile forγ when changing the time acceptance parameters (Section IVD) and for a newly available binning scheme for theD→K3π decay (Section IVE). Thereafter, the unfolded values forγ and precisions (sensitivity) for the Run 1 & 2 LHCb dataset for the various generated configurations ofδ(∗)B andr(∗)B are presented in Section IVG. We conclude with Sections IVH and IVI, in which we study the intriguing case whereγ=74∘ (see LHCb 2018 combination [12], recently superseded by [16]), and we test the effect of dropping or not dropping the least abundant expected decays modesB0s→˜D(∗)0(ππ)ϕ andB0s→˜D(∗)0(Kππ0)ϕ in the Run 1 & 2 LHCb dataset. -
The sensitivity study was performed with the CKM angle
γ true value set to(65.66+0.90−2.65)∘ (1.146 rad), as obtained by the CKMfitter group, while excluding any measured values ofγ in its global fit [42]. As a reminder, the average of the LHCb measurements isγ=(74.0+5.0−5.8)∘ [12]; therefore, the valueγ=74∘ was also tested (see Subsection IVH).The value of the strong phases
δ(∗)B is a nuisance parameter that cannot be predicted or guessed by any argument, and therefore, six different values are assigned thereto: 0, 1, 2, 3, 4, and 5 rad (0∘ ,57.3∘ ,114.6∘ ,171.9∘ ,229.2∘ , and286.5∘ ). This corresponds to 36 tested configurations (6×6 ).As both interfering diagrams displayed in Fig. 1 are color-suppressed, the value of the ratio of the
ˉb→ˉucˉs andˉb→ˉcuˉs tree-level amplitudesr(∗)B is expected to be|VubVcs|/|VcbVus|∼0.4 . This assumption is well supported by the study performed withB0s→D∓sK± decays by the LHCb collaboration, for which a value ofrB=0.37+0.10−0.09 has been measured [14]. However, as the decayB0s→D∓sK± is color-favored, it is important to test other values obtained from already measured colour-suppressed B-meson decays, as non-factorizing final state interactions can modify the decay dynamics [48]. Among these, the decayB0→DK∗0 plays such a role, for which the LHCb has obtainedrB=0.22+0.17−0.27 [42], which has been confirmed by a more recent and accurate computation:rB=0.265±0.023 [18]. The value ofrB is known to impact the precision onγ measurements strongly as1/rB [49]. Therefore, the two extreme values0.22 and0.40 forr(∗)B were tested for the sensitivity study, whereas the values forrB andr∗B are expected to be similar.This leads to a total of 72 tested configurations for the
r(∗)B ,δB , andδ∗B parameters (2×6×6 ). -
As the first step, different configurations for the observables corresponding to Sections IIB and IIC are computed. The observables are obtained with the value of the angle
γ and with the values of the four nuisance parametersr(∗)B andδ(∗)B fixed to various sets of initial true values (see Section IVA), whereas the external parameters listed in Table 3 and the normalization factorsCKπ ,CKπ,Dπ0 , andCKπ,Dγ are left free to vary within their uncertainties. In the second step, for the obtained observables, including their uncertainties that we assume to be their square root, as well as all the other parameters except forγ ,r(∗)B , andδ(∗)B , a globalχ2 fit is performed to compute the resulting p-value distributions of theγ ,r(∗)B , andδ(∗)B parameters. As the third step, for the obtained observables, including their uncertainties, as well as all the other parameters except forγ ,r(∗)B , andδ(∗)B , 4000 pseudoexperiments are generated according to Eqs. (14)-(34) for the various tested configurations. As the fourth step, for each of the generated pseudoexperiments, all of the quantities are varied within their uncertainties. Thereafter, a globalχ2 fit is performed to unfold the value of the parametersγ ,r(∗)B0s , andδ(∗)B0s for each of the 4000 generated pseudoexperiments. As the fifth step, for the distribution of the 4000 values of the fittedγ ,r(∗)B , andδ(∗)B , an extended unbinned maximum likelihood fit is performed to compute the most probable value for each of the former five parameters, together with their dispersions. The resulting values are compared to their injected initial true values. Finally, the sensitivity toγ ,r(∗)B , andδ(∗)B is deduced, and any bias correlation is eventually highlighted and studied. -
Figure 3 displays the 1-D p-value profiles of
γ at step 2 of the procedure described in Section IVB. The figure is obtained for an example set of initial parameters:γ=65.66∘ (1.146 rad),r(∗)B=0.4 ,δB=3.0 rad, andδ∗B=2.0 rad. The assumed integrated luminosity in this case is that of the LHCb data collected in Run 1 & 2. The corresponding fitted value isγ=(65.7+6.3−33.8)∘ , which is thus in excellent agreement with the initial tested true value. Figure 3 also depicts the corresponding distributions obtained from a full frequentist treatment on the Monte-Carlo simulation basis [50], whereγ=(65.7+6.9−34.9)∘ . This is considered as a demonstration that the two estimates onγ are in quite fair agreement, at least at the68.3 % confidence level (CL), such that no obvious under-coverage is experienced with the nominal method, based on theROOT functionTMath::Prob [51]. On the upper part of the distribution, the relative under-coverage of the “Prob ” method is approximately6.3/6.9≃91 %. As opposed to the full frequentist treatment on the Monte-Carlo simulation basis, the nominal retained method allows performing computations of a very large number of pseudoexperiments within a reasonable amount of time and with non-prohibitive CPU resources. For the LHCb Run 1 & 2 dataset, 72 configurations of 4000 pseudoexperiments were generated (288000 pseudoexperiments in total). The entire study was repeated another two times for prospective studies with future anticipated LHCb data, such that more than approximately 864000 pseudoexperiments were generated for this publication (see Section V). In the same figure, one can also observe the effect of modifying the value ofr(∗)B from0.4 to0.22 , for whichγ=(65.7+12.0−60.7)∘ , where the upper uncertainty scales are roughly as expected:1/r(∗)B (6.3×0.4/0.22= 12.6). Compared to the full frequentist treatment on the Monte-Carlo simulation, whereγ=(65.7+13.2−∞)∘ , the relative under-coverage of the “Prob ” method is approximately12.0/13.2≃91 %. Finally, the p-value profile ofγ is also displayed when dropping the information provided by theB0s→˜D∗0ϕ mode, thus retaining only that of theB0s→˜D0ϕ mode. In this case,γ is equal to(65.7+6.3(12.0)−∞)∘ ,r(∗)B=0.4 (0.22 ), such that the CL interval is noticeably enlarged on the lower side of theγ angle distribution (further details can be found in Section VC).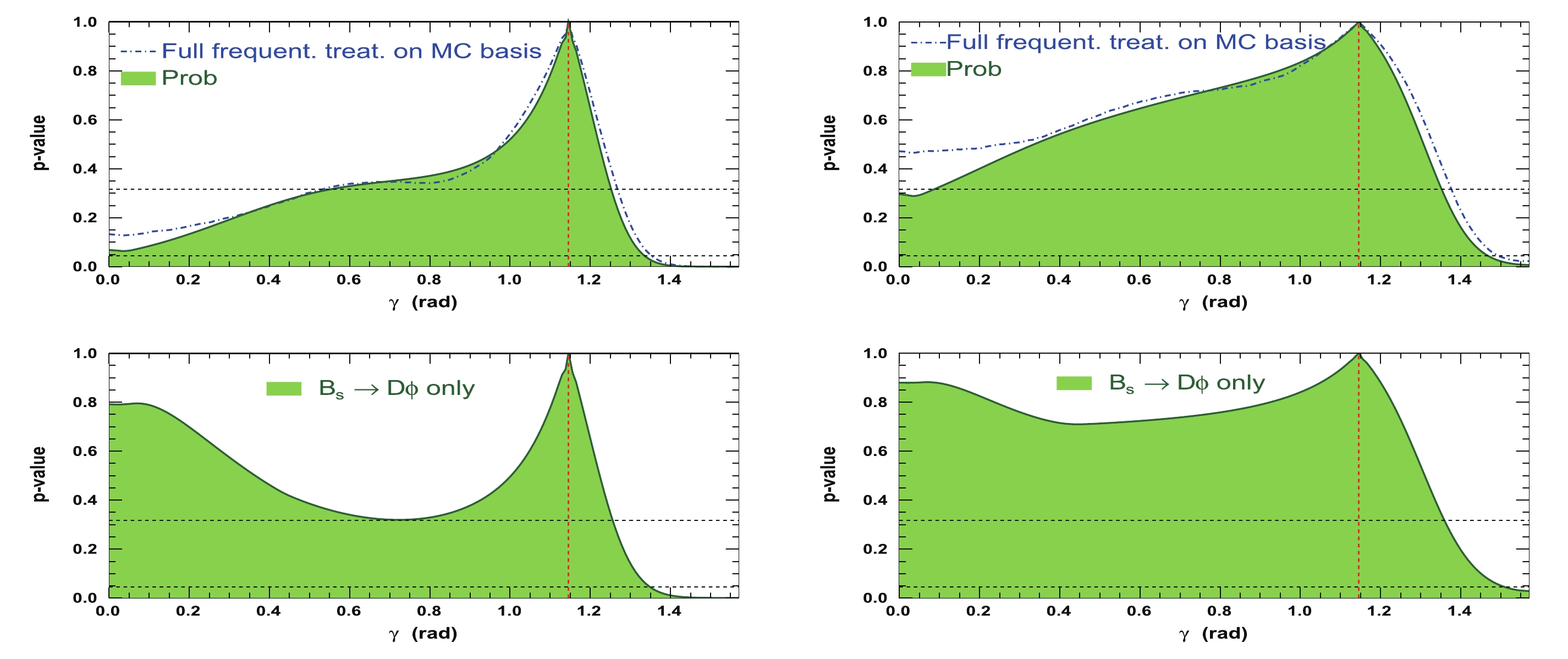
Figure 3. (color online) Profile of p-value of global
χ2 fit toγ after computing observables (top left) for a set of true initial parameters:γ=1.146 rad,r(∗)B=0.4 ,δB=3.0 rad, andδ∗B=2.0 rad (the corresponding distribution obtained from the full frequentist treatment on the Monte-Carlo simulation basis [50] is superimposed on the same distribution). The related p-value profile forr(∗)B=0.22 is also presented (top right). The assumed integrated luminosity assumed is that of the LHCb data collected in Run 1 & 2. Profile of p-value of globalχ2 fit toγ after computing observables, where only decay modeB0s→˜D0ϕ is used, and for a set of true initial parameters:γ=1.146 rad,r(∗)B=0.4 (bottom left) and0.22 (bottom right),δB=3.0 rad, andδ∗B=2.0 rad. In each figure, the vertical dashed red line indicates the initialγ true value, and the two horizontal dashed black lines refer to 68.3 and 95.4% CLs.Figure 4 displays the 1-D p-value profile of the nuisance parameters
r(∗)B andδ(∗)B for the same set of initial parameters (γ=65.66∘ (1.146 rad),r(∗)B=0.4 ,δB=3.0 rad, andδ∗B=2.0 rad) and the same projected integrated luminosity. It can be observed that the p-value is the maximum at the initial tested value, as expected.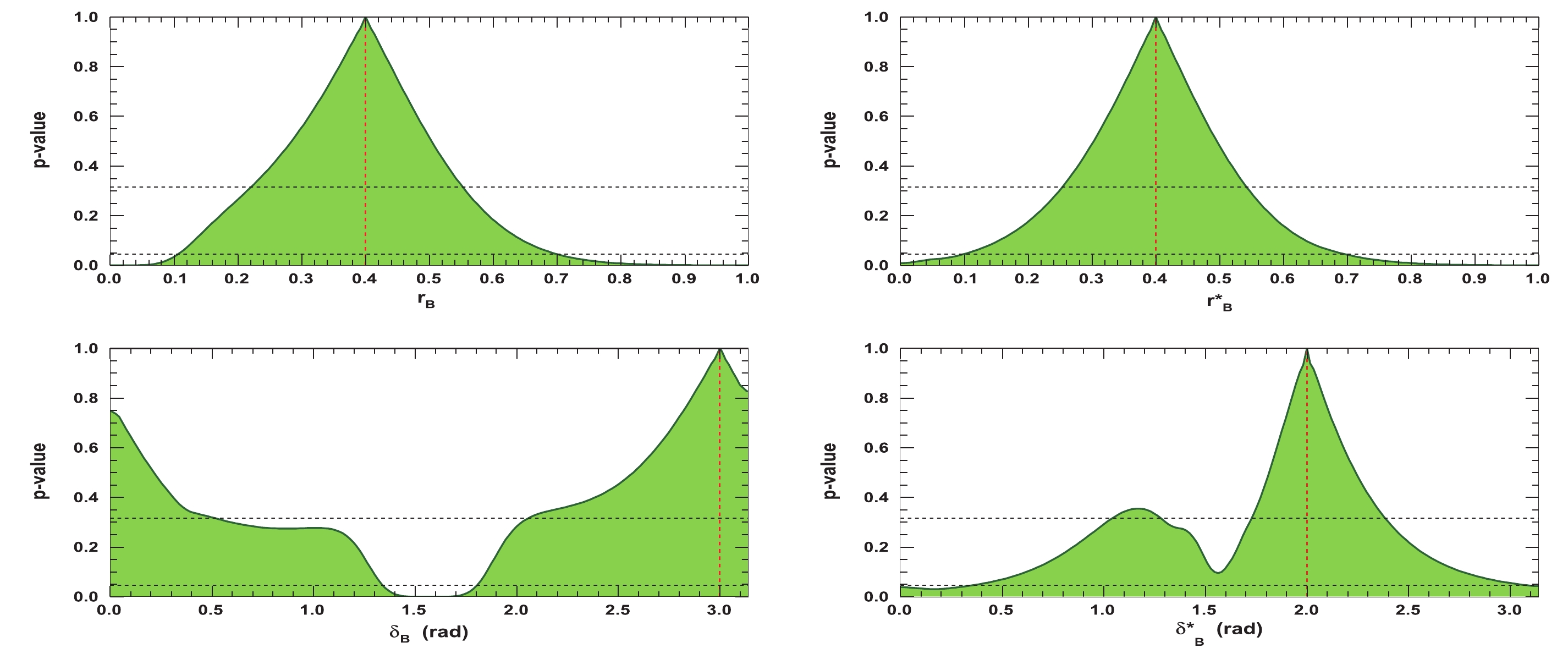
Figure 4. (color online) Profiles of p-value distributions of global
χ2 fit tor(∗)B (top left (right)) andδ(∗)B (bottom left (right)) after computing observables for set of initial true parameters:γ=65.66∘ (1.146 rad),r(∗)B=0.4 ,δB=3.0 rad, andδ∗B=2.0 rad. In each figure, thevertical dashed red line indicates the initialr(∗)B andδ(∗)B true values, and the two horizontal dashed black lines refer to 68.3 and 95.4% CLs.The 2-D p-value profiles of the nuisance parameters
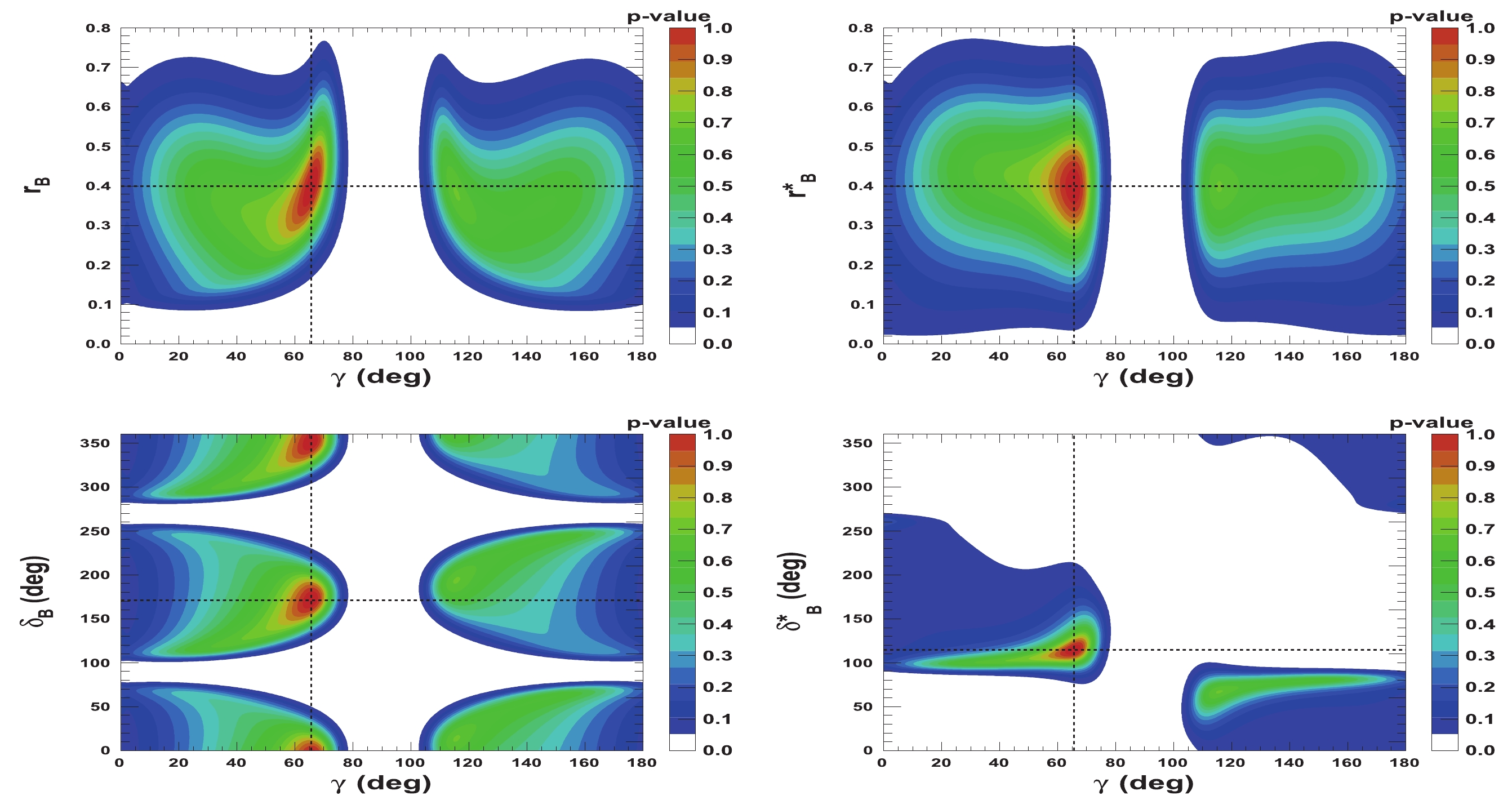
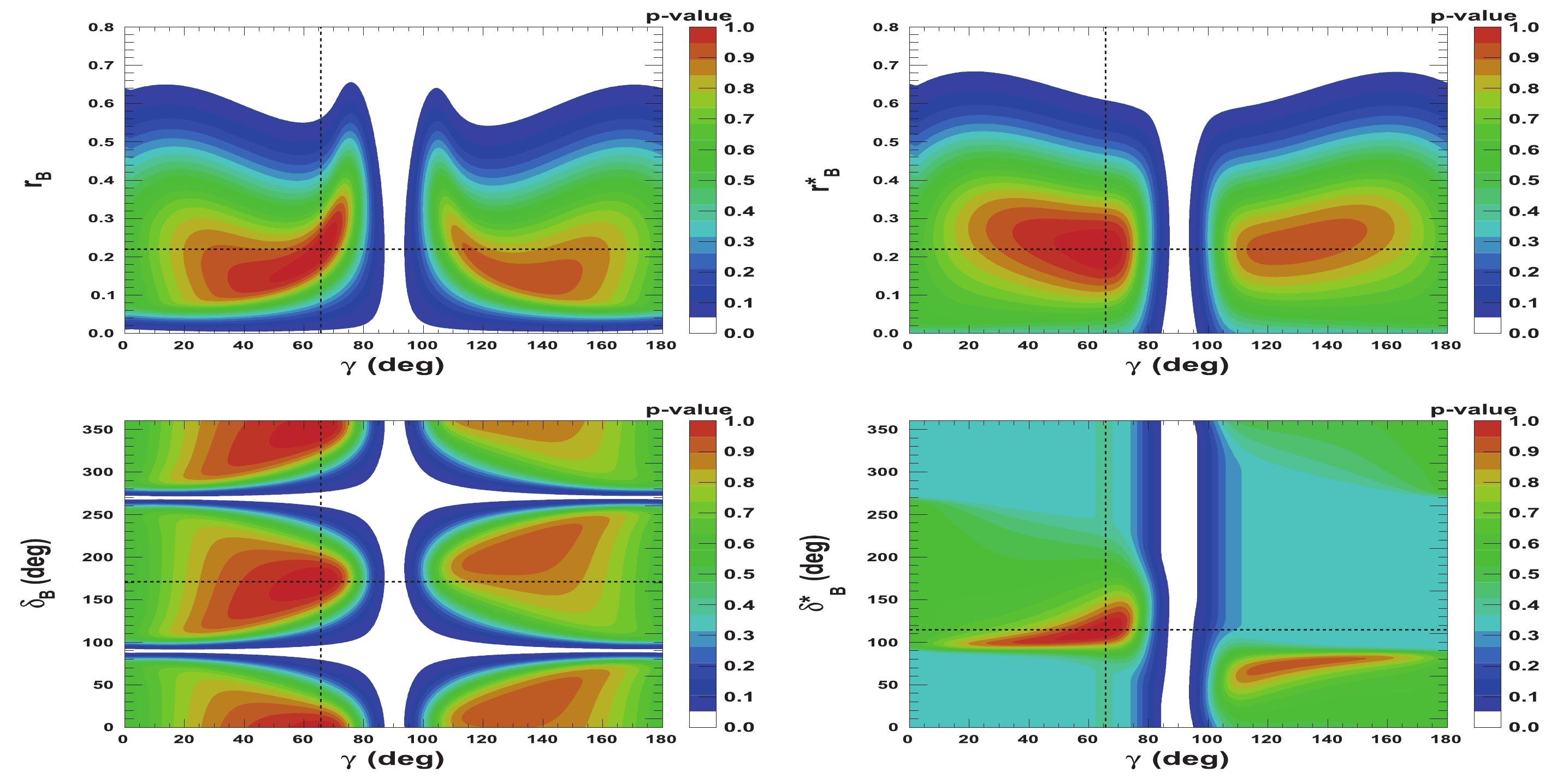
r(∗)B andδ(∗)B as a function ofγ are presented in Figs. 5-8. Figures 5 and 6 correspond to two other example configurationsγ=1.146 rad,δB=1.0 rad, andδ∗B=5.0 rad, andr(∗)B=0.4 andr(∗)B=0.22 , respectively. Figures 7 and 8 represent the configurationsγ=1.146 rad,δB=3.0 rad, andδ∗B=2.0 rad, andr(∗)B=0.4 andr(∗)B=0.22 , respectively. These 2-D views allow the correlation between the different parameters to be observed. In general, large correlations betweenδ(∗)B andγ are observed. In the case of configurations wherer(∗)B=0.4 , a large fraction of theδ(∗)B vs.γ plane can be excluded at a95 % CL, whereas the fraction is significantly reduced for the correspondingr(∗)B=0.22 configurations. For theδ∗B vs.γ plane, the advantage of our Cartesian coordinate approach (see Section IIB and IIC) can easily be observed, together with the fact that in the case of the modeD∗0→D0γ , there is an effective strong phase shift ofπ with respect to theD∗0→D0π0 [37], such that additional constraints enable the fold ambiguities with respect to the associatedδB vs.γ plane to be removed.
Figure 5. (color online) 2-D p-value profile distribution of nuisance parameters
r(∗)B andδ(∗)B as a function ofγ . In each figure, the dashed black lines indicate the initial true values:γ=65.66∘ (1.146 rad),δB=57.3∘ (1.0 rad), andδ∗B=286.5∘ (5.0 rad), as well asr(∗)B=0.4 .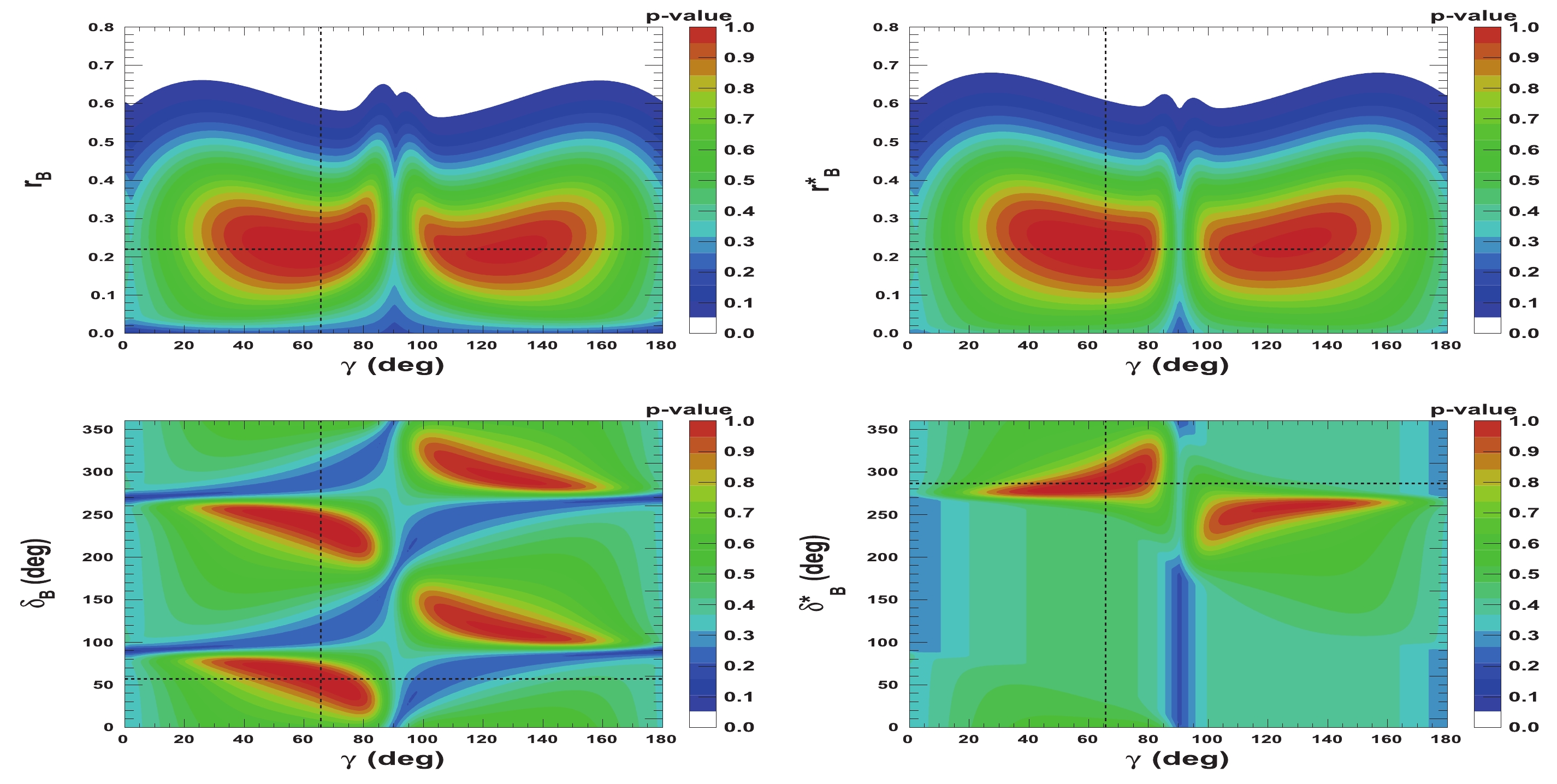
Figure 6. (color online) 2-D p-value profile distribution of nuisance parameters
r(∗)B andδ(∗)B as a function ofγ . In each figure, the dashed black lines indicate the initial true values:γ=65.66∘ (1.146 rad),δB=57.3∘ (1.0 rad), andδ∗B=286.5∘ (5.0 rad), as well asr(∗)B=0.22 . -
Figure 9 demonstrates that for a tested configuration of
γ=1.146 rad,r(∗)B=0.4 , andδ(∗)B=1.0 rad, the impact of the time acceptance parametersA andB can eventually be non negligible and the parameters affect the profile distribution of the p-value of the globalχ2 fit toγ . For the given example, the fitted value ofγ is either(65.3+14.3−38.4)∘ or(66.5+13.8−51.0)∘ when the time acceptance is either accounted for or not. The reason due to which the precision improves when the time acceptance is taken into account may not be intuitive. This is because, forB/A≃1.6 , as opposed to the case ofB/A≃1.0 , the impact of the first term in Eq. (14), which is directly proportional tocos(δB+2βs−γ) , is amplified with respect to the second term, for which the sensitivity toγ is more diluted.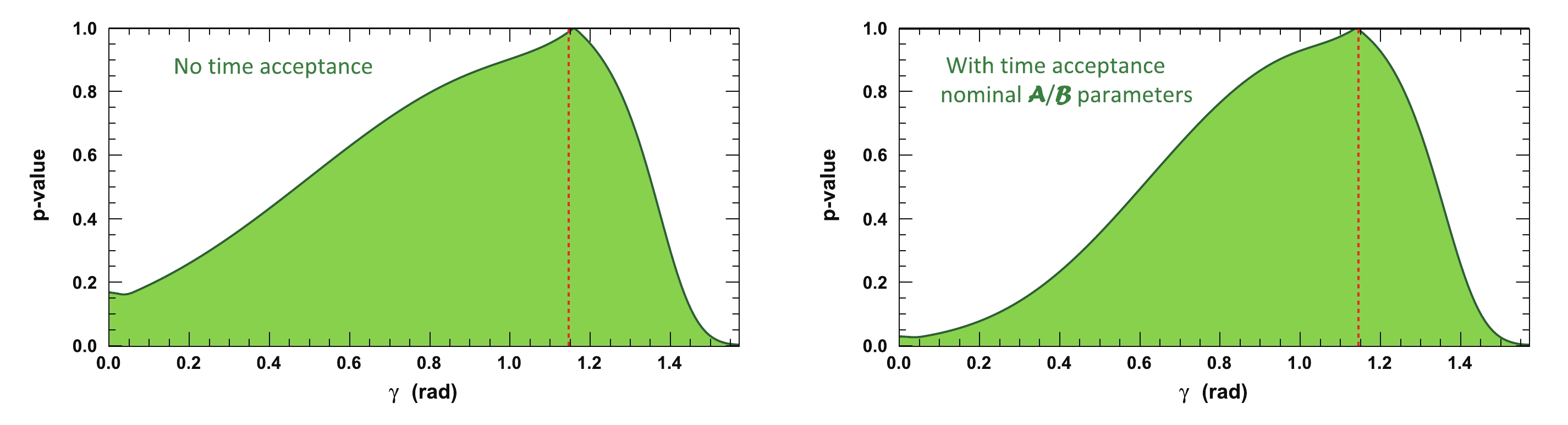
Figure 9. (color online) Profile of p-value distribution of global
χ2 fit toγ for a set of true initial parameters:γ=1.146 rad,r(∗)B=0.4 , andδ(∗)B=1.0 rad. The assumed integrated luminosity is that of the LHCb data collected in Run 1 & 2 when the time acceptance valuesA andB are set to 1 in Eqs. (14) to (34): no time acceptance (top left) or to their nominal valuesA=0.488±0.005 andB=0.773±0.008 (top right), as computed in Section IIA. The dashed red line indicates the initialγ true valueγ=65.66∘ (1.146 rad).Even if the parameters
A andB are computed to a precision at the percentage level (Section IIA), we further investigate the effect of changing their values. Note that for this study, the overall efficiency is maintained constant, whereas the shape of the acceptance function is varied. The valuesα ,β , andξ are changed in Eq. (10), and the results of those changes are listed in Table 4. Whenα increases, bothA andB become larger, but the value of the ratioB/A decreases. Whenβ orξ decreases, the three values ofA ,B , andB/A increase. The effect of changingβ orξ alone is small. A modification ofα has a much greater impact onA andB . However, all of these changes have a weak impact on the precision of the fittedγ value. This is quite encouraging, as it means that the relative efficiency loss caused by the time acceptance effects will not cause a significant change in the sensitivity to the CKMγ angle. As a result, the time acceptance requirements can be varied without substantial concern to improve the signal purity and statistical significance when analyzing theB0s→˜D(∗)0ϕ decays with LHCb data.α 
β 
ξ 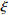
A 
B 
B/A 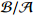
Fitted γ (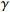
∘ )
1.0 2.5 0.01 0.367 0.671 1.828 66.5+13.8−40.1 
1.5 2.5 0.01 0.488 0.773 1.584 65.3+14.3−38.4 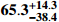
2.0 2.5 0.01 0.570 0.851 1.493 65.3+13.2−37.8 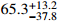
1.5 2.0 0.01 0.484 0.751 1.552 65.9+13.2−39.0 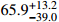
1.5 3.0 0.01 0.491 0.789 1.607 66.5+13.2−38.4 
1.5 2.5 0.02 0.480 0.755 1.573 66.5+13.8−39.5 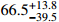
1.5 2.5 0.005 0.492 0.783 1.591 65.3+13.8−36.7 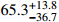
Table 4. Expected value of
γ as a function of different time acceptance parameters. The second line corresponds to the nominal values. The nominal set of parametersA andB is indicated in bold. -
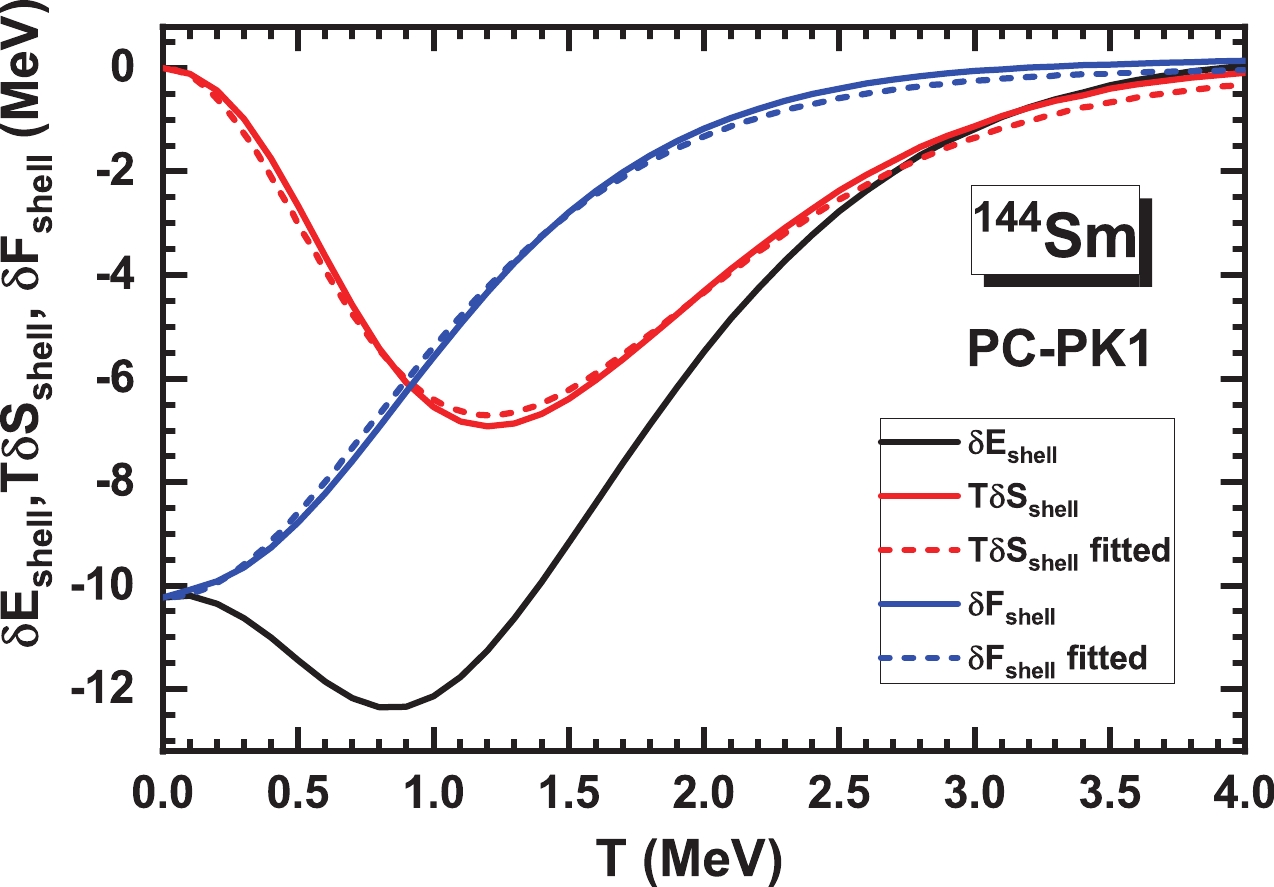
According to Ref. [46], the averaged values of the
K3π input parameters over the phase space, which are defined asRK3πDe−iδK3πD=∫A∗ˉD0→K3π(x)AD0→K3π(x)dxAˉD0→K3πAD0→K3π,
(35) are used here and correspond to a relatively limited value for the coherence factor:
RK3πD=(43+17−13) % [36]. A more attractive approach may be to perform the analysis in disjoint bins of the phase space. In this case, the parameters are re-defined within each bin. New values forRK3πD andδK3πD in each bin from Ref. [52] have alternatively been employed. No noticeable changes were observed in theγ andr(∗)B fitted p-value profiles, but it is possible that certain fold-effects onδ(∗)B , e.g., as observed in Figs. 5-7, become less probable. The lack of significant improvement is expected as the˜D0→K3π mode is not the dominant decay, and also because the new measurements ofRK3πD andδK3πD in each bin still have large uncertainties. -
As explained in Section IVB, for each of the tested
γ ,r(∗)B , andδ(∗)B configurations, 4000 pseudoexperiments are generated, for which the values ofγ ,r(∗)B , andδ(∗)B are unfolded from the globalχ2 fits (see Section IVC for illustrations). Figure 10 displays the extended unbinned maximum likelihood fits to the nuisance parametersr(∗)B andδ(∗)B . The initial configuration isγ=65.66∘ (1.146 rad),r(∗)B=0.4 ,δB=171.9∘ (3 rad), andδ∗B=114.6∘ (2 rad), with an integrated luminosity that is equivalent to that of the LHCb Run 1 & 2 data. It can be compared with Fig. 4. All of the distributions are fitted with the Novosibirsk empirical function, the description of which contains a Gaussian core part and a left or right tail, depending on the sign of the tail parameter [53]. The fitted values ofr(∗)B are centered at their initial tested values of 0.4, with a resolution of 0.14, and no bias is observed. Forδ(∗)B , the fitted value is(176±42)∘ ((104±13)∘ ) for an initial true value that is equal to171.9∘ (114.6∘ ). The fitted value forδ∗B is slightly shifted by approximately2/3 of a standard deviation, but its measurement is much more precise than that ofδB , as it is measured from both theD∗0→D0γ andD∗0→D0π0 observables.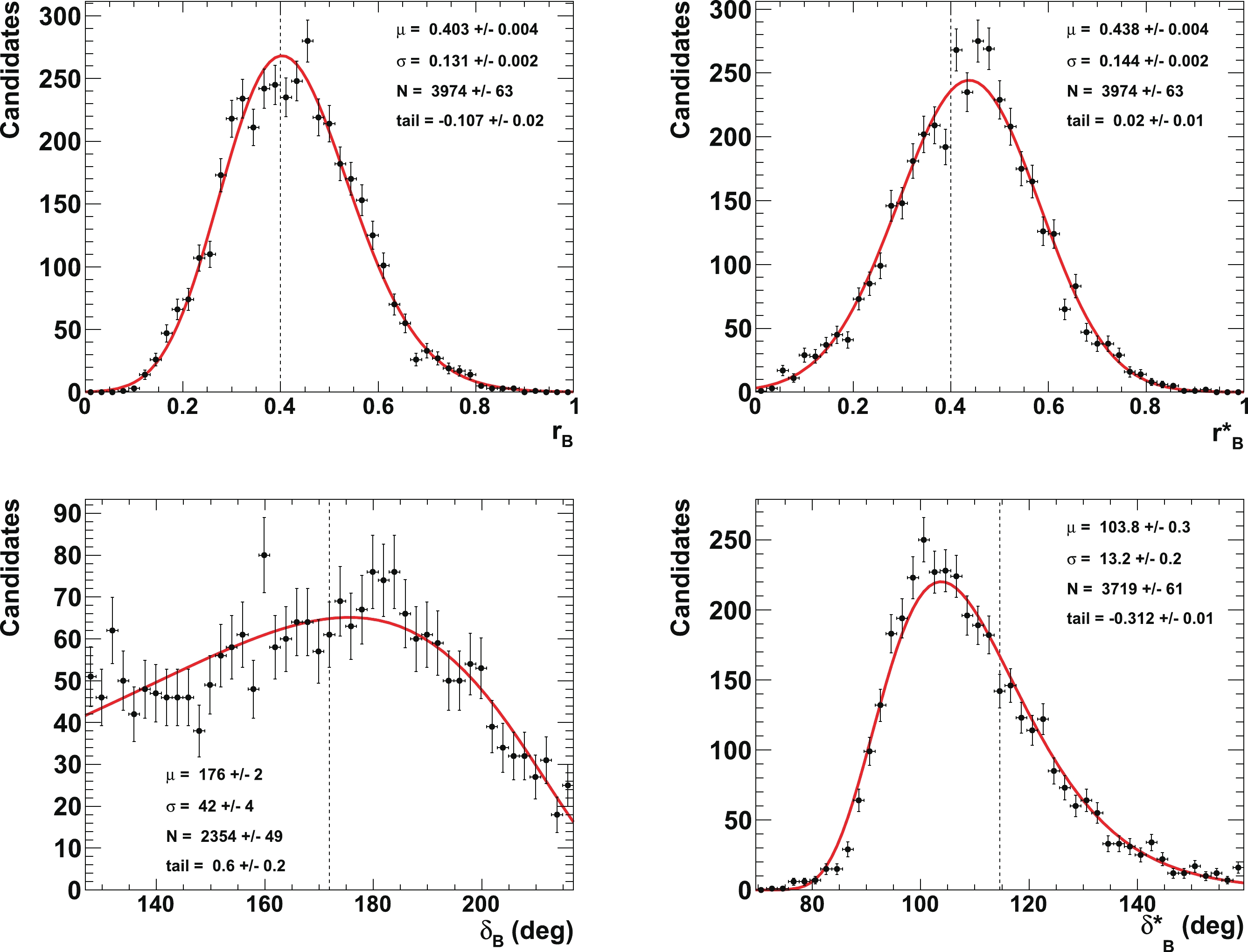
Figure 10. (color online) Fit to distributions of nuisance parameters
r(∗)B (top left (right)) andδ(∗)B (bottom left (right)) obtained from 4000 pseudoexperiments. The initial configuration isγ=65.66∘ (1.146 rad),r(∗)B=0.4 ,δB=171.9∘ (3 rad), andδ∗B=114.6∘ (2 rad). The distributions ofδ(∗)B are plotted and fitted within±45∘ of their initial true values. In the distributions, only the candidates with values ofγ ∈ [0∘, 90∘] are considered.Figure 11 presents the corresponding fit to the CKM angle
γ , where the valuer(∗)B=0.22 is also tested. This figure can be compared to the initial p-value profiles illustrated in Fig. 3. As shown in Figs. 7 and 8,γ is correlated with the nuisance parametersr(∗)B andδ(∗)B . Such correlations may generate long tails in the distributions, as obtained from the 4000 pseudoexperiments. To account for these tails, extended unbinned maximum likelihood fits, constituting two Novosibirsk functions with opposite-side tails, are performed on theγ distributions. With an initial value of65.66∘ , the fitted value forγ returns a central value equal toμγ=(65.9±0.3)∘ with a resolution ofσγ=(8.8±0.2)∘ whenr(∗)B=0.4 , andμγ=(66.6±0.7)∘ with a resolution ofσγ=(14.4±0.5)∘ whenr(∗)B=0.22 . The worse resolution obtained withr(∗)B=0.22 follows the empirical behavior1/r(∗)B (8.8×0.4/0.22≃16.0 ). Again, no bias is observed.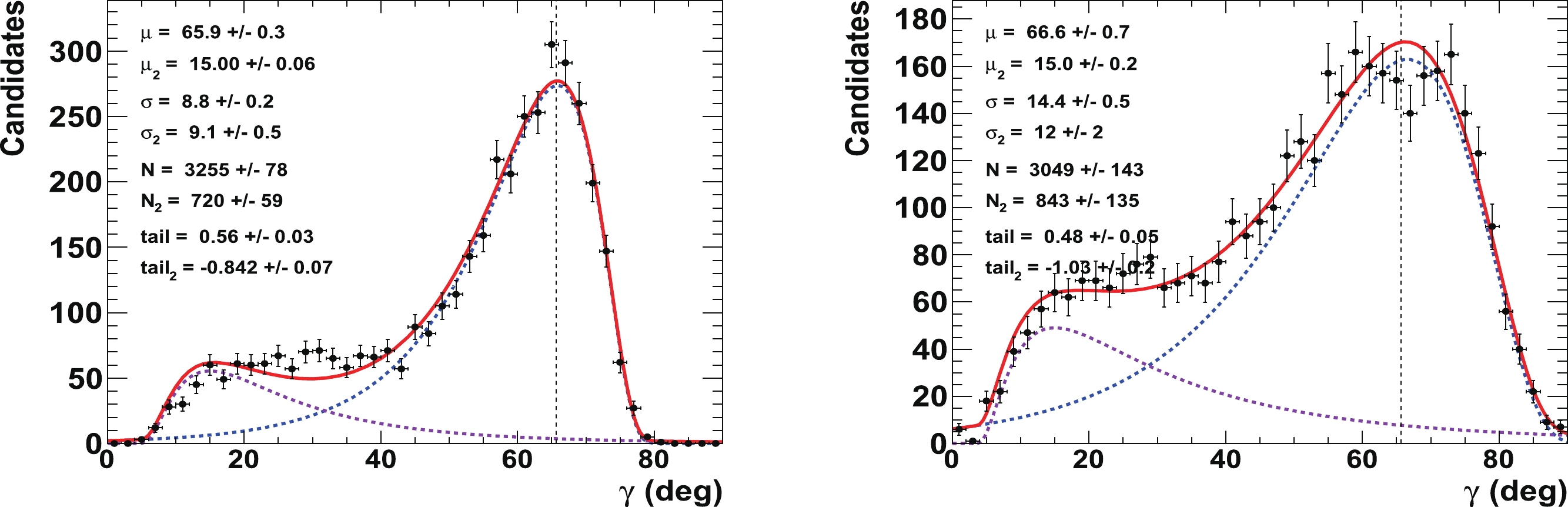
Figure 11. (color online) Fit to distributions of nuisance parameters
γ obtained from 4000 pseudoexperiments. The initial configuration isγ=65.66∘ (1.146 rad),r(∗)B=0.4 (left) and 0.22 (right),δB=171.9∘ (3 rad), andδ∗B=114.6∘ (2 rad). In the distributions, only the candidates with a value ofγ ∈ [0∘, 90∘] are considered. The purple dashed curve represents tails generated by the correlations with the nuisance parametersr(∗)B andδ(∗)B , whereas the blue dashed curve indicates the core part of the distribution, and the plain red line is the sum of the two components of the fit.Finally, Fig. 12 displays the 2-D distributions of the nuisance parameters
r(∗)B andδ(∗)B as a function ofγ obtained from 4000 pseudoexperiments. This figure can be compared with the corresponding p-value profiles presented in Fig. 7.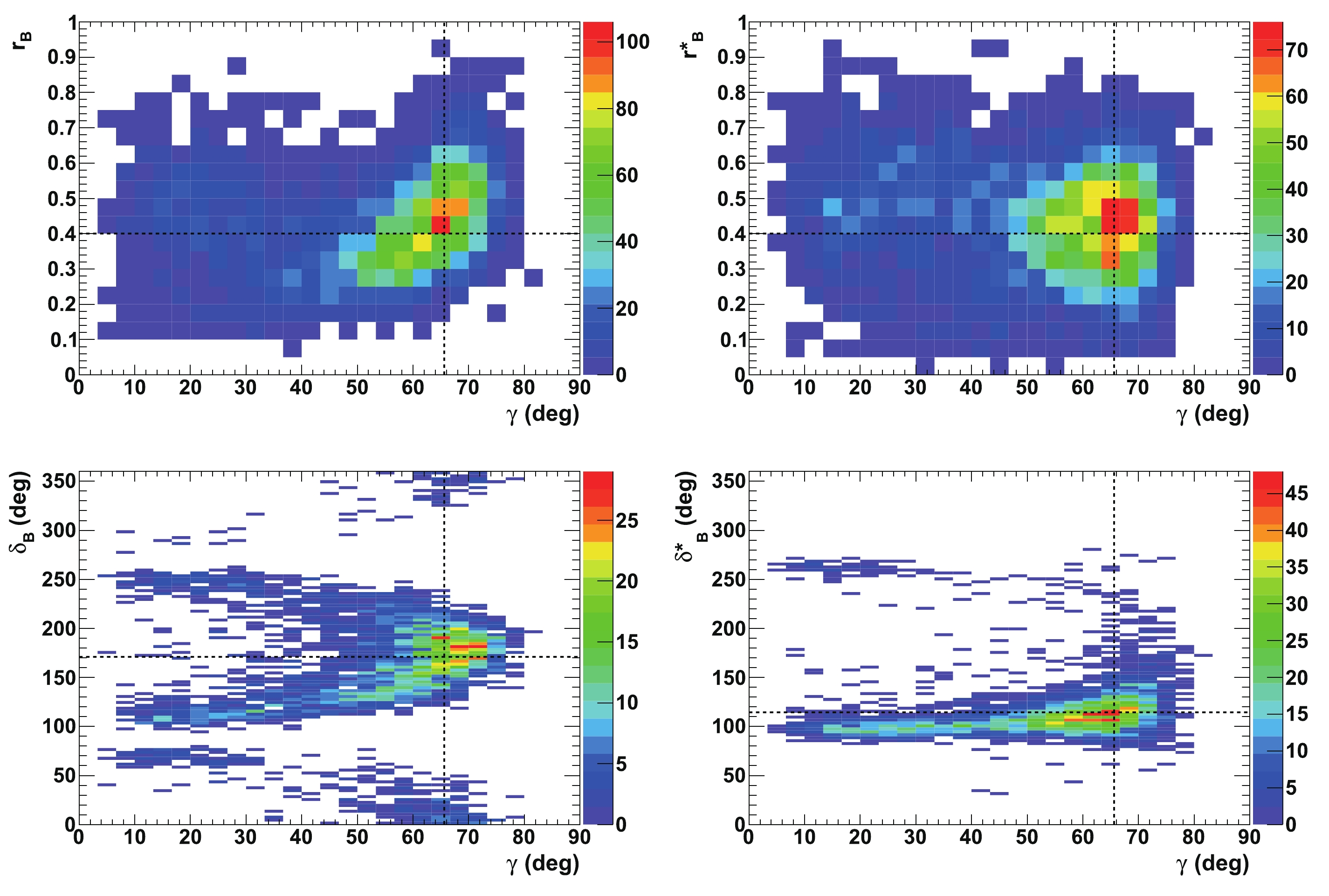
Figure 12. (color online) 2-D distributions of nuisance parameters
r(∗)B andδ(∗)B as a function ofγ obtained from 4000 pseudoexperiments. In each figure, the horizontal dashed black lines indicate the initial true values:γ=65.66∘ (1.146 rad),δB=171.9∘ (3.0 rad), andδ∗B=114.6∘ (2.0 rad), as well asr(∗)B=0.4 . -
According to Section IVA, 72 configurations of nuisance parameters
δ(∗)B andr(∗)B have been tested forγ=65.66∘ (1.146 rad) and 4000 pseudoexperiments have been generated for each set, following the procedure described in Section IVB and illustrated in Section IVF. The assumed integrated luminosity in this section is that of the LHCb data collected in Run 1 & 2.The fitted mean values of
γ (μγ ) forr(∗)B=0.4 and0.22 as a function ofδ(∗)B , for an initial true value of65.66∘ (1.146 rad), are displayed in Table 5, whereas the corresponding resolutions (σγ ) are listed in Table 6. In general, the fitted means are compatible with the trueγ values within less than one standard deviation. Forr(∗)B=0.4 , the resolution varies fromσγ=8.3∘ to12.9∘ . Forr(∗)B=0.22 , the resolution is worse, as expected, and it varies fromσγ=13.9∘ to18.7∘ . Forr(∗)B=0.22 , the distribution ofγ of the 4000 pseudoexperiments has its maximum above90∘ forδ(∗)B=286.5∘ and it is therefore not considered.δB 
δ∗B 
0 57.3 114.6 171.9 229.2 286.5 r(∗)B=0.4 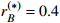
0.0 66.2±0.4 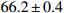
65.9±0.4 
67.4±0.4 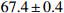
65.9±0.4 
65.9±0.4 
67.4±0.4 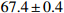
57.3 65.4±0.4 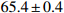
69.2±0.4 
71.4±0.4 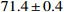
65.5±0.3 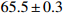
67.7±0.4 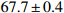
72.6±0.4 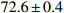
114.6 65.8±0.4 
70.5±0.4 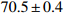
72.9±0.4 
65.9±0.3 
69.3±0.4 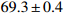
73.8±0.4 
171.9 65.6+0.5−0.4 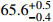
65.2±0.3 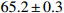
65.9±0.3 
65.5±0.3 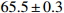
65.2±0.4 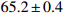
66.1±0.3 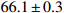
229.2 64.9±0.4 
67.6±0.4 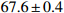
68.9±0.4 
65.0±0.4 
67.1±0.4 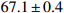
69.0±0.4 
286.5 66.1±0.4 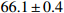
71.0±0.4 
75.7±0.4 
65.7±0.3 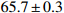
69.8±0.4 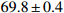
78.8±0.4 
δB 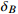
δ∗B 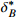
0 57.3 114.6 171.9 229.2 286.5 r(∗)B=0.22 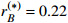
0.0 67.2+0.9−1.0 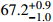
68.1+1.0−0.9 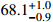
68.3+0.9−0.8 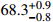
67.1±0.8 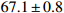
68.4±0.9 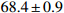
69.0±0.8 
57.3 67.4±0.9 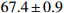
72.2±0.8 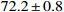
74.1+0.8−0.7 
66.6±0.7 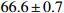
71.5±0.8 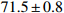
75.5±0.8 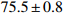
114.6 65.7±0.9 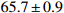
71.8±0.6 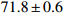
74.9±0.6 
68.0±0.6 
71.2±0.7 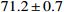
74.9±0.6 
117.9 65.4±0.7 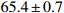
66.9±0.7 
66.6±0.7 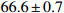
64.7±0.7 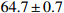
65.2±0.6 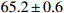
68.3±0.6 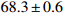
229.2 65.9+0.9−1.0 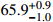
69.1±0.8 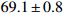
70.1+0.7−0.8 
67.7±0.7 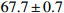
67.4±0.7 
71.0+0.6−0.7 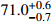
286.5 67.5±0.9 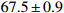
75.8±0.8 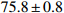
77.5+0.8−0.7 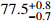
68.1±0.6 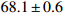
72.8+0.9−0.8 
83.5+2.4−1.5 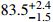
Table 5. Fitted mean values of
γ (μγ ) (in [deg]) forr(∗)B=0.4 (top) and0.22 (bottom), as a function ofδ(∗)B , for initial true value of65.66∘ .δB 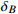
δ∗B 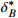
0 57.3 114.6 171.9 229.2 286.5 r(∗)B=0.4 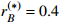
0.0 9.6±0.4 
11.2±0.3 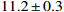
11.2±0.3 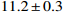
9.4±0.4 
12.2±0.4 
11.2±0.3 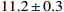
57.3 11.2±0.3 
12.6±0.3 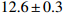
11.8±0.3 
10.2±0.3 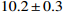
12.4±0.3 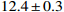
12.9±0.3 
114.6 11.4±0.3 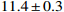
11.9±0.3 
11.1±0.3 
10.0±0.3 
11.3±0.3 
11.9±0.3 
171.9 8.3±0.4 
9.8±0.3 
8.8±0.2 
8.9±0.3 
9.5±0.3 
9.0±0.2 
229.2 10.8±0.3 
11.7±0.3 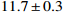
10.8±0.3 
10.3±0.3 
12.4±0.3 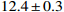
11.7±0.3 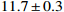
286.5 11.0±0.3 
12.9±0.3 
11.6±0.3 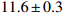
9.2±0.2 
11.7±0.3 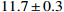
13.2+0.6−0.5 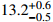
δB 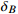
δ∗B 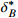
0 57.3 114.6 171.9 229.2 286.5 r(∗)B=0.22 
0.0 16.5±0.7 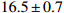
16.8±0.7 
16.0±0.6 
16.8+0.8−0.7 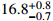
15.9+0.0−0.6 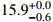
16.0±0.7 
57.3 16.7±0.6 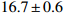
18.1+0.9−0.8 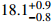
17.1+1.0−0.9 
14.3±0.5 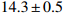
16.8±0.7 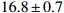
17.6+1.3−1.1 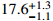
114.6 16.1+0.6−0.7 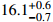
15.9±0.6 
13.9±0.5 
14.1±0.5 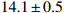
15.1±0.6 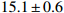
14.9±0.6 
171.9 15.7+0.7−0.6 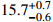
14.5±0.5 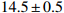
14.4±0.5 
15.5±0.6 
15.7+0.0−0.5 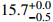
14.0±0.5 
229.2 15.9±0.6 
15.7+0.5−0.6 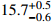
15.4±0.6 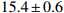
14.6+0.6−0.5 
15.6±0.5 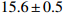
14.4±0.6 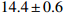
286.5 16.9+0.7−0.6 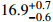
18.0+1.3−1.1 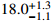
16.7+1.2−1.0 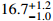
14.9+0.5−0.6 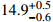
16.1±0.7 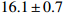
18.7+2.8−2.1 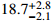
Table 6. Fitted resolution of
γ (σγ ) (in [deg]) forr(∗)B=0.4 (top) and0.22 (bottom), as a function ofδ(∗)B .The obtained values for
μγ andσγ are also displayed in Figs. 13 and 14. It is clear that the resolution onγ depends to the first order onr(∗)B , and then to the second order onδ(∗)B . The best agreement with respect to the tested initial true value ofγ is obtained whenδ(∗)B=0∘ (0 rad) or180∘ (π rad), and the best resolutions (the lowest values ofσγ ) are also obtained in this case. The largest CP violation effects and best sensitivity toγ are observed. In contrast, the worst sensitivity is obtained whenδ(∗)B=90∘ (π/2 rad) or270∘ (3π/2 rad). The other best and worst positions forδ(∗)B can easily be deduced from Eq. (14). In most cases, forr(∗)B=0.4 (0.22), the value of the resolution isσγ∼10∘ (15∘ ) and the fitted mean valueμγ∼65.66∘ or slightly larger.
Figure 13. (color online) Fitted mean values of
γ (μγ ) forr(∗)B=0.22 (red circles) and 0.4 (blue squares) as a function ofδ(∗)B for initial true value of65.66∘ (1.146 rad). All of the listed values are in [deg]. In each figure, the horizontal dashed black line indicates the initialγ true value. All of the plotted uncertainties are statistical only.
Figure 14. (color online) Fitted resolutions of
γ (σγ ), forr(∗)B=0.22 (red circles) and 0.4 (blue squares) as a function ofδ(∗)B for initial true value of65.66∘ (1.146 rad). All of the listed values are in [deg]. In each figure, the horizontal dashed black lines are guides for the eye atσγ=5∘ ,10∘ ,15∘ , and20∘ . All of the plotted uncertainties are statistical only.For completeness, the fitted means and resolutions for the nuisances parameters
r(∗)B andδ(∗)B are presented inFigs. A1-A4 in Appendix A. It can be observed that the fitted mean values ofr(∗)B andδ(∗)B are in good agreement with their initial tested true values, within one standard deviation of their fitted resolutions.
Figure A1. (color online) Fitted mean values of
rB (μrB ), forr(∗)B=0.22 (red circles) and 0.4 (blue squares) as a function ofδ(∗)B for initial true value ofγ of65.66∘ (1.146 rad). In each figure, the horizontal dashed black line indicates the initialrB true value, and the displayed uncertainties are the fitted resolutions onrB (i.e.σrB ).
Figure A2. (color online) Fitted mean values of
r∗B (μr∗B ), forr(∗)B=0.22 (red circles) and 0.4 (blue squares) as a function ofδ(∗)B for initial true value ofγ of65.66∘ (1.146 rad). In each figure, the horizontal dashed black line indicates the initialr∗B true value, and the displayed uncertainties are the fitted resolutions onr∗B (i.e.σr∗B ).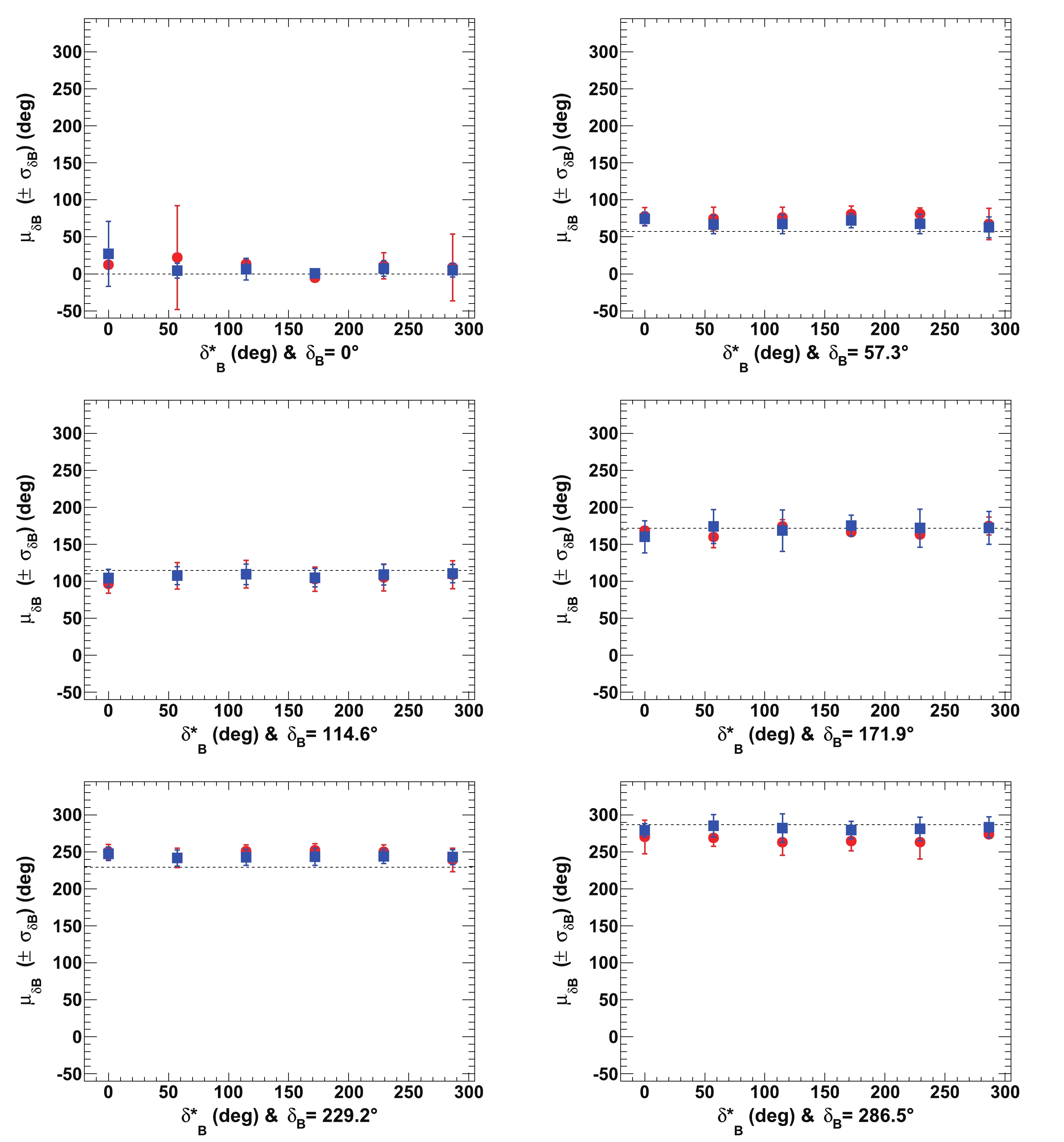
Figure A3. (color online) Fitted mean values of
δB (μδB ), forr(∗)B=0.22 (red circles) and 0.4 (blue squares) as a function ofδ(∗)B for initial true value ofγ of65.66∘ (1.146 rad). In each figure, the horizontal dashed black line indicates the initialδB true value, and the displayed uncertainties are the fitted resolutions onδB (i.e.σδB ).
Figure A4. (color online) Fitted mean values of
δ∗B (μδ∗B ), forr(∗)B=0.22 (red circles) and 0.4 (blue squares) as a function ofδ(∗)B for initial true value ofγ of65.66∘ (1.146 rad). In each figure, the dashed black line indicates the initialδ∗B true value, and the displayed uncertainties are the fitted resolutions onδ∗B (i.e.σδ∗B ). -
Configurations in which
γ=74∘ (see Ref. [12]) have also been tested. The potential problem in this case is that, as the true value ofγ is closer to the90∘ boundary, the unfolding of this parameter may become more difficult for many configurations of the nuisance parametersr(∗)B andδ(∗)B . It is clear from Eq. (14) that the sensitivity toγ is null at90∘ . This is illustrated in Fig. B1 in Appendix B, which can be compared with Fig. 11. In this case, the initial tested configuration isγ=74∘ ,r(∗)B=0.4 and 0.22,δB=171.9∘ (3 rad), andδ∗B=114.6∘ (2 rad). For these configurations,μγ=(73.7±0.3)∘ ((74.2±0.7)∘) andσγ=(7.7±0.3)∘ ((14.7±0.6)∘) forr(∗)B=0.4 (0.22). There is limited degradation of the resolution compared to the corresponding configuration when the true value ofγ is65.66∘ . The fit toγ for the pseudoexperiments corresponding to the configurationγ=74∘ ,r(∗)B=0.4 and 0.22,δB=57.3∘ (1 rad), andδ∗B=286.5∘ (5 rad) is presented in Fig. B2 in Appendix B. Forr(∗)B=0.22 , it can clearly be observed that the fittedγ value approaches the boundary limit of90∘ , and the corresponding resolution is approximately18∘ . Such behavior can be clearly understood from the 2-D distribution presented in Fig. 6. This is comparable to the case listed in Tables 5 and 6 whenδ(∗)B=286.5∘ (near3π/2 rad) andr(∗)B=0.22 .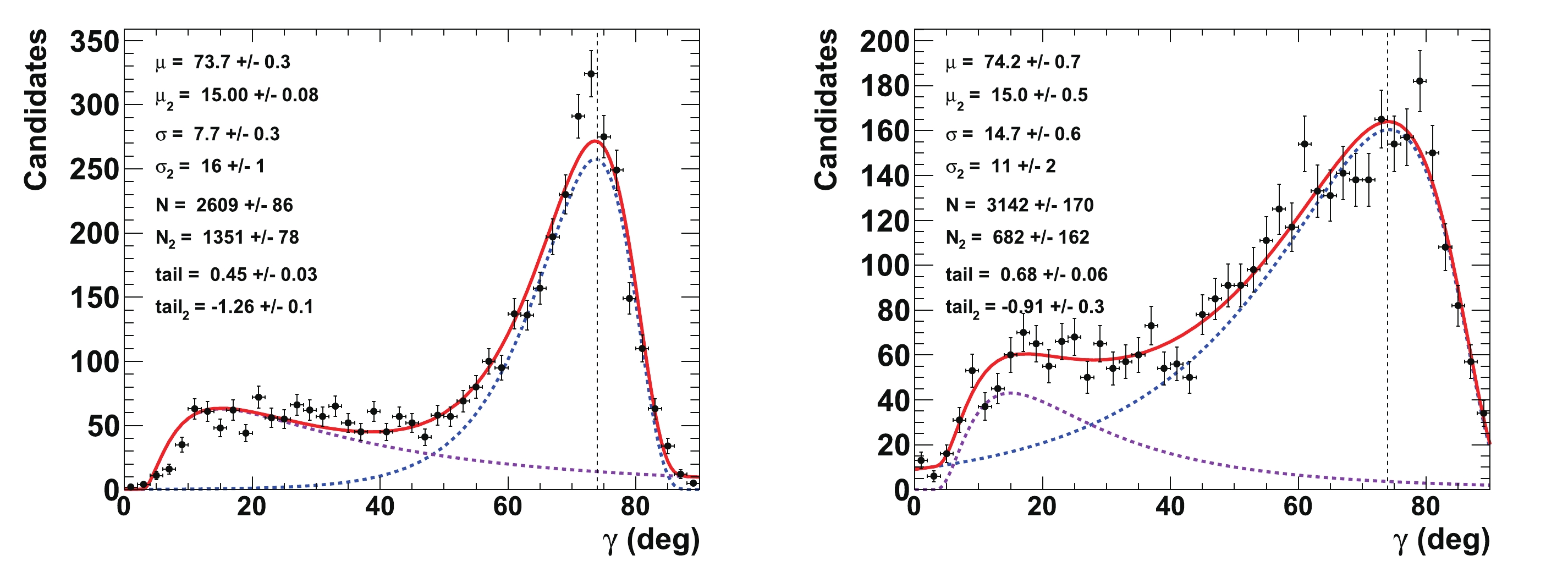
Figure B1. (color online) Fit to distributions of nuisance parameters
γ obtained from 4000 pseudoexperiments. The initial configuration isγ=74∘ ,r(∗)B=0.4 (left) and 0.22 (right),δB=171.9∘ (3 rad), andδ∗B=114.6∘ (2 rad). In the distributions, only the candidates with a value ofγ ∈ [0∘, 90∘] are considered. The purple dashed curve represents the tails generated by the correlations with the nuisance parametersr(∗)B andδ(∗)B , whereas the blue dashed curve indicates the core part of the distribution, and the plain red line is the sum of the two components of the fit.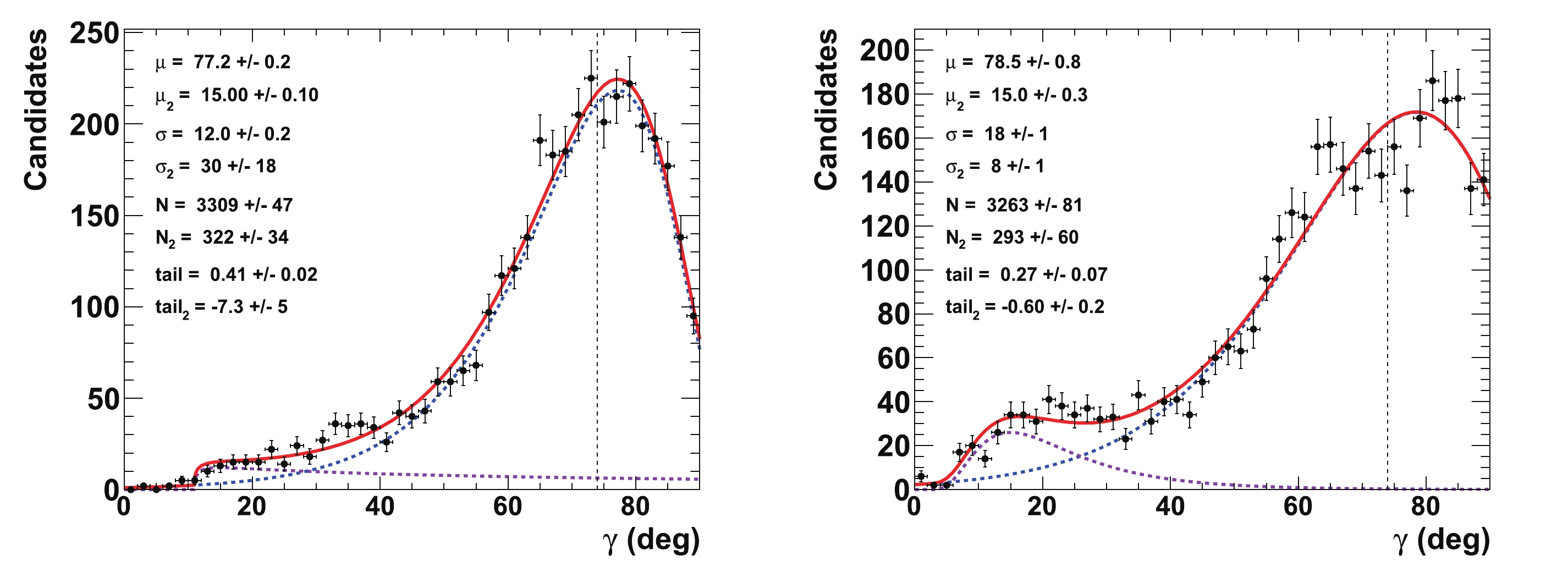
Figure B2. (color online) Fit to distributions of nuisance parameters
γ obtained from 4000 pseudoexperiments. The initial configuration isγ=74∘ ,r(∗)B=0.4 (left) and 0.22 (right),δB=57.9∘ (1 rad), andδ∗B=286.5∘ (5 rad). In the distributions, only the candidates with a value ofγ ∈ [0∘, 90∘] are considered. The purple dashed curve represents the tails generated by the correlations with the nuisance parametersr(∗)B andδ(∗)B , whereas the blue dashed curve indicates the core part of the distribution, and the plain red line is the sum of the two components of the fit. -
As listed in Table 2 the expected yields for the D-meson decays to
ππ andKππ0 are somewhat lower than those for the other modes, down to a few tens of events. Again, these yields have been computed from LHCb studies onB±→˜D0(π/K)± , as reported in Refs. [28] and [29], and normalized to Ref. [22] with respect to the modeB0s→˜D(∗)0(Kπ)ϕ . Therefore, the selections are not necessarily against the signalsB0s→˜D(∗)0(ππ)ϕ andB0s→˜D(∗)0(Kππ0)ϕ , and the expected yields may be underestimated, as well as all the sub-decays listed in Table 2. Furthermore, it should be noted that the modeππ is aCP -eigenstate, whereas theKππ0 3-body decay also has a large coherence factor valueRKππ0D=(81±6) % [36]. Nevertheless, the effect of using theB0s→˜D(∗)0(ππ)ϕ andB0s→˜D(∗)0(Kππ0)ϕ decays or not has been studied and is reported here, and in Section VC, the effect of including the decaysB0s→˜D∗0ϕ or not is also discussed for future more abundant datasets.According to Fig. C1 in Appendix C, there is a relative loss on the precision in the unfolded value of
γ of approximately3 to15 %, when theB0s→˜D(∗)0(ππ)ϕ decays are not used. Figure C2 in Appendix C indicates that a relative loss in precision of approximately3 to22 % is observed when theB0s→˜D(∗)0(Kππ0)ϕ decays are not used.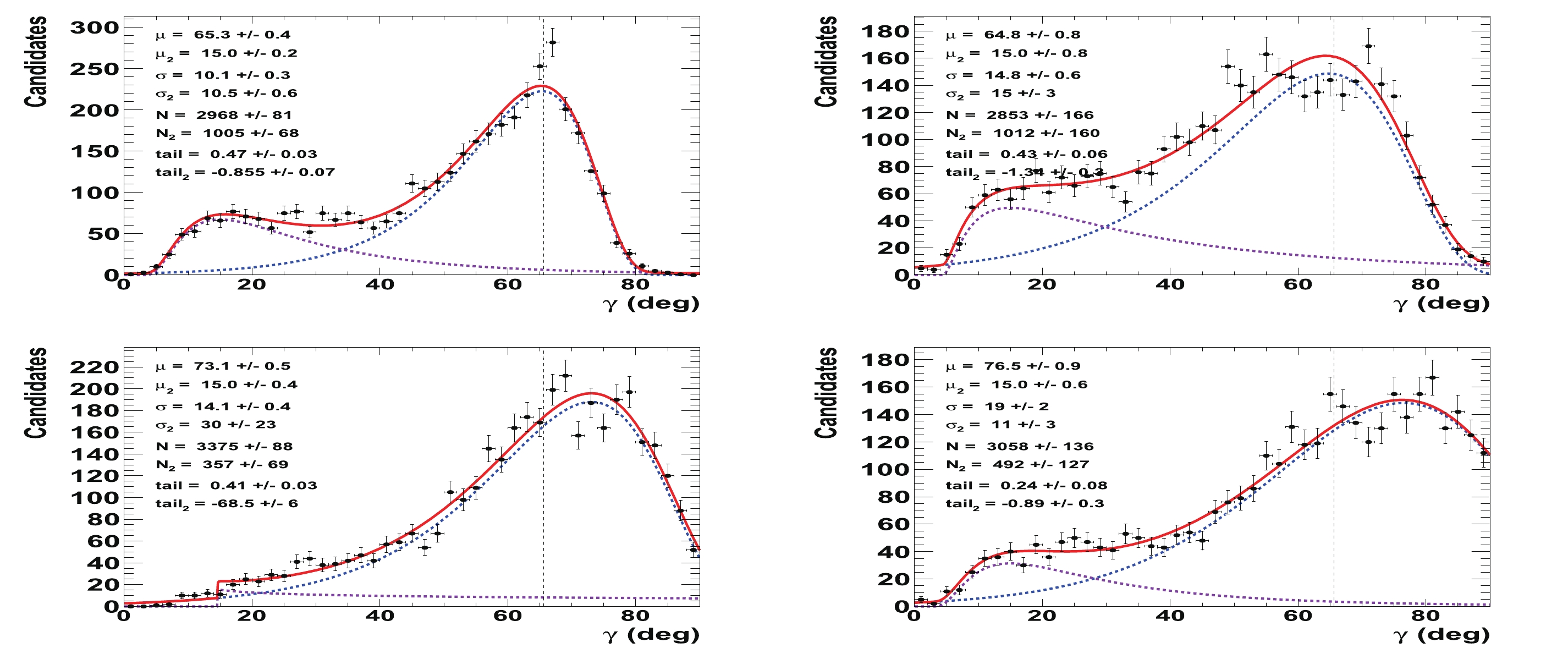
Figure C1. (color online) Fit to distributions of
γ obtained from 4000 pseudoexperiments for Run 1 & 2 LHCb dataset. The initial configuration isγ=65.66∘ ,rB=0.4 (left) and 0.22 (right), and (top)δB=171.9∘ (3 rad) andδ∗B=114.6∘ (2 rad) or (bottom)δB=57.3∘ (1 rad) andδ∗B=286.5∘ (5 rad) (w/oB0s→˜D(∗)0(ππ)ϕ ). The purple dashed curve represents the tails generated by the correlations with the nuisance parametersr(∗)B andδ(∗)B , whereas the blue dashed curve is the core part of the distribution, and the plain red line is the sum of the two components of the fit.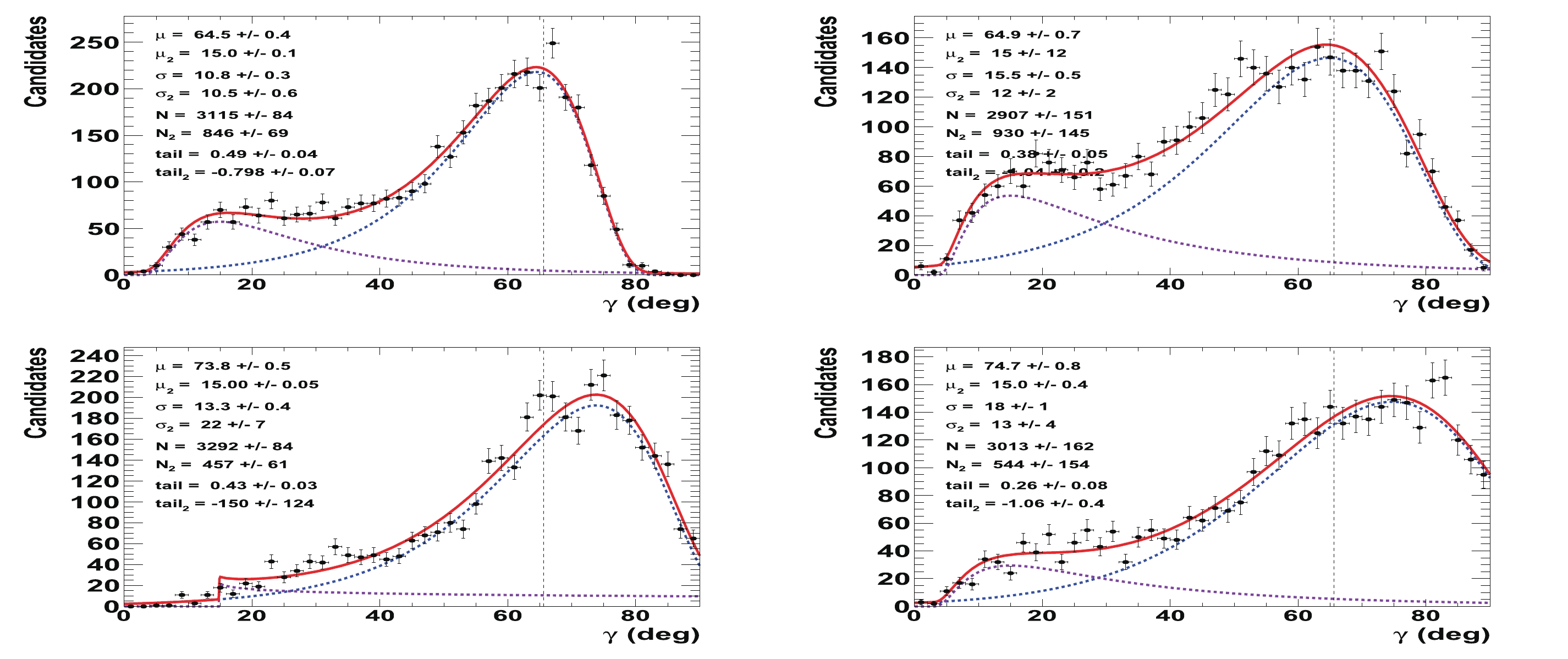
Figure C2. (color online) Fit to distributions of
γ obtained from 4000 pseudoexperiments for Run 1 & 2 LHCb dataset. The initial configuration isγ=65.66∘ ,rB=0.4 (left) and 0.22 (right), and (top)δB=171.9∘ (3 rad) andδ∗B=114.6∘ (2 rad) or (bottom)δB=57.3∘ (1 rad) andδ∗B=286.5∘ (5 rad) (w/oB0s→˜D(∗)0(Kππ0)ϕ ). The purple dashed curve represents the tails generated by the correlations with the nuisance parametersr(∗)B andδ(∗)B , whereas the blue dashed curve is the core part of the distribution, and the plain red line is the sum of the two components of the fit. -
The prospectives on the sensitivity to the CKM angle
γ withB0s→˜D(∗)0ϕ decays have also been studied for the foreseen LHCb integrated luminosities at the end of LHC Run 3 and for the possible full HL-LHC future LHCb program. According to Ref. [20], the LHCb trigger efficiency will be improved by a factor of 2 at the beginning of LHC Run 3. The full expected LHCb dataset ofpp collisions at√s=13 TeV corresponding to the sum of the Run 1, 2, and 3 LHCb dataset should be equal to 23 fb-1 by 2025, whereas it is expected to be 300 fb-1 by the second half of the 2030 decade. The final integrated LHCb luminosity accounts for an LHCb detector upgrade phase II. In the following, the projected event yields, as listed in Table 2, after 2025 and after 2038 have been scaled by a factorFlater=6.3 and90 , respectively, and with uncertainties on observables of1/√Flater . -
For this prospective sensitivity study, we have made the conservative assumption that the precision on the strong parameters of the D-meson decays to
Kπ ,K3π , andKππ0 listed in Table 3 should be improved by a factor of 2 at the end of the LHCb program (see the BES-III experiment prospectives [54]). The procedure described for the LHCb Run 1 & 2 data in Section IV has been repeated. The values of the normalization factorsCKπ ,CKπ,Dπ0 , andCKπ,Dγ obtained for Run 1 & 2 (see Section III) have been scaled to their expected equivalent rate for Runs 1 to 3 and full HL-LHC LHCb datasets. The statistical uncertainties of the computed observables (see Secttion IVC) obtained for the Run 1 & 2 LHCb data have been scaled by the square root of a factor two times (trigger improvement) the relative increase in the anticipated collectedB0s -meson yield: 2.2 (8.8) for the Run 1 to 3 (full HL-LHC) LHCb dataset. Thereafter, for the Run 1 & 2 sensitivity studies, the same2×6×6 configurations of ther(∗)B , andδ(∗)B nuisance parameters have been tested (r(∗)B=0.22 or 0.4 andδ(∗)B=0 , 1, 2, 3, 4, 5 rad, andγ=65.66∘ (1.146 rad)).The 2-D p-value distribution profiles of the nuisance parameters
r(∗)B andδ(∗)B as a function ofγ are presented in Figs. 15 and 16 for the expected Run1−3 LHCb dataset, and in Figs. 17 and 18 for the full HL-LHC LHCb dataset. For the purpose of these illustrations, the initial configuration of true values isγ=65.66∘ (1.146 rad),δB=171.9∘ (3.0 rad), andδ∗B=114.6∘ (2.0 rad), as well asr(∗)B=0.4 (0.22). Therefore, the distributions can be directly compared to those depicted in Figs. 7 and 8. The surface of the excluded regions at a 95.4% CL inr(∗)B vs. γ andδ(∗)B vs. γ clearly increases with the additional data, but even in the semi-asymptotic regime, for the full expected HL-LHC LHCb dataset, one can clearly observe possible strong correlations betweenγ and the nuisance parametersr(∗)B andδ(∗)B . This is also visible in Figs. D1 and D2 in Appendix D, which are the equivalent version for the full expected HL-LHC LHCb dataset of the Run 1 & 2 LHCb dataset presented in Figs. 5 and 6 for the configurationsγ=65.66∘ (1.146 rad),δB=57.3∘ (1.0 rad), andδ∗B=286.5∘ (5.0 rad), as well asr(∗)B=0.4 (0.22).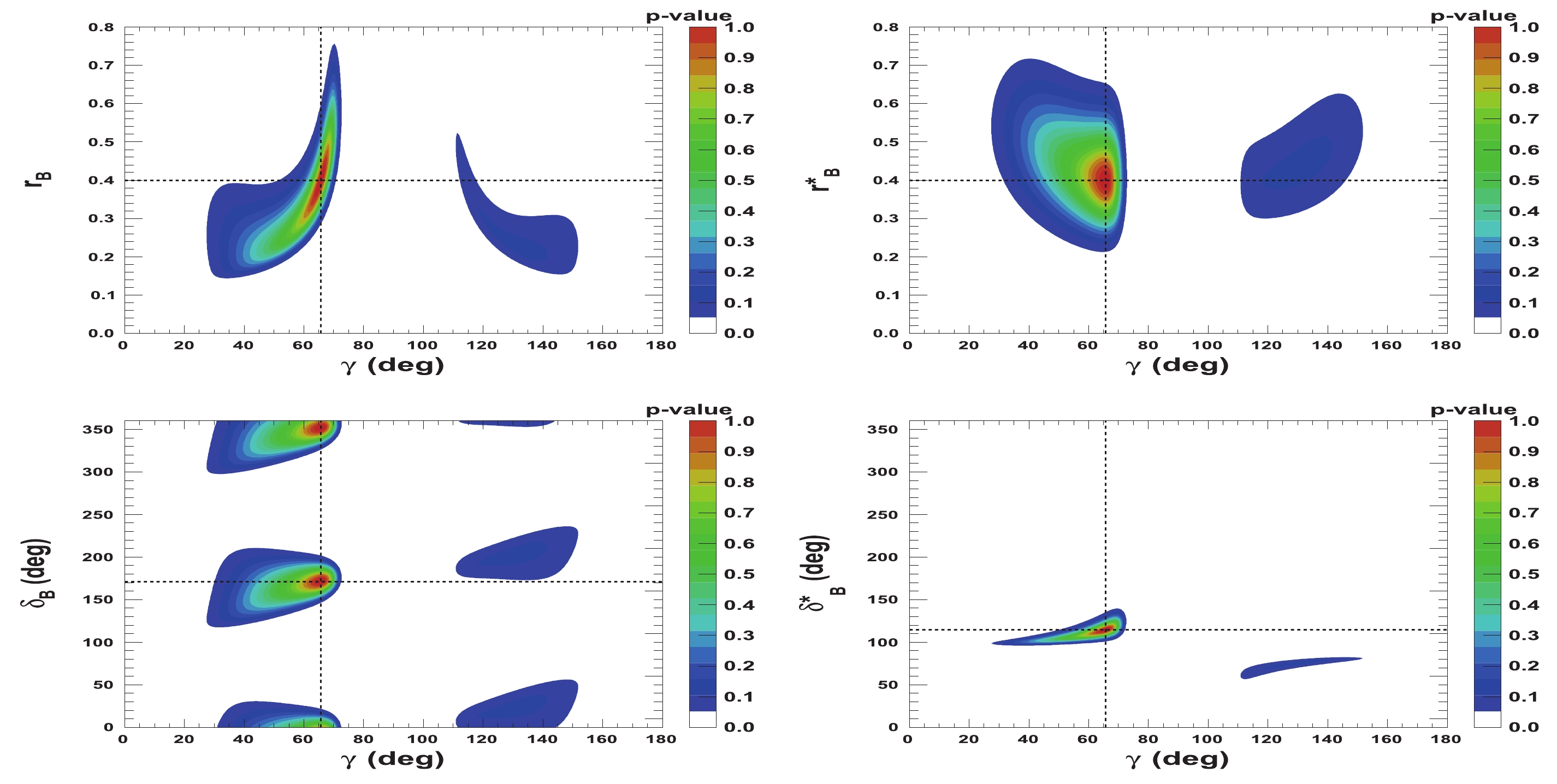
Figure 15. (color online) 2-D p-value profiles of nuisance parameters
r(∗)B andδ(∗)B (Runs1−3 ) as a function ofγ . In each figure, the dashed black lines indicate the initial true valuesγ=65.66∘ (1.146 rad),δB=171.9∘ (3.0 rad), andδ∗B=114.6∘ (2.0 rad), as well asr(∗)B=0.4 .
Figure 16. (color online) 2-D p-value profiles of nuisance parameters
r(∗)B andδ(∗)B for Run1−3 LHCb dataset as a function ofγ . In each figure, the dashed black lines indicate the initial true valuesγ=65.66∘ (1.146 rad),δB=171.9∘ (3.0 rad), andδ∗B=114.6∘ (2.0 rad), as well asr(∗)B=0.22 .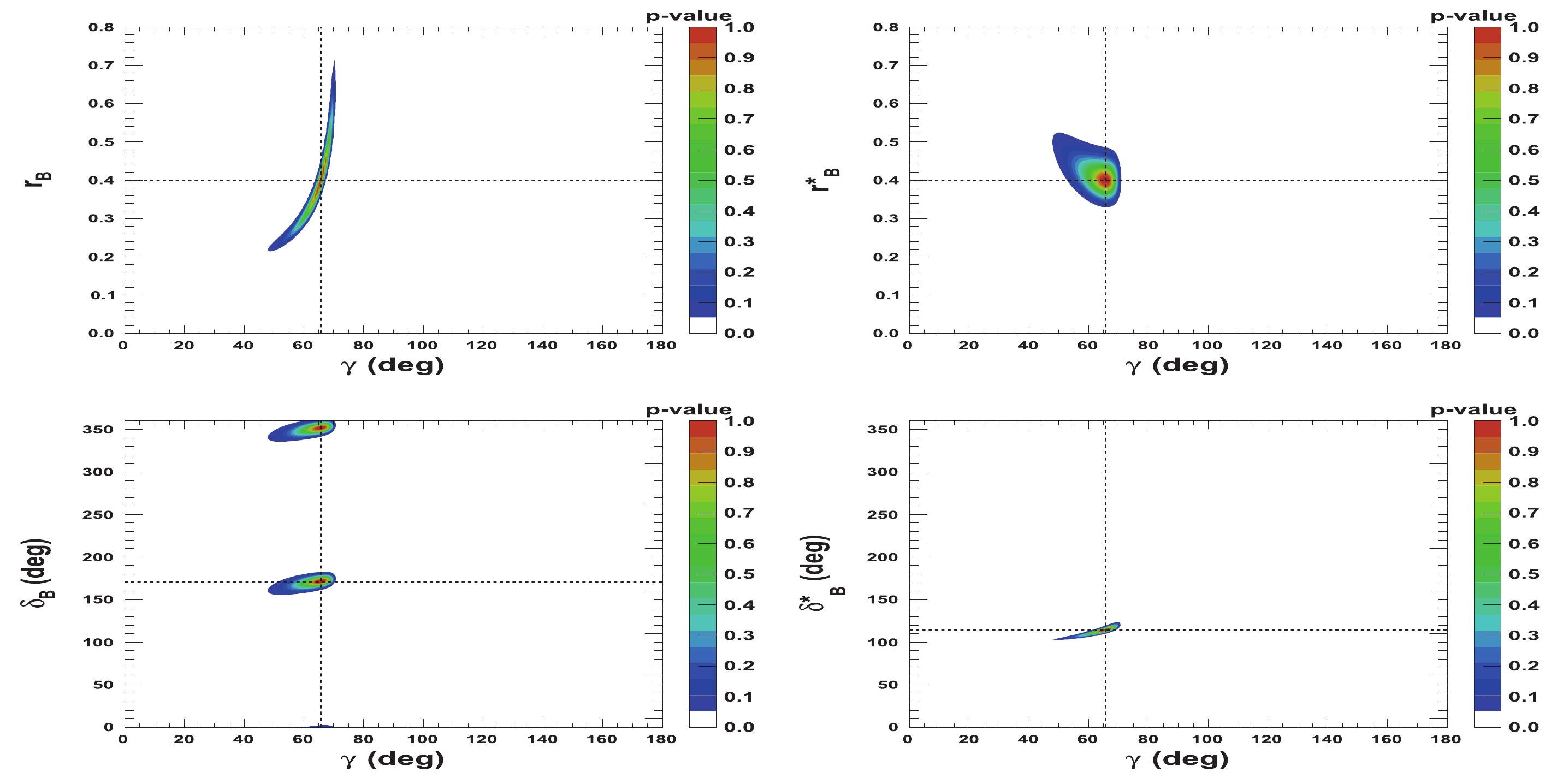
Figure 17. (color online) 2-D p-value profiles of nuisance parameters
r(∗)B andδ(∗)B for full HL-LHC LHCb dataset as a function ofγ . In each figure, the dashed black lines indicate the initial true valuesγ=65.66∘ (1.146 rad),δB=171.9∘ (3.0 rad), andδ∗B=114.6∘ (2.0 rad), as well asr(∗)B=0.4 .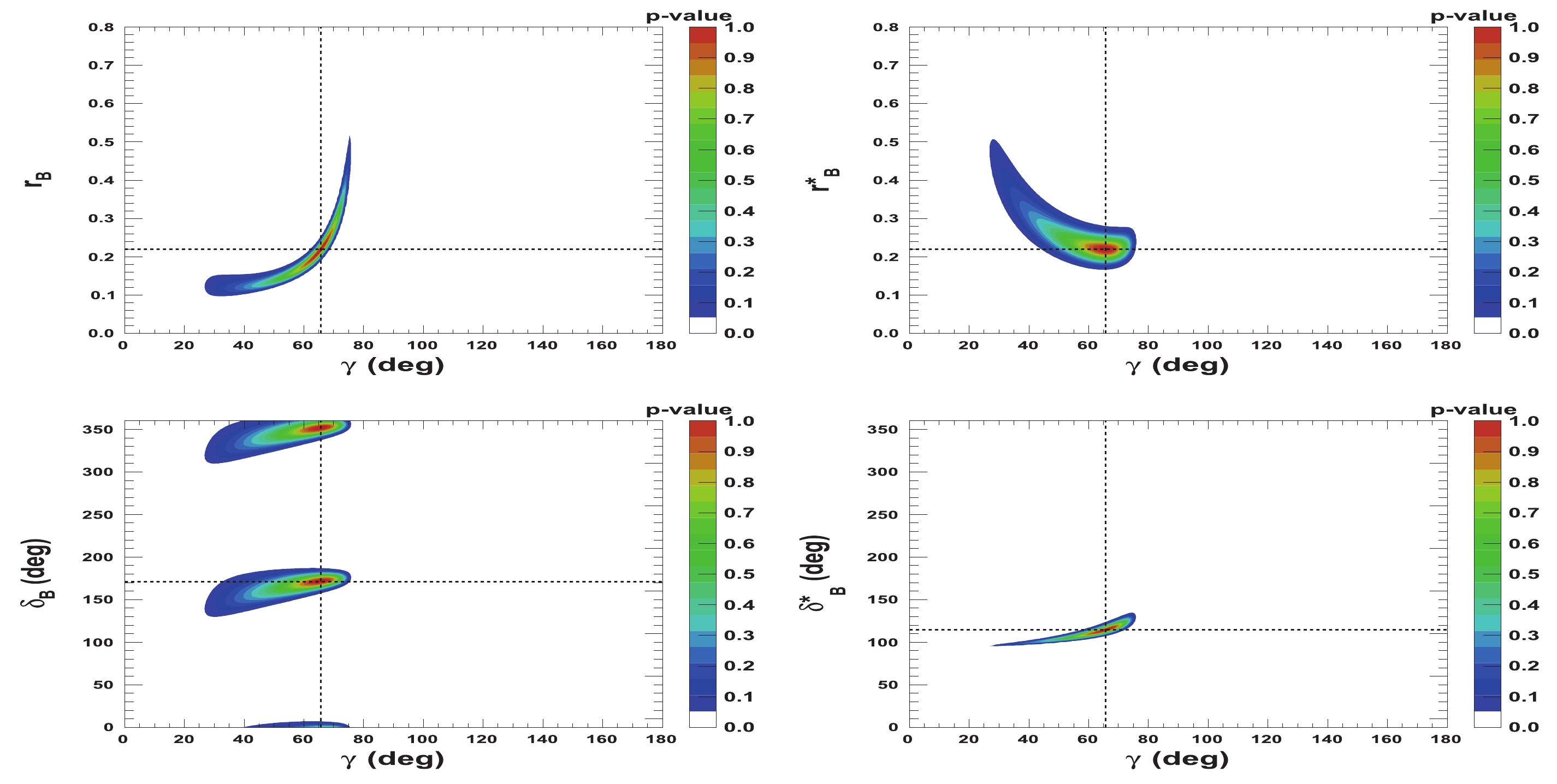
Figure 18. (color online) 2-D p-value profiles of nuisance parameters
r(∗)B andδ(∗)B for full HL-LHC LHCb dataset as a function ofγ . In each figure, the dashed black lines indicate the initial true valuesγ=65.66∘ (1.146 rad),δB=171.9∘ (3.0 rad), andδ∗B=114.6∘ (2.0 rad), as well asr(∗)B=0.22 .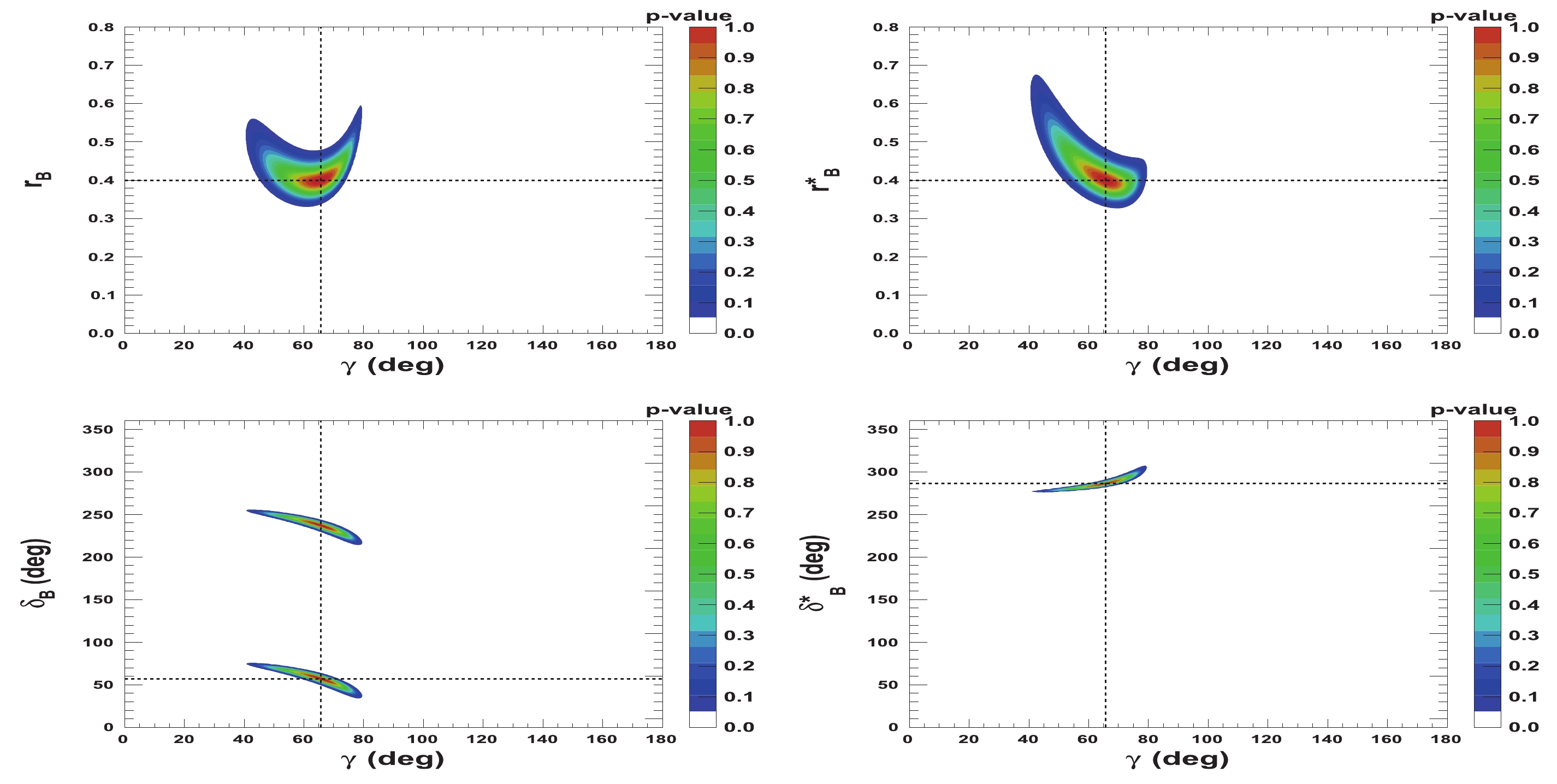
Figure D1. (color online) 2-D p-value profiles of nuisance parameters
r(∗)B andδ(∗)B for full HL-LHC LHCb dataset as a function ofγ . In each figure, the dashed black lines indicate the initialr(∗)B andδ(∗)B (γ ) true values:γ=65.66∘ (1.146 rad),δB=57.3∘ (1.0 rad), andδ∗B=286.5∘ (5.0 rad), as well asr(∗)B=0.4 .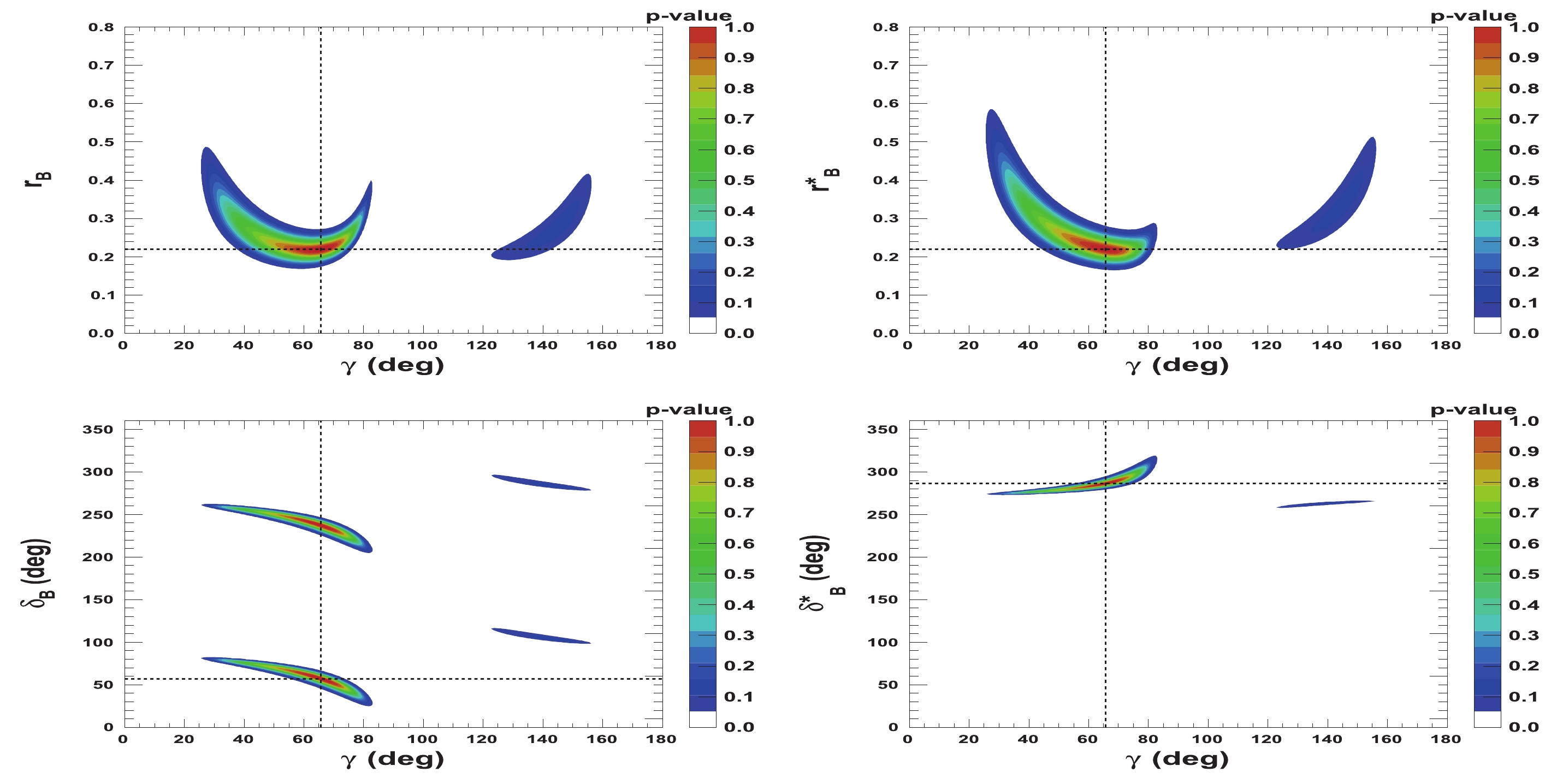
Figure D2. (color online) 2-D p-value profiles of nuisance parameters
r(∗)B andδ(∗)B for full HL-LHC LHCb dataset as a function ofγ . In each figure, the dashed black lines indicate the initial true valuesγ=65.66∘ (1.146 rad),δB=57.3∘ (1.0 rad), andδ∗B=286.5∘ (5.0 rad), as well asr(∗)B=0.22 .For the configuration
γ=65.66∘ (1.146 rad),δB= 171.9∘ (3.0 rad), andδ∗B=114.6∘ (2.0 rad), as well asr(∗)B=0.4 (0.22), Fig. 19 presents the fittedγ distribution obtained for 4000 pseudoexperiments for the expected Run1−3 LHCb dataset. The fitted values areμγ=(67.7±0.1)∘ ((73.5±0.2)∘) andσγ=(3.5±0.1)∘ ((5.5±0.2)∘) forr(∗)B=0.4 (0.22). The fitted values presented in Fig. 21 areμγ=(68.1± 0.1)∘ ((71.1±0.1)∘) andσγ=(2.5±0.1)∘ ((5.3±0.1)∘) forr(∗)B=0.4 (0.22), respectively, for the expected full HL-LHC LHCb dataset. The fitted values are slightly shifted up with respect to the initialγ true value but are compatible within one standard deviation. When comparing with the numbers listed in Table 6, it can be observed that the resolution improves as8.8/3.5=2.5 (14.4/5.5=2.6) forr(∗)B=0.4 (0.22) when moving from the Run 1 & 2 to the expected Run1−3 LHCb datasets, whereas a factor of 2.2 is expected. However, when moving from the expected Run1−3 to the full expected HL-LHC LHCb datasets, the improvement is only3.5/2.5=1.4 (5.5/5.3=1.040) forr(∗)B=0.4 (0.22), whereas one may naively expect an improvement8.8/2.2=4.0 . This is certainly partially owing to the strong correlations between the nuisance parametersr(∗)B andδ(∗)B . A more sophisticated simultaneous global fit to the nuisance parametersr(∗)B andδ(∗)B , andγ may be useful.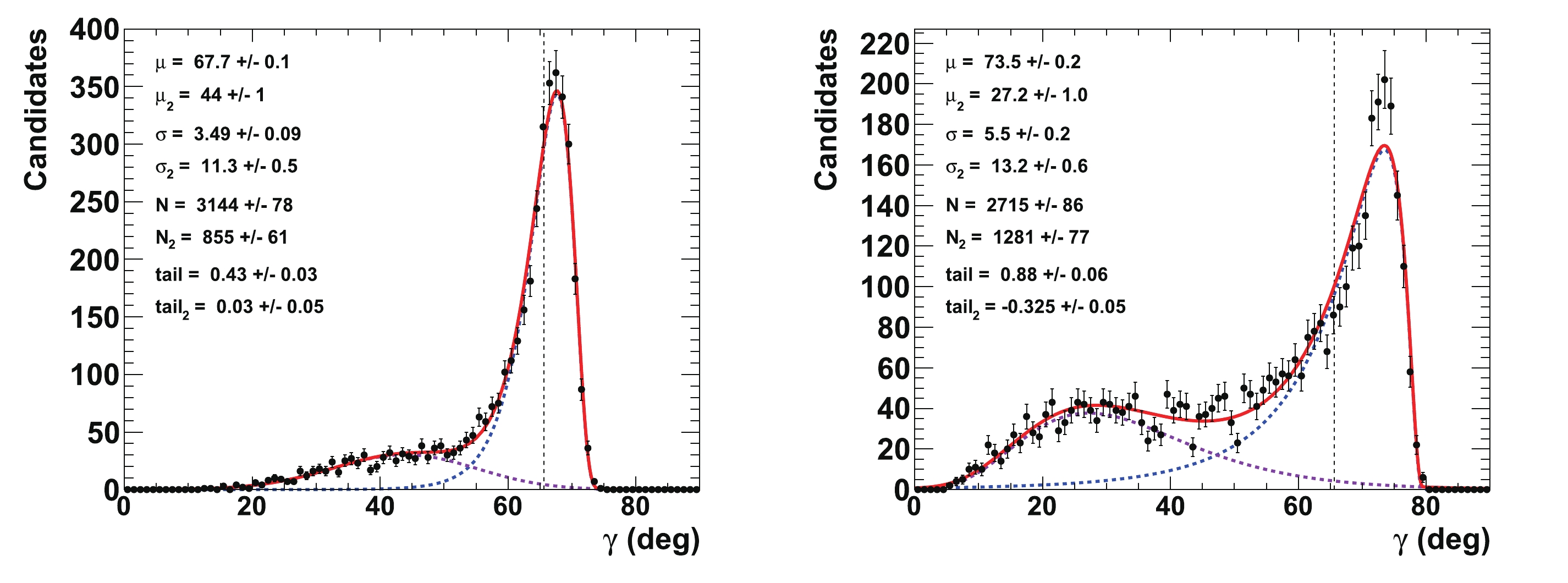
Figure 19. (color online) Fit to distributions of
γ obtained from 4000 pseudoexperiments for expected Run1−3 LHCb dataset. The initial configuration isγ=65.66∘ ,r(∗)B=0.4 (left) and 0.22 (right),δB=171.9∘ (3 rad), andδ∗B=114.6∘ (2 rad). The purple dashed curve represents the tails generated by the correlations with the nuisance parametersr(∗)B andδ(∗)B , whereas the blue dashed curve indicates the core part of the distribution, and the plain red line is the sum of the two components of the fit.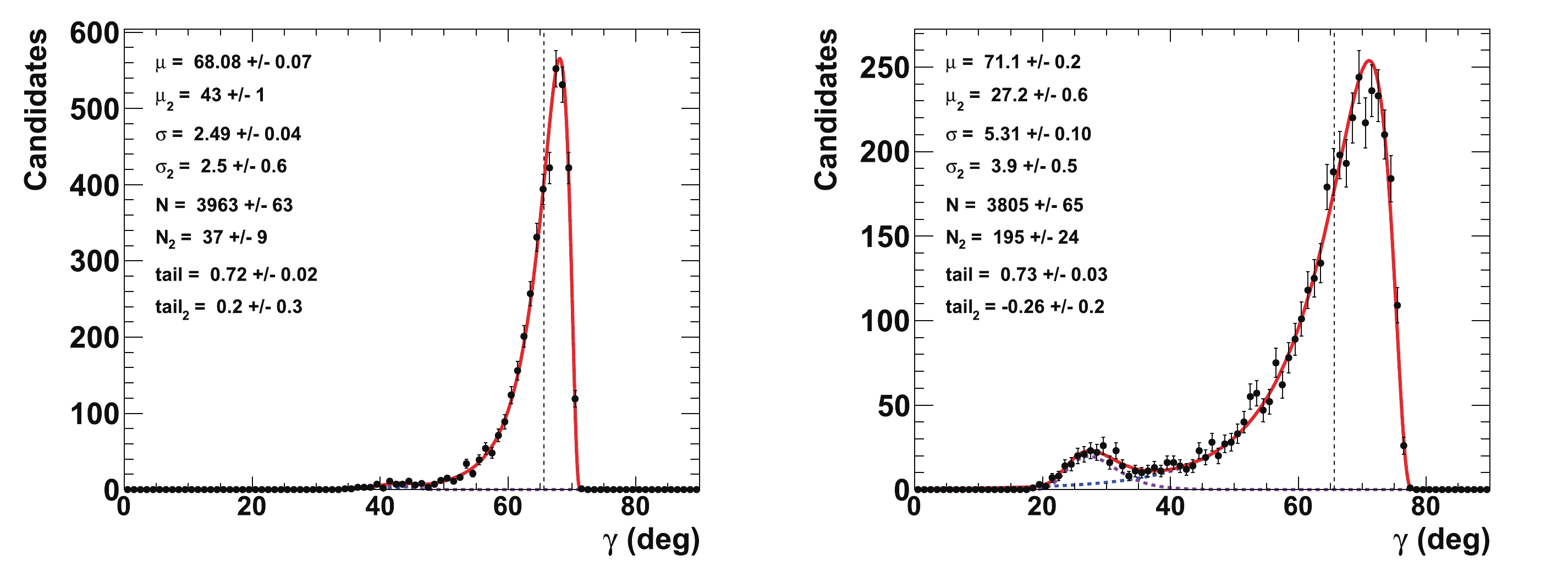
Figure 21. (color online) Fit to distributions of
γ obtained from 4000 pseudoexperiments for expected full HL-LHC LHCb dataset. The initial configuration isγ=65.66∘ ,r(∗)B=0.4 (left) and 0.22 (right),δB=171.9∘ (3 rad), andδ∗B=114.6∘ (2 rad). The purple dashed curve represents the tails generated by the correlations with the nuisance parametersr(∗)B andδ(∗)B , whereas the blue dashed curve is the core part of the distribution, and the plain red line is the sum of the two components of the fit.It must also be remembered that
TMath::Prob still exhibits some under-coverage, namely 79(91) % and 94(89) % forr(∗)B=0.4 (0.22), with respect to the full frequentist treatment on the Monte-Carlo simulation basis [50], as presented in Fig. 23, with the Run1−3 and full expected HL-LHC LHCb datasets, respectively. The relative scale factorsFK3π ,FKππ0 ,FKK , andFππ used in this study already have a precision above 2%. The precision on the normalization factorsCKπ ,CKπ,Dπ0 , andCKπ,Dγ may also benefit from another improved precision of the branching fraction of the decay modesB0s→¯D(∗)0ϕ and of the longitudinal polarization fraction in the modeB0s→¯D∗0ϕ . However, the normalization factors are the same for all sets of Eqs. (14)-(34) forB0s→˜D0ϕ or˜D∗0(π0, γ)ϕ , and their improved precision should be a second order effect. All of the above listed improvements are expected to occur to benefit from the total expected HL-LHC LHCb dataset fully.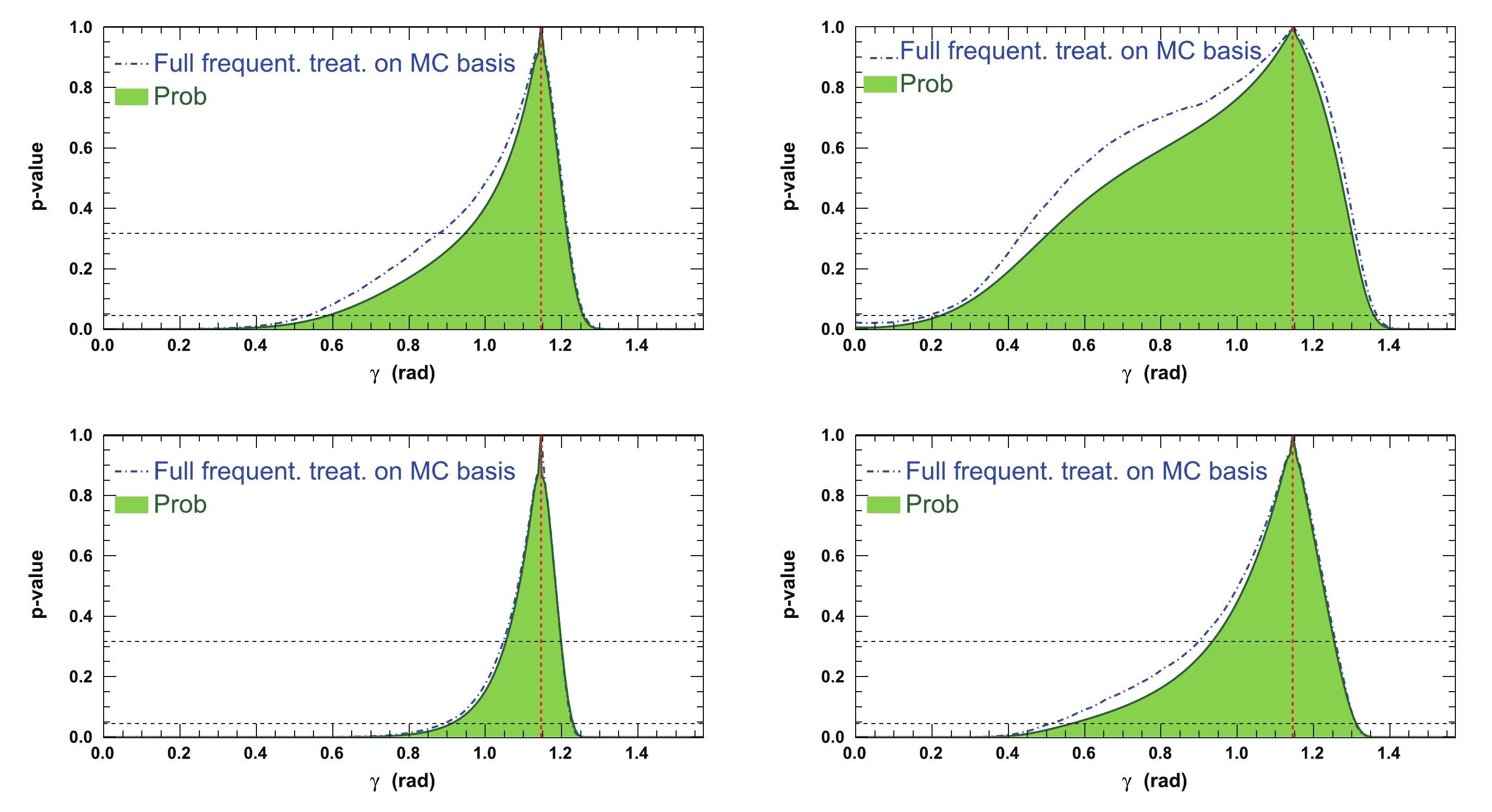
Figure 23. (color online) Profiles of p-value distributions of global
χ2 fit toγ for set of true initial parametersγ=1.146 rad,r(∗)B=0.4 (left)0.22 (right),δB=3.0 rad, andδ∗B=2.0 rad. The assumed integrated luminosity is that of the LHCb data expected to be collected after LHC Run 3 (top) and after the full HL-LHC (bottom) period. The corresponding distribution obtained from the full frequentist treatment on the Monte Carlo simulation basis [50] is superimposed on the profile obtained withTMath::Prob . In each figure, the vertical dashed red line indicates the initialγ true value, and the two horizontal dashed black lines refer to 68.3 and 95.4% CLs.The expected resolutions on
γ for the other usual configuration (δ(∗)B=0 , 1, 2, 3, 4, 5 rad, andγ=65.66∘ (1.146 rad)) are presented in Fig. 20 forr(∗)B=0.4 and in Fig. 22 forr(∗)B=0.22 , for the Run 1 & 2, Run1−3 , and full HL-LHC LHCb datasets. Forr(∗)B=0.4 , the resolution mainly ranges from3.4∘ to7.8∘ for Run1−3 and from2.2∘ to7.1∘ , or better, for the full HL-LHC dataset. Forr(∗)B=0.22 , the resolution ranges from5.5∘ to8.2∘ for Run1−3 and from3.3∘ to7.8∘ , or better, for the full HL-LHC dataset.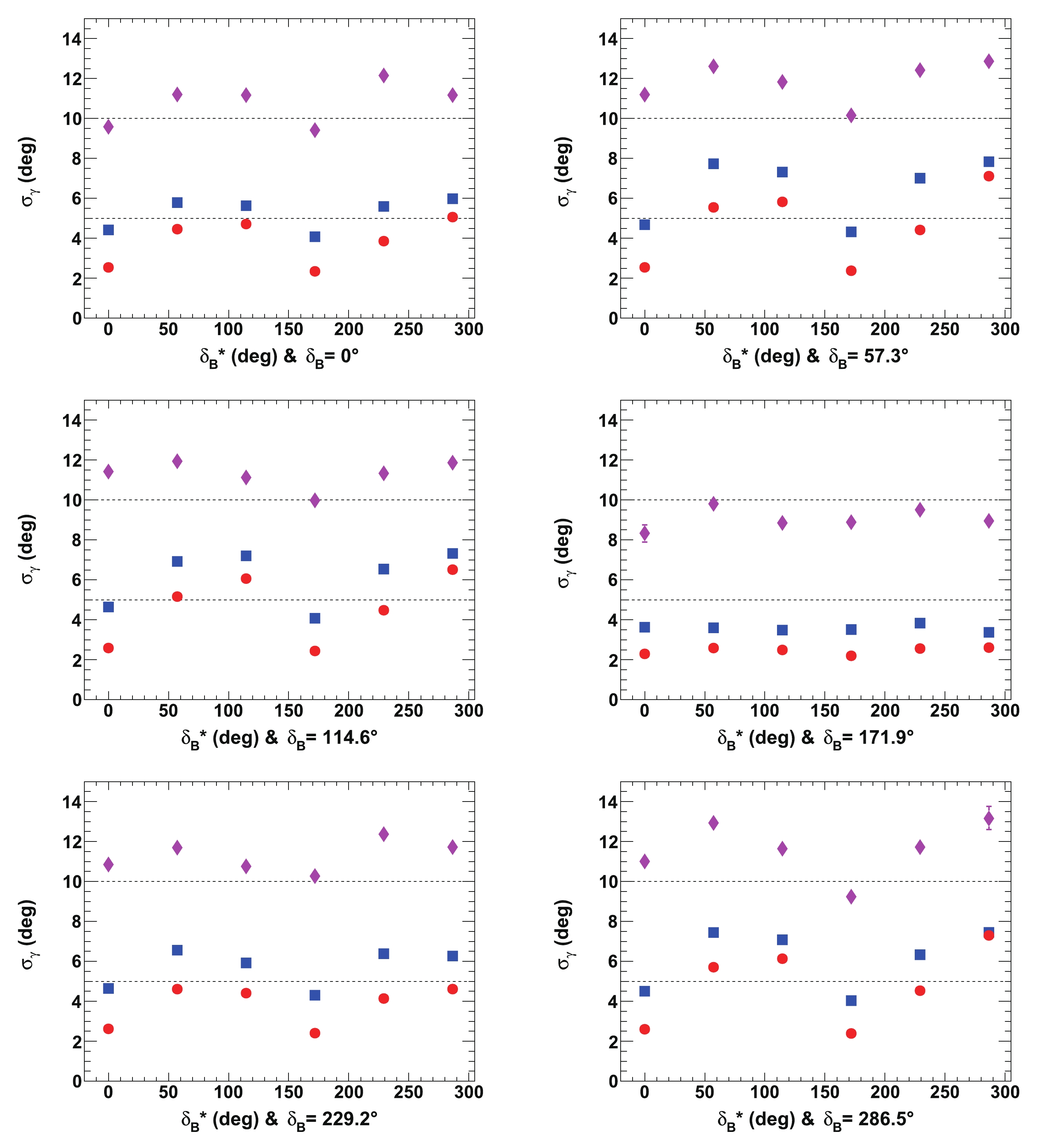
Figure 20. (color online) Fitted mean values of
γ (σγ ) for Run 1 & 2 (pink lozenges), Run1−3 (blue squares), and full HL-LHC (red circles) LHCb dataset as a function ofδ(∗)B , forr(∗)B=0.4 and initial true value of65.66∘ (1.146 rad). In each figure, the horizontal dashed black lines are guides for the eye atσγ=5∘ and10∘ .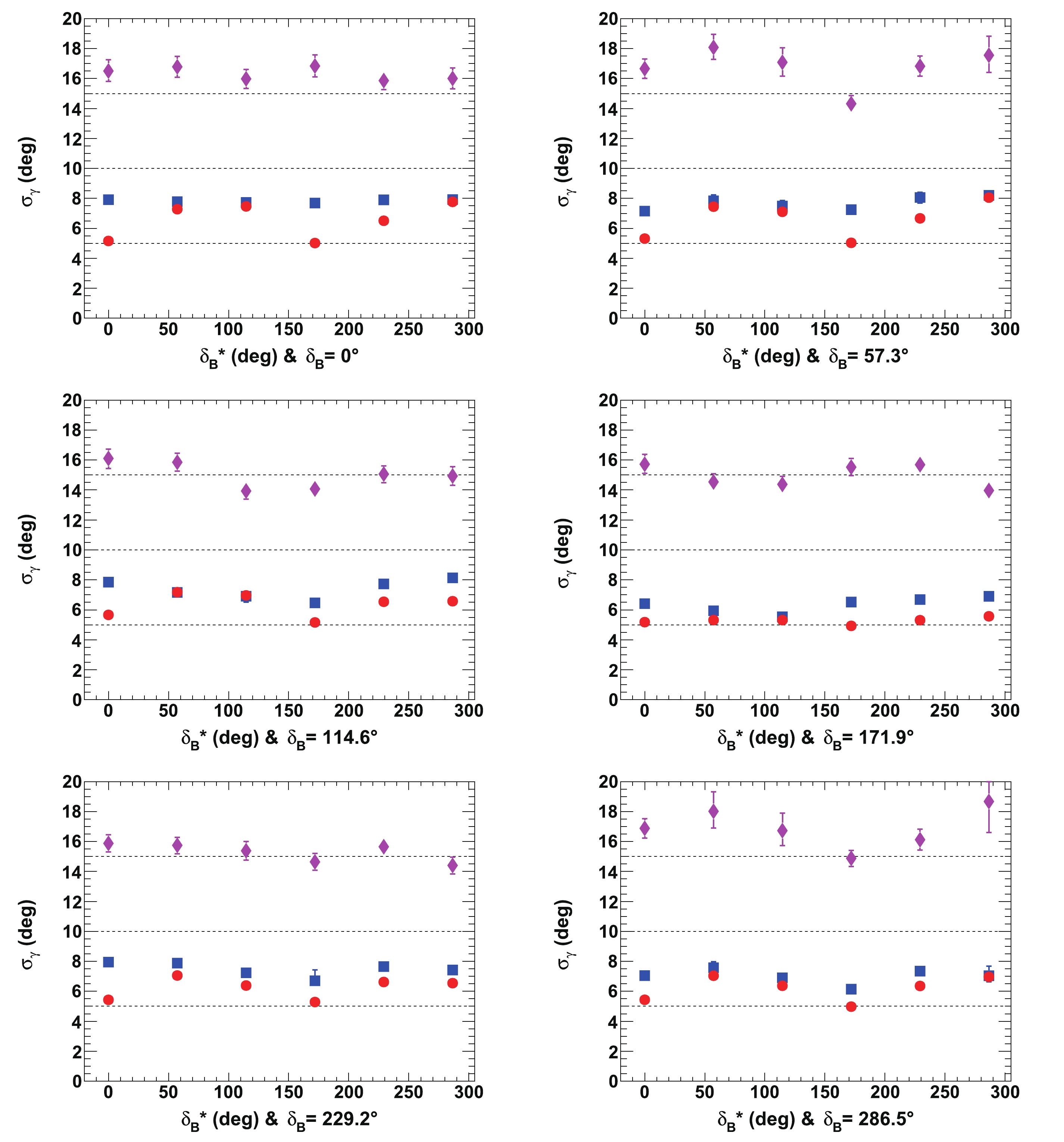
Figure 22. (color online) Fitted resolutions of
γ (σγ ) for Run 1 & 2 (pink lozenges), Run1−3 (blue squares), and full HL-LHC (red circles) LHCb dataset as a function ofδ(∗)B , forr(∗)B=0.22 and initial true value of65.66∘ (1.146 rad). In each figure, the horizontal dashed black lines are guides for the eye atσγ=5∘, 10∘, and15∘ .Another expected improvement could arise from a time-dependent CP Dalitz plane analysis of the decay
B0s→˜D(∗)0CPK+K− , as anticipated in Ref. [55]. With the ultimate HL-LHC LHCb dataset, it should be possible to perform such an analysis, thus including theB0s→˜D(∗)0ϕ decay, to extract the CKM angleγ , as proposed a few years ago in [56].For completeness, an alternate definition of the resolution as half of the 68.3% CL frequentist intervals of the 1-D p-value profiles of a 68.3% CL is provided in Appendix E in Figs. E1 and E2. A better scaling of the performances is observed with the size of the datasets, whereas relatively worse resolutions are obtained with respect to those displayed in Figs. 20 and 22. However, the effects of the nuisance parameters
r(∗)B andδ(∗)B are treated in a simplified manner compared to the full treatment by the generated pseudoexperiments.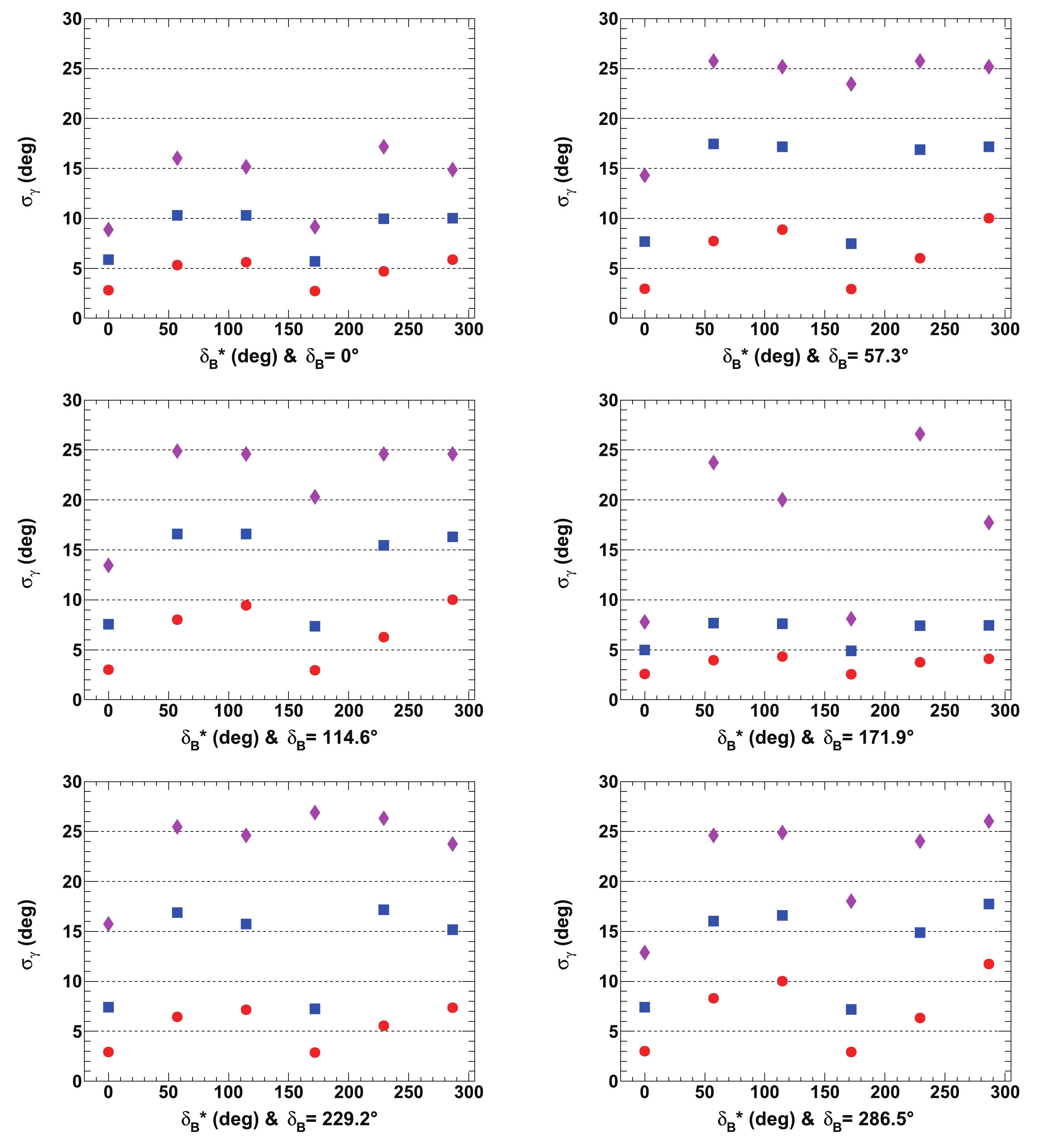
Figure E1. (color online) Half of 68.3% CL intervals of 1-D p-value profiles of
γ for Run 1 & 2 (pink lozenges), Run1−3 (blue squares), and full HL-LHC (red circles) LHCb dataset as a function ofδ(∗)B , forr(∗)B=0.4 and initial true value of65.66∘ . In each figure, the horizontal dashed black lines are guides for the eye.
Figure E2. (color online) Half of 68.3% CL intervals of 1-D p-value profiles of
γ for Run 1 & 2 (pink lozenges), Run1−3 (blue squares), and full HL-LHC (red circles) LHCb dataset as a function ofδ(∗)B , forr(∗)B=0.22 and initial true value of65.66∘ . In each figure, the horizontal dashed black lines are guides for the eye. -
Most of the strong parameters of the D-meson decays to
Kπ ,K3π , andKππ0 are external parameters and are obtained from beauty- or charm-factories, such as BaBar, Belle, CLEO-c, and LHCb [27]. Improvements in their determination are expected soon from the updated BES-III experiment [50] or later, from future superτ -charm factories [57-59]. Several scenarios have been tested to verify the effects of these improvements to theγ sensitivity. With the set of parametersγ=1.146 rad (65.66∘ ),r(∗)B=0.4 , andδB=3.0 rad, as well asδ∗B=2.0 , the uncertainties of the current measurements of the D-meson parameters listed in Table 3 have been scaled down, and their effects on the fittedγ values from pseudoexperiments are listed in Table 7. As the uncertainties of the external parameters are not yet dominant (Run 1 & 2 data), a study has also been performed for the expected full HL-LHC dataset. However, with substantially more data, future improvements in the measurements of the strong parameters from the D-meson decays do not appear to influence the sensitivity to the CKM angleγ significantly.Uncertainties of D-meson params. Now ×1/2 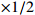
×1/5 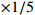
×1/10 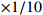
Run 1 & 2 ( r(∗)B=0.4 )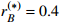
8.8±0.2 
8.1±0.3 
8.0±0.3 
7.8±0.2 
Run 1 & 2 ( r(∗)B=0.22 )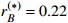
12.9±0.3 
13.2±0.5 
13.1±0.5 
12.8±0.9 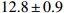
Full HL-LHC ( r(∗)B=0.4 )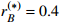
2.6±0.1 
2.5±0.1 
2.5±0.1 
2.5±0.1 
Full HL-LHC ( r(∗)B=0.22 )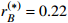
5.4±0.1 
5.3±0.1 
5.2±0.1 
5.1±0.1 
Table 7. Fitted resolutions of
γ (σγ ) in [deg] obtained from 4000 pseudoexperiments as a function of decreasing uncertainties of strong D-meson parameters (see Table 3).This exercise was repeated using the same initial configuration of the parameters
γ ,r(∗)B , andδ(∗)B , for the uncertainty ony=ΔΓs/2Γs . The results of this study are listed in Table 8, Again, no obvious sensitivity to these changes is highlighted, neither for Run 1 & 2 nor for the full HL-LHC dataset. To our knowledge, it should be stressed that the tested improvements in y have not been supported by any published prospective studies.Uncertainty on y=ΔΓs/2Γs 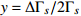
Now ×1/2 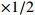
×1/5 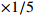
×1/10 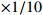
Run 1 & 2 ( r(∗)B=0.4 )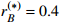
8.8±0.2 
8.3±0.2 
8.2±0.2 
8.1±0.3 
Run 1 & 2 ( r(∗)B=0.22 )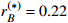
12.9±0.3 
12.6±0.4 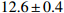
12.5±0.5 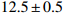
12.5±0.5 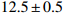
Full HL-LHC ( r(∗)B=0.4 )
2.5±0.1 
2.5±0.1 
2.5±0.1 
2.5±0.1 
Full HL-LHC ( r(∗)B=0.22 )
5.3±0.1 
5.3±0.1 
5.2±0.1 
5.2±0.1 
Table 8. Fitted resolutions of
γ (σγ ) in [deg] obtained from 4000 pseudoexperiments as function of decreasing uncertainties ofy=ΔΓs/2Γs . For the full HL-LHC dataset, the uncertainties for the strong D-meson parameters are divided by 2 with respect to the current measurements (see Table 3).From the above studies, it can be concluded that the possibly large correlations of
γ with respect to the nuisances parametersr(∗)B andδ(∗)B definitely dominate the ultimate precision onγ for the extraction with theB0s→˜D(∗)0ϕ modes. -
It has been demonstrated in Ref. [22] that the decays
B0s→˜D∗0ϕ can be reconstructed in a clean manner together withB0s→˜D0ϕ , with a similar rate and a partial reconstruction method, where theγ orπ0 produced in the decay of˜D∗0 are omitted. Thus far, these modes have been included in the sensitivity studies. Figures F1-F6 in Appendix F present the 2-D p-value profiles of the nuisance parametersrB andδB as a function ofγ as well as the fit to the distributions ofγ obtained from 4000 pseudoexperiments for the Run 1 & 2, Run1−3 , and full HL-LHC LHCb datasets for the initial true valuesγ=65.66∘ (1.146 rad),δB=171.9∘ (3.0 rad),δB=114.6∘ (2.0 rad), andr(∗)B=0.4 (0.22). The information fromB0s→˜D∗0ϕ decays has not been included in these figures. According to Figs. F2, F4, and F6 in Appendix F, there is a relative loss in the precision of the unfolded value ofγ of approximately 20 (40%) when theB0s→˜D∗0ϕ decays are not used forr(∗)B=0.4 (0.22). For future datasets, the improvement obtained by including theB0s→˜D∗0ϕ modes is less significant, but not negligible, and aids in improving the measurement ofγ .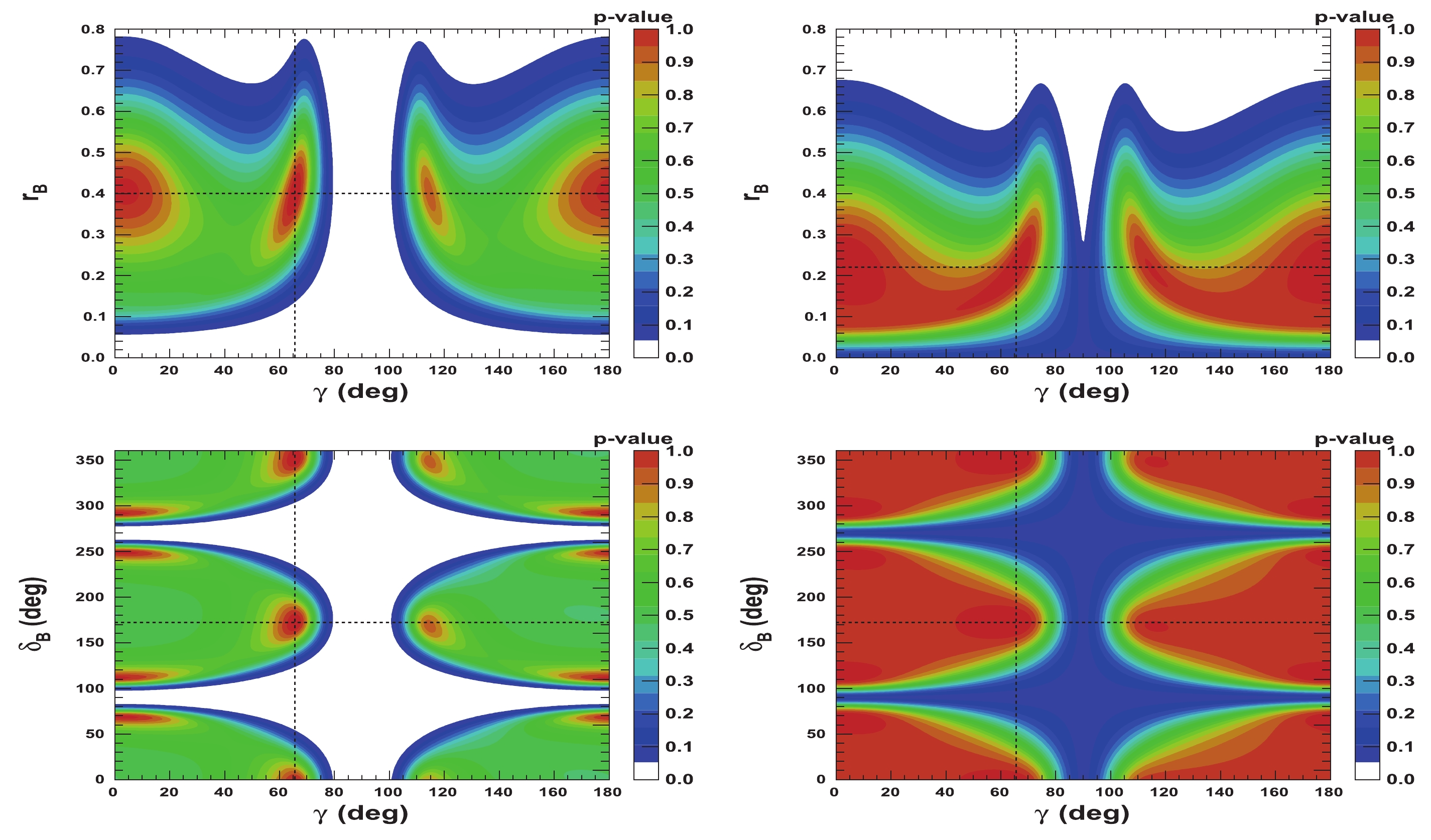
Figure F1. (color online) 2-D p-value profiles of nuisance parameters
rB andδB , for the Run 1 & 2 LHCb dataset, as a function ofγ . In each figure, the dashed black lines indicate the initial true valuesγ=65.66∘ (1.146 rad),δB=171.9∘ (3.0 rad), andrB=0.4 (left) and 0.22 (right) (w/oB0s→D∗0ϕ ).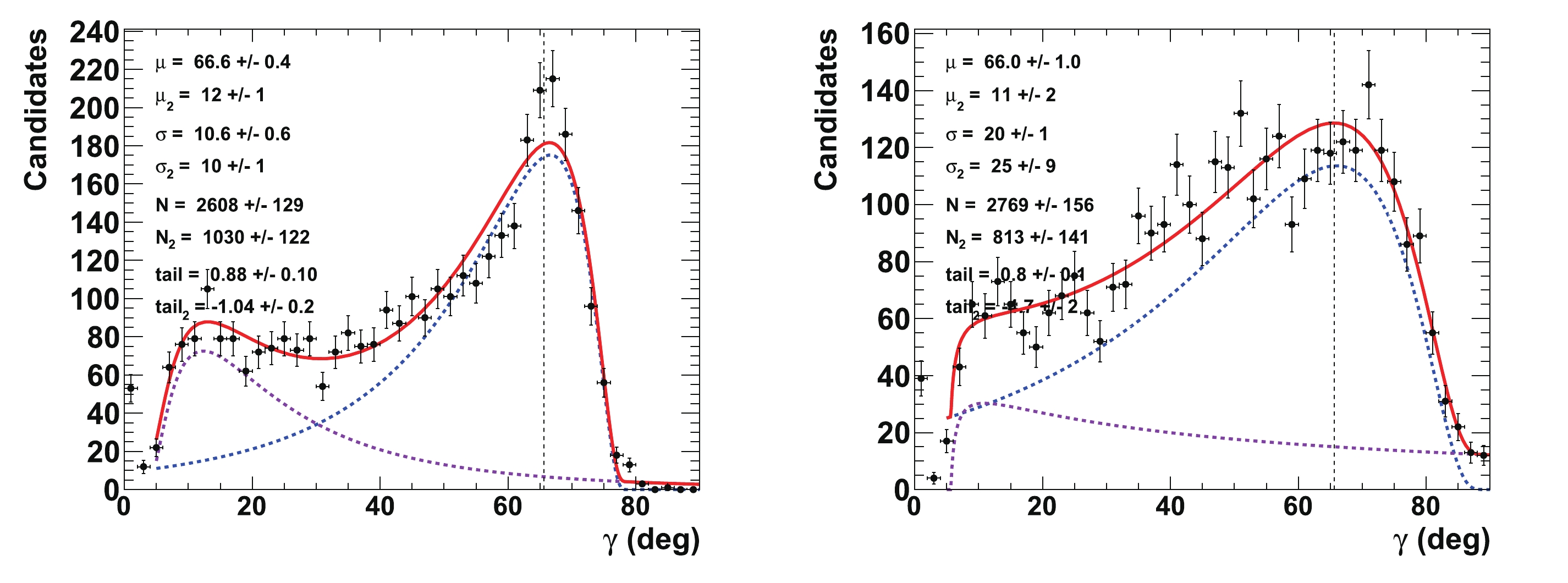
Figure F2. (color online) Fit to distributions of
γ obtained from 4000 pseudoexperiments for Run 1 & 2 LHCb dataset. The initial configuration isγ=65.66∘ ,rB=0.4 (left) and 0.22 (right), andδB=171.9∘ (3 rad) (w/oB0s→D∗0ϕ ). The purple dashed curve represents the tails generated by the correlations with the nuisance parametersr(∗)B andδ(∗)B , whereas the blue dashed curve indicates the core part of the distribution, and the plain red line is the sum of the two components of the fit.
Figure F3. (color online) 2-D p-value profiles of nuisance parameters
rB andδB (Run1−3 LHCb) as function ofγ . In each figure, the dashed black lines indicate the initial true valuesγ=65.66∘ (1.146 rad),δB=171.9∘ (3.0 rad), andδ∗B=114.6∘ (2.0 rad), as well asrB=0.4 (left) and 0.22 (right) (w/oB0s→D∗0ϕ ).
Figure F4. (color online) Fit to distributions of
γ obtained from 4000 pseudoexperiments (Run1−3 LHCb). The initial configuration isγ=65.66∘ ,rB=0.4 (left) and 0.22 (right), andδB=171.9∘ (3 rad) (w/oB0s→D∗0ϕ ). The purple dashed curve represents the tails generated by the correlations with the nuisance parametersr(∗)B andδ(∗)B , whereas the blue dashed curve indicates the core part of the distribution, and the plain red line is the sum of the two components of the fit.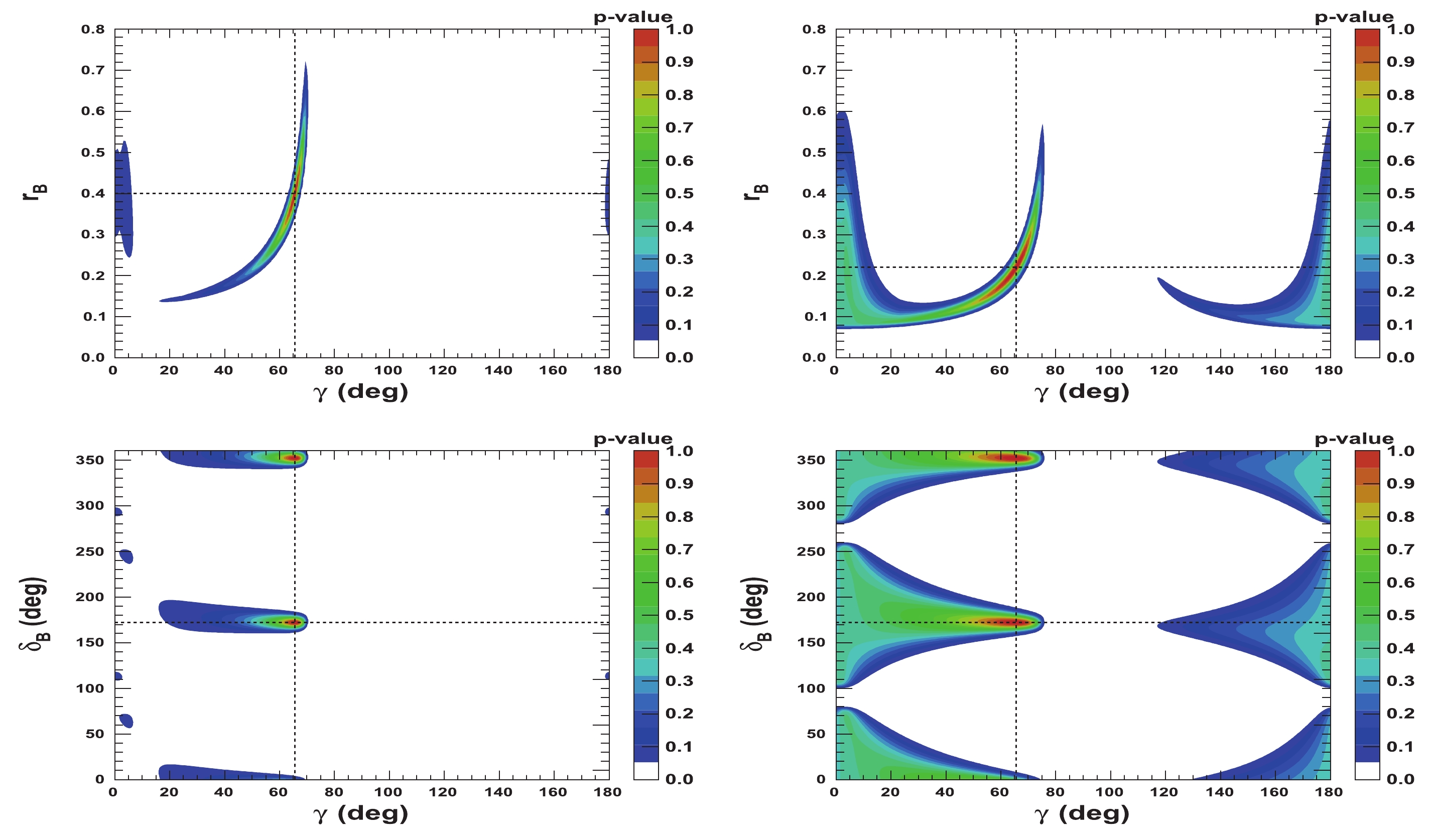
Figure F5. (color online) 2-D p-value profiles of nuisance parameters
rB andδB (full HL-LHC LHCb) as function ofγ . In each figure, the dashed black lines indicate the initial true valuesγ=65.66∘ (1.146 rad) andδB=171.9∘ (3.0 rad), as well asrB=0.4 (left) and 0.22 (right) (w/oB0s→D∗0ϕ ).
Figure F6. (color online) Fit to distributions of
γ obtained from 4000 pseudoexperiments (full HL-LHC LHCb). The initial configuration isγ=65.66∘ ,rB=0.4 (left) and 0.22 (right), andδB=171.9∘ (3 rad) (w/oB0s→D∗0ϕ ). The purple dashed curve represents the tails generated by the correlations with the nuisance parametersr(∗)B andδ(∗)B , whereas the blue dashed curve indicates the core part of the distribution, and the plain red line is the sum of the two components of the fit. -
Untagged
B0s→˜D(∗)0ϕ decays provide another theoretically clean pathway to the measurement of the CKM-angleγ . By using the expected event yields for D decays onKπ ,K3π ,Kππ0 ,KK , andππ , we have demonstrated that a precision onγ of approximately8∘ to19∘ can be achieved with LHCb Run 1 & 2 data. With additional data, a precision onγ of3∘−8∘ can be achieved with the LHCb Run1−3 dataset (23 fb-1 in 2025). Ultimately, a precision of the order of2∘−7∘ is expected with the full HL-LHC LHCb dataset (300 fb-1 in 2038). The asymptotic sensitivity is dominated by the possibly large correlations ofγ with respect to the nuisance parametersr(∗)B andδ(∗)B . The use of this method can improve our knowledge ofγ fromB0s decays and aid in understanding the discrepancy ofγ between measurements withB+ andB0s modes.We are grateful to all members of the CKMfitter group for their comments and providing us with their private software based on a frequentist approach for computing the many pseudoexperiments performed for this study. In particular, we would like to thank J. Charles for his helpful comments on starting this analysis.






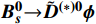

 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF















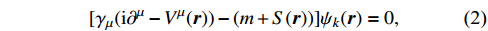
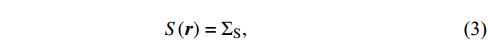



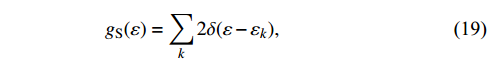
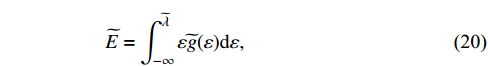
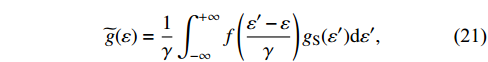
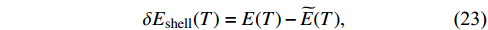
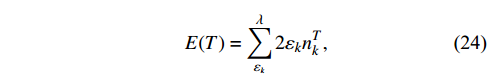


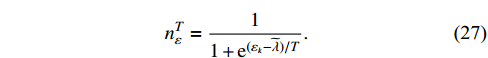
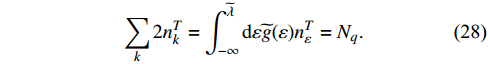

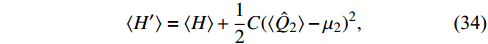
 DownLoad:
DownLoad: