-
The history of multiquarks can be traced to 1964, when Gell-Mann and Zweig proposed such configurations in building the quark model [1, 2]. Although the existence of tetraquarks and pentaquarks has long been speculated, it has rarely, if ever, been proven. The scenario has changed since 2003, owing to the observation of numerous charmoniumlike/bottomoniumlike XYZ states [3], hidden-charm
$ P_c $ states [4–7], doubly-charm$ T_{cc}^+ $ states [8, 9], and fully-charm tetraquark [10] states, which cannot be well explained within the traditional quark model. They are very good candidates for tetraquark and pentaquark states. Details regarding the experimental as well as theoretical progress can be found in review papers [11–17].In 2017, the LHCb Collaboration observed four
$ J/\psi\phi $ structures, i.e.,$ X(4140) $ ,$ X(4274) $ ,$ X(4500) $ and$ X(4700) $ , in the$ B^+\to J/\psi\phi K^+ $ decay process [18, 19], among which$ X(4140) $ and$ X(4274) $ were confirmed to be consistent with previous measurements performed by the CDF Collaboration [20, 21], CMS Collaboration [22], D0 Collaboration [23], and BABAR Collaboration [24], while$ X(4500) $ and$ X(4700) $ were new resonances. Inspired by these structures observed in the$ J/\psi\phi $ invariant mass spectrum,$ X(4140) $ and$ X(4274) $ were considered to be the$ cs\bar c\bar s $ tetraquark ground states, whereas$ X(4500) $ and$ X(4700) $ were interpreted as the$ cs\bar c\bar s $ tetraquark excited states, in various theoretical methods [25–33].Recently, the LHCb Collaboration performed an improved full amplitude analysis of the
$ B^+\to J/\psi\phi K^+ $ decay using a signal yield 6 times larger than that previously analyzed [34]. They confirmed the four$ J/\psi\phi $ states previously reported in Refs. [18, 19]. In addition, a new$ X(4685) $ state was observed in the$ J/\psi\phi $ final state with$ 15\sigma $ significance, and its spin-parity was determined to be$ J^P=1^+ $ . Considering its observed channel, the quantum numbers of$ X(4685) $ should be$ J^{PC}=1^{++} $ with positive charge conjugation parity. Its mass and decay width are measured as$ m=4684\pm7^{+13}_{-16} $ MeV and$ \Gamma=126\pm15^{+37}_{-41} $ MeV. One may wonder if$ X(4685) $ and$ X(4700) $ are the same resonance, as they were observed in the same final states with very similar masses and decay widths. However, LHCb determined their spin-parity as$ J^P=1^+ $ for$ X(4685) $ and$ J^P=0^+ $ for$ X(4700) $ [34]. They are definitely two different states.After the observations of the above
$ J/\psi\phi $ resonances, there have been efforts to understand their underlying structures in the diquark-antidiquark picture. If$ X(4140) $ and$ X(4274) $ can be assigned as the S-wave$ cs\bar c\bar s $ tetraquark ground states with$ J^{PC}=1^{++} $ , the$ X(4685) $ state may be interpreted as the D-wave$ cs\bar c\bar s $ excited tetraquark state. In Ref. [28], the authors calculated the masses of the excited hidden-charm tetraquarks without internal diquark excitation (λ-mode excitation) by using the relativistic quark model. The mass of the D-wave$ cs\bar{c}\bar{s} $ tetraquark with$ J^{PC}=1^{++} $ was calculated to be approximately 4.8 GeV. The same λ-mode excited$ 1^{++} $ D-wave$ cs\bar{c}\bar{s} $ tetraquarks were also studied in the color flux-tube model with masses of approximately 4.9 and 5.2 GeV for the$ \bar{\mathbf{3}}_{cs}\otimes\mathbf{3}_{\bar{c}\bar{s}} $ and$ \mathbf{6}_{cs}\otimes\bar{\mathbf{6}}_{\bar{c}\bar{s}} $ color structures, respectively [35]. In Ref. [29], the D-wave$ cs\bar{c}\bar{s} $ tetraquarks were investigated in different excitation modes by considering internal excited diquarks (ρ-mode excitation) in the relativistic quark model. The masses of the ρ-mode D-wave$ cs\bar{c}\bar{s} $ tetraquarks with$ J^{PC}=1^{++} $ were predicted as 4.6–4.7 GeV, which are far lower than those of the λ-mode tetraquarks. Additionally, the authors of Ref. [36] calculated the mass of the ground state of the$ 1^{++} $ S-wave$ cs\bar{c}\bar{s} $ tetraquark to be approximately 4.6 GeV according to the QCD sum rules, which is far higher than those obtained in Ref. [25]. In Ref. [37],$ X(4685) $ was also considered as the axialvector$ 2S $ radial excited$ cs\bar{c}\bar{s} $ tetraquark state.According to the above analyses and theoretical investigations, the newly observed
$ X(4685) $ state may be explained as a ρ-mode excited$ D $ -wave$ cs\bar{c}\bar{s} $ tetraquark with$ J^{PC}=1^{++} $ . In this work, we systematically study the mass spectra of the D-wave$ cs\bar{c}\bar{s} $ with$ J^{PC}=1^{++} $ and$ 1^{+-} $ in both color symmetric$ \mathbf{6}_{cs}\otimes\bar{\mathbf{6}}_{\bar{c}\bar{s}} $ and antisymmetric$ \bar{\mathbf{3}}_{cs}\otimes\mathbf{3}_{\bar{c}\bar{s}} $ configurations within the framework of QCD sum rules [38, 39]. We investigate the D-wave tetraquarks in different excitation structures, including the ρ-mode and λ-mode.The remainder of this paper is organized as follows. In Sec. II, we construct the nonlocal D-wave interpolating currents for
$ cs\bar{c}\bar{s} $ tetraquark states with$ J^{PC}=1^{++} $ and$ 1^{+-} $ in various excitation structures and color configurations. In Sec. III, we introduce the formalism of tetraquark QCD sum rules and calculate the two-point correlation functions and spectral densities for all currents. We perform numerical analyses to extract the full mass spectra of these D-wave$ cs\bar{c}\bar{s} $ tetraquark states in Sec. IV. The last section presents a summary. -
In this section, we construct the D-wave
$ cs\bar{c}\bar{s} $ tetraquark interpolating currents with$ J^{PC}=1^{++} $ and$ 1^{+-} $ . The$ cs\bar{c}\bar{s} $ tetraquark is composed of$ cs $ diquark and$ \bar c\bar s $ antidiquark fields. By analogy with the heavy baryon system, the orbital angular momentum of the tetraquark can be decomposed into$ \bf{L}=\bf{L_\rho}+\bf{L_\lambda}=\bf{l}_{\rho_1}+\bf{l}_{\rho_2}+\bf{L_\lambda} $ , where$ \bf{l}_{\rho_1} $ ($ \bf{l}_{\rho_2} $ ) represents the internal orbital angular momentum for the$ cs $ ($ \bar c\bar s $ ) field, and$ \bf{L}_\lambda $ represents the orbital angular momentum between the diquark and antidiquark fields. It is convenient to denote the orbital excitation of the tetraquark system as$ (L_\lambda,L_\rho\{l_{\rho_1},l_{\rho_2}\}) $ , as shown in Fig. 1. The D-wave excited$ cs\bar{c}\bar{s} $ tetraquarks are the excitations with$ L_{\rho}+L_\lambda=2 $ . There exist several different excitation structures for the D-wave tetraquarks:$ (L_\lambda,L_\rho\{l_{\rho_1},l_{\rho_2}\})= (2,0\{0,0\}) $ ,$ (1,1\{1,0\}) $ ,$ (1,1\{0,1\}) $ ,$ (0,2\{1,1\}) $ ,$ (0,2\{2,0\}) $ ,$ (0,2\{0,2\}) $ . We study all these D-wave tetraquarks by constructing the interpolating currents with the same structures and quantum numbers.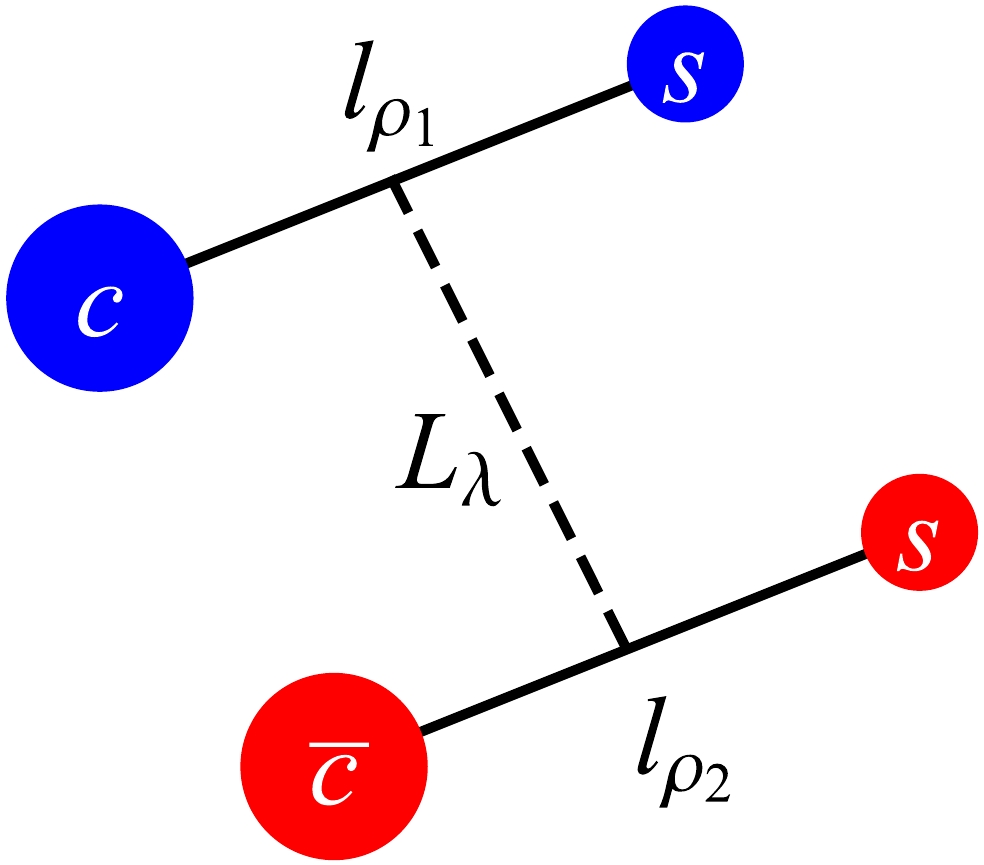
Figure 1. (color online) Excitation structure of the hidden-charm
$ cs\bar{c}\bar{s} $ tetraquark system, in which$ \bf{l}_{\rho_1} $ ($ \bf{l}_{\rho_2} $ ) represents the internal orbital angular momentum for the$ cs $ ($ \bar c\bar s $ ) field, and$ \bf{L_\lambda} $ represents the orbital angular momentum between the diquark and antidiquark fields.The color structure of a diquark-antidiquark tetraquark operator
$ [cs][\bar{c}\bar{s}] $ can be expressed via${S U(3)}$ symmetry:$ \begin{aligned}[b]& (\mathbf{3}\otimes\mathbf{3})_{[cs]}\otimes(\bar{\mathbf{3}}\otimes\bar{\mathbf{3}})_{[\bar{c}\bar{s}]}\\=&(\mathbf{6}\oplus\bar{\mathbf{3}})_{[cs]}\otimes(\mathbf{3}\oplus\bar{\mathbf{6}})_{[\bar{c}\bar{s}]}\\ =&(\mathbf{6}\otimes\bar{\mathbf{6}})\oplus(\bar{\mathbf{3}}\otimes\mathbf{3})\oplus(\mathbf{6}\otimes\mathbf{3})\oplus(\bar{\mathbf{3}}\otimes\bar{\mathbf{6}})\\ =&(\mathbf{1}\oplus\mathbf{8}\oplus\mathbf{27})\oplus(\mathbf{1}\oplus\mathbf{8})\oplus(\mathbf{8}\oplus{\mathbf{10}})\oplus(\mathbf{8}\oplus\overline{\mathbf{10}})\, , \end{aligned} $

(1) in which the color singlet structures come from the
$ \mathbf{6}_{cs}\otimes\bar{\mathbf{6}}_{\bar{c}\bar{s}} $ and$ \bar{\mathbf{3}}_{cs}\otimes\mathbf{3}_{\bar{c}\bar{s}} $ terms, which are denoted as the color symmetric and antisymmetric configurations, respectively. In this work, we consider both these color configurations. We use only the S-wave good diquark field$ \mathcal{O}_S=c^T_{a}\mathbb{C}\gamma_{5}s_{b} $ with$ J^P={0^+} $ to compose the D-wave$ cs\bar{c}\bar{s} $ tetraquark currents by inserting covariant derivative operators. For example, one can obtain a ρ-mode P-wave diquark field with$ J^P=1^- $ $ \begin{align} \mathcal{O}_{P,\,\mu}=c^T_{a}\mathbb{C}\gamma_{5}D_{\mu}s_{b}\, , \end{align} $

(2) and a ρ-mode D-wave diquark field with
$ J^P=2^+ $ $ \begin{align} \mathcal{O}_{D,\,\mu\nu}=c^T_{a}\mathbb{C}\gamma_{5}D_{\mu}D_{\nu}s_{b}\, , \end{align} $

(3) where
$D_{\mu}=\partial_{\mu}+{\rm i} g_sA_\mu$ is the covariant derivative, the subscripts$ a,b $ are color indices,$ \mathbb{C} $ denotes the charge conjugate operator, and T represents the transpose of the quark fields. The corresponding charge conjugate antidiquark fields are$ \begin{aligned}[b] \bar{\mathcal{O}}_S=&\bar{c}_{a}\mathbb{C}\gamma_5\bar{s}^T_{b},\\ \bar{\mathcal{O}}_{P,\, \mu}=&\bar{c}_{a}\mathbb{C}\gamma_5 D_\mu \bar{s}^T_{b},\\ \bar{\mathcal{O}}_{D,\, \mu\nu}=&\bar{c}_{a}\mathbb{C}\gamma_5 D_\mu D_\nu\bar{s}^T_{b}. \end{aligned} $

(4) To compose the λ-mode excited tetraquark operator, one should insert the covariant derivative operator between the diquark and antidiquark fields.
$ \begin{aligned} L_\lambda=0&:\qquad \mathcal{O}_S\bar{\mathcal{O}}_S,\\ L_\lambda=1&:\qquad \mathcal{O}_SD_{\mu}\bar{\mathcal{O}}_S,\\ L_\lambda=2&:\qquad \mathcal{O}_SD_\mu D_\nu\bar{\mathcal{O}}_S.\\ \end{aligned} $

(5) Considering both the symmetric and antisymmetric color configurations, we construct the D-wave
$ cs\bar{c}\bar{s} $ interpolating tetraquark currents with$ J^{PC}=1^{++} $ as$\begin{aligned}[b]\\[-5pt] J_{1,\, \mu\nu}^{A}=&[c_a^T\mathbb{C}\gamma_5s_b]\{D_\mu,D_\nu\}\left([\bar{c}_a\mathbb{C}\gamma_5\bar{s}_b^T]-[\bar{c}_b\mathbb{C}\gamma_5\bar{s}_a^T]\right)+[\bar{c}_a\mathbb{C}\gamma_5\bar{s}^T_b]\{D_\mu,D_\nu\}\left([c_a^T\mathbb{C}\gamma_5s_b]-[c_b^T\mathbb{C}\gamma_5s_a]\right),\\ J_{1,\, \mu\nu}^{S}=&[c_a^T\mathbb{C}\gamma_5s_b]\{D_\mu,D_\nu\}\left([\bar{c}_a\mathbb{C}\gamma_5\bar{s}_b^T]+[\bar{c}_b\mathbb{C}\gamma_5\bar{s}_a^T]\right)+[\bar{c}_a\mathbb{C}\gamma_5\bar{s}^T_b]\{D_\mu,D_\nu\}\left([c_a^T\mathbb{C}\gamma_5s_b]+[c_b^T\mathbb{C}\gamma_5s_a]\right),\\ J_{2,\, \mu\nu}^{A}=&[c_a^T\mathbb{C}\gamma_5D_\mu s_b]\;D_\nu\left([\bar{c}_a\mathbb{C}\gamma_5\bar{s}_b^T]-[\bar{c}_b\mathbb{C}\gamma_5\bar{s}_a^T]\right)+[\bar{c}_a\mathbb{C}\gamma_5D_\mu\bar{s}^T_b]\;D_\nu\left([c_a^T\mathbb{C}\gamma_5s_b]-[c_b^T\mathbb{C}\gamma_5s_a]\right), \\ J_{2,\, \mu\nu}^{S}=&[c_a^T\mathbb{C}\gamma_5D_\mu s_b]\;D_\nu\left([\bar{c}_a\mathbb{C}\gamma_5\bar{s}_b^T]+[\bar{c}_b\mathbb{C}\gamma_5\bar{s}_a^T]\right)+[\bar{c}_a\mathbb{C}\gamma_5D_\mu\bar{s}^T_b]\;D_\nu\left([c_a^T\mathbb{C}\gamma_5s_b]+[c_b^T\mathbb{C}\gamma_5s_a]\right), \\ J_{3,\, \mu\nu}^{A}=&[c_a^T\mathbb{C}\gamma_5s_b]\;D_\mu\left([\bar{c}_a\mathbb{C}\gamma_5D_\nu\bar{s}_b^T]-[\bar{c}_b\mathbb{C}\gamma_5D_\nu\bar{s}_a^T]\right)+[\bar{c}_a\mathbb{C}\gamma_5\bar{s}^T_b]\;D_\mu\left([c_a^T\mathbb{C}\gamma_5D_\nu s_b]-[c_b^T\mathbb{C}\gamma_5D_\nu s_a]\right), \\ J_{3,\, \mu\nu}^{S}=&[c_a^T\mathbb{C}\gamma_5s_b]\;D_\mu\left([\bar{c}_a\mathbb{C}\gamma_5D_\nu\bar{s}_b^T]+[\bar{c}_b\mathbb{C}\gamma_5D_\nu\bar{s}_a^T]\right)+[\bar{c}_a\mathbb{C}\gamma_5\bar{s}^T_b]\;D_\mu\left([c_a^T\mathbb{C}\gamma_5D_\nu s_b]+[c_b^T\mathbb{C}\gamma_5D_\nu s_a]\right), \\ J_{4,\, \mu\nu}^{A}=&[c_a^T\mathbb{C}D_\mu\gamma_5s_b]\left([\bar{c}_a\mathbb{C}\gamma_5D_\nu\bar{s}_b^T]-[\bar{c}_b\mathbb{C}\gamma_5D_\nu\bar{s}_a^T]\right)+[\bar{c}_a\mathbb{C}\gamma_5D_\mu\bar{s}^T_b]\left([c_a^T\mathbb{C}\gamma_5D_\nu s_b]-[c_b^T\mathbb{C}\gamma_5D_\nu s_a]\right), \\ J_{4,\, \mu\nu}^{S}=&[c_a^T\mathbb{C}D_\mu\gamma_5s_b]\left([\bar{c}_a\mathbb{C}\gamma_5D_\nu\bar{s}_b^T]+[\bar{c}_b\mathbb{C}\gamma_5D_\nu\bar{s}_a^T]\right)+[\bar{c}_a\mathbb{C}\gamma_5D_\mu\bar{s}^T_b]\left([c_a^T\mathbb{C}\gamma_5D_\nu s_b]+[c_b^T\mathbb{C}\gamma_5D_\nu s_a]\right), \\ J_{5,\, \mu\nu}^{A}=&[c_a^T\mathbb{C}\gamma_5D_\mu\;D_\nu s_b]\left([\bar{c}_a\mathbb{C}\gamma_5\bar{s}_b^T]-[\bar{c}_b\mathbb{C}\gamma_5\bar{s}_a^T]\right)+[\bar{c}_a\mathbb{C}\gamma_5D_\mu\;D_\nu\bar{s}^T_b]\left([c_a^T\mathbb{C}\gamma_5s_b]-[c_b^T\mathbb{C}\gamma_5s_a]\right),\\ J_{5,\, \mu\nu}^{S}=&[c_a^T\mathbb{C}\gamma_5D_\mu\;D_\nu s_b]\left([\bar{c}_a\mathbb{C}\gamma_5\bar{s}_b^T]+[\bar{c}_b\mathbb{C}\gamma_5\bar{s}_a^T]\right)+[\bar{c}_a\mathbb{C}\gamma_5D_\mu\;D_\nu\bar{s}^T_b]\left([c_a^T\mathbb{C}\gamma_5s_b]+[c_b^T\mathbb{C}\gamma_5s_a]\right),\\ J_{6,\, \mu\nu}^{A}=&[c_a^T\mathbb{C}\gamma_5s_b]\left([\bar{c}_a\mathbb{C}\gamma_5D_\mu\;D_\nu \bar{s}_b^T]-[\bar{c}_b\mathbb{C}\gamma_5D_\mu\;D_\nu \bar{s}_a^T]\right)+[\bar{c}_a\mathbb{C}\gamma_5\bar{s}^T_b]\left([c_a^T\mathbb{C}\gamma_5D_\mu\;D_\nu s_b]-[c_b^T\mathbb{C}\gamma_5D_\mu\;D_\nu s_a]\right), \\ J_{6,\, \mu\nu}^{S}=&[c_a^T\mathbb{C}\gamma_5s_b]\left([\bar{c}_a\mathbb{C}\gamma_5D_\mu\;D_\nu \bar{s}_b^T]+[\bar{c}_b\mathbb{C}\gamma_5D_\mu\;D_\nu \bar{s}_a^T]\right)+[\bar{c}_a\mathbb{C}\gamma_5\bar{s}^T_b]\left([c_a^T\mathbb{C}\gamma_5D_\mu\;D_\nu s_b]+[c_b^T\mathbb{C}\gamma_5D_\mu\;D_\nu s_a]\right),\\ \end{aligned} $ 
(6) and the D-wave
$ cs\bar{c}\bar{s} $ interpolating tetraquark currents with$ J^{PC}=1^{+-} $ as$ \begin{aligned}[b] J_{7,\, \mu\nu}^{A}=&[c_a^T\mathbb{C}\gamma_5s_b]\{D_\mu,D_\nu\}\left([\bar{c}_a\mathbb{C}\gamma_5\bar{s}_b^T]-[\bar{c}_b\mathbb{C}\gamma_5\bar{s}_a^T]\right)-[\bar{c}_a\mathbb{C}\gamma_5\bar{s}^T_b]\{D_\mu,D_\nu\}\left([c_a^T\mathbb{C}\gamma_5s_b]-[c_b^T\mathbb{C}\gamma_5s_a]\right),\\ J_{7,\, \mu\nu}^{S}=&[c_a^T\mathbb{C}\gamma_5s_b]\{D_\mu,D_\nu\}\left([\bar{c}_a\mathbb{C}\gamma_5\bar{s}_b^T]+[\bar{c}_b\mathbb{C}\gamma_5\bar{s}_a^T]\right)-[\bar{c}_a\mathbb{C}\gamma_5\bar{s}^T_b]\{D_\mu,D_\nu\}\left([c_a^T\mathbb{C}\gamma_5s_b]+[c_b^T\mathbb{C}\gamma_5s_a]\right),\\ J_{8,\, \mu\nu}^{A}=&[c_a^T\mathbb{C}\gamma_5D_\mu s_b]\;D_\nu\left([\bar{c}_a\mathbb{C}\gamma_5\bar{s}_b^T]-[\bar{c}_b\mathbb{C}\gamma_5\bar{s}_a^T]\right)-[\bar{c}_a\mathbb{C}\gamma_5D_\mu\bar{s}^T_b]\;D_\nu\left([c_a^T\mathbb{C}\gamma_5s_b]-[c_b^T\mathbb{C}\gamma_5s_a]\right), \\ J_{8,\, \mu\nu}^{S}=&[c_a^T\mathbb{C}\gamma_5D_\mu s_b]\;D_\nu\left([\bar{c}_a\mathbb{C}\gamma_5\bar{s}_b^T]+[\bar{c}_b\mathbb{C}\gamma_5\bar{s}_a^T]\right)-[\bar{c}_a\mathbb{C}\gamma_5D_\mu\bar{s}^T_b]\;D_\nu\left([c_a^T\mathbb{C}\gamma_5s_b]+[c_b^T\mathbb{C}\gamma_5s_a]\right), \\ J_{9,\, \mu\nu}^{A}=&[c_a^T\mathbb{C}\gamma_5s_b]\;D_\mu\left([\bar{c}_a\mathbb{C}\gamma_5D_\nu\bar{s}_b^T]-[\bar{c}_b\mathbb{C}\gamma_5D_\nu\bar{s}_a^T]\right)-[\bar{c}_a\mathbb{C}\gamma_5\bar{s}^T_b]\;D_\mu\left([c_a^T\mathbb{C}\gamma_5D_\nu s_b]-[c_b^T\mathbb{C}\gamma_5D_\nu s_a]\right), \\ J_{9,\, \mu\nu}^{S}=&[c_a^T\mathbb{C}\gamma_5s_b]\;D_\mu\left([\bar{c}_a\mathbb{C}\gamma_5D_\nu\bar{s}_b^T]+[\bar{c}_b\mathbb{C}\gamma_5D_\nu\bar{s}_a^T]\right)-[\bar{c}_a\mathbb{C}\gamma_5\bar{s}^T_b]\;D_\mu\left([c_a^T\mathbb{C}\gamma_5D_\nu s_b]+[c_b^T\mathbb{C}\gamma_5D_\nu s_a]\right), \\ J_{10,\, \mu\nu}^{A}=&[c_a^T\mathbb{C}D_\mu\gamma_5s_b]\left([\bar{c}_a\mathbb{C}\gamma_5D_\nu\bar{s}_b^T]-[\bar{c}_b\mathbb{C}\gamma_5D_\nu\bar{s}_a^T]\right)-[\bar{c}_a\mathbb{C}\gamma_5D_\mu\bar{s}^T_b]\left([c_a^T\mathbb{C}\gamma_5D_\nu s_b]-[c_b^T\mathbb{C}\gamma_5D_\nu s_a]\right), \\ J_{10,\, \mu\nu}^{S}=&[c_a^T\mathbb{C}D_\mu\gamma_5s_b]\left([\bar{c}_a\mathbb{C}\gamma_5D_\nu\bar{s}_b^T]+[\bar{c}_b\mathbb{C}\gamma_5D_\nu\bar{s}_a^T]\right)-[\bar{c}_a\mathbb{C}\gamma_5D_\mu\bar{s}^T_b]\left([c_a^T\mathbb{C}\gamma_5D_\nu s_b]+[c_b^T\mathbb{C}\gamma_5D_\nu s_a]\right), \\ J_{11,\, \mu\nu}^{A}=&[c_a^T\mathbb{C}\gamma_5D_\mu\;D_\nu s_b]\left([\bar{c}_a\mathbb{C}\gamma_5\bar{s}_b^T]-[\bar{c}_b\mathbb{C}\gamma_5\bar{s}_a^T]\right)-[\bar{c}_a\mathbb{C}\gamma_5D_\mu\;D_\nu\bar{s}^T_b]\left([c_a^T\mathbb{C}\gamma_5s_b]-[c_b^T\mathbb{C}\gamma_5s_a]\right),\\ J_{11,\, \mu\nu}^{S}=&[c_a^T\mathbb{C}\gamma_5D_\mu\;D_\nu s_b]\left([\bar{c}_a\mathbb{C}\gamma_5\bar{s}_b^T]+[\bar{c}_b\mathbb{C}\gamma_5\bar{s}_a^T]\right)-[\bar{c}_a\mathbb{C}\gamma_5D_\mu\;D_\nu\bar{s}^T_b]\left([c_a^T\mathbb{C}\gamma_5s_b]+[c_b^T\mathbb{C}\gamma_5s_a]\right),\\ J_{12,\, \mu\nu}^{A}=&[c_a^T\mathbb{C}\gamma_5s_b]\left([\bar{c}_a\mathbb{C}\gamma_5D_\mu\;D_\nu \bar{s}_b^T]-[\bar{c}_b\mathbb{C}\gamma_5D_\mu\;D_\nu \bar{s}_a^T]\right)-[\bar{c}_a\mathbb{C}\gamma_5\bar{s}^T_b]\left([c_a^T\mathbb{C}\gamma_5D_\mu\;D_\nu s_b]-[c_b^T\mathbb{C}\gamma_5D_\mu\;D_\nu s_a]\right), \\ J_{12,\, \mu\nu}^{S}=&[c_a^T\mathbb{C}\gamma_5s_b]\left([\bar{c}_a\mathbb{C}\gamma_5D_\mu\;D_\nu \bar{s}_b^T]+[\bar{c}_b\mathbb{C}\gamma_5D_\mu\;D_\nu \bar{s}_a^T]\right)-[\bar{c}_a\mathbb{C}\gamma_5\bar{s}^T_b]\left([c_a^T\mathbb{C}\gamma_5D_\mu\;D_\nu s_b]+[c_b^T\mathbb{C}\gamma_5D_\mu\;D_\nu s_a]\right), \end{aligned} $

(7) where
$ \{D_\mu,D_\nu\}=D_\mu D_\nu+D_\nu D_\mu $ . The interpolating currents with the superscripts "S" and "A" denote the symmetric$ [cs]_{\mathbf{6}}[\bar{c}\bar{s}]_{\bar{\mathbf{6}}} $ and antisymmetric$ [cs]_{\bar{\mathbf{3}}}[\bar{c}\bar{s}]_{\mathbf{3}} $ color structures, which are abbreviated as$ \mathbf{3} $ and$ \mathbf{6} $ , respectively, hereinafter. The excitation structures$(L_\lambda,L_\rho\{l_{\rho_1}, l_{\rho_2}\})$ , color configurations, and$ J^{PC} $ quantum numbers for these interpolating currents are presented in Table 1. The abbreviation$ \mathbf{3}_{\lambda\lambda}/\mathbf{6}_{\lambda\lambda} $ ($ \mathbf{3}_{\rho\rho}/\mathbf{6}_{\rho\rho} $ ) indicates that the corresponding current contains two λ-orbital (ρ-orbital) momentums with an antisymmetric/symmetric color structure, while$ \mathbf{3}_{\lambda\rho}/\mathbf{6}_{\lambda\rho} $ indicates that the current contains one λ-orbital momentum and one ρ-orbital momentum with an antisymmetric/symmetric color structure. In the following, we investigate the mass spectra for the D-wave$ cs\bar{c}\bar{s} $ tetraquarks by using these interpolating currents. Among the currents belonging to the$ (0,2\{2,0\}) $ and$ (0,2\{0,2\}) $ structures, we only study the$ (0,2\{2,0\}) $ ones, because the$ (0,2\{0,2\}) $ currents would yield the same results in our calculations.$ (L_\lambda,L_\rho\{l_{\rho_1},l_{\rho_2}\}) $ 

$ [cs]_{\bar{\mathbf{3}}}[\bar{c}\bar{s}]_{\mathbf{3}} $ 

$ [cs]_{\mathbf{6}}[\bar{c}\bar{s}]_{\bar{\mathbf{6}}} $ 

$ J^{PC} $ 

$ (2,0\{0,0\}) $ 

$ J_{1,\, \mu\nu}^{A}(\mathbf{3}_{\lambda\lambda}) $ 

$ J_{1,\, \mu\nu}^{S}(\mathbf{6}_{\lambda\lambda}) $ 

$ 1^{++} $ 

$ J_{7,\, \mu\nu}^{A}(\mathbf{3}_{\lambda\lambda}) $ 

$ J_{7,\, \mu\nu}^{S}(\mathbf{6}_{\lambda\lambda}) $ 

$ 1^{+-} $ 

$ (1,1\{1,0\}) $ 

$ J_{2,\, \mu\nu}^{A}(\mathbf{3}_{\lambda\rho}) $ 

$ J_{2,\, \mu\nu}^{S}(\mathbf{6}_{\lambda\rho}) $ 

$ 1^{++} $ 

$ J_{8,\, \mu\nu}^{A}(\mathbf{3}_{\lambda\rho}) $ 

$ J_{8,\, \mu\nu}^{S}(\mathbf{6}_{\lambda\rho}) $ 

$ 1^{+-} $ 

$ (1,1\{0,1\}) $ 

$ J_{3,\, \mu\nu}^{A}(\mathbf{3}_{\lambda\rho}) $ 

$ J_{3,\, \mu\nu}^{S}(\mathbf{6}_{\lambda\rho}) $ 

$ 1^{++} $ 

$ J_{9,\, \mu\nu}^{A}(\mathbf{3}_{\lambda\rho}) $ 

$ J_{9,\, \mu\nu}^{S}(\mathbf{6}_{\lambda\rho}) $ 

$ 1^{+-} $ 

$ (0,2\{1,1\}) $ 

$ J_{4,\, \mu\nu}^{A}(\mathbf{3}_{\rho\rho}) $ 

$ J_{4,\, \mu\nu}^{S}(\mathbf{6}_{\rho\rho}) $ 

$ 1^{++} $ 

$ J_{10,\, \mu\nu}^{A}(\mathbf{3}_{\rho\rho}) $ 

$ J_{10,\, \mu\nu}^{S}(\mathbf{6}_{\rho\rho}) $ 

$ 1^{+-} $ 

$ (0,2\{2,0\}) $ 

$ J_{5,\, \mu\nu}^{A}(\mathbf{3}_{\rho\rho}) $ 

$ J_{5,\, \mu\nu}^{S}(\mathbf{6}_{\rho\rho}) $ 

$ 1^{++} $ 

$ J_{11,\, \mu\nu}^{A}(\mathbf{3}_{\rho\rho}) $ 

$ J_{11,\, \mu\nu}^{S}(\mathbf{6}_{\rho\rho}) $ 

$ 1^{+-} $ 

$ (0,2\{0,2\}) $ 

$ J_{6,\, \mu\nu}^{A}(\mathbf{3}_{\rho\rho}) $ 

$ J_{6,\, \mu\nu}^{S}(\mathbf{6}_{\rho\rho}) $ 

$ 1^{++} $ 

$ J_{12,\, \mu\nu}^{A}(\mathbf{3}_{\rho\rho}) $ 

$ J_{12,\, \mu\nu}^{S}(\mathbf{6}_{\rho\rho}) $ 

$ 1^{+-} $ 

Table 1. Excitation structures, color configurations, and
$J^{PC}$ quantum numbers for the D-wave$cs\bar{c}\bar{s}$ interpolating currents given by Eqs. (6) and (7). -
In this section, we introduce the method of QCD sum rules for the hidden-charm tetraquark states. The two-point correlation functions for the tensor currents can be written as
$ \begin{aligned}[b] \Pi_{\mu\nu,\,\rho\sigma}(q^2)&={\rm i}\int {\rm d}^4x {\rm e}^{{\rm i} q\cdot x}\langle 0|T\left[J_{\mu\nu}(x)J^{\dagger}_{\rho\sigma}(0)\right]|0\rangle\\ &=T^+_{\mu\nu\rho\sigma}\Pi_1(q^2)+\cdots \, , \end{aligned} $

(8) where
$ \begin{aligned}[b] T^\pm_{\mu\nu\rho\sigma}&=\left(\frac{q_\mu q_\nu}{q^2}\eta_{\nu\sigma}\pm(\mu\leftrightarrow\nu)\right)\pm(\rho\leftrightarrow\sigma),\\ \eta_{\mu\nu}&=\frac{q_{\mu} q_{\nu}}{q^{2}}-g_{\mu \nu}, \end{aligned} $

(9) $ \Pi_{1}\left(q^{2}\right) $ is the polarization function related to the spin-1 intermediate state, and$ "\cdots" $ represents other tensor structures relating to different hadron states. The tensor current can couple to the spin-1 physical state X through$ \begin{aligned}[b] \langle0|J_{\mu\nu}(x)|1^{\mathbb{P}\mathbb{C}}(\overrightarrow{\mathbf{p}},r)\rangle&=Z\epsilon^{\mu\nu\alpha\beta}\in_\alpha(\overrightarrow{\mathbf{p}},r)p_\beta,\\ \langle0|J_{\mu\nu}(x)|1^{(-\mathbb{P})\mathbb{C}}(\overrightarrow{\mathbf{p}},r)\rangle&=Z_+(\in^\mu(\overrightarrow{\mathbf{p}},r)p^\nu+\in^\nu(\overrightarrow{\mathbf{p}},r)p^\mu)\\ &+Z_-(\in^\mu(\overrightarrow{\mathbf{p}},r)p^\nu-\in^\nu(\overrightarrow{\mathbf{p}},r)p^\mu), \end{aligned} $

(10) where
$ Z, Z_+, Z_- $ are coupling constants,$ \epsilon^{\mu\nu\alpha\beta} $ is the antisymmetical tensor, and$ \in_\alpha $ is the polarization tensor.At the hadron level, the two-point correlation function can be written as
$ \begin{equation} \Pi(q^2)=\frac{1}{\pi}\int^{\infty}_{s_<}\frac{\mathrm{Im}\Pi(s)}{s-q^2-{\rm i}\epsilon}{\rm d}s, \end{equation} $

(11) where we use the form of the dispersion relation, and
$ s_< $ denotes the physical threshold. The imaginary part of the correlation function is defined as the spectral function, which is usually evaluated at the hadron level by inserting intermediate hadron states$ \sum_n|n\rangle\langle n| $ $ \begin{aligned}[b] \rho(s)\equiv\frac{1}{\pi}\mathrm{Im}\Pi(s)&=\sum_n\delta(s-M^2_n)\langle 0|\eta|n\rangle\langle n|\eta^\dagger|0\rangle\\ &=f^2_X\delta(s-m^2_X)+\mathrm{continuum}, \end{aligned} $

(12) where we have adopted the usual parametrization of one-pole dominance for the ground state X and a continuum contribution. Researchers have investigated the excited mesons [40–42], baryons [43], and tetraquarks [44–46] in QCD sum rules by using the non-local interpolating currents under the "pole+continuum" approximation. The spectral density
$ \rho(s) $ can also be evaluated at the quark-gluon level via the operator product expansion (OPE). To pick out the contribution of the lowest lying resonance in (12), the QCD sum rules are established as$ \begin{equation} \mathcal{L}_k(s_0,M_{\rm B}^2)=f^2_Xm^{2k}_{H}{\rm e}^{-m^2_H/M_{\rm B}^2}=\int^{s_0}_{4m_c^2}{\rm d} s\,{\rm e}^{-s/M_{\rm B}^2}\rho(s)s^k, \end{equation} $

(13) where
$M_{\rm B}$ represents the Borel mass introduced by the Borel transformation, and$ s_0 $ is the continuum threshold. The mass of the lowest-lying hadron can be thus extracted as$ \begin{equation} m_X(s_0,M_{\rm B}^2)=\sqrt{\frac{\mathcal{L}_1(s_0,M_{\rm B}^2)}{\mathcal{L}_0(s_0,M_{\rm B}^2)}}, \end{equation} $

(14) which is the function of two parameters
$M_{\rm B}^2$ and$ s_0 $ . We discuss the details of obtaining suitable parameter working regions in QCD sum rule analyses in next section. Using the operator production expansion method, the two-point function can also be evaluated at the quark-gluonic level as a function of various QCD parameters, such as QCD condensates, quark masses, and the strong coupling constant$ \alpha_s $ . To evaluate the Wilson coefficients, we adopt the heavy quark propagator in the momentum space and the strange quark propagator in the coordinate space:$ \begin{aligned}[b] {\rm i} S_{c}^{a b}(p)=&\frac{{\rm i} \delta^{a b}}{\hat{p}-m_{c}} +\frac{{\rm i}}{4} g_{s} \frac{\lambda_{a b}^{n}}{2} G_{\mu \nu}^{n} \frac{\sigma^{\mu \nu}\left(\hat{p}+m_{c}\right)+\left(\hat{p}+m_{c}\right) \sigma^{\mu \nu}}{12} \\ &+\frac{{\rm i} \delta^{a b}}{12}\langle g_{s}^{2} G G\rangle m_{c} \frac{p^{2}+m_{c} \hat{p}}{(p^{2}-m_{c}^{2})^{4}}\, , \\ {\rm i} S_{s}^{ab}(x)=&\frac{{\rm i}\delta^{ab}}{2\pi^2x^4}\hat{x}-\frac{\delta^{ab}}{12}\langle\bar{s}s\rangle+\frac{{\rm i}}{32\pi^2}\frac{\lambda^n_{ab}}{2}g_sG^n_{\mu\nu}\frac{1}{x^2}(\sigma^{\mu\nu}\hat{x}+\hat{x}\sigma^{\mu\nu})\\ &+\frac{\delta^{ab}x^2}{192}\langle\bar{s}g_s\sigma\cdot Gs\rangle-\frac{m_s\delta^{ab}}{4\pi^2x^2}+\frac{{\rm i}\delta^{ab}m_s\langle\bar{s}s\rangle}{48}\hat{x}\\&-\frac{{\rm i} m_s\langle\bar{s}g_s\sigma\cdot Gs\rangle\delta^{ab}x^2\hat{x}}{1152}\, , \end{aligned} $

(15) where
$ \hat{p}=p^{\mu}\gamma_{\mu} $ and$ \hat{x}=x^{\mu}\gamma_{\mu} $ . In this work, we evaluate the Wilson coefficients of the correlation function up to dimension ten condensates at the leading order of$ \alpha_s $ . We find that the calculations are highly complex owing to the existence of the covariant derivative operators. The results of spectral functions are too lengthy to present here; thus, they are provided in the Appendix. -
In this section, we perform the QCD sum rule analyses for the
$ cs\bar{c}\bar{s} $ tetraquark systems. We use the following values of the quark masses and various QCD condensates [3, 47–55]:$ \begin{aligned}[b] &m_c(m_c)=1.27\pm0.02\;\mathrm{GeV},\\ &m_c/m_s=11.76^{+0.05}_{-0.10} \, , \\ &\langle \bar{q}q\rangle=-(0.24\pm0.03)^3\;\mathrm{GeV}^3,\\ &\langle \bar{q}g_s\sigma\cdot Gq\rangle=-M_0^2\langle \bar{q}q\rangle,\\ &\langle \bar{q}q\bar{q}q\rangle=\langle \bar{q}q\rangle^2\,,\\ &M_0^2=(0.8\pm0.2)\;\mathrm{GeV}^2,\\ &\langle \bar{s}s\rangle/\langle \bar{q}q\rangle=0.8\pm0.1,\\ &\langle g_s^2GG\rangle=(0.48\pm0.14)\;\mathrm{GeV}^4,\\ \end{aligned} $

(16) where the charm quark mass
$ m_c $ is the "running" mass in the$ \overline{\text{MS}} $ scheme. To ensure the unified renormalization scale in our analyses, we use the renormalization scheme and scale independent$ m_c/m_s $ mass ratio from PDG [3] to obtain the strange quark mass$ m_s $ .To establish a stable mass sum rule, one should initially find the appropriate parameter working regions, i.e, for the continuum threshold
$ s_0 $ and the Borel mass$M_{\rm B}^2$ . The threshold$ s_0 $ can be determined via the minimized variation of the hadronic mass$ m_X $ with respect to the Borel mass$M_{\rm B}^2$ . The lower bound on the Borel mass$M_{\rm B}^2$ can be fixed by requiring a reasonable OPE convergence, while its upper bound is determined through a sufficient pole contribution. The pole contribution is defined as$ \begin{equation} \mathrm{PC}(s_0,M_{\rm B}^2)=\frac{\mathcal{L}_0(s_0,M_{\rm B}^2)}{\mathcal{L}_0(\infty,M_{\rm B}^2)}, \end{equation} $

(17) where
$ \mathcal{L}_0 $ is defined in Eq. (13).As an example, we use the color antisymmetric current
$ J_{5,\mu\nu}^{A}(x) $ with$ J^{PC}=1^{++} $ in the$ (0,2\{2,0\}) $ excitation mode to show the details of the numerical analysis. For this current, the dominant non-perturbative contribution to the correlation function comes from the quark condensate, which is proportional to the charm quark mass$ m_c $ . Figure 2 shows the contributions of the perturbative term and various condensate terms to the correlation function with respect to$M_{\rm B}^2$ when$ s_0 $ tends to infinity. It is clear that the Borel mass$M_{\rm B}^2$ should be large enough to ensure the convergence of the OPE series. In this work, we require that the perturbative term be two times larger than the quark condensate term, providing the lower bound of the Borel mass$M_{\rm B}^2\geq2.82\;\mathrm{GeV}^2$ . The other QCD condensates are far smaller than the quark condensate in this region of$M_{\rm B}^2$ . Studying the pole contribution defined in Eq. (17) reveals that the PC is very small for such D-wave$ cs\bar{c}\bar{s} $ tetraquark systems owing to the high dimension of the interpolating current. To find an upper bound on the Borel mass, we require the pole contribution to be larger than$ 20\% $ . As a result, the reasonable Borel window for the current$ J_{5,\mu\nu}^{A}(x) $ is obtained as$2.94\;\mathrm{GeV}^2\leq M_{\rm B}^2\leq3.90\;\mathrm{GeV}^2$ .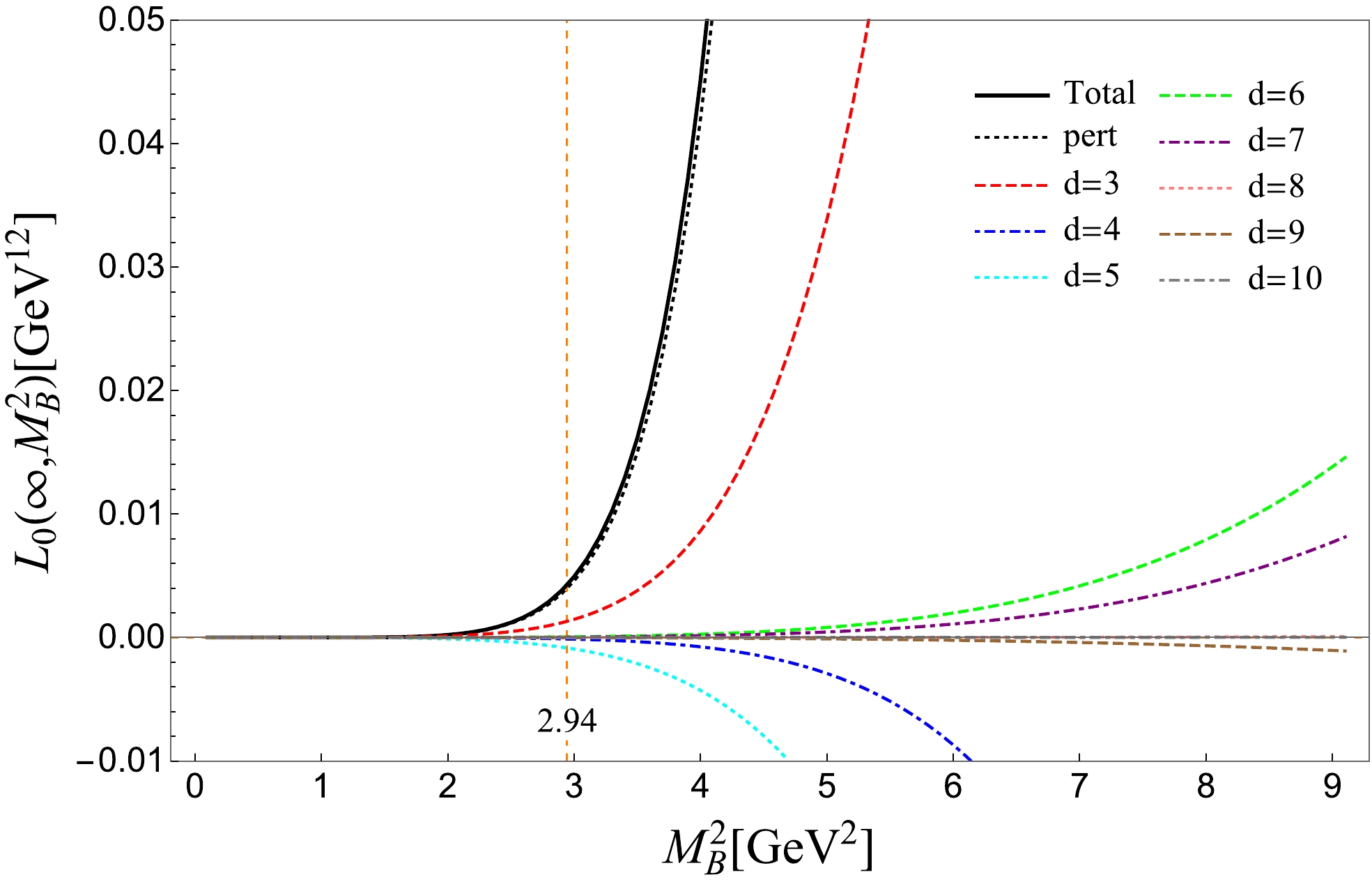
Figure 2. (color online) Contributions of various OPE terms to the correlation function for the current
$ J_{5,\mu\nu}^{A}(x) $ as a function of$M_{\rm B}^2$ when$ s_0\to\infty $ .As mentioned previously, the variation of the extracted hadron mass
$ m_X $ with respect to$M_{\rm B}^2$ should be minimized to obtain the optimal value of the continuum threshold$ s_0 $ . We show the variation of$ m_X $ with$ s_0 $ in the left panel of Fig. 3, from which the optimized value of the continuum threshold can be chosen as$s_0\approx(30.0\pm 1.5) \mathrm{GeV}^2$ . In the right panel of Fig. 3, the mass sum rules are established to be very stable in the above parameter regions of$ s_0 $ and$M_{\rm B}^2$ . The hadron mass for this D-wave$ cs\bar{c}\bar{s} $ tetraquark with$ J^{PC}=1^{++} $ can be obtained as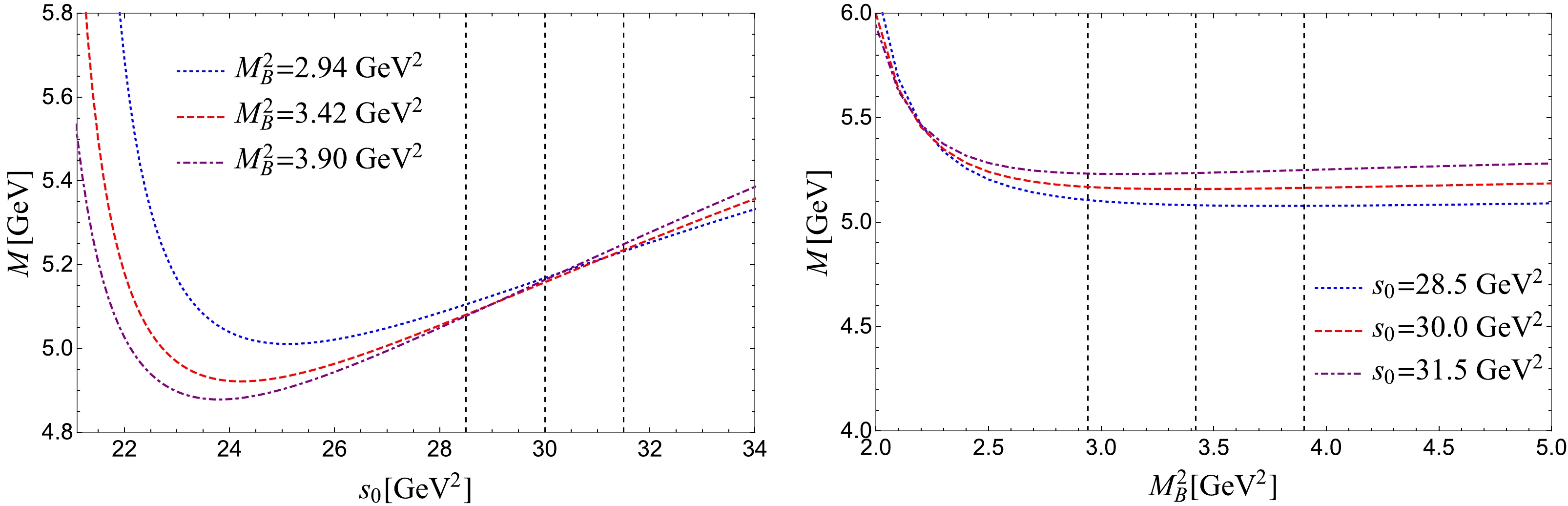
Figure 3. (color online) Mass curves for the interpolating current
$ J_{5,\mu\nu}^A(x) $ with$ J^{PC}=1^{++} $ .$ \begin{equation} m_{J_{5}^A}=5.16_{-0.13}^{+0.12} \; \text{GeV}\,, \end{equation} $

(18) where the errors come from the uncertainties of the threshold
$ s_0 $ , Borel mass$M_{\rm B}^2$ , quark masses, and various QCD condensates in Eq. (16). Performing the same numerical analyses for all the interpolating currents in Eqs. (6)–(7), we find that only the currents$ J_{5,\mu\nu}^{S}(x) $ ,$ J_{11,\mu\nu}^{A(S)}(x) $ , and$ J_{4,\mu\nu}^{A(S)}(x) $ with$ J^{PC}=1^{++} $ exhibit the same mass sum rule behaviors as$ J_{5,\mu\nu}^A(x) $ . We present the numerical results in Table 2.$ (L_\lambda,L_\rho\{l_{\rho_1},l_{\rho_2}\}) $ 

Current $ J^{PC} $ 

$m_{A}/\mathrm{GeV}$ 

$ s_{0,A}/\mathrm{GeV}^2 $ 

$M_{{\rm B},A}^2 /\mathrm{GeV}^2$ 

$PC_{A}({\text{%} })$ 

$ m_{S} / \mathrm{GeV} $ 

$ s_{0,S}/ \mathrm{GeV}^2 $ 

$M_{{\rm B},S}^2 /\mathrm{GeV}^2$ 

$PC_{S}({\text{%} })$ 

$ (2,0\{0,0\}) $ 

$ J_{1,\mu\nu}^{A(S)} $ 

$ 1^{++} $ 

$ 4.70^{+0.12}_{-0.11} $ 

$ 27(\pm5{\text{%}}) $ 

$ 3.27\sim3.92 $ 

27.3 $ 4.91^{+0.11}_{-0.12} $ 

$ 28(\pm5{\text{%}}) $ 

$ 3.56\sim4.20 $ 

26.5 $ (2,0\{0,0\}) $ 

$ J_{7,\mu\nu}^{A(S)} $ 

$ 1^{+-} $ 

$ 4.78^{+0.12}_{-0.11} $ 

$ 27(\pm5{\text{%}}) $ 

$ 3.58\sim4.16 $ 

25.4 $ 4.89^{+0.10}_{-0.11} $ 

$ 28(\pm5{\text{%}}) $ 

$ 3.60\sim4.50 $ 

28.5 $ (1,1\{1,0\}) $ 

$ J_{2,\mu\nu}^{A(S)} $ 

$ 1^{++} $ 

$ 4.80^{+0.12}_{-0.16} $ 

$ 28(\pm5{\text{%}}) $ 

$ 3.15\sim3.94 $ 

39.6 $ 4.84^{+0.12}_{-0.16} $ 

$ 29(\pm5{\text{%}}) $ 

$ 2.63\sim4.13 $ 

37.9 $ (1,1\{1,0\}) $ 

$ J_{8,\mu\nu}^{A(S)} $ 

$ 1^{+-} $ 

$ 4.81\pm0.10 $ 

$ 27(\pm5{\text{%}}) $ 

$ 3.71\sim4.51 $ 

26.3 $ 4.85^{+0.11}_{-0.10} $ 

$ 28(\pm5{\text{%}}) $ 

$ 4.69\sim5.16 $ 

28.2 $ (1,1\{0,1\}) $ 

$ J_{3,\mu\nu}^{A(S)} $ 

$ 1^{++} $ 

$ 4.80^{+0.11}_{-0.10} $ 

$ 26(\pm5{\text{%}}) $ 

$ 2.75\sim3.31 $ 

26.1 $ 4.82^{+0.12}_{-0.11} $ 

$ 27(\pm5{\text{%}}) $ 

$ 3.37\sim 4.11 $ 

47.0 $ (1,1\{0,1\}) $ 

$ J_{9,\mu\nu}^{A(S)} $ 

$ 1^{+-} $ 

$ 4.98^{+0.13}_{-0.23} $ 

$ 26(\pm5{\text{%}}) $ 

$ 2.73\sim3.14 $ 

24.0 $ 4.92^{+0.11}_{-0.10} $ 

$ 28(\pm5{\text{%}}) $ 

$ 3.55\sim3.91 $ 

23.4 $ (0,2\{1,1\}) $ 

$ J_{4,\mu\nu}^{A(S)} $ 

$ 1^{++} $ 

$ 4.80^{+0.10}_{-0.11} $ 

$ 26(\pm5{\text{%}}) $ 

$ 2.51\sim3.14 $ 

27.5 $ 4.80^{+0.10}_{-0.11} $ 

$ 26(\pm5{\text{%}}) $ 

$ 2.52\sim3.15 $ 

27.4 $ (0,2\{1,1\}) $ 

$ J_{10,\mu\nu}^{A(S)} $ 

$ 1^{+-} $ 

$ 4.83^{+0.10}_{-0.11} $ 

$ 28(\pm5{\text{%}}) $ 

$ 3.06\sim3.82 $ 

28.6 $ 4.83^{+0.10}_{-0.12} $ 

$ 28(\pm5{\text{%}}) $ 

$ 3.08\sim3.82 $ 

28.3 $ (0,2\{2,0\}) $ 

$ J_{5,\mu\nu}^{A(S)} $ 

$ 1^{++} $ 

$ 5.16^{+0.12}_{-0.13} $ 

$ 30(\pm5{\text{%}}) $ 

$ 2.94\sim3.90 $ 

41.4 $ 4.69\pm0.09 $ 

$ 24(\pm5{\text{%}}) $ 

$ 2.22\sim2.82 $ 

27.5 $ (0,2\{2,0\}) $ 

$ J_{11,\mu\nu}^{A(S)} $ 

$ 1^{+-} $ 

$ 5.19^{+0.12}_{-0.13} $ 

$ 30(\pm5{\text{%}}) $ 

$ 3.55\sim3.92 $ 

43.4 $ 4.67\pm0.09 $ 

$ 23(\pm5{\text{%}}) $ 

$ 2.69\sim2.87 $ 

21.6 $ (1,1)_\mathrm{mix} $ 

$ J_{2,\mu\nu}^{A(S)}+J_{3,\mu\nu}^{A(S)} $ 

$ 1^{++} $ 

$ 4.80\pm0.10 $ 

$ 27(\pm5{\text{%}}) $ 

$ 3.01\sim3.76 $ 

24.1 $ 4.93^{+0.09}_{-0.10} $ 

$ 29(\pm5{\text{%}}) $ 

$ 3.22\sim4.02 $ 

38.4 $ (1,1)_\mathrm{mix} $ 

$ J_{8,\mu\nu}^{A(S)}+J_{9,\mu\nu}^{A(S)} $ 

$ 1^{+-} $ 

$ 4.80^{+0.11}_{-0.13} $ 

$ 26(\pm5{\text{%}}) $ 

$ 2.71\sim3.13 $ 

30.2 $ 4.94\pm0.10 $ 

$ 29(\pm5{\text{%}}) $ 

$ 3.37\sim4.21 $ 

38.2 Table 2. Hadron masses of the
$ cs\bar{c}\bar{s} $ tetraquark states with different$ J^{PC} $ quantum numbers and$ (L_\lambda,L_\rho\{l_{\rho_1},l_{\rho_2}\}) $ excitation structures. The subscripts "A" and "S" denote the numerical results for the color antisymmetric and symmetric currents, respectively.Except for
$ J_{5,\mu\nu}^{A(S)}(x) $ ,$ J_{11,\mu\nu}^{A(S)}(x) $ , and$ J_{4,\mu\nu}^{A(S)}(x) $ , the interpolating currents exhibit very different mass sum rule behaviors. As shown in the left panel for$ J_{1,\mu\nu}^S(x) $ , the extracted hadron mass increases monotonically with the continuum threshold$ s_0 $ . Thus, one is not able to find an optimal value of$ s_0 $ to minimize the variation of the hadron mass with respect to$ M_B^2 $ . For such a situation, we define the following hadron mass$ \bar{m}_X $ and quantity$ \chi^2(s_0) $ to study the stability of the mass sum rules:$ \bar{m}_X(s_0)=\sum\limits^N_{i=1}\frac{m_X(s_0,M_{{\rm B},i}^2)}{N}, $

(19) $ \chi^2(s_0)=\sum\limits^N_{i=1}\left[\frac{m_X(s_0,M_{{\rm B},i}^2)}{\bar{m}_X(s_0)}-1\right]^2, $

(20) where
$M_{{\rm B},i}^2(i=1,2,\dots,N)$ represents N definite values for the Borel parameter$M_{\rm B}^2$ in the Borel window. According to the above definition, the optimal choice for the continuum threshold$ s_0 $ in the QCD sum rule analysis can be obtained by minimizing the quantity$ \chi^2(s_0) $ , which is a function of only$ s_0 $ . This relation is shown in the right panel of Fig. 4, in which there is a minimum point at approximately$ s_0\approx28.0\;\mathrm{GeV}^2 $ . We can thus determine the working range for the continuum threshold to be$ s_0=(28.0\pm1.4)\;\mathrm{GeV}^2 $ , as shown in the left panel of Fig. 4. The hadron mass is thus obtained as
Figure 4. (color online) Mass curves (left) and
$ \chi^2 $ curve (right) for the current$ J_{1,\mu\nu}^S(x) $ with$ J^{PC}=1^{++} $ .$ \begin{equation} m_{J_{1}^S}=4.91_{-0.12}^{+0.11} \; \text{GeV}. \end{equation} $

(21) In these analyses, we find that the OPE series for the
$ J_{4,\mu\nu}^{A(S)}(x) $ and$ J_{10,\mu\nu}^{A(S)}(x) $ belonging to the$ (0,2\{1,1\}) $ structure differ significantly from those of other interpolating currents. As shown in the Appendix, the quark condensate does not contribute to the correlation function for any of the$ (0,2\{1,1\}) $ currents.By performing similar analyses, we obtain the numerical results for all the other interpolating currents in Eqs. (6) and (7), and they are presented in Table 2. The extracted hadron masses from
$ J_{1,\mu\nu}^{A}(x) $ and$ J_{5,\mu\nu}^{S}(x) $ with$ J^{PC}=1^{++} $ agree well with the mass of the newly observed resonance$ X(4685) $ , implying that$ X(4685) $ can be interpreted as a D-wave$ cs\bar{c}\bar{s} $ tetraquark state with$ J^{PC}=1^{++} $ in the excitation mode of$ (2,0\{0,0\}) $ or$ (0,2\{2,0\}) $ .$ J^{PC} $ 

S-wave P-wave $ 1^{++} $ 

$ D_{s0}^*\bar{D}_{s1},D_{s}\bar{D}_s^*,D_{s}\bar{D}_{s1}^*, $ 

$ D_s\bar{D}_{s1},D_{s0}^*\bar{D}_{s}^*,D_{s0}^*\bar{D}_{s1}^*, $ 

$ D_{s1}\bar{D}_{s1},D_{s1}\bar{D}_{s2}^*, $ 

$ D_{s}^*\bar{D}_{s1},D_{s1}^*\bar{D}_{s1},D_s^*\bar{D}_{s2}^*, $ 

$ J/\psi\phi $ 

$ D_{s1}^*\bar{D}_{s2}^*,h_c(1P)\phi $ 

$ 1^{+-} $ 

$ D_{s0}^*\bar{D}_{s1},D_{s}\bar{D}_s^*,D_{s}\bar{D}_{s1}^*, $ 

$ D_s\bar{D}_{s1},D_{s0}^*\bar{D}_{s}^*,D_{s0}^*\bar{D}_{s1}^*, $ 

$ D_{s1}\bar{D}_{s1},D_{s1}\bar{D}_{s2}^*, $ 

$ D_{s}^*\bar{D}_{s1},D_{s1}^*\bar{D}_{s1},D_s^*\bar{D}_{s2}^*, $ 

$ \eta_c\phi $ 

$ \chi_{c0}(1P)\phi,\chi_{c1}(1P)\phi $ 

Table 3. Possible decay channels of the D-wave
$ cs\bar{c}\bar{s} $ tetraquark states with$ J^{PC}=1^{++} $ and$ 1^{+-} $ .Considering the same physical picture for the
$ (1,1\{1,0\}) $ and$ (1,1\{0,1\}) $ excitation structures, the interpolating currents$ J_{2,\mu\nu}^{A(S)}(x) $ and$ J_{3,\mu\nu}^{A(S)}(x) $ exhibit similar mass sum rules. The currents$ J_{2,\mu\nu}^{A}(x) $ and$ J_{3,\mu\nu}^{A}(x) $ give almost degenerate hadron masses, as shown in Table 2. To study their mixing effects, we also perform analyses for the mixed currents$ J_{2,\mu\nu}^{A(S)}+J_{3,\mu\nu}^{A(S)} $ . Our calculations show that the off-diagonal correlator$ \Pi_{23}^{A(S)}(q^2) $ is nonzero, implying that the currents$ J_{2,\mu\nu}^{A}(x) $ and$ J_{3,\mu\nu}^{A}(x) $ may couple to the same hadron state. The same situation arises for the interpolating currents$ J_{8,\mu\nu}^{A(S)}(x) $ and$ J_{9,\mu\nu}^{A(S)}(x) $ , which couple to the same tetraquark state. -
We investigated the mass spectra for the D-wave
$ cs\bar{c}\bar{s} $ tetraquark states with$ J^{PC}=1^{++} $ and$ 1^{+-} $ in the framework of QCD sum rules. We constructed the D-wave non-local interpolating tetraquark currents with covariant derivative operators in the $ (L_\lambda,L_\rho\{l_{\rho_1},l_{\rho_2}\})=(2,0\{0,0\}), (1,1\{1,0\}), (1,1\{0,1\}),(0,2\{1,1\}),(0,2\{2,0\}),(0,2\{0,2\}) $ excitation structures. The two-point correlation functions were calculated up to dimension ten condensates in the leading order of$ \alpha_s $ . We established reliable mass sum rules for all these currents and obtained the mass spectra of D-wave$ cs\bar{c}\bar{s} $ tetraquarks, as shown in Table 2. Our results support the interpretation of the recently observed$ X(4685) $ structure as a D-wave$ cs\bar{c}\bar{s} $ tetraquark state with$ J^{PC}=1^{++} $ in the$ (2,0\{0,0\}) $ or$ (0,2\{2,0\}) $ excitation mode. However, some other possibilities of the excitation modes cannot be excluded by our results within errors.The mass spectra of
$ cs\bar c\bar s $ tetraquark states in different color configurations were studied in Ref. [35], and the results indicated that the masses of color symmetric tetraquarks are lower than those of color antisymmetric tetraquarks in the ground state ($ L=0 $ ). Similar results were obtained for the fully heavy tetraquark states [56–58]. However, the situation is different for the excited$ cs\bar c\bar s $ tetraquarks: the masses of color antisymmetric tetraquarks are lower than those of color symmetric tetraquarks. Such behavior is consistent with our results in Table 2 for the D-wave$ cs\bar c\bar s $ tetraquarks, except for those in the$ (0,2\{2,0\}) $ structures with two ρ-mode excitations. In Table 2, the masses for the positive$ \mathbb{C} $ -parity tetraquarks follow the relation$6_{\rho\rho} < 3_{\lambda\lambda} < 3_{\lambda\rho} < 3_{\rho\rho}$ , and those for the negative$ \mathbb{C} $ -parity tetraquarks exhibit the relation$6_{\rho\rho} < 3_{\lambda\lambda} < 6_{\lambda\lambda} < 3_{\rho\rho}$ , which is consistent with the conclusion for P-wave$ cc\bar c\bar c $ systems [57].We present the mass spectra of these
$ cs\bar{c}\bar{s} $ tetraquarks in comparison with the corresponding two-meson open-charm mass thresholds in Fig. 5. Clearly, these D-wave$ cs\bar{c}\bar{s} $ tetraquarks with$ J^{PC}=1^{++} $ and$ 1^{+-} $ lie above the mass thresholds of$ D_s\bar{D}_s^* $ ,$ J/\psi\phi $ , and$ \eta_c\phi $ . Accordingly, we present their possible decay channels in both the S-wave and P-wave in Table 3. We suggest searching for these D-wave$ cs\bar{c}\bar{s} $ tetraquarks in both the hidden-charm channels$ J/\psi\phi $ and$ \eta_c\phi $ , as well as open-charm channels such as$ D_s\bar{D}_s^* $ and$ D_{s}\bar{D}_{s1}^* $ . -
The spectral functions for the D-wave interpolating current
$ J_{i}^{A(S)} $ can be written as$ \begin{aligned}[b] \rho_{i;A(S)}(s) =& \rho^{\rm pert}_{i;A(S)}(s)+\langle \bar{s}s\rangle\rho^{\langle \bar{s}s\rangle}_{i;A(S)}(s)+m_s\langle\bar{s}s\rangle\rho^{m_s\langle \bar{s}s\rangle}_{i;A(S)}(s)+\langle g_s^2G^2\rangle\rho^{\langle g_s^2G^2\rangle}_{i;A(S)}(s)+\langle \bar{s}\sigma\cdot G s\rangle\rho^{\langle \bar{s}\sigma\cdot G s\rangle}_{i;A(S)}(s)+m_s\langle \bar{s}\sigma\cdot G s\rangle\rho^{m_s\langle \bar{s}\sigma\cdot G s\rangle}_{i;A(S)}(s)\\ & +\langle \bar{s}s \bar{s}s\rangle\rho^{\langle \bar{s}s \bar{s}s\rangle}_{i;A(S)}(s)+\langle \bar{s}s\rangle\langle \bar{s}\sigma\cdot G s\rangle\rho^{\langle \bar{s}s\rangle\langle \bar{s}\sigma\cdot G s\rangle}_{i;A(S)}(s)+\langle g_s^2G^2\rangle\langle \bar{s}s\rangle\rho^{\langle g_s^2G^2\rangle\langle \bar{s}s\rangle}_{i;A(S)}(s)+m_s\langle g_s^2G^2\rangle\langle \bar{s}s\rangle\rho^{m_s\langle g_s^2G^2\rangle\langle \bar{s}s\rangle}_{i;A(S)}(s)\\ & +\langle g_s^2G^2\rangle^2\rho^{\langle g_s^2G^2\rangle^2}_{i;A(S)}(s)+\langle g_s^2G^2\rangle\langle \bar{s}\sigma\cdot G s\rangle\rho^{\langle g_s^2G^2\rangle\langle \bar{s}\sigma\cdot G s\rangle}_{i;A(S)}(s)+m_s\langle g_s^2G^2\rangle\langle \bar{s}\sigma\cdot G s\rangle\rho^{m_s\langle g_s^2G^2\rangle\langle \bar{s}\sigma\cdot G s\rangle}_{i;A(S)}(s). \\\end{aligned} $ 
(22) The spectral functions for the
$ (2,0\{0,0\}) $ structure are given as follows:$ \begin{aligned}[b]\rho^{\rm pert}_{1,7;A(S)}(s) =& -\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{x}{1612800 \pi ^5 (y-1)^5} F\left(s,x,y\right){}^3 c_1 \left(2 (x-1) \left(10 ((x (5 (13 x-42) x+273)-140) x\right.\right.\\ & \left.\left. +35)y^2-28 \left(39 x^2-45 x+20\right) y-21 x (2 x+5)+\left(10 ((x (2 (x-35) x+189)-140) x+35) y^2+28 (x ((15 x\right.\right.\right.\\ & \left.\left.\left.-74)x+70)-20) y+21 ((23 x-30) x+10)\right) c_p+210\right) x^2 y F\left(s,x,y\right){}^3+42 x \left((x-1) (y-1) \left(10 ((x (5 (13 x\right.\right.\right.\\ & \left.\left.-42) x+273)-140) x+35) y^4-((x (50 x+923)-1165) x+590) y^3+2 ((32 x-165) x+190) y^2+60 (x)\right.\right.\\ & \left.\left. -2) y+10s x+\left(-4 ((x ((59 x-184) x+195)-90) x+15) x y^3+((x (58 (x-3) x+195)-120) x+30) y^2\right.\right.\right.\\& \left.\left.\left.+2 (3 (16 x-45)x+110) x y-60 y+5 (3 x-8) x+30\right) m_c m_s+c_p \left((x-1) (y-1) \left(10 ((x (2 (x-35) x+189)\right.\right.\right.\right.\\& \left.\left.\left.\left.-140) x+35) y^2+((450 x-2183) x+2065) x y-590 y+24 (23 x-30) x+240\right) s x y^2+\left(-4 (x (4 (x-5) x\right.\right.\right.\right.\\ & +35)-15) (x-1) x y^3+(((4 (64-13 x) x-245) x+20) x+30) y^2-2 \left(37 x^3-60 x+30\right) y+55 x^2-80 x\\ & \left.\left.\left.\left.\left.+30\right) m_c m_s\right)\right)\right. F\left(s,x,y\right){}^2+15 (y-1) \left((x-1) (y-1) \left(50 ((x (5 (13 x-42) x+273)-140) x+35) y^4\right.\right.\right.\\ & \left.\left.\left.-14 ((x (10 x+361)-455) x+230) y^3+7 ((79 x-310) x+330) y^2+420 (x-2) y+70\right) s^2 y x^2+14 m_c m_s\right.\right.\\ & \left(\left(-11 ((x ((59 x-184) x+195)\right.\right.-90) x+15) x y^4+((x (2 (96 x-325) x+765)-440) x+105) y^3+(x ((153 x\\ & \left.\left.\left.\left.-485) x+430)-150) y^2+5 ((7 x-12) x+12) y+5 (x-3)\right) s x+\left(6 (4 (2 x ((2 x-5) x+5)-5) x+5) y^2\right.\right.\right.\right.\\ \end{aligned} $

$ \begin{aligned}[b] & \left.\left.\left.\left.+5 (7 (x-4) x+30) x y-60 y+10 (x-3) x+30\right) m_c m_s\right)+c_p \left((x-1) (y-1) \left(50 ((x (2 (x-35) x+189)\right.\right.\right.\right.\\ & \left.-140) x+35) y^2+14 (x ((180 x-851) x+805)-230) y+147 ((23 x-30) x+10)\right) s^2 x^2 y^3+7 m_c m_s \left(\left(-22\right.\right.\\ & (x (4 (x-5) x+35)-15) (x-1) x y^3-2 (x (((143 x-680) x+595) x+20)-105) y^2-((x (359 x+240)\\ & \left.-930) x+420) y+35 ((11 x-16) x+6)\right) s x y+2 \left(2 (4 (2 x ((x-5) x+10)-15) x+15) y^2+5 (11 (x-4) x\right. \\ & \left.\left.\left.\left.\left.+42) x y-60 y+10 (7 x-9) x+30\right) m_c m_s\right)\right)\right)F\left(s,x,y\right)+60 (y-1)^2 s \left((x-1) (y-1) \left(4 ((x (5 (13 x-42) x\right.\right.\right.\\& \left.\left.\left.+273)-140) x+35) y^3-56 (2 (4 x-5) x+5) y^2+21 ((3 x-10) x+10) y+35 (x-2)\right) s^2 x^2 y^3+2 \left((x-1) \right.\right.\right.\\ & \left.(y-1) \left(2 ((x (2 (x-35) x+189)-140) x+35) y^2\right.+14 (x ((8 x-37) x+35)-10) y+7 (23 x-30) x+70\right)\\ & \left. s^2 x^2 y^3+7 \left(2 \left(2 (4 (2 x ((x-5) x+10)-15) x+15) y^2\right.\right.+5 (11 (x-4) x+42) x y-60 y+10 (7 x-9) x+30\right)\\ & \left.\left. m_c m_s-s x y (x y-1) \left(55 x^2-80 x+2 (x (4 (x-5) x+35)\right. -15)(x-1) y^2+2 (x ((17 x-80) x+90)-30) y\right.\right.\\ & \left.\left.\left.+30\right)\right) m_c m_s\right) c_p y+7 m_c m_s \left(\left(-4 ((x ((59 x-184) x+195)\right.\right.-90) x+15) x y^4+4 ((x-2) ((17 x-25) x+25) x\\ & \left.\left.\left.\left.+15) y^3+(x ((53 x-210) x+250)-120) y^2+5 ((7 x-20) x+18) y+20 x-30\right) s x y+2 \left(12 (4 (2 x ((2 x-5)\right.\right.\right.\right.\\ & \left.\left.\left.\left. x+5)-5) x+5) y^3+10 (x (5 (x-4) x+24)-12) y^2+30 ((x-3) x+3) y+15 (x-2)\right) m_c m_s\right)\right)\right),\\ \rho^{\langle\bar{s}s\rangle}_{1,7;A(S)}(s) =& -\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y \frac{c_1 x m_c}{96 \pi ^3 (y-1)^4} F\left(s,x,y\right){}^2 \left(2 s (y-1) \left((x-1) y^2 \left((x (26 x-1)-14) c_p-22 x^2+50 x\right.\right.\right.\\ & \left.\left.-10\Big)+11 x y^4 \left(x^3 \left(c_p-23\right)-2 x \left(c_p+5\right)+c_p+7 x^4+24 x^2+1\right)-y^3 \left((x (x (11 x+37)-19)-7) (x-1)\right.\right.\right.\\ & \left.\left.\left. c_p-2 ((21 x-40) x+28) x+7\right)-(x-1) y \left(7 (x-1) c_p+10 x-4\right)-x+1\right) F\left(s,x,y\right)+\left((x-1) (y-1) c_p\right.\right. \\ & \left.\left.\left.\left(2 \left(x^2+x-1\right)\right.x y^2+x (2-5 x) y+x+y-1\right)+2 ((x ((7 x-23) x+24)-10) x+1) x y^3+(x ((5 x-9) x\right.\right.\\& \left.\left.+7)-1) y^2-(x-1)((5 x-9) x+2) y-(x-1)^2\right) F\left(s,x,y\right){}^2+6 s^2 (y-1)^2 y \left(-2 y^3 \left((x (x+2)-4) x^2 c_p\right.\right.\right.\\ & \left.\left.\left.+c_p-2 (2 (x-2) x+3) x+1\right)+(x-1) y^2 \left(2 \left(2 x^2+x-2\right) c_p+x (11-4 x)-4\right)+2 x y^4 \left(x^3 \left(c_p-23\right)\right.\right.\right.\\ & \left.\left.-2 x \left(c_p+5\right)+c_p+7 x^4+24 x^2+1\Big)-(x-1)^2 y \left(2 c_p+3\right)-x+1\right)\right),\\ \rho^{\langle m_s\bar{s}s\rangle}_{1,7;A(S)}(s) =& -\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{c_1}{96 \pi ^3 (y-1)^3} F\left(s,x,y\right) \left(-2 F\left(s,x,y\right){}^2 \left(m_c^2 \left(5 x^2 c_p+y^2 \left(x^4 \left(c_p+9\right)-4 x^3 \left(c_p+5\right)\right.\right.\right.\right.\\ & \left.+2 x^2 \left(5 c_p+9\right)-8 x \left(c_p+1\right)+2 \left(c_p+1\right)\right)+2 y \left((x ((x-7) x+7)-2) c_p+((x-5) x+5) x-2\right)-6 x c_p\\ & \left.\left.\left.+2 c_p+x^2-2 x+2\right)-s (x-1) x (y-1) \left(35 y^4 \left(((x ((x-4) x+10)-8) x+2) c_p+(3 x ((x-4) x+6)-8) x\right.\right.\right.\right.\\ & \left.\left.\left.+2\Big)+y^3 \left(59 (x ((x-7) x+7)-2) c_p+(233-x (81 x+233)) x-118\right)+2 y^2 \left(12 ((5 x-6) x+2) c_p+(14 x\right.\right.\right.\right.\\ & \left.\left.\left.\left.-33) x+38\right)+12 (x-2) y+2\right)\right)-3 s (y-1) F\left(s,x,y\right) \left(2 m_c^2 \left(2 y^3 \left(x^4 \left(c_p+9\right)-4 x^3 \left(c_p+5\right)+2 x^2 \left(5 c_p\right.\right.\right.\right.\right.\\& \left.+9\Big)-8 x \left(c_p+1\right)+2 \left(c_p+1\right)\right)+4 y^2 \left((x ((x-7) x+7)-2) c_p+x (x-2)^2-2\right)+x y \Big(2 (5 x-6) c_p+3 (x\\ & \left.\left.\left.-2)\Big)+y \left(4 c_p+6\right)+x-2\right)-s (x-1) x (y-1) y \left(25 y^4 \left(((x ((x-4) x+10)-8) x+2) c_p+(3 x ((x-4) x\right.\right.\right.\right.\\ & \left.\left.\left.+6)-8) x+2\Big)+2 y^3 \left(23 (x ((x-7) x+7)-2) c_p+(91-x (27 x+91)) x-46\right)+y^2 \left(21 ((5 x-6) x+2) c_p\right.\right.\right.\right.\\ & \left.\left.\left.+(29 x-62) x+66\Big)+12 (x-2) y+2\right)\right)+(x-1) x y \left(5 y^2 \Big(((x ((x-4) x+10)-8) x+2) c_p+(3 x ((x-4) x\right.\right.\\ & \left.\left.+6)-8) x+2\Big)+4 y \left(2 (x ((x-7) x+7)-2) c_p-3 (x (x+3)-3) x-4\right)+3 ((5 x-6) x+2) c_p-3 x+6\right)\right.\\ & \left. F\left(s,x,y\right){}^3+6 s^3 (x-1) x (y-1)^3 y^3 \left(2 y^3 \left(((x ((x-4) x+10)-8) x+2) c_p+(3 x ((x-4) x+6)-8) x+2\right)\right.\right.\\ & \left.\left.\left.+4 y^2 \left((x ((x\right.-7) x+7)-2) c_p-(x (x+4)-4) x-2\right)+x y \left(2 (5 x-6) c_p+3 (x-2)\right)+y \left(4 c_p+6\right)+x-2\right)\right),\\ \rho^{\langle g_sG^2\rangle}_{1,7;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{max}}_{y_{\min}}{\rm d}y \frac{x^2 c_1 m_c}{1935360 \pi ^5 (x-1)^3 (y-1)^5} \left(-2 (x-1) \left(2 (y ((x ((x-3) x+3) y-3) y+3)-1) \left(10 \left(\left(2 c_p\right.\right.\right.\right.\right.\\ & \left.\left.\left.\left.+65\Big) x^4-70 \left(c_p+3\right) x^3+21 \left(9 c_p+13\right) x^2-140 \left(c_p+1\right) x+35 \left(c_p+1\right)\right) y^2+28 \left(-39 x^2+45 x+(x\right.\right.\right.\right.\\ & \left.\left.\left.\left.((15 x-74) x+70)-20) c_p-20\right) y+21 \left((2 x+5) (-x)+((23 x-30) x+10) c_p+10\right)\right) x y m_c+21 \left(-4\right.\right.\right.\\ \end{aligned} $

$ \begin{aligned}[b] & \left.\Big(\left(\left(x \left(4 x^2\right.\right.\right.\right.-34 x+105\Big)-125\Big) x+45\Big) c_p (x-1)^2+(x ((x ((59 x-327) x+762)-949) x+660)-255) x\\ & +45\Big) x y^5+\left(3 (x (2 ((-7 (x-8) x-116) x+61) x+65)-120) x+(150-x ((4 (x (23 (x-8) x+494)-554) x\right.\\ & \left.+805) x+220)) c_p+150\right) y^4-2 \left((3 x ((-5 (x-13) x-218) x+300)-650) x+((x ((5 (17 x-59) x+141) x\right.\\ & \left.+535)-660) x+210) c_p+210\right) y^3+2 \left((x ((50 x-279) x+570)-540) x+((x (4 (5 x-61) x+725)-690)\right.\\ & x+210)c_p+210\Big) y^2+6 \left(((11 x-45) x+60) x+2 (x (8 (x-5) x+45)-15) c_p-30\right) y+5 \left((3 x-8) x\right.\\ & \left.\left.\left.\left.+((11 x-16) x+6) c_p+6\right)\right) m_s\right) F\left(s,x,y\right){}^3+21 \left(2 (y ((x ((x-3) x+3) y-3) y+3)-1) \left(4 \left((x ((59 x-184)\right.\right.\right.\right.\\ & \left.x+195)-90) x+(x (4 (x-5) x+35)-15) (x-1) c_p+15\right) x y^3+\left(((-58 (x-3) x-195) x+120) x+(x\right.\\ & \left.(x (4 (13 x-64) x+245)-20)-30) c_p-30\right) y^2+2 \left((3 (45-16 x) x-110) x+\left(37 x^3-60 x+30\right) c_p+30\right)\\& \left.\left.\left.y-30 \left(c_p+1\right)-5 x^2 \left(11 c_p\right.+3\Big)+40 \left(2 c_p+1\right) x\right) m_s m_c^2-2 s (x-1) x (y-1) (y ((x ((x-3) x+3) y-3) y\right.\right. \\ & \left.+3)-1) \left(10 \left(\left(2 c_p+65\right) x^4-70\right.\right.\left(c_p+3\right) x^3+21 \left(9 c_p+13\right) x^2-140 \left(c_p+1\right) x+35 \left(c_p+1\right)\right) y^4\\ & \left.\left.\left.+\left(50 \left(9 c_p-1\right) x^3-\left(2183 c_p+923\right) x^2\right.+5 \left(413 c_p+233\right) x-590 \left(c_p+1\right)\right) y^3+\left(8 \left(69 c_p+8\right) x^2\right.\right.\right.\\& \left.\left.\left.\left.-30 \left(24 c_p+11\right) x+240 c_p+380\right) y^2+60 (x-2) y+10\right) m_c+3 (x-1) (y-1) \left(22 \left(4 c_p+59\right) y^6 x^7\right.\right.\right.\\ & \left.\left.\left.\left.+2 \left(-33 \left(14 c_p+109\right) y+253 c_p+83\right) y^5 x^6+\left(66 \left(59 c_p\right.\right.+254\Big) y^2-16 \left(253 c_p+83\right) y+935 c_p+10\right) y^4\right.\right.\right.\\ & \left.\left.\left. x^5-y^3 \left(2 (11 y (949 y-109)-670) y+11 \left(\left(738 y^2-988 y\right.\right.\right.+295\Big) y+20\Big) c_p+470\Big) x^4+\left(6 ((20 y (121 y\right.\right.\right.\right.\\ & -2)-913) y+408) y+(y ((20 (440 y-607) y+1407) y+2828)-576) c_p-286\Big) y^2 x^3-5 y \left(2 (y ((y (561 y\right.\\ & \left.+205)-794) y+513)-147) y+((y (2 (y (473 y-427)-644) y+1739)-609) y+77) c_p+30\right) x^2+10\\ & \left. \left(y \left((y ((11 y (9 y+20)-630) y+560)-251) y+(y ((y (99 y+235)\right.\right.-548) y+292)-56) (y-1) c_p+52\right)\\ & \left.\left.\left.\left.\left.-4\right) x-30 (y-1) \left(\left(((29 y-50) y+39) y+(y-1) ((29 y-25) y+7) c_p\right.\right.-14\right) y+2\right)\right) s m_s\right)F\left(s,x,y\right){}^2 \\ & +6 (y-1) s \left(7 (y ((x ((x-3) x+3) y-3) y+3)-1) \left(22 \left(4 c_p+59\right) y^4 x^5\right.\right.-2 y^3 \Big(88 \left(3 c_p+23\right) y-143 c_p\\ & \left.\left.\left.+192\Big) x^4+\left(110 \left(11 c_p+39\right) y^2+20 \left(65-68 c_p\right) y+359 c_p-306\right) y^2 x^3-5 y \Big(44 \left(5 c_p+9\right) y^3+34\right.\right.\right.\\ & \left(9-7 c_p\right) y^2-2 \left(24 c_p+97\right) y+77 c_p+14\Big) x^2+10 \Big(y \Big(33 \left(c_p+1\right) y^3+4 \Big(c_p+22\Big) y^2-\left(93 c_p+86\right) y\\ & \left.\left. +56 c_p+12\Big)-1\Big) x-30 (y-1) \left(\left(7 \left(c_p+1\right) y-7 c_p-3\right) y+1\right)\Big) m_s m_c^2-s (x-1)x (y-1) y (y ((x ((x-3) x\right.\right.\\ & \left.\left.\left.\left.+3) y-3) y+3)-1) \left(\left(50 \left(2 c_p+65\right) y^3 x^4-140 y^2 \left(25 \left(c_p+3\right) y-18 c_p+1\right) x^3\right.\right.+7 \Big(150 \left(9 c_p+13\right) y^2\right.\right.\right.\right.\\ & \left.\left.\left.\left.\left.\left.-2 \left(851 c_p+361\right) y+483 c_p+79\Big) y x^2+70 \left(\left(-100 \left(c_p+1\right) y^2+7 \left(23 c_p+13\right) y\right.\right.-63 c_p-31\right) y+6\right) x\right.\right.\right.\right.\\ & \left.\left.\left.\left.\left.+70 (y-1) \left((25 y-21) \left(c_p+1\right) y+12\right)\right) y+70\right) m_c+21 (x-1) (y-1) \left(4 \left(4 c_p+59\right)\right.y^6 x^7+4 \Big(-327 y\right.\right.\right.\\ & \left.\left.\left.+(23-42 y) c_p+8\Big) y^5 x^6+\left(8 (381 y-32) y+2 \left(354 y^2-368 y+85\right) c_p+15\right) y^4 x^5-y^3 \Big((4 y (949 y\right.\right.\right.\\ & \left.-119)-195) y+2 \left(\left(738 y^2-988 y+295\right) y+20\right) c_p+90\Big) x^4+\left(3 ((4 y (220 y-7)-323) y\right.+168) y\right.\\ & \left.+2 (y ((4 (200 y-273) y+93) y+292)-64) c_p-68\right) y^2 x^3-5 y \left((y (4 (y (51 y+19)-78) y+231)-75) y\right.\\ & \left.+2 ((y (2 (y (43 y-35)-72) y+193)-75) y+11) c_p+9\right) x^2+10 \left(y \left((y ((2 y (9 y+22)-135) y+136)\right.\right.\\ & \left.\left.-70) y+2 (y ((y (9 y+25)-60) y+36)-8) (y-1) c_p+18\right)-2\right) x-30 (y-1) (3 (y-1) y+1) \left(2 (y-1)\right.\\ & \left.\left.\left.\left. \left(c_p+1\right) y+1\right)\right) s y m_s\right) F\left(s,x,y\right)+6 (y ((x ((x-3) x+3) y-3) y+3)-1) (y-1)^2 \left(7 \left(4 \left(4 c_p+59\right) y^4 x^5-4\right.\right.\right.\\ & \left.\left.\left. y^3 \left(8 \left(3 c_p+23\right) y-13 c_p+17\right) x^4+\left(4 (195 y+59) y+(4 (55 y-56) y+42) c_p-53\right) y^2 x^3-5 y \left(6 (2 y (6 y\right.\right.\right.\right.\\ & \left.+5)-7) y+(4 (y (10 y-7)-8) y+22) c_p+7\right) x^2+10 \left(6 \left(c_p+1\right) y^4+4 \left(2 c_p+5\right) y^3-5 \left(6 c_p+5\right) y^2+2 \right.\\ & \left.\left.\left.\left.\left(8 c_p+5\right) y-2\right) x-30 (y-1) \left(2 (y-1) \left(c_p+1\right) y+1\right)\right) m_c m_s-s (x-1) x (y-1) y^2 \left(4 \left(2 c_p+65\right) y^3 x^4\right.\right.\right.\\ & -56 y^2 \left(5 y \left(c_p+3\right)-4 c_p\right) x^3+7 \left(12 \left(9 c_p+13\right) y^2-4 \left(37 c_p+16\right) y+46 c_p+9\right) y x^2+35 \left(1-2 y\right.\\ \end{aligned} $

$ \begin{aligned}[b] &\left.\left.\left.\left.\left. \left(8 (y-1) y\right.+2 (4 y-3) (y-1) c_p+3\right)\right) x+70 (y-1) \left(2 (y-1) \left(c_p+1\right) y+1\right)\right)\right) s^2 y m_c\right),\\ \rho^{\langle\bar{s}\sigma\cdot Gs\rangle}_{1,7;A(S)}(s) =& -\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{ \frac{c_1 m_c}{192 \pi ^3 (y-1)^3} F\left(s,x,y\right) \left(3 s (y-1) \left(y \left(x^2 \left(7 c_p-38\right)-7 c_p+44 x-4\right)+11 x y^4 \left(9 x^3\right.\right.\right. \\ & \left.\left.\left.\left(c_p-7\right)-12 x^2 \left(c_p-5\right)+2 x \left(c_p-11\right)+c_p+19 x^4+1\right)+y^3 \left(-(x-1) (x (11 x (9 x+5)-59)-7) c_p\right.\right.\right.\\ & \left.\left.\left.+2 ((12 x (x+4)-97) x+68) x-7\right)+(x-1) y^2 \left((11 x (8 x-5)-14) c_p-2 (6 (5 x-12) x+5)\right)-3 x+1\right)\right.\\ & \left. F\left(s,x,y\right)+2 \left((x-1) (y-1) c_p (x (y (2 x ((9 x-3) y-8)-2 y+9)-1)+y-1)+y \left(x \left(2 ((x ((19 x-63) x\right.\right.\right.\right.\\ & \left.\left.\left.\left.+60)-22) x+1) y^2+((2 x (x+7)-21) x+16) y-10 (x-3) x-22\right)-y\right)+x (4-3 x)+2 y-1\right) F(s,x,y)^2\right.\\ & \left.+6 s^2 (y-1)^2 y \left(2 x y^4 \left(9 x^3 \left(c_p-7\right)-12 x^2 \left(c_p-5\right)+2 x \left(c_p-11\right)+c_p+19 x^4+1\right)-2 y^3 \left((x (9 x-4)-1)\right.\right.\right. \\ & \left.(x-1) (x+1) c_p-2 ((x (x+5)-10) x+7) x+1\right)+(x-1) y^2 \left(2 (x (8 x-5)-2) c_p+x (31-12 x)-4\right)\\ & \left.\left.+2 x y \left(x \left(c_p-5\right)+7\right)-y \left(2 c_p+3\right)-2 x+1\right)\right)+\frac{c_2 x m_c}{128 \pi ^3 (x-1) (y-1)^4} F\left(s,x,y\right) \left(3 s (y-1) \left(-2 y^4 \left(\left(55 x^2\right.\right.\right.\right.\\ & \left.\left.-75 x+31\right) (x-1)^2 c_p+(x ((10 x-83) x+134)-103) x+31\right)+11 y^5 \left(((5 x-6) x+2) (x-1)^2 c_p\right.\\ & \left.\left.\left.+(x ((x ((7 x-30) x+51)-48) x+27)-10) x+2\right)+y^3 \left(((55 x-102) x+65) (x-1)^2 c_p-2 x ((2 x (x+17)\right.\right.\right.\\ & \left.\left.\left.-95) x+102)+75\right)+2 (x-1) y^2 \left((9 x-16) (x-1) c_p+(9 x-32) x+24\right)+x y \left(7 (x-2) c_p+11 x-26\right)\right.\right.\\ & \left.\left.+y \left(7 c_p+15\right)+2 x-2\right) F\left(s,x,y\right)+2 \left((x-1)^2 (y-1)^2 c_p (y (x (2 (5 x-6) y+3)+4 y-3)+1)+2 ((x ((x\right.\right. \\ & ((7 x-30) x+51)-48) x+27)-10) x+2) y^4+(x (((37-6 x) x-55) x+39)-11) y^3+(11-x ((2 x (x+4)\\ & \left.\left.-27) x+30)) y^2+(3 x-5) (x-1)^2 y+(x-1)^2\right) F\left(s,x,y\right){}^2+6 s^2 (y-1)^2 y \left(2 y^5 \left(((5 x-6) x+2) (x-1)^2 c_p\right.\right.\right.\\ & \left.+(x ((x ((7 x-30) x+51)-48) x+27)-10) x+2\right)-4 y^4 \left(((5 x-7) x+3) (x-1)^2 c_p+(x ((x-8) x+13)\right.\\ & -10) x+3\Big)+2 y^3 \left((5 (x-2) x+7) (x-1)^2 c_p+((21-8 x) x-22) x+8\right)+(x-1) y^2 \left(4 (x-2) (x-1) c_p\right.\\ & \left.\left.\left.+(4 x-15) x+12\right)+x y \left(2 (x-2) c_p+3 x-8\right)+y \left(2 c_p+5\right)+x-1\right)\right)\Bigg\},\\ \rho^{m_s\langle\bar{s}\sigma\cdot Gs\rangle}_{1,7;A(S)}(s) = &-\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1}{288 \pi ^3 (y-1)^2} \left(3 s (y-1) y F(s,x,y) \left(s (x-1) (y-1) \left(25 y^4 \left(x^4 \left(5 c_p-1\right)-4 x^3\right.\right.\right.\right.\\ & \left. \left(c_p+3\right)+6 x^2 \left(c_p+5\right)-8 x \left(c_p+1\right)+2 \left(c_p+1\right)\right)-2 y^3 \left(((x (42 x+115)-161) x+46) c_p+(x (152 x\right.\\ & \left.+185)-91) x+46\Big)+y^2 \left(21 ((7 x-6) x+2) c_p+(95 x-62) x+66\right)+12 (x-2) y+2\right)-6 x m_c^2 \left(2 c_p (y (x (4\right.\\ & \left.\left.\left. y-5)-2 y+2)+1)+2 y (4 (2 x-3) x y+x+6 y-4)+3\right)\right)+3 F\left(s,x,y\right){}^2 \left(s (x-1) (y-1) \left(35 y^4 \left(x^4 \Big(5 c_p\right.\right.\right.\right.\\ & \left.-1\Big)-4 x^3 \Big(c_p+3\Big)+6 x^2 \left(c_p+5\right)-8 x \left(c_p+1\right)+2 \left(c_p+1\right)\right)-y^3 \left(((x (96 x+295)-413) x+118) c_p+(x \right.\\ & \left.\left.(436 x+475)-233) x+118\right)+2 y^2 \left(12 ((7 x-6) x+2) c_p+(52 x-33) x+38\right)+12 (x-2) y+2\right)-3 x m_c^2\\ & \left.\left.\left. \left(2 y^2 \left(2 x \left(c_p-3\right)\right.\right.-c_p+4 x^2+3\right)-y \left((5 x-2) c_p+x+2\right)+c_p+1\right)\right)+2 (x-1) y \left(-4 y \left((x (x+4)-2)\right.\right.\\ & \left. (3 x-2) c_p+3 (6 x+5) x^2-9 x+4\right)+5 y^2 \Big(x^4 \left(5 c_p-1\right)-4 x^3 \left(c_p+3\right)+6 x^2 \left(c_p+5\right)-8 x \left(c_p+1\right)+2 \\ & \left.\left.\left(c_p+1\right)\Big)+3 ((7 x-6) x+2) c_p+6 x^2-3 x+6\right) F\left(s,x,y\right){}^3+3 s^3 (x-1) (y-1)^3 y^3 \left(2 x^4 y^3 \left(5 c_p-1\right)-8 x^3 \right.\right.\\ & y^2 (y+1) \left(c_p+3\right)+x^2 y \Big(12 y^2\left(c_p+5\right)-4 y \left(5 c_p+8\right)+14 c_p+9\Big)+2 x y \Big(-8 y^2 \left(c_p+1\right)+2 y \left(7 c_p+4\right)\\ & \left.\left. \left.-6 c_p-3\Big)+2 (y-1) \left(2 (y-1) y \Big(c_p\right.+1\Big)+1\right)+x\right)\right)+\frac{c_2}{128 \pi ^3 (y-1)^3} \left(-3 F(s,x,y)^2 \left(m_c^2 \left((y-1) c_p (x y-1)\right.\right.\right.\\ & \left. \left(\left(x^2+x-1\right) y-x+1\right)+y \left(x \left(\left(-x^2+x-3\right) y+(x ((8 x-15) x+9)-1) y^2-2 x+5\right)+y\right)-x-2 y+1\right)\\ & -s x (y-1) \left(y \left(35 x y^4\right.\right.\left(\left(x^3-2 x+1\right) c_p+(3 x ((x-5) x+8)-10) x+1\right)-y^3 \left((x (x (35 x+72)-13)-35)\right.\\ & \left.(x-1) c_p+(x ((11 x+115) x+275)-202) x+35\right)+y^2 \Big((x (37 x+46)-59) (x-1) c_p+5 (x (12 x+47)\\ & \left.\left.\left.-45) x+59\Big)-2 (x-1)^2 y \left(12 c_p+19\right)-11 x+12\right)-1\right)\right)+3 s (y-1) F(s,x,y) \left(s x (y-1) y \left(y \left(25 x y^4 \right.\right.\right.\\ \end{aligned} $

$ \begin{aligned}[b]& \left.\left(\left(x^3-2 x+1\right) c_p+(3 x ((x\right.-5) x+8)-10) x+1\right)-y^3 \Big((x (x (25 x+63)-17)-25) (x-1) c_p+2 (x (x\\& \left.\left.\left.\left.+20) (2 x+5)-74) x+25\Big)+y^2 \left((x (38 x+29)-46) (x-1) c_p+(x (46 x+179)-175) x+46\right)-3 (x-1)^2\right.\right.\right.\right.\\ & \left.\left.\left. y \left(7 c_p+11\right)-11 x+12\right)-1\Big)-2 m_c^2 \left(y \left(2 (y-1) c_p (x y-1) \left(\left(x^2+x-1\right) y-x+1\right)+y \left(x \left(2 (x ((8 x-15)\right.\right.\right.\right.\right.\right.\\ & \left.\left.\left.\left.\left. x+9)-1) y^2-2 ((x-3) x+5) y-4 x+11\right)+2 y\right)-3 x-4 y+3\right)-1\right)\right)+2 x y \left((x-1) (y-1) c_p \left(x \Big(5 \Big(x^2\right.\right.\\ & \left.+x-1\Big) y^2-4 (x+1) y+3\Big)+5 y-3\right)+5 ((3 x ((x-5) x+8)-10) x+1) x y^3-(2 (x ((x+10) x+15)-12) x\\ & \left.\left.+5) y^2+((x (7 x+33)-30)x+8) y-3 (x-1)^2\right) F(s,x,y)^3+3 s^3 x (y-1)^3 y^3 \left(2 x y^4 \left(\left(x^3-2 x+1\right) c_p+(3 x\right.\right.\right.\\ & \left. ((x-5) x+8)-10) x+1\right)-2 y^3 \left((x (x+2)-4) x^2 c_p+c_p+2 (2 x (x+2)-3) x+1\right)+y^2 \left(2 \left(2 x^2+x-2\right)\right.\\ & \left.\left.\left. (x-1) c_p+(x (4 x+15)-15) x+4\right)-(x-1)^2 y \left(2 c_p+3\right)-x+1\right)\right)\Bigg\},\\ \rho^{\langle\bar{s}s\bar{s}s\rangle}_{1,7;A(S)}(s) =&- \int^{z_{\max}}_{z_{\min}}{\rm d}z \frac{c_1}{24 \pi } \left(m_c m_s \left(s (z-1) \left(z \left(-14 c_p+22 z-5\right)+2\right) G(s,z)+\left(-2 c_p+4 z-3\right) G(s,z)^2+2 s^2 (z-1)^2 z\right.\right.\\ & \left.\left. \left(z \left(-2 c_p+2 z-1\right)+1\right)\right)+m_s^2 \left(-2 s \left(z \left(z \left((35 z-24) (z-1) c_p+(35 z-59) z+38\right)-12\right)+1\right) G(s,z)\right.\right.\\ & \left.\left.-2 z (5 z-3) \left(c_p+1\right) G(s,z)^2+s^2 (-(z-1)) z \left((z-1) z \left((25 z-21) z \left(c_p+1\right)+12\right)+1\right)\right)+2 m_c^2 G(s,z)\right.\\& \left. \left(c_p G(s,z)+G(s,z)+4 s (z-1) z \left(c_p+1\right)+2 s\right)\right)+\int^{1}_{0}{\rm d}z \frac{c_1}{24 \pi }s^3 (z-1)^3 z^3 m_s^2 \left(2 (z-1) z \left(c_p+1\right)+1\right),\\ \rho^{\langle\bar{s}s\rangle\langle\bar{s}\sigma\cdot Gs\rangle}_{1,7;A(S)}(s) =& - \int^{z_{\max}}_{z_{\min}}{\rm d}z\Bigg\{\frac{ c_1}{288 \pi } \left(12 m_c^2 \left(\left(\left(8 z^2-4 z-2\right) c_p+8 z^2-4 z+2\right) G(s,z)+2 s (z (11 z-7)-2) (z-1) z c_p\right.\right.\\ & \left.\left.+2 s (z ((11 z-18) z+14)-5) z+s\right)+m_c m_s \left(s \left(-4 (z-1) (10 z (12 z-7)-21) z c_p+((5 ((10 z-59) z+9) z\right.\right.\right.\\ & \left.\left.\left.+51) z+45) z-12\right)-8 \left((z (15 z-7)-3) c_p+3 (5 z-2) z+3\right) G(s,z)\right)-4 z m_s^2 \left(2 \left(2 (2 (5 (3 z-4) z+4) z\right.\right.\right.\\ & \left.+3) c_p+4 (5 (3 z-4) z+9) z-9\right) G(s,z)+s (z-1) \left(((2 (z (130 z-77)-115) z+127) z+8) c_p+2 (z ((130 z\right.\\ & \left.\left.\left.-77) z+11)-10) z\right)\right)\right)+\frac{c_2 }{64 \pi }\left(4 m_c^2 \left(\left(c_p+1\right) G(s,z)+2 s (z-1) z c_p+2 s (z-1) z+s\right)+m_c m_s \left(\Big(-4 c_p\right.\right.\\ & \left.\left.+8 z-6\Big) G(s,z)+s (z-1) \left(-14 z c_p+(22 z-5) z+2\right)\right)-2 m_s^2 \left(2 (5 z-3) z \left(c_p+1\right) G(s,z)+s z \Big(z \Big((35 z\right.\right.\\ & \left.\left.-24) (z-1) c_p+(35 z-59) z+38\Big)-12\Big)+s\right)\right)\Bigg\}+\int^{1}_{0}{\rm d}z\Bigg\{\frac{c_1 s^2 (z-1) z}{144 \pi } \left(m_c m_s \left(z \left(3 (z (35 z-27)-4)\right.\right.\right.\\ & \left.\left.+\left(z-1) c_p(60 z^2-58 z+15\right) z-4\right)-2\right)+2 (z-1) z m_s^2 \Big((((2 z (73 z-77)-39) z+37) z+12) c_p\\ & \left.+(z (2 (73 z-77) z+79)-26) z-4\Big)-12 (z-1) z m_c^2 \left(2 (z-1) z \left(c_p+1\right)+1\right)\right)-\frac{{\rm i} c_2 s^2 (z-1)^2 z}{32 \pi } m_c m_s\\ & \left(z \left(-2 c_p+2 z-1\right)+1\right)\Bigg\},\\ \rho^{\langle g_sG^2\rangle\langle \bar{s}s\rangle}_{1,7;A(S)}(s) = & \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{x c_1}{576 \pi ^3 (x-1)^3 (y-1)^4} m_c \left(-3 (x-1) \left(2 ((x ((x ((7 x-37) x+81)-95) x+61)-21) x\right.\right.\\ & +3) x y^5-((x ((6 (x-4) x+13) x+9)-15) x+5) y^4+((x ((-2 (x-4) x-43) x+66)-49) x+14) y^3\\ & +(x ((17 x-42) x+41)-14) y^2-3 (x-2) (x-1)^2 y-(x-1)^2+(y ((y (-2 x+2 (4 x-3) (x-1) y+7)-3) x\\ & \left.-5 y+4)-1) (x-1)^2 (y-1)^2 c_p\right) F(s,x,y)^2+\left(-2 (y ((x ((x-3) x+3) y-3) y+3)-1) \left(2 ((x ((7 x-23) x\right.\right.\\ & +24)-10) x+1) x y^3+(x ((5 x-9) x+7)-1) y^2-(x-1) ((5 x-9) x+2) y-(x-1)^2+(x-1) \\ & \left.\left(2 \left(x^2+x-1\right) x y^2+x (2-5 x) y+y+x-1\right) (y-1) c_p\right) m_c^2-3 s (x-1) (y-1) \left(11 \left((4 x-3) c_p (x-1)^3\right.\right.\\ & \left.+(x ((x ((7 x-37) x+81)-95) x+61)-21) x+3\right) x y^6+\Big(-(11 x (8 x-5)-29) c_p (x-1)^3+2 ((2 x (2\\ & -5 (x-4) x)-53) x+50) x-29\Big) y^5+\Big(((11 x (4 x-5)-65) x+83) c_p (x-1)^2-4 x ((x ((x-4) x+48)\\ & -83) x+66)+79\Big) y^4+\left(-((11 x-76) x+86) c_p (x-1)^2+2 (9 (5 x-13) x+122) x-89\right) y^3-(x-1) \\ \end{aligned} $

$ \begin{aligned}[b] & \left.\left.\left((17 x-64) x+3 (6 x-13) (x-1) c_p+53\right) y^2-\left(7 c_p+16\right) y+\left(-10 x-7 (x-2) c_p+26\right) x y-2 x+2\right)\right)\\ & F(s,x,y)-s (y-1) \left((y ((x ((x-3) x+3) y-3) y+3)-1) \left(11 \left(7 x^4+\left(c_p-23\right) x^3+24 x^2-2 \left(c_p+5\right) x\right.\right.\right.\\ & \left.+c_p+1\right) x y^4-\left(-2 ((21 x-40) x+28) x+(x (x (11 x+37)-19)-7) (x-1) c_p+7\right) y^3+\left(-22 x^2+50 x\right.\\ & \left.\left.+(x (26 x-1)-14) c_p-10\right) (x-1) y^2-(x-1) \left(10 x+7 (x-1) c_p-4\right) y-x+1\right) m_c^2+3 (x-1) (y-1)\\& \left(2 \left((4 x-3) c_p (x-1)^3+(x ((x ((7 x-37) x+81)-95) x+61)-21) x+3\right) x y^6-2 \left((8 x+3) c_p (x-1)^4\right.\right.\\ & \left.+2 \left(x^4-4 x^3+5 x-5\right) x+3\right) y^5+\left(2 ((x (4 x-5)-7) x+9) c_p (x-1)^2-3 x (11 (x-2) x+19)+18\right) y^4\\ & +\left(-2 ((x-8) x+10) c_p (x-1)^2+((19 x-54) x+60) x-23\right) y^3-(x-1) \left((4 x-17) x+2 (2 x-5) (x-1)\right.\\ & \left.\left.\left.\left. c_p+16\right) y^2-2 \left(c_p+3\right) y+\left(-3 x-2 (x-2) c_p+9\right) x y-x+1\right) s y\right)\right)\Bigg\}\\ & -\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\frac{c_1 s^2 x y}{576 \pi ^3 (x-1)^3 (y-1)^2} m_c^3 (y (y (x ((x-3) x+3) y-3)+3)-1) \left(-2 y^3 \left((x (x+2)-4) x^2 c_p\right.\right.\\ & \left.\left.+c_p-2 (2 (x-2) x+3) x+1\right)+(x-1) y^2 \left(2 \left(2 x^2+x-2\right) c_p+x (11-4 x)-4\right)+2 x y^4 \left(x^3 \left(c_p-23\right)\right.\right.\\ & \left.\left.-2 x \left(c_p+5\right)+c_p+7 x^4+24 x^2+1\right)-(x-1)^2 y \left(2 c_p+3\right)-x+1\right),\\ \rho^{m_s\langle g_sG^2\rangle\langle \bar{s}s\rangle}_{1,7;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1}{576 \pi ^3 (x-1)^3 (y-1)^3} m_c^2 \left(-(y ((x ((x-3) x+3) y-3) y+3)-1) \left(5 c_p x^2+x^2-6 c_p\right.\right.\\ & x-2 x+\left(\left(c_p+9\right) x^4-4 \left(c_p+5\right) x^3+2 \left(5 c_p+9\right) x^2-8 \left(c_p+1\right) x+2 \left(c_p+1\right)\right) y^2+2 \left(((x-5) x+5) x\right.\\ & \left.\left.+(x ((x-7) x+7)-2) c_p-2\right) y+2 c_p+2\right) m_c^2+(x-1) (y-1) \left(35 \left((3 x ((x-4) x+6)-8) x+((x ((x-4)\right.\right.\\ & \left. x+10)-8) x+2) c_p+2\right) ((x-3) x+3) x^2 y^7+\left(((x (x (x (10-81 x)+374)-256)-837) x+486) x\right.\\ & \left.+(x (((x (59 (x-10) x+1724)-2176) x+543) x+486)-210) c_p-210\right) x y^6+\left(((x (((393-26 x) x\right.\\ & \left.-1115) x+2667)-1635) x+684) x+3 (x ((x (((38 x-121) x+41) x+643)-721) x+228)-8) c_p-24\right)\\ &y^5+\Big((x (1537-3 x ((35 x-99) x+571))-892) x+((x (((437-47 x) x-2273) x+2431)-880) x+72) c_p\\ & +72\Big) y^4+\left(((x (20 x+491)-737) x+634) x+(x (((917-95 x) x-1217) x+574)-84) c_p-96\right) y^3\\ & +\left(((180-43 x) x-256) x-12 (x-1) ((11 x-13) x+4) c_p+72\right) y^2-6 \left(2 c_p+5\right) y+3 \Big(-7 x+2 (6-5 x)\\& \left. c_p+18\Big) x y-5 x+6\right) s+(x-1) \left(10 \left((3 x ((x-4) x+6)-8) x+((x ((x-4) x+10)-8) x+2) c_p+2\right)\right.\\ & ((x-3) x+3) x^2 y^6+2 \left(\left(72-x \left(\left(12 x^3-63 x+52\right) x+114\right)\right) x+(x (((x (8 (x-10) x+233)-292) x+66)\right.\\ & \left. x+72)-30) c_p-30\right) x y^5+3 \left(((3 x (((22-3 x) x-54) x+106)-194) x+72) x+(x ((x ((x (9 x-26)-6) x\right.\\ & \left.+200)-218) x+72)-4) c_p-4\right) y^4+2 \left((x (277-3 x (6 (x-4) x+103))-142) x+2 ((x (((37-4 x) x\right.\\ & \left.-172) x+191)-77) x+9) c_p+18\right) y^3-2 \left((3 x ((x-26) x+38)-88) x+((x ((17 x-137) x+203)-112)\right.\\ & \left.x+21) c_p+21\right) y^2-6 \left(((x-7) x+9) x+(3 x (2 (x-3) x+5)-4) c_p-4\right) y-3 \left((x-2) x+((5 x-6) x+2)\right.\\ & \left.\left.\left. c_p+2\right)\right) F(s,x,y)\right)\Bigg\}+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{\frac{ c_1 s}{1152 \pi ^3 (x-1)^3 (y-1)^2} m_c^2 (y (y (x ((x-3) x+3) y-3)+3)-1)\\ & \left(2 m_c^2 \left(2 y^3 \left(x^4 \left(c_p+9\right)-4 x^3 \left(c_p+5\right)+2 x^2 \left(5 c_p+9\right)-8 x \left(c_p+1\right)+2 \left(c_p+1\right)\right)+4 y^2 \left((x ((x-7) x+7)\right.\right.\right.\\ & \left.\left.\left.-2) c_p+x (x-2)^2-2\right)+x y \left(2 (5 x-6) c_p+3 (x-2)\right)+y \left(4 c_p+6\right)+x-2\right)-s (x-1) x (y-1) y \left(25 y^4\right.\right.\\ & \left(((x ((x-4) x+10)-8) x+2) c_p+(3 x ((x-4) x+6)-8) x+2\right)+2 y^3 \Big(23 (x ((x-7) x+7)-2) c_p+(91\\ & \left.\left.-x (27 x+91)) x-46\Big)+y^2 \left(21 ((5 x-6) x+2) c_p+(29 x-62) x+66\right)+12 (x-2) y+2\right)\right)\Bigg\},\\ \rho^{\langle g_sG^2\rangle^2}_{1,7;A(S)}(s) = &-\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{c_1 x^3 y^4}{5806080 \pi ^5 (x-1)^2 (y-1)^2} m_c^4 \left(28 y \Big((x ((15 x-74) x+70)-20) c_p-39 x^2+45 x\right.\\ \end{aligned} $

$ \begin{aligned}[b]& \left.-20\Big)+10 y^2 \left(x^4 \left(2 c_p+65\right)-70 x^3 \left(c_p+3\right)+21 x^2 \left(9 c_p+13\right)-140 x \left(c_p+1\right)+35 \left(c_p+1\right)\right)+21 \Big(((23 x\right.\\& \left.-30) x+10) c_p-(2 x+5) x+10\Big)\right)-\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\frac{c_1 s x^3 y^3}{1658880 \pi ^5 (x-1)^2 (y-1)} m_c^4 \Big(y^2 \left(8 x^2 \left(69 c_p+8\right)\right.\\ & \left.-30 x \left(24 c_p+11\right)+240 c_p+380\right)+y^3 \left(50 x^3 \left(9 c_p-1\right)-x^2 \left(2183 c_p+923\right)+5 x \left(413 c_p+233\right)\right.\\ & \left.-590 \left(c_p+1\right)\right)+10 y^4 \left(x^4 \left(2 c_p+65\right)-70 x^3 \left(c_p+3\right)+21 x^2 \left(9 c_p+13\right)-140 x \left(c_p+1\right)+35 \left(c_p+1\right)\right)\\ & +60 (x-2) y+10\Big),\\ \rho^{\langle g_sG^2\rangle\langle \bar{s}\sigma\cdot Gs\rangle}_{1,7;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1}{2304 \pi ^3 (x-1)^3 (y-1)^3} m_c \left(6 (x-1) \left(y^4 \left(\left(2 \left(8 x^3-15 x+6\right) x+5\right) (x-1)^2 c_p\right.\right.\right.\\ & \left.+(x (2 (3 x ((x-2) x-4)-7) x+33)-24) x+5\right)-2 x y^5 \left(\left(8 x^2-3\right) (x-1)^3 c_p+(x ((x ((19 x-97) x+201)\right.\\ & \left.-219) x+121)-33) x+3\right)+2 y^3 \left(-(x-1) (x (((8 x-19) x+12) x+5)-7) c_p+\left(\left(2 x \left(x^2+x+19\right)-53\right)\right.\right.\\& \left.\left.\left.\left. x+33\right) x-7\right)+2 y^2 \left((x ((x-3) x+6)-7) (x-1) c_p-((x (4 x+13)-33) x+28) x+7\right)+6 (x-1) y \Big(c_p\right.\right.\\ & \left.+(x-3) x+1\Big)+(1-x) \left(x \left(c_p-3\right)+c_p+1\right)\right) F(s,x,y)-2 m_c^2 (y (y (x ((x-3) x+3) y-3)+3)-1) \Big((x\\ & -1)(y-1) c_p (x (y (2 x ((9 x-3) y-8)-2 y+9)-1)+y-1)+y \left(x \left(2 ((x ((19 x-63) x+60)-22) x+1) y^2\right.\right.\\ & \left.\left.+((2 x (x+7)-21) x+16) y-10 (x-3) x-22\right)-y\right)+x (4-3 x)+2 y-1\Big)+3 s (x-1) (y-1) \left(y^5 \Big(\Big(11\right.\\ & \left(8 x^3-15 x+6\right) x+29\Big) (x-1)^2 c_p+2 (x ((x (10 (x-2) x-53)-100) x+156)-90) x+29\Big)-11 x y^6\\& \left(\left(8 x^2-3\right) (x-1)^3 c_p+(x ((x ((19 x-97) x+201)-219) x+121)-33) x+3\right)+y^4 \left(-(x-1) (x (11 ((8 x\right.\\ & \left.-19) x+12) x+58)-83) c_p+2 ((x (4 (x+8) x+213)-340) x+213) x-79\right)+y^3 \left((11 x ((x-3) x+6)\right.\\ & \left.-86) (x-1) c_p-2 (9 (2 x (x+6)-29) x+208) x+89\right)+y^2 \Big(3 (x-1) (x+13) c_p+((51 x-209) x+217) x\\ & \left.\left.-53\Big)+x y \left(x \left(30-7 c_p\right)-52\right)+y \left(7 c_p+16\right)+4 x-2\right)\right)-\frac{c_2 x y^2}{768 \pi ^3 (x-1)^2 (y-1)^2} m_c^3 \Big(y \Big(((x (x+21)\\ & -21) x+5) c_p+\left(x^2+x-1\right) x+5\Big)-2 \left((1-2 x)^2 c_p-x^2+x+1\right)+2 x y^3 \left((x-1) ((x-4) x+6) x c_p+c_p\right.\\ & \left.+(3 (x-2) x+2) x+1\right)+y^2 \left(3 (x (((x-4) x-2) x+4)-1) c_p+(((20-11 x) x-12) x+2) x-3\right)\Big)\Bigg\}\\ & +\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{\frac{c_1 s }{2304 \pi ^3 (x-1)^3 (y-1)^2}m_c \left(m_c^2 (-(y (y (x ((x-3) x+3) y-3)+3)-1)) \left(y \left(x^2 \left(7 c_p-38\right)\right.\right.\right.\\ & \left.-7 c_p+44 x-4\right)+11 x y^4 \left(9 x^3 \left(c_p-7\right)-12 x^2 \left(c_p-5\right)+2 x \left(c_p-11\right)+c_p+19 x^4+1\right)+y^3 \left(-(x-1)\right. \\& \left.(x (11 x (9 x+5)-59)-7) c_p+2 ((12 x (x+4)-97) x+68) x-7\right)+(x-1) y^2 \left((11 x (8 x-5)-14) c_p\right.\\ & \left.\left.-2 (6 (5 x-12) x+5)\right)-3 x+1\right)-3 s (x-1) (y-1) y \left(2 y^5 \left(-\left(\left(8 x^3-15 x+6\right) x+3\right) (x-1)^2 c_p+2 ((x (x\right.\right.\\ & \left.\left. (5-(x-2) x)+9)-15) x+9) x-3\right)+2 x y^6 \left(\left(8 x^2-3\right) (x-1)^3 c_p+(x ((x ((19 x-97) x+201)-219) x\right.\right.\\ & \left.+121)-33) x+3\right)+y^4 \left(2 (x (((8 x-19) x+12) x+6)-9) (x-1) c_p-3 ((x (6 x+25)-46) x+31) x+18\right)\\ & +y^3 \left(-2 (x-1) (x ((x-3) x+6)-10) c_p+((x (7 x+46)-123) x+104) x-23\right)+y^2 \left(-2 (x-1) (x+5) c_p\right.\\ & \left.\left.\left.-(x-2) (12 x-31) x+16\right)+x y \left(x \left(2 c_p-9\right)+18\right)-2 y \left(c_p+3\right)-2 x+1\right)\right)-\frac{c_2 s x y }{1536 \pi ^3 (x-1)^2 (y-1)}\\ & m_c^3 \left(y \left(-y \left(11 (1-2 x)^2 c_p-6 x^2+8 x+24\right)+11 x y^4 \left((x-1) ((x-4) x+6) x c_p+c_p+(3 (x-2) x+2) x+1\right)\right.\right.\\ & +y^3 \left(3 (2 x ((3 (x-4) x-4) x+10)-5) c_p-(((59 x-104) x+44) x+8) x-15\right)+y^2 \left(((x (7 x+111)-111)\right.\\ & \left.\left.\left. x+26) c_p+2 ((5 x-7) x+7) x+30\right)+9\right)-1\right)\Bigg\},\\ \rho^{m_s\langle g_sG^2\rangle\langle \bar{s}\sigma\cdot Gs\rangle}_{1,7;A(S)}(s) =& -\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 }{3456 \pi ^3 (x-1)^2 (y-1)^2}m_c^2 \left(10 ((x-3) x+3) x y^6 \left(x^4 \left(5 c_p-1\right)-4 x^3 \left(c_p+3\right)+6 x^2\right.\right.\\ \end{aligned} $

$ \begin{aligned}[b]& \left. \left(c_p+5\right)-8 x \left(c_p+1\right)+2 \left(c_p+1\right)\right)-2 y^5 \left(12 x^6 \left(c_p+6\right)+4 x^5 \left(c_p-39\right)-5 x^4 \left(13 c_p+3\right)+4 x^3 \Big(61 c_p\right.\\ & \left.+31\Big)+42 x^2 \left(7-3 c_p\right)-72 x \left(c_p+1\right)+30 \left(c_p+1\right)\right)+6 y^4 \left(((x (((7 x-8) x+48) x+28)-84) x+26) c_p\right.\\ & \left.+(x (255-2 x (5 (x-3) x+23))-88) x+26\right)-y^3 \left(((x (5 (x+28) x+228)-452) x+152) c_p+(x (x (168\right.\\ & \left.-19 x)+930)-458) x+152\right)+y^2 \left(((x (15 x+98)-184) x+68) c_p+3 (x (21 x+88)-66) x+68\right)\\ & \left.+3 y \left((x (x+12)-4) c_p-(x-14) x-4\right)-9 x \left(c_p+1\right)\right)+\frac{c_2 y}{1536 \pi ^3 (x-1)^2 (y-1)^2} m_c^2 \left(3 y \left((x ((7 x-17) x\right.\right.\\ & \left.+11)-2) c_p+\left(-3 x^2+x+3\right) x-2\right)+10 x y^5 \left(((x ((x ((x-6) x+15)-20) x+19)-10) x+2) c_p\right.\\ & \left.+(x ((9 x-19) x+8)-2) (x-1)\right)+4 x y^4 \left((4 x ((x ((x-5) x+10)-19) x+14)-13) c_p+(46-x ((x (5 x\right.\\ & \left.+17)-90) x+106)) x-13\right)+x y^3 \Big(3 ((x ((x-3) x+66)-66) x+17) c_p+(x ((33 x-145) x+216)-112)\\ & x+51\Big)+y^2 \left((3-2 x ((x (3 x+44)-56) x+20)) c_p+2 (x ((7 x-18) x+12)-14) x+3\right)\\ & \left.+3 \left((1-2 x)^2 c_p+1\right)\right)\Bigg\}+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{-\frac{c_1 }{3456 \pi ^3 (x-1)^3 (y-1)^2}m_c^2 \left(s (x-1) (y-1) \left(y \Big(35 ((x-3) x\right.\right.\\ & +3) x y^6 \left(x^4 \left(5 c_p-1\right)-4 x^3 \left(c_p+3\right)+6 x^2 \left(c_p+5\right)-8 x \left(c_p+1\right)+2 \left(c_p+1\right)\right)-y^5 \left(4 x^6 \left(24 c_p+109\right)\right.\\ & +7 x^5 \left(c_p-119\right)-5 x^4 \left(97 c_p+91\right)+2 x^3 \left(911 c_p+491\right)+9 x^2 \left(233-107 c_p\right)-486 x \left(c_p+1\right)+210\\ & \left. \left(c_p+1\right)\right)+y^4 \left(3 ((x (((56 x-65) x+344) x+241)-621) x+188) c_p+(x (((21-40 x) x-194) x+4797)\right.\\ & \left.-1527) x+564\right)-y^3 \left(((x ((85 x+364) x+1203)-1807) x+568) c_p+(x (x (552-29 x)+3291)-1465)\right.\\ & \left.x+652\right)+y^2 \left(((x (78 x+583)-773) x+262) c_p+(x (267 x+1087)-767) x+418\right)-2 y \left(3 ((13 x-24)\right.\\ & \left.\left.x+8) c_p+(61 x-114) x+77\right)-3 x \left(6 c_p+13\right)+30\Big)-2\right)-3 x m_c^2 (y (y (x ((x-3) x+3) y-3)+3)-1)\\ & \left.\left(2 y^2 \left(2 x \left(c_p-3\right)-c_p+4 x^2+3\right)-y \left((5 x-2) c_p+x+2\right)+c_p+1\right)\right)+\frac{c_2 y}{1536 \pi ^3 (x-1)^3 (y-1)^2} m_c^2\\ & \left(m_c^2 \left(4 x^2 c_p+y^4 \left(((x ((x ((x-6) x+15)-20) x+19)-10) x+2) c_p+(x ((x ((x-6) x+23)-36) x+27)\right.\right.\right.\\ & \left.-10) x+2\right)+2 y^3 \left((x ((x ((x-5) x+10)-18) x+13)-3) c_p+((x ((x-13) x+26)-24) x+11) x-3\right)\\ & +y^2 \left(((x ((x-4) x+30)-28) x+7) c_p+(x ((9 x-20) x+26)-16) x+7\right)-4 y \Big((1-2 x)^2 c_p+(x-1) x\\ & \left.+1\Big)-4 x c_p+c_p+1\right)-s (x-1) (y-1) \left(35 x y^6 \left(((x ((x ((x-6) x+15)-20) x+19)-10) x+2) c_p\right.\right.\\ & \left.+(x ((9 x-19) x+8)-2) (x-1)\right)+x y^5 \left((x ((59 x ((x-5) x+10)-1106) x+811)-188) c_p+(631\right.\\ & \left.-x ((x (70 x+247)-1290) x+1486)) x-188\right)+x y^4 \left(3 (2 (x ((3 x-11) x+132)-128) x+65) c_p\right.\\ & \left.+(x ((137 x-568) x+786)-406) x+223\right)+y^3 \left((6-x (4 (x (3 x+95)-107) x+131)) c_p+(x ((37 x\right.\\& \left.-120) x+84)-147) x+6\right)+y^2 \left(6 (3 x-2) (5 (x-1) x+1) c_p+(x (23-18 x)+49) x-12\right)\\ & \left.\left.\left. +x y \left(24 (x-1)\right.c_p-3 x-8\right)+y \left(6 c_p+9\right)+x-3\right)\right)\Bigg\},\\ \end{aligned} $

(23) where
$ F(s,x,y)=\dfrac{m_c^2 (1-x y)}{1-x}-s (1-y) y $ ,$ G(s,z)=m_c^2-s(1-z)z $ ,$y_{\max}=\dfrac{1}{2}+\dfrac{\sqrt{4 m_c^2 s (x-1)+\left(s (x-1)-m_c^2 x\right)^2}+m_c^2 x}{2 s (1-x)}$ ,$y_{\min}=\dfrac{1}{2}-\dfrac{\sqrt{4 m_c^2 s (x-1)+\left(s (x-1)-m_c^2 x\right)^2}-m_c^2 x}{2 s (1-x)}$ ,$x_{\max}=\left(1-2 \sqrt{m_c^2/s}\right)/\left(\sqrt{m_c^2/s}-1\right)^2$ ,$z_{\max}=\dfrac{1}{2}\left(1+\sqrt{1-4 m_c^2/s}\right)$ ,$z_{\min}=\dfrac{1}{2}\left(1-\sqrt{1-4 m_c^2/s}\right)$ , coefficient$ c_p=1 $ for current$ J_{1,\mu\nu}^{A(S)} $ while$ c_p=-1 $ for current$ J_{7,\mu\nu}^{A(S)} $ , and$ c_1=12,c_2=-8,c_3=4 $ for color antisymmetric current$ J_{i,\mu\nu}^{A} $ while$ c_1=24,c_2=8,c_3=20 $ for color symmetric current$ J_{i,\mu\nu}^{S} $ . The spectral functions for$ (1,1\{1,0\}) $ structure are shown as$\begin{aligned}[b]\rho^{\rm pert}_{2,8;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{x^2 }{51609600 \pi ^5 (y-1)^5}F(s,x,y)^3 c_1 \left((x-1) x \left((350 (4 y-3) y+(231-8 y (-266 x+5 ((x\right.\right.\\ & -14) x+42) y+147)) x y+35) x+(x (140 (4 y-1) y+(8 (5 x (20 x y+21)-28 (5 y+4)) y+483) x y-105)\\& \left.-70 (y-1)) c_p\right) F(s,x,y)^3+42 x \left((x-1) (y-1) s \left(\left(\left(\left(-20 ((x-14) x+42) y^2+6 (141 x-32) y-29\right) x\right.\right.\right.\right.\\ & \left.\left.+500 y-410\right) y+105\right) x y+\left(-20 (y-1) y+2 \Big(55 (2 y-1) y+\left(\left(115 x+40 \left(5 x^2-7\right) y-96\right) y+143\right) x y\right.\\ & \left.\left.-15\Big) x y-5\right) c_p\right)+\left(50 (y-1)+\left(-10 (3 y+10) y+((8 (7 x-8) y x-183 x+166) y+140) x y+2 (y (x (y (11 x\right.\right.\\ & \left.\left.\left.+8 ((x-9) x+5) y+68)-20)-40 y)+5) c_p+15\right) x\right) m_c m_s\right) F(s,x,y)^2+30 (y-1) \Big((x-1) (y-1) \\ & \left(50 \left(-(x-14) x+4 \left(5 x^2-7\right) c_p-42\right) x y^3+14 \left((131 x+3) x+4 ((5 x+2) x+5) c_p+75\right) y^2+7 \left((82 x-30)\right.\right.\\ & \left.\left. c_p-35 (x+4)\right) y+385\right) s^2 x^2 y^2+14 \left(30 (y-1)+\left(\left(-2 \left(7 c_p+17\right) y x^2+5 \left(8 c_p y+12 y-2 c_p+3\right) x-20 y\right.\right.\right.\\ & \left.\left.\left. \left(c_p+2\right)-10\right) y+5\right) x\right) m_c^2 m_s^2+7 \left(10 (y+1) y+(-30 (y+4) y+((22 (7 x-8) y x-419 x+330) y+346) x y\right.\\ & \left.-125) x y+2 (y ((((2 y (34 x+11 ((x-9) x+5) y+75)-107) x-110 y+30) y+30) x+10 y)-5) c_p+20\right) s x\\ & \left.m_c m_s\Big) F(s,x,y)+120 (y-1)^2 s \left((x-1) (y-1) \left(\left(4 \left(-(x-14) x+4 \left(5 x^2-7\right) c_p-42\right) x y^2+14 \left(\Big(9 x+4 c_p\right.\right.\right.\right.\right.\\ & \left.\left.\left.+3\Big) x+5\right) y+7 \left(4 c_p-5\right) x-70\right) y+35\right) s^2 x^2 y^3+7 \left(10 (y-1) y+\left(y (x ((4 (7 x-8) y x-77 x+82) y+44)\right.\right.\\ & \left.\left.-30 y)+2 (2 y (9 x+2 ((x-9) x+5) y-5)-5) (x y-1) c_p-25\right) x y+10\right) s x m_c m_s y+14 \left(\left(-4 \left((17 x-30) x\right.\right.\right.\\ & \left.\left.\left.\left.\left.+((7 x-20) x+10) c_p+20\right) x y^2+5 \left(\left(x+(4-8 x) c_p+6\right) x+6\right) y+10 \left(c_p-2\right) x-30\right) y+15\right) m_c^2 m_s^2\right)\right),\\ \rho^{\langle\bar{s}s\rangle}_{2,8;A(S)}(s) =& -\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{c_1 (x-1) x^2}{3072 \pi ^3 (y-1)^3} m_c F(s,x,y)^2 \Big(4 s (y-1) y (11 x y-5) (2 x y-1) F(s,x,y)+(x y-1)\\& (8 x y+1) F(s,x,y)^2+12 s^2 (y-1)^2 y^2 (x y (4 x y-3)+1)\Big),\\ \rho^{\langle m_s\bar{s}s\rangle}_{2,8;A(S)}(s) =& -\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{c_1 x}{6144 \pi ^3 (y-1)^3} F(s,x,y) \left(12 s (y-1) F(s,x,y) \left(2 m_c^2 \Big(x y \left(-4 ((x-2) x+2) y^2+6 y-3\right)\right.\right.\\ & \left.+2 (y-1) y+1\Big)+s (x-1) x (y-1) y^2 \left(y \left(50 ((x-2) x+2) x y^2-2 (x+15) (2 x+1) y+29 x+28\right)-11\right)\right)\\ & +4 F(s,x,y)^2 \left(4 m_c^2 (y (-((x-2) x+2) x y+x+1)-1)+s (x-1) x (y-1) y (y (x (2 y (70 ((x-2) x+2) y-11 x\right.\\ & \left.-68)+57)-100 y+82)-21)\right)+(x-1) c_p \left((x (y (-8 ((x-4) x+2) y-15 x+4)+3)+2 (y-1)) F(s,x,y)^2\right.\\& -4 s (y-1) (2 y (x (y (11 ((x-4) x+2) y+25 x-11)-3)-2 y+2)-1) F(s,x,y)-24 s^2 x (y-1)^2 y^3 (2 ((x-4) x\\ & \left.+2) y+5 x-3)\right) F(s,x,y)+(x-1) x \left(40 ((x-2) x+2) x y^3-8 ((x+3) x+5) y^2+3 (x+10) y-1\right) F(s,x,y)^3\\ & \left.+24 s^3 (x-1) x (y-1)^3 y^3 \left(y \left(x \left(4 ((x-2) x+2) y^2-6 y+3\right)-2 y+2\right)-1\right)\right),\\ \rho^{\langle g_sG^2\rangle}_{2,8;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{x^2 c_1}{30965760 \pi ^5 (x-1)^3 (y-1)^5} m_c \left((x-1) x \left((-350 (4 y-3) y+(8 y (-266 x+5 ((x-14) x\right.\right.\\ & +42) y+147)-231) x y-35) (x y-1) ((x+((x-3) x+3) y-3) y+1) (-x) m_c+21 \left(8 ((x ((7 x-41) x+101)\right.\\& -108) x+40) x^2 y^5+(x (((23-31 x) x-469) x+1056)-610) x y^4+(((x (25 x+541)-1020) x+320) x\\ & \left.+310) y^3+(13 x (x (5-14 x)+35)-600) y^2+5 ((23 x-65) x+74) y+45 x-80\right) m_s+c_p \left((x y-1) ((x+((x\right.\\ & -3) x+3) y-3) y+1) (x (140 (4 y-1) y+(8 (5 x (20 x y+21)-28 (5 y+4)) y+483) x y-105)-70 (y-1)) m_c\\ & +42 \left(8 ((x ((x-13) x+38)-44) x+15) x^2 y^5+(x ((x (27 x-71)-7) x+278)-140) x y^4+((((103-15 x) x\right.\\ & \left.\left.\left.-330) x+100) x+20) y^3+(x (x (95-16 x)+35)-30) y^2-5 (x (x+7)-2) y+5 x\right) m_s\right)\right) F(s,x,y)^3\\ & +21 \left((y ((x ((x-3) x+3) y-3) y+3)-1) \left(50 (y-1)+\left(-10 (3 y+10) y+((8 (7 x-8) y x-183 x+166) y\right.\right.\right.\\ & \left.\left.+140) x y+2 (y (x (y (11 x+8 ((x-9) x+5) y+68)-20)-40 y)+5) c_p+15\right) x\right) x m_s m_c^2+(x-1) (y-1)\\ & \left(s x (y ((x ((x-3) x+3) y-3) y+3)-1) \left(\Big(\left(\left(-20 ((x-14) x+42) y^2+6 (141 x-32) y-29\right) x+500 y-410\right) y\right.\right.\\ & \left.+105\Big) x y+\left(-20 (y-1) y+2 \left(55 (2 y-1) y+\left(\left(115 x+40 \left(5 x^2-7\right) y-96\right) y+143\right) x y-15\right) x y-5\right) c_p\right)\\ \end{aligned} $

$ \begin{aligned}[b] & -6 ((((x-2) x+2) y-2) y+1) \left(x \Big(y \left(2 \left(7 c_p+17\right) y x^2-5 \left(8 c_p y+12 y-2 c_p+3\right) x+20 \left(c_p+2\right) y+10\right)\right.\\ & \left.\left.-5\Big)-30 (y-1)\right) m_s^2\right) m_c+3 (x-1) (y-1) \left(22 \left((x ((7 x-41) x+101)-108) x+2 ((x ((x-13) x+38)-44)\right.\right.\\ & \left.x+15) c_p+40\right) x^2 y^6+\left((x ((x+367) x+377)-2040) (-x)+4 (x (2 (28 x-61) (x-1) x+305)-185) c_p\right.\\ & \left.-1370\right) x y^5+\left(3 ((11 x (5 x+33)-864) x+440) x+(2 (((287-45 x) x-1041) x+400) x+100) c_p+470\right) y^4\\ & -\left(((558 x-757) x+5) x+2 (((49 x-421) x+20) x+80) c_p+900\right) y^3+\left((181 x-315) x-2 (62 x+105) c_p x\right.\\ & \left.\left.\left.+70 c_p+620\right) y^2+5 \left(3 x+2 (7 x+1) c_p\right) y-170 y-10 c_p+20\right) s x m_s\right) F(s,x,y)^2-6 (y-1) \left(14 (y ((x ((x\right.\\ & -3) x+3) y-3) y+3)-1) \left(x \left(y \left(2 \left(7 c_p+17\right) y x^2-5 \left(8 c_p y+12 y-2 c_p+3\right) x+20 \left(c_p+2\right) y+10\right)-5\right)\right.\\ & \left.-30 (y-1)\right) m_s^2 m_c^3-7 s x (y ((x ((x-3) x+3) y-3) y+3)-1) \left(10 (y+1) y+(-30 (y+4) y+((22 (7 x-8) y x\right.\\ & -419 x+330) y+346) x y-125) x y+2 (y ((((2 y (34 x+11 ((x-9) x+5) y+75)-107) x-110 y+30) y\\ & \left.+30) x+10 y)-5) c_p+20\right) m_s m_c^2-s (x-1) (y-1) \left(s x^2 y^2 (y ((x ((x-3) x+3) y-3) y+3)-1) \left(50 \left(-(x\right.\right.\right.\\ & \left.-14) x+4 \left(5 x^2-7\right) c_p-42\right) x y^3+14 \left((131 x+3) x+4 ((5 x+2) x+5) c_p+75\right) y^2+7 \left((82 x-30) c_p\right.\\ & \left.\left.-35 (x+4)\right) y+385\right)-42 ((((x-2) x+2) y-2) y+1) \left(y \left(4 \left((17 x-30) x+((7 x-20) x+10) c_p+20\right) x y^2\right.\right.\\ & \left.\left.\left.+5 \left(x \left(-x+(8 x-4) c_p-6\right)-6\right) y-10 x \left(c_p-2\right)+30\right)-15\right) m_s^2\right) m_c-21 s^2 (x-1) x (y-1) y \left(\left(4 \left((x ((7 x\right.\right.\right.\\ & \left.-41) x+101)-108) x+2 ((x ((x-13) x+38)-44) x+15) c_p+40\right) x^2 y^5+\left((x ((x+67) x+51)-352)\right.\\ & \left. (-x)+4 (x (((11 x-38) x+36) x+43)-30) c_p-250\right) x y^4+\left(\left((x (35 x+163)-468) x+2 (((39-5 x) x\right.\right.\\ & \left.\left. -189) x+90) c_p+300\right) x+70\right) y^3-\left(\left(16 \left(c_p+6\right) x^2-\left(188 c_p+193\right) x+70 c_p+125\right) x+140\right) y^2\\ & \left. \left.\left.+\left(\left(9 x-2 (18 x+5) c_p+5\right) x+120\right) y+5 \left(2 c_p-1\right) x-50\right) y+10\right) m_s\right) F(s,x,y)+6 (y ((x ((x-3) x+3) y\\ & -3) y+3)-1) (y-1)^2 s m_c \left((x-1) (y-1) \left(\left(4 \left(-(x-14) x+4 \left(5 x^2-7\right) c_p-42\right) x y^2+14 \left(\left(9 x+4 c_p+3\right)\right.\right.\right.\right.\\ & \left.\left. \left.x+5\right) y+7 \left(4 c_p-5\right) x-70\right) y+35\right) s^2 x^2 y^3+7 \left(10 (y-1) y+\left(y (x ((4 (7 x-8) y x-77 x+82) y+44)\right.\right.\\ & \left.\left.-30 y)+2 (2 y (9 x+2 ((x-9) x+5) y-5)-5) (x y-1) c_p-25\right) x y+10\right) s x m_c m_s y+14 \left(\left(-4 \left((17 x-30) x\right.\right.\right.\\ & \left.\left.\left.\left.\left.+((7 x-20) x+10) c_p+20\right) x y^2+5 \left(\left(x+(4-8 x) c_p+6\right) x+6\right) y+10 \left(c_p-2\right) x-30\right) y+15\right) m_c^2 m_s^2\right)\right)\\ & +\frac{c_3}{23592960 \pi ^5 (y-1)^3} (x-1) x^2 \left(c_p+1\right) F(s,x,y)^2 \Big(8 s (y-1) y ((393 x-200) y+73) F(s,x,y)+((477 x\\ & -230) y+43) F(s,x,y)^2+24 s^2 (y-1)^2 y^2 ((64 x-30) y+15)\Big)\Bigg\},\\ \rho^{\langle\bar{s}\sigma\cdot Gs\rangle}_{2,8;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Big\{-\frac{c_1 x }{3072 \pi ^3 (y-1)^3}m_c F(s,x,y) \left(3 s (y-1) \left(y c_p \left(x \left(2 y \left(22 (x-1) x y^2-4 (x-1) (3 x+5)y\right.\right.\right.\right.\right.\\ & \left.\left.\left.\left.+13 x-9\right)-7\right)-2 y\right)+c_p+y (x (y (x (y (66 (x-1) y-41 x-23)+42)+69 y-28)-15)-21 y+19)-2\right)\\ & F(s,x,y)+\Big(x \left(y \left(2 (x-1) c_p (y (8 x y-2 x-7)+1)+x (y (24 (x-1) y-9 x-13)+7)+25 y-12\right)+2\right)-y\\ & +1\Big) F(s,x,y)^2+6 s^2 (y-1)^2 y \left(y \left(x \left(2 c_p (x y-1) (2 (x-1) (2 y-1) y+1)+y (x (y (12 (x-1) y-7 x-3)+6)\right.\right.\right.\\ & \left.\left.\left.\left.+11 y-2)-4\right)-5 y+5\right)-1\right)\right)-\frac{c_2 x^2}{2048 \pi ^3 (y-1)^3} m_c F(s,x,y) \left(3 s (y-1) y \left(x y \left(44 (x-1) y^2+(26-39 x) y\right.\right.\right.\\ & \left.\left.+11\right)+18 (y-1) y+2\right) F(s,x,y)+(x y (y (16 (x-1) y-13 x+6)+4)+10 (y-1) y+3) F(s,x,y)^2+6 s^2\\& \left.(y-1)^2 y^3 \left(8 (x-1) x y^2+(x (6-7 x)+2) y+x-2\right)\right)\Bigg\},\\ \rho^{m_s\langle\bar{s}\sigma\cdot Gs\rangle}_{2,8;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1}{9216 \pi ^3 (y-1)^2} \left(c_p \left(12 s x (y-1) y F(s,x,y) \left(6 m_c^2 (2 y (((x-4) x+2) y+2 x-1)-1)\right.\right.\right.\\ & \left.+s (x-1) (y-1) y^2 \left(x \left(50 \left(x^2-2\right) y^2+8 (x+7) y+11\right)-4 y+3\right)\right)+3 F(s,x,y)^2 \left(12 x y m_c^2 (((x-4) x+2) y\right.\\ & \left.+x)+s (x-1) (y-1) \left(2 x y \left(y \left(7 x \left(y \left(20 \left(x^2-2\right) y+7 x+24\right)-1\right)-22 y+11\right)+3\right)+4 (y-1) y+1\right)\right)\\ \end{aligned} $

$ \begin{aligned}[b] & +(x-1) \left(x \left(y \left(x \left(8 y \left(10 \left(x^2-2\right) y+9 x+8\right)-21\right)-16 y+4\right)+3\right)+2 (y-1)\right) F(s,x,y)^3+24 s^3 (x-1) x^2\\ & \left. (y-1)^3 y^4 \left(2 y \left(\left(x^2-2\right) y+1\right)+1\right)\right)+x \left(-6 s (y-1) y F(s,x,y) \left(6 m_c^2 (y (x+8 y-12)+5)-s (x-1) (y-1)\right.\right.\\& \left. y (y (x (2 y (25 (5 (x-2) x+6) y+119 x-183)+139)-30 y+28)-11)\right)+3 F(s,x,y)^2 \left(s (x-1) (y-1) y (y \right.\\ & \left.(x (14 y (x (50 (x-2) y+51)+60 y-72)+313)-100 y+82)-21)-6 m_c^2 (y (3 x+4 y-8)+1)\right)+(x-1)\\ & (y (x (8 y (5 (5 (x-2) x+6) y+32 x-39)+51)-40 y+30)-1) F(s,x,y)^3+6 s^3 (x-1) (y-1)^3 y^3 \Big(y \Big(x \Big(4 (5\\ & \left.\left. (x-2) x+6) y^2+6 (3 x-5) y+13\Big)-2 y+2\Big)-1\Big)\right)\right)-\frac{c_2}{8192 \pi ^3 (y-1)^3} x \left(2 \left(6 s (y-1)^2 y F(s,x,y) \left(m_c^2 (2 x y\right.\right.\right.\\ & \left. (3-4 x y)-2)+s x y^2 (x (x y (5 y (10 (x-1) y-10 x+3)+36)+7 (5 y-4) y-8)-9 y+9)\right)+3 (y-1)\\ & F(s,x,y)^2 \left(s x y (y (x (5 x y (2 y (14 (x-1) y-14 x+5)+19)+90 (y-1) y-7)-10 y+14)-2)-2 m_c^2 (x y-1)\right.\\ & \left. (2 x y+1)\right)+x (y (x (y (x (20 y (2 x (y-1)-2 y+1)+23)+20 y-34)+8)+8 y-2)-3) F(s,x,y)^3+6 s^3\\ & \left. (x-1) x (y-1)^4 y^4 (x y (4 x y-3)+1)\right)+c_p \left(3 s (y-1) \left(y \left(x \left(2 y \Big(22 (x-1) x y^2-6 (x-1) (2 x+5) y+23 x\right.\right.\right.\right.\\ & \left.\left.\left.-24\Big)+3\right)-4 y+4\right)-1\right) F(s,x,y)+2 (x-1) (x y (y (8 x y-2 x-13)+7)+y-1) F(s,x,y)^2+12 s^2 x (y-1)^2\\ & \left.\left. y^2 \left(y \left(4 (x-1) x y^2-2 (x-1) (x+3) y+5 x-6\right)+1\right)\right) F(s,x,y)\right)\Bigg\},\\ \rho^{\langle\bar{s}s\bar{s}s\rangle}_{2,8;A(S)}(s) =& \int^{z_{max}}_{z_{min}}{\rm d}z\frac{c_1 c_p}{768 \pi } m_s^2 G(s,z) \left(G(s,z)+s (1-2 z)^2\right),\\ \rho^{\langle\bar{s}s\rangle\langle\bar{s}\sigma\cdot Gs\rangle}_{2,8;A(S)}(s) =& \int^{z_{\max}}_{z_{\min}}{\rm d}z\Bigg\{-\frac{c_1}{2304 \pi } \left(m_c m_s \left(s \left(z^2 \left(-\left(6 c_p+23\right)\right)+3 \left(c_p-2\right)+17 z\right)-11 G(s,z)\right)+m_s^2 \left(\left(-8 z^2 \left(3 c_p+5\right)\right.\right.\right.\\ & \left.\left.+2 z \left(7 c_p+15\right)+c_p-1\right) G(s,z)-s (z-1) z \left(6 (11 z-8) z c_p+c_p+2 (50 z-41) z+21\right)\right)+6 m_c^2 (2 G(s,z)\\ & \left.+2 s (z-1) z+s)\right)+\frac{c_2 c_p}{2048 \pi } m_s^2 \left(2 G(s,z)+s (1-2 z)^2\right)\Bigg\}+\int^{1}_{0}{\rm d}z\Bigg\{\frac{c_1 s^2 (z-1) z}{2304 \pi } m_s \left((z-1) z m_s \left((2 (6 z-5) z\right.\right.\\ & \left.\left.+1) c_p+30 z^2-28 z+11\right)+(7 (z-1) z+3) m_c\right)\Bigg\},\\ \rho^{\langle g_sG^2\rangle\langle \bar{s}s\rangle}_{2,8;A(S)}(s) =& \int^{x_{max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1 x^2}{18432 \pi ^3 (x-1)^2 (y-1)^3} m_c \left(2 F(s,x,y) \left(m_c^2 (x y-1)^2 (y (((x-3) x+3) y+x-3)+1)\right.\right.\\ & \left. (8 x y+1)+3 s (x-1) (y-1) y (x y (y (x (y (22 (x-1) y-17 x-19)+29)+41 y-28)-4)-13 (y-1) y-2)\right)\\ & +3 (x-1) (x y-1) (x (8 y-5) y ((x-1) y-1)+11 (y-1) y+3) F(s,x,y)^2+2 s (y-1) y \left(m_c^2 (11 x y-5) (x y-1)\right.\\ & (2 x y-1) (y (((x-3) x+3) y+x-3)+1)+3 s (x-1) (y-1) y^2 \left(y \left(x \Big(x \left(4 (x-1) y^2-3 (x+1) y+5\right)+7 y\right.\right.\\ & \left.\left.\left.\left.-6\Big)-1\right)+1\right)\right)\right)\Bigg\}-\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\frac{c_1 s^2 x^2 y^2}{9216 \pi ^3 (x-1)^2 (y-1)} m_c^3 (x y-1) (x y (4 x y-3)+1) (y (((x-3) x+3) y\\ & +x-3)+1),\\ \rho^{m_s\langle g_sG^2\rangle\langle \bar{s}s\rangle}_{2,8;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 x}{18432 \pi ^3 (x-1)^3 (y-1)^3} m_c^2 \left((x-1) \left(c_p (x y-1) (y (((x-3) x+3) y+x-3)+1) (x (y (8\right.\right.\\& ((x-4) x+2) y+15 x-4)-3)-2 y+2)-40 x^3 ((x-3) x+3) ((x-2) x+2) y^6+8 \Big(\left(x^3+14 x-36\right) x\\ & +45\Big) x^2 y^5+3 (x (x (3 (x-21) x+131)-166)-24) x y^4+(x (((41 x-95) x+224) x+162)-24) y^3+((x (4 x\\ & \left.-45)-109) x+48) y^2+3 (x (x+7)-12) y-x+12\right) F(s,x,y)+4 m_c^2 (x y-1) (y (((x-3) x+3) y+x-3)\\ & +1) \left(((x-2) x+2) x y^2-(x+1) y+1\right)+s (x-1) (y-1) \left(c_p (2 y (x (y (11 ((x-4) x+2) y+25 x-11)-3)\right.\\ & -2 y+2)-1) (x y-1) (y (((x-3) x+3) y+x-3)+1)+y \left(-140 x^3 ((x-3) x+3) ((x-2) x+2) y^6+2 ((x\right.\\ & ((11 x+35) x+89)-366) x+570) x^2 y^5-x (((x (33 x+427)-1041) x+1686) x+204) y^4+(x (((161 x\\ \end{aligned} $

$ \begin{aligned}[b] & \left.\left.\left.-361) x+1078) x+402)-24) y^3+((x (20 x-379)-277) x+48) y^2+17 (3 x+5) x y-3 x-48 y+24\right)-6\right)\right)\Bigg\}\\ &+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Big\{\frac{c_1 s x}{18432 \pi ^3 (x-1)^3 (y-1)^2} m_c^2 (y (y (x ((x-3) x+3) y-3)+3)-1) \left(2 m_c^2 \left(y \left(x \left(4 ((x-2) x\right.\right.\right.\right.\\ & \left.\left.\left.+2) y^2-6 y+3\right)-2 y+2\right)-1\right)-s (x-1) x (y-1) y^2 \left(y \left(-2 c_p (2 ((x-4) x+2) y+5 x-3)+50 ((x-2) x\right.\right.\\ & \left.\left.\left.+2) x y^2-2 (x+15) (2 x+1) y+29 x+28\right)-11\right)\right)\Big\},\\ \rho^{\langle g_sG^2\rangle^2}_{2,8;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 x^3 y^3}{371589120 \pi ^5 (x-1)^2 (y-1)^2} m_c^4 \Big(c_p (x (x y (8 y (5 x (20 x y+21)-28 (5 y+4))+483)\\ & +140 (4 y-1) y-105)-70 (y-1))+x (x y (8 y (7 (38 x-21)-5 ((x-14) x+42) y)+231)+350 (4 y-3) y\\ & +35)\Big)\Bigg\}+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{\frac{c_1 s x^3 y^3 }{53084160 \pi ^5 (x-1)^2 (y-1)}m_c^4 \left(c_p \left(2 x y \left(x y \left(y \left(40 \left(5 x^2-7\right) y+115 x-96\right)+143\right)\right.\right.\right.\\ & \left.\left.+55 (2 y-1) y-15\right)-20 (y-1) y-5\right)+x y \left(y \Big(x \left(-20 ((x-14) x+42) y^2+6 (141 x-32) y-29\right)+500 y\right.\\ & \left.\left.-410\Big)+105\right)\right)\Bigg\},\\ \rho^{\langle g_sG^2\rangle\langle \bar{s}\sigma\cdot Gs\rangle}_{2,8;A(S)}(s) =& \int^{x_{max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1 x}{36864 \pi ^3 (x-1)^3 (y-1)^3} m_c \left(3 (x-1) \left(8 (2 x-3) (x-1) x^2 y^5 \left(2 (x-1) c_p+x+1\right)+y^3\right.\right.\\ & \left(2 (x (2 x-27)-2) (x-1)^2 c_p+\left(\left(22 x^2-30 x-111\right) x+94\right) x+13\right)-x y^4 \left(10 (2 (x-1) x-5) (x-1)^2 c_p\right.\\ & \left.+((x (x+58)-149) x+50) x+37\right)+y^2 \left(2 (8 x+3) (x-1)^2 c_p+((92-13 x) x-33) x-28\right)-y \Big(2 (x-1)^2 c_p\\ & \left.+(15 x+16) x-19\Big)+7 x-4\right) F(s,x,y)+m_c^2 (y (y (x ((x-3) x+3) y-3)+3)-1) \left(x \left(y \left(2 (x-1) c_p (y (x (8 y\right.\right.\right.\\ & \left.\left.\left.-2)-7)+1)+x (y (24 (x-1) y-9 x-13)+7)+25 y-12\right)+2\right)-y+1\right)+3 s (x-1) (y-1) \Big(22 (2 x-3)\\ & (x-1) x^2 y^6 \left(2 (x-1) c_p+x+1\right)-x y^5 \left(4 (x (17 x-4)-17) (x-2) (x-1) c_p+((x (19 x+98)-329) x\right.\\ & \left.+118) x+89\right)+y^4 \left(2 (x (x ((7 x-89) x+177)-87)-5) c_p+((x (67 x-104)-231) x+246) x+5\right)+y^3\\ & \left((((45 x-127) x+47) x+16) c_p+((205-36 x) x-132) x-14\right)+y^2 \left((4 x (3 x+4)-7) c_p+5 x (2-5 x)-2\right)\\ & \left.-y \left(8 x c_p+c_p+x-9\right)+c_p-2\Big)\right)-\frac{c_2 x^2 y}{24576 \pi ^3 (x-1)^2 (y-1)^2} m_c^3 (x y-1) \Big(y (x (y (8 ((x-3) x+3) y+6 x\\& -15)+3)-9 y+9)-2\Big)\Bigg\}+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{-\frac{c_1 s x}{36864 \pi ^3 (x-1)^3 (y-1)^2} m_c \left(m_c^2 (y (y (x ((x-3) x+3) y-3)\right.\\ & +3)-1) \left(y c_p \left(x \left(2 y \left(22 (x-1) x y^2-4 (x-1) (3 x+5) y+13 x-9\right)-7\right)-2 y\right)+c_p+y (x (y (x (y (66 (x-1) y\right.\\ & \left.-41 x-23)+42)+69 y-28)-15)-21 y+19)-2\right)+3 s (x-1) (y-1) y \left(y \left(4 (2 x-3) (x-1) x^2 y^5 \Big(2 (x\right.\right.\\& -1) c_p+x+1\Big)-x y^4 \left(4 (x (3 x-1)-3) (x-2) (x-1) c_p+(3 x (x+8)-11) (x-2) x+15\right)+y^3 \left(2 (x ((x-15)\right.\\ & \left.x+33)-18) x c_p+(x (11 x-6)-48) (x-1) x-3\right)+y^2 \left(x \left(2 (2 (2 x-7) x+7) c_p+x (37-6 x)-33\right)+6\right)\\ & \left.\left.\left. y \left((4 x+2) c_p-5 x+9\right)-2 x \left(c_p+1\right)-8 y+5\right)-1\right)\right)-\frac{c_2 s x^2 y^2}{24576 \pi ^3 (x-1)^2 (y-1)} m_c^3 \Big(x y (y (x (y (22 ((x\\ & -3) x+3) y-4 x+17)-2)-83 y+60)-17)+23 (y-1) y+7\Big)\Bigg\},\\ \rho^{m_s\langle g_sG^2\rangle\langle \bar{s}\sigma\cdot Gs\rangle}_{2,8;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1}{110592 \pi ^3 (x-1)^2 (y-1)^2} m_c^2 \left(8 x^2 y^5 \left((((x (9 x-19)-29) x+30) x+54) c_p+(((32 x\right.\right.\\ & \left.-135) x+133) x+48) x-105\right)+40 ((x-3) x+3) x^3 y^6 \left(2 \left(x^2-2\right) c_p+5 (x-2) x+6\right)+x y^4 \left((x ((15 x+91)\right.\\ & \left.x+143)-1098) x c_p+198 c_p+3 ((x (17 x+159)-659) x+630) x-24\right)+y^3 \left((x (x (x (61-41 x)+790)\right.\\ & \left.-210)-6) c_p+(((1423-255 x) x-1728) x+222) x\right)+y^2 \left(((91-x (36 x+343)) x+12) c_p+((609-274 x)\right.\\ \end{aligned} $

$ \begin{aligned}[b]& \left.\left.x-263) x\right)+y \left((x (57 x+5)-8) c_p+21 x (7-5 x)\right)-3 x c_p+2 c_p-17 x\right)+\frac{c_2 x y}{49152 \pi ^3 (x-1)^2 (y-1)^2} m_c^2 \Big(y^2 \\ & \left(x^3 \left(-\left(c_p+3\right)\right)+x^2 \left(94-33 c_p\right)+3 x \left(c_p+7\right)+4 c_p\right)+4 x y^4 \left(2 ((x ((x-4) x+6)-6) x+2) c_p+(((2 x-11)\right. \\ & \left.x+21) x+16) x+10\right)+y^3 \left((x (x ((5 x-14) x+54)-18)-2) c_p+(3 x (x+1) (8 x-31)-70) x\right)+y \left((2 x (5 x\right.\\ & \left.+2)-3) c_p+7 x (1-3 x)+6\right)-2 x c_p+c_p-40 x^2 ((x-2) ((x-2) x+2) x+2) y^5-2 x-6\Big)\Bigg\}\\ & +\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{\frac{c_1}{110592 \pi ^3 (x-1)^3 (y-1)^2} m_c^2 \left(c_p \left(-12 x y m_c^2 (x y-1) (((x-4) x+2) y+x) (y (((x-3) x+3) y\right.\right.\\ & +x-3)+1)-s (x-1) (y-1) \left(y \left(2 (((7 x (7 x+3)-799) x+570) x+774) x^2 y^5+280 \left(x^2-2\right) ((x-3) x+3)\right.\right.\\ & x^3 y^6+2 ((x ((29 x+236) x+233)-1749) x+216) x y^4-2 (((x (65 x+116)-1324) x+321) x+6) y^3\\ & \left.\left.\left.+((311-x (61 x+885)) x+24) y^2+(2 x (79 x-2)-19) y-42 x+7\right)-1\right)\right)+x \left(6 m_c^2 (x y-1) (y (3 x+4 y-8)\right.\\ & +1) (y (((x-3) x+3) y+x-3)+1)-s (x-1) (y-1) y \left(y \left(140 ((x-3) x+3) (5 (x-2) x+6) x^2 y^5+2 (x ((21\right.\right.\\ & (17 x-75) x+1483) x+738)-1410) x y^4+(((x (313 x+1243)-5793) x+6078) x+12) y^3+(((3857\\& \left.\left.\left.\left.-739 x) x-5334) x+174) y^2+(3 (721-268 x) x-347) y-331 x+251\right)-69\right)\right)\right)\\ & -\frac{c_2 x y}{98304 \pi ^3 (x-1)^3 (y-1)^2} m_c^2 \left(s (x-1) (y-1) \left(y c_p \left(-44 x ((x ((x-4) x+6)-6) x+2) y^4+4 ((((23-8 x) x\right.\right.\right.\\ & \left.-82) x+31) x+2) y^3-2 (((x-112) x+28) x+8) y^2+(14-x (67 x+14)) y+14 x-6\right)+c_p+2 \left(x y \left(y \Big(140\right.\right.\\ & ((x-2) ((x-2) x+2) x+2) x y^4-2 ((((11 x-58) x+108) x+158) x+50) y^3+((5 x (23-8 x)+519) x\\ & \left.\left.\left.+182) y^2+(2 (x-182) x-135) y+73 x+57\Big)-5\right)-18 (y-1) y-6\right)\right)-4 m_c^2 \left(2 ((x-2) ((x-2) x+2) x+2)\right.\\ & \left.\left.x y^4+((x-4) x (x+1)-2) y^3+(x (6-(x-3) x)+4) y^2-(5 x+3) y+x+1\right)\right)\Bigg\},\\ \end{aligned} $

(24) where the coefficient
$ c_p=1 $ for current$ J_{2,\mu\nu}^{A(S)} $ and$ c_p=-1 $ for current$ J_{8,\mu\nu}^{A(S)} $ . The spectral functions for the$ (1,1\{0,1\}) $ structure are given as$ \begin{aligned}[b] \rho^{pert}_{3,9;A(S)}(s) =& -\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{{\rm i} x^2}{51609600 \pi ^5 (y-1)^5} F(s,x,y)^3 c_1 \left((x-1) x \left(-40 \left((27 x-14) x+4 \left(5 x^2-7\right) c_p-42\right) x^2\right.\right.\\ & y^3-56 x \left(7 (12 x-7) x+((3 x-4) x+10) c_p+25\right) y^2+7 \left(x (140-57 x)+(x (50-99 x)+10) c_p\right) y\\ & \left.+35 \left(x+(3 x-2) c_p\right)\right) F(s,x,y)^3+42 x \left(\left(-20 c_p (y-1)-110 (y-1)-75 x+((y (443 x-8 ((27 x-88) x\right.\right.\\ & +40) y-1026)-120) x+470 y+140) x y+2 (y ((50-y (41 x+8 ((x-9) x+5) y+38)) x+40 y-20)-15) x\\ & \left. c_p\right) m_c m_s-s (x-1) (y-1) y \left((100 (5 y-4) y+(2 (909 x+10 (x (27 x-14)-42) y-364) y+127) x y+95) x\right.\\ & \left.\left.+2 \left(x \left(y \left(\left(\left(3 x+40 \left(5 x^2-7\right) y+16\right) y+144\right) x+110 y-80\right)-15\right)-10 (y-1)\right) c_p\right)\right) F(s,x,y)^2+30 (y-1)\\& \left((x-1) (y-1) \left(50 \left((27 x-14) x+4 \left(5 x^2-7\right) c_p-42\right) x y^3-14 \left(-303 x^2+93 x+4 (2 x-5) (3 x+1) c_p-75\right)\right.\right.\\ & \left.y^2+7 \left(37 x+20 (3 x-2) c_p-130\right) y+315\right) \left(-s^2\right) x^2 y^2+14 \left(x \left(y \left(-75 x+2 \left((37 x-50) x+((7 x-20) x\right.\right.\right.\right.\\ & \left.\left.\left.\left.+10) c_p+20\right) y+5 (6 x-4) c_p+70\right)-5\right)-30 (y-1)\right) m_c^2 m_s^2-7 s x \left(\left((-10 (115 y+12) y+((-719 x+22 ((27 \right.\right.\\ & x-88) x+40) y+2190) y+26) x y+95) x+350 y+4 (10 (y-1)+(((y (59 x+11 ((x-9) x+5) y+50)-71) x\\ & \left.\left.\left.-55 y+35) y+15) x) c_p-270\right) y+60\right) m_c m_s\right) F(s,x,y)-120 s (y-1)^2 \left((x-1) (y-1) \left(70 (y-1) y+\left(4 \Big((27 x\right.\right.\right.\\ & \left.\left.-14) x+4 \left(5 x^2-7\right) c_p-42\Big) y^2-14 \left(-23 x+4 (x-2) c_p+5\right) y+21\right) x y+35\right) s^2 x^2 y^3+7 \left(50 (y-1) y\right.\\ & +\Big(\left((y (-97 x+4 ((27 x-88) x+40) y+342)-16) x-190 y+8 (7 x+((x-9) x+5) y-5) (x y-1) c_p+20\right) y\\ & \left.+5\Big) x y+20\right) s x m_c m_s y+14 \left(30 (y-1) y+\left(-4 \left(\left(7 c_p+37\right) x^2-10 \left(2 c_p+5\right) x+10 \left(c_p+2\right)\right) y^2-5 (3 x-2)\right.\right.\\ & \left.\left.\left.\left. \left(4 c_p-3\right) y+10\right) x y+15\right) m_c^2 m_s^2\right)\right), \end{aligned} $

$ \begin{aligned}[b] \rho^{\langle\bar{s}s\rangle}_{3,9;A(S)}(s) =& -\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{c_1 x^2}{3072 \pi ^3 (y-1)^4} m_c F(s,x,y)^2 \left(4 s (y-1) (y (x (y (x (y (22 (x-3) (2 x-1) y-34 x+153)\right.\\ & -12)-75 y-4)+9)+19 y-15)+3) F(s,x,y)+(x y-1) (x (y (8 (x-3) (2 x-1) y-8 x+13)-5)-5 y+5)\\ & \left. F(s,x,y)^2+12 s^2 (y-1)^2 y (y (x y (x (y (4 (x-3) (2 x-1) y-4 x+25)-4)-13 y+2)+x+3 (y-1))+1)\right),\\ \rho^{\langle m_s\bar{s}s\rangle}_{3,9;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{c_1 x}{6144 \pi ^3 (y-1)^3} F(s,x,y) \left(12 s (y-1) F(s,x,y) \left(2 m_c^2 (y (x (2 y (2 ((5 x-6) x+2) y-2 x+1)\right.\right.\\ & \left.-1)-2 y+2)-1)+s (x-1) x (y-1) y^2 (y (x (2 y (25 (x (3 x-2)-2) y+130 x-21)+11)+30 y-26)+9)\right)\\ & +4 F(s,x,y)^2 \left(4 m_c^2 (y (x (((5 x-6) x+2) y-4 x+3)-1)+1)+s (x-1) x (y-1) y (x y (2 y (70 (x (3 x-2)-2) y\right.\\ &\left.+385 x-88)+35)+20 (5 y-4) y+19)\right)+(x-1) c_p \left(8 s (y-1) y (x (y (11 ((x-4) x+2) y+30 x-16)-3)\right.\\ & -2 y+2) F(s,x,y)+(x (y (8 ((x-4) x+2) y+21 x-10)-3)-2 y+2) F(s,x,y)^2+48 s^2 x (y-1)^2 y^3 (((x-4)\\ & \left. x+2) y+3 x-2)\right) F(s,x,y)+(x-1) x (y (x (8 y (5 (x (3 x-2)-2) y+35 x-13)+13)+40 y-28)-1)\\ & \left. F(s,x,y)^3+24 s^3 (x-1) x (y-1)^3 y^3 \left(y \left(4 (x (3 x-2)-2) x y^2+2 ((10 x-1) x+1) y+x-2\right)+1\right)\right), \end{aligned} $

$\begin{aligned} \rho^{\langle g_sG^2\rangle}_{3,9;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{x^2 c_1}{30965760 \pi ^5 (x-1)^3 (y-1)^5} m_c \left((x-1) x \left(\left(y \left(40 (x (27 x-14)-42) x y^2+56 (7 (12 x-7)\right.\right.\right.\right.\\ & \left.\left. x+25) y+399 x-980\right)-35\right) (x y-1) ((x+((x-3) x+3) y-3) y+1) x m_c+21 \left(8 ((x (3 (9 x-47) x+281)\right.\\ & -268) x+80) x^2 y^5+(x (((1743-451 x) x-2629) x+2836)-1050) x y^4+((((121-15 x) x-900) x+120) x\\ & \left.+370) y^3+(x ((38 x-175) x+695)-660) y^2+5 (3 (9 x-23) x+74) y+45 x-80\right) m_s+c_p \Big((x y-1)\\ & ((x+((x-3) x+3) y-3) y+1) \Big(x \left(70 (8 y-5) y+\left(8 y \left(7 (3 x-4)+20 \left(5 x^2-7\right) y\right)+693\right) x y-105\right)-70\\& (y-1)\Big) m_c+42 \Big(8 ((x ((x-13) x+38)-44) x+15) x^2 y^5+(x (((57 x-191) x+173) x+188)-140) x y^4\\ & +((((103-15 x) x-420) x+180) x+30) y^3-(((16 x-185) x+25) x+60) y^2-35 x (x+1) y+40 y+15 x\\ & \left.-10\Big) m_s\Big)\right) F(s,x,y)^3+21 \left((y ((x ((x-3) x+3) y-3) y+3)-1) x \left(110 (y-1)+(-10 (47 y+14) y+((-443 x\right.\right.\\ & +8 ((27 x-88) x+40) y+1026) y+120) x y+75) x+2 (10 (y-1)+(((y (41 x+8 ((x-9) x+5) y+38)-50)\\ & \left.x-40 y+20) y+15) x) c_p\right) m_s m_c^2+(x-1) (y-1) \left(s x y (y ((x ((x-3) x+3) y-3) y+3)-1) \left((100 (5 y-4) y\right.\right.\\ & +(2 (909 x+10 (x (27 x-14)-42) y-364) y+127) x y+95) x+2 \left(x \left(y \left(\left(\left(3 x+40 \left(5 x^2-7\right) y+16\right) y+144\right)\right.\right.\right. \\ & \left.\left.\left.\left.x+110 y-80\right)-15\right)-10 (y-1)\right) c_p\right)-6 ((((x-2) x+2) y-2) y+1) \left(x \left(y \left(-75 x+2 \left((37 x-50) x+((7 x\right.\right.\right.\right.\\ & \left.\left.\left.\left.\left.-20) x+10) c_p+20\right) y+5 (6 x-4) c_p+70\right)-5\right)-30 (y-1)\right) m_s^2\right) m_c+3 (x-1) (y-1) \Big(22 \left((x (3 (9 x-47)\right.\\ & \left.x+281)-268) x+2 ((x ((x-13) x+38)-44) x+15) c_p+80\right) x^2 y^6+\left((((2833-741 x) x-4217) x+5860)\right.\\ & \left.x+4 (x (((81 x-278) x+272) x+230)-185) c_p-2490\right) x y^5+\left((((109-15 x) x-2952) x+1200) x+4\right.\\ & \left.((((121-15 x) x-573) x+270) x+30) c_p+810\right) y^4-\left((x (18 x-877)-235) x+4 \Big(\left(22 x^2-278 x+85\right) x\right.\\ & \left.+60\Big) c_p+1460\right) y^3+\left((41 x-535) x-16 (x (14 x+5)-10) c_p+1160\right) y^2+5 \left(27 x+4 (3 x-2) c_p-86\right) y\\& \left.+60\Big) s x m_s\right) F(s,x,y)^2-6 (y-1) \left(14 (y ((x ((x-3) x+3) y-3) y+3)-1) \left(x \left(y \left(-75 x+2 \left((37 x-50) x\right.\right.\right.\right.\right.\\ & \left.\left.\left.\left.+((7 x-20) x+10) c_p+20\right) y+5 (6 x-4) c_p+70\right)-5\right)-30 (y-1)\right) m_s^2 m_c^3-7 s x (y ((x ((x-3) x+3) y-3)\\ & y+3)-1) \left(\left((-10 (115 y+12) y+((-719 x+22 ((27 x-88) x+40) y+2190) y+26) x y+95) x+350 y+4\right.\right.\\ & \left.\left.(10 (y-1)+(((y (59 x+11 ((x-9) x+5) y+50)-71) x-55 y+35) y+15) x) c_p-270\right) y+60\right) m_s m_c^2\\ & -s (x-1) (y-1) \left(s x^2 y^2 (y ((x ((x-3) x+3) y-3) y+3)-1) \left(50 \left((27 x-14) x+4 \left(5 x^2-7\right) c_p-42\right) x y^3\right.\right.\\ & \left.-14 \left(-303 x^2+93 x+4 (2 x-5) (3 x+1) c_p-75\right) y^2+7 \left(37 x+20 (3 x-2) c_p-130\right) y+315\right)-42 ((((x-2)\\ & x+2) y-2) y+1) \left(4 \left(\left(7 c_p+37\right) x^2-10 \left(2 c_p+5\right) x+10 \left(c_p+2\right)\right) x y^3+5 \left(x (3 x-2) \left(4 c_p-3\right)-6\right) y^2\right.\\ \end{aligned} $

$ \begin{aligned}[b]& \left.\left.-10 (x-3) y-15\right) m_s^2\right) m_c-21 s^2 (x-1) x (y-1) y \left(\left(4 \left((x (3 (9 x-47) x+281)-268) x+2 ((x ((x-13) x\right.\right.\right.\\& \left.+38)-44) x+15) c_p+80\right) x^2 y^5+\Big((((373-101 x) x-531) x+892) x+8 (x (((8 x-29) x+33) x+14)-15)\\& c_p-410\Big) x y^4+\left(\left(((23-5 x) x-588) x+24 ((2 x-17) x+10) c_p+320\right) x+110\right) y^3-\left(\left((6 x-223) x+8 ((2 x\right.\right.\\ & \left.\left.\left.\left.\left.-31) x+20) c_p+95\right) x+220\right) y^2-x \left(11 x+8 (7 x-5) c_p+35\right) y+210 y+15 x-100\right) y+20\right) m_s\right) F(s,x,y)\\ & -6 s (y-1)^2 (y ((x ((x-3) x+3) y-3) y+3)-1) m_c \left((x-1) (y-1) \left(70 (y-1) y+\left(4 \left((27 x-14) x+4 \left(5 x^2-7\right)\right.\right.\right.\right.\\ & \left.\left.\left. c_p-42\right) y^2-14 \left(-23 x+4 (x-2) c_p+5\right) y+21\right) x y+35\right) \left(-s^2\right) x^2 y^3-7 s x \left(50 (y-1) y+\left(\left((y (-97 x+4 ((27\right.\right.\right.\\ & \left.\left.\left. x-88) x+40) y+342)-16) x-190 y+8 (7 x+((x-9) x+5) y-5) (x y-1) c_p+20\right) y+5\right) x y+20\right) m_c m_s\\ & y+14 \left(4 \left((37 x-50) x+((7 x-20) x+10) c_p+20\right) x y^3+5 \left(x (3 x-2) \left(4 c_p-3\right)-6\right) y^2-10 (x-3) y-15\right)\\ & m_c^2 m_s^2\Big)\Big)\Bigg\}, \end{aligned} $

$\begin{aligned}[b]\rho^{\langle\bar{s}\sigma\cdot Gs\rangle}_{3,9;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1 x}{3072 \pi ^3 (y-1)^3} m_c F(s,x,y) \left(3 s (y-1) \left(y \left(4 (x-1) (y-1) c_p (x y (11 x y-10)+1)\right.\right.\right.\\ & \left.\left.+x (x y (y (22 ((6 x-25) x+9) y-73 x+517)-51)-7 (29 y+2) y+30)+41 y-33\right)+6\right) F(s,x,y)\\& +\left(2 (x-1) (y-1) c_p (x y (8 x y-7)+1)+x y (x (y (8 ((6 x-25) x+9) y-65 x+223)+11)-75 y-26)+12 x\right.\\ & \left.+9 y-9\right) F(s,x,y)^2+6 s^2 (y-1)^2 y \left(x y \left(y \left(8 (x-1) (y-1) c_p (x y-1)+x (y (4 ((6 x-25) x+9) y-7 x+89)\right.\right.\right.\\ & -17)-37 y+6\Big)+4\Big)+7 (y-1) y+2\Big)\Big)-\frac{c_2 x^2}{2048 \pi ^3 (x-1) (y-1)^4} m_c F(s,x,y) \left(3 s (y-1) \left(y \left(44 ((x-3) x+1)\right.\right.\right.\\ & ((x-1) x+1) x y^4+(x (((197-56 x) x-47) x+12)-18) y^3+((15-22 x (x+3)) x+32) y^2+3 (4 x+5) x y\\ & \left.\left.-9 x-36 y+18\right)-3\right) F(s,x,y)+\left(16 ((x-3) x+1) ((x-1) x+1) x y^4+(x (((103-32 x) x-53) x+24)\right.\\ & \left.-10) y^3-(x ((7 x+11) x+14)-16) y^2+9 (x-1) y-3 x+3\right) F(s,x,y)^2+6 s^2 (y-1)^2 y \left(y \left(\left(x \left(-8 x^3+29 x^2\right.\right.\right.\right.\\ & \left.\left.\left.+x-4\right)-2\right) y^3+8 ((x-3) x+1) ((x-1) x+1) x y^4+((9-4 x (x+4)) x+4) y^2+(x (4 x-1)-6) y-x+4\right)\\& -1\Big)\Big)\Bigg\}, \end{aligned} $

$\begin{aligned}[b] \rho^{m_s\langle\bar{s}\sigma\cdot Gs\rangle}_{3,9;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1}{9216 \pi ^3 (y-1)^2} \left(c_p \left(24 s x (y-1) y^2 F(s,x,y) \left(6 m_c^2 (((x-4) x+2) y+3 x-2)+s\right.\right.\right.\\& \left.(x-1) (y-1) y \left(x \left(25 \left(x^2-2\right) y^2+2 (25-9 x) y-3\right)-2 y+2\right)\right)+6 y F(s,x,y)^2 \left(6 x m_c^2 (((x-4) x+2) y+3 x\right.\\ & \left.-2)+s (x-1) (y-1) \left(x \left(y \left(x \left(7 y \left(20 \left(x^2-2\right) y-9 x+40\right)-36\right)-22 y+16\right)+3\right)+2 (y-1)\right)\right)+(x-1) \\ & \left(x \left(y \left(x \left(8 y \left(10 \left(x^2-2\right) y-3 x+20\right)-27\right)-16 y+10\right)+3\right)+2 (y-1)\right) F(s,x,y)^3+48 s^3 (x-1) x^2 (y-1)^3\\ & \left. y^5 \left(\left(x^2-2\right) y-x+2\right)\right)-x \left(6 s (y-1) y F(s,x,y) \left(6 m_c^2 \left(8 (1-2 x)^2 y^2-3 x y-1\right)+s (x-1) (y-1) y \left(y \left(x \Big(50\right.\right.\right.\right.\\ & \left.\left.\left.(5 x (3 x-2)-6) y^2+(954 x-66) y+29\Big)+30 y-26\right)+9\right)\right)+3 F(s,x,y)^2 \left(6 m_c^2 \Big(y \left(4 (1-2 x)^2 y-9 x+4\right)\right.\\& \left.+1\Big)+s (x-1) (y-1) y (x y (2 y (70 (5 x (3 x-2)-6) y+1401 x-164)+83)+20 (5 y-4) y+19)\right)+(x-1)\\ & (y (x (8 y (5 (5 x (3 x-2)-6) y+126 x-29)+21)+40 y-28)-1) F(s,x,y)^3+6 s^3 (x-1) (y-1)^3 y^3 (x y (2 y\\& \left.\left.(2 (5 x (3 x-2)-6) y+37 x-1)+3)+2 (y-1) y+1)\right)\right)+\frac{c_2 x}{4096 \pi ^3 (x-1) (y-1)^3} \left(6 s (y-1) F(s,x,y)\right.\\ & \left(2 m_c^2 (y (x y (x (y (4 (4 x-3) (x-1) y-12 x+25)-4)-13 y+2)+x+3 (y-1))+1)+s (x-1) x (y-1) y^2 (y (x\right.\\ & \left.(x y (y (50 (2 x-3) (x+1) y+62 x+65)-148)+5 (33 y-4) y-11)-37 y+33)-9)\right)+3 (x-1) F(s,x,y)^2\\ & \left(2 m_c^2 (y (2 (4 x-3) x y-2 x+1)-1) (x y-1)+s x (y-1) y (y (x (y (x (2 y (70 (2 x-3) (x+1) y+108 x+55)-415)\right.\\ \end{aligned} $

$ \begin{aligned}[b]& \left.+470 y-26)-38)-108 y+92)-21)\right)-(x-1)^2 (y-1) c_p F(s,x,y) \left(6 s (y-1) y (x y (11 x y-10)+1) F(s,x,y)\right.\\ & \left.+(x y (8 x y-7)+1) F(s,x,y)^2+24 s^2 x (y-1)^2 y^3 (x y-1)\right)+(x-1) x (y (x (y (x (4 y (10 (2 x-3) (x+1) y+26 x\\ & -5)-139)+140 y+22)-20)-30 y+26)-3) F(s,x,y)^3+6 s^3 (x-1) x (y-1)^3 y^3 (y (x (y (x (y (4 (2 x-3)\\ & \left.(x+1) y+4 x+7)-12)+13 y-2)-1)-3 y+3)-1)\right)\Bigg\}, \end{aligned} $

$\begin{aligned}[b]\rho^{\langle\bar{s}s\bar{s}s\rangle}_{3,9;A(S)}(s) =& \int^{z_{\max}}_{z_{\min}}{\rm d}z\frac{c_1 c_p}{768 \pi } m_s^2 G(s,z) (G(s,z)+4 s (z-1) z),\\ \rho^{\langle\bar{s}s\rangle\langle\bar{s}\sigma\cdot Gs\rangle}_{3,9;A(S)}(s) =& \int^{z_{\max}}_{z_{\min}}{\rm d}z\Bigg\{-\frac{c_1}{2304 \pi } \left(m_s^2 \left(\left((4 z (5-6 z)+1) c_p+4 z (7-10 z)+1\right) G(s,z)-s (z-1) z \left(\left(66 z^2-58 z-2\right) c_p\right.\right.\right.\\ & \left.\left.+20 (5 z-4) z+19\right)\right)-m_c m_s \left(\left(6 c_p+13\right) G(s,z)+s \left(z \left(12 (z-1) c_p+29 z-21\right)+6\right)\right)+6 m_c^2 (2 G(s,z)\\ & +2 s (z-1) z+s)\Big)+\frac{c_2 c_p}{1024 \pi } m_s^2 (G(s,z)+2 s (z-1) z)\Bigg\}+\int^{1}_{0}dz\Bigg\{\frac{c_1}{2304 \pi } s^2 (z-1) z m_s \Big((z-1) z m_s \Big(12 (z-1) z\\\ & \left.\left.c_p+30 z^2-26 z+9\right)+(3 (z-1) z+2) m_c\right)\Bigg\}, \end{aligned} $

$\begin{aligned}[b] \rho^{\langle g_sG^2\rangle\langle \bar{s}s\rangle}_{3,9;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1 x^2}{18432 \pi ^3 (x-1)^3 (y-1)^4} m_c \left(2 F(s,x,y) \left(m_c^2 (x y-1)^2 (y (((x-3) x+3) y+x-3)+1)\right.\right.\\ & (x (y (8 (x-3) (2 x-1) y-8 x+13)-5)-5 y+5)+3 s (x-1) (y-1) \left(y \left(22 ((x (2 (x-5) x+19)-18) x+5)\right.\right.\\ & x^2 y^5+(x (((209-56 x) x-234) x+350)-137) x y^4+(37-2 x (x (32 x+87)-36)) y^3+2 (x (x (11 x+40)\\ & \left.\left.\left.-2)-33) y^2-6 (2 x (x+2)-9) y+9 x-21\right)+3\right)\right)+3 (x-1) \left(8 ((x (2 (x-5) x+19)-18) x+5) x^2 y^5\right.\\ & +(x (((119-32 x) x-146) x+162)-55) x y^4+((x+2) ((x-26) x+4) x+15) y^3+2 (x ((5 x+2) x+14)-13)\\ & \left.y^2+2 (x-7) (x-1) y+3 (x-1)\right) F(s,x,y)^2+2 s (y-1) \left(m_c^2 (x y-1) (y (((x-3) x+3) y+x-3)+1) (y (x (y\right.\\ & (x (y (22 (x-3) (2 x-1) y-34 x+153)-12)-75 y-4)+9)+19 y-15)+3)+3 s (x-1) (y-1) y \Big(y \Big(4 ((x\\ & (2 (x-5) x+19)-18) x+5) x^2 y^5+2 \left(5-2 x^2\right) y+(x (((29-8 x) x-28) x+54)-23) x y^4+(5-4 x (3 x (x\\ & \left.\left.+3)-5)) y^3+2 (x (2 x (x+5)-5)-5) y^2+x-5\Big)+1\Big)\right)\right)\Bigg\}-\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\frac{c_1 s^2 x^2 y }{9216 \pi ^3 (x-1)^3 (y-1)^2}m_c^3\\ & (x y-1) (y (((x-3) x+3) y+x-3)+1) (y (x y (x (y (4 (x-3) (2 x-1) y-4 x+25)-4)-13 y+2)+x\\ & +3 (y-1))+1), \end{aligned} $

$\begin{aligned}[b] \rho^{m_s\langle g_sG^2\rangle\langle \bar{s}s\rangle}_{3,9;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 x}{18432 \pi ^3 (x-1)^3 (y-1)^3}m_c^2 \left((x-1) \left(c_p (x y-1) (y (((x-3) x+3) y+x-3)+1) (x\right.\right.\\ & (y (8 ((x-4) x+2) y+21 x-10)-3)-2 y+2)+8 ((x ((35 x-118) x+104)-24) x+45) x^2 y^5\\ & +40 \left(x^2 ((3 x-11) x+13)-6\right) x^3 y^6+(x (x (x (73 x+101)-669)-204)-72) x y^4+(x (((935-169 x) x\\ & \left.-310) x+252)-24) y^3+(((179-220 x) x-193) x+48) y^2+(-61 (x-1) x-36) y+x+12\right) F(s,x,y)\\ & +4 m_c^2 (x y-1) (y (((x-3) x+3) y+x-3)+1) (y (x (((5 x-6) x+2) y-4 x+3)-1)+1)+s (x-1) (y-1)\\ & \left(y \left(2 c_p (x y-1) (y (((x-3) x+3) y+x-3)+1) (x (y (11 ((x-4) x+2) y+30 x-16)-3)-2 y+2)+2 (((11\right.\right.\\ & (35 x-113) x+839) x+6) x+570) x^2 y^5+140 \left(x^2 ((3 x-11) x+13)-6\right) x^3 y^6+(x (x (x (155 x+691)\\ & -2229)-936)-204) x y^4+(x (((2353-425 x) x-92) x+492)-24) y^3+(((209-656 x) x-409) x+48) y^2\\ & \left.\left.\left.+((173-65 x) x-48) y-25 x+24\right)-6\right)\right)\Bigg\}+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{-\frac{{\rm i} c_1 s x}{18432 \pi ^3 (x-1)^3 (y-1)^2} m_c^2 (y (y (x ((x-3) \end{aligned} $

$ \begin{aligned}[b]& x+3) y-3)+3)-1) \left(2 m_c^2 \left(y \left(-4 x ((5 x-6) x+2) y^2+2 ((2 x-1) x+1) y+x-2\right)+1\right)-s (x-1) x (y-1) y^2\right.\\ & \left.\left(y \left(4 c_p (((x-4) x+2) y+3 x-2)+x (2 y (25 (x (3 x-2)-2) y+130 x-21)+11)+30 y-26\right)+9\right)\right)\Bigg\}, \end{aligned} $

$\begin{aligned}[b] \rho^{\langle g_sG^2\rangle\langle \bar{s}\sigma\cdot Gs\rangle}_{3,9;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1 x}{36864 \pi ^3 (x-1)^3 (y-1)^3} m_c \left(3 (x-1) \left(2 (x-1) (y-1) c_p (x y (y (x (y (8 (2 x-3) (x-1) y\right.\right.\\& -2 x+21)-8)-25 y+18)-5)+3 (y-1) y+1)+8 \left(\left(x \left(6 x^2-32 x+63\right)-62\right) x+15\right) x^2 y^5+(x (((374\\ & -105 x) x-379) x+490)-137) x y^4+(((2 x (3 x-68)-165) x+20) x+23) y^3+(x ((45 x+34) x+57)-38)\\ & \left.y^2+((5 x-36) x+19) y+7 x-4\right) F(s,x,y)+m_c^2 (y (y (x ((x-3) x+3) y-3)+3)-1) \left(2 (x-1) (y-1) c_p (x y\right.\\ & \left.(8 x y-7)+1)+x y (x (y (8 ((6 x-25) x+9) y-65 x+223)+11)-75 y-26)+12 x+9 y-9\right)+3 s (x-1)\\ & (y-1) \left(y \left(4 (x-1) (y-1) c_p (x y (y (x (y (11 (2 x-3) (x-1) y-2 x+27)-11)-34 y+27)-8)+3 (y-1) y\right.\right.\\ & +1)+22 \left(\left(x \left(6 x^2-32 x+63\right)-62\right) x+15\right) x^2 y^5+(x ((3 (218-61 x) x-547) x+1118)-361) x y^4\\ & +(((x (2 x-341)-666) x+212) x+67) y^3+(x (19 x (6 x+17)-33)-118) y^2+(100-x (53 x+59)) y+26 x\\ & \left.\left.-41\Big)+6\right)\right)+\frac{c_2 x^2 y}{24576 \pi ^3 (x-1)^2 (y-1)^2} m_c^3 (x y-1) (y (x (y (8 ((x-1) x+1) y+26 x-17)-3)+5 y-1)-2)\Bigg\}\\ & +\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{\frac{c_1 s x}{36864 \pi ^3 (x-1)^3 (y-1)^2} m_c \left(m_c^2 (-(y (y (x ((x-3) x+3) y-3)+3)-1)) \left(y \left(4 (x-1) (y-1)\right.\right.\right.\\ & \left.c_p (x y (11 x y-10)+1)+x (x y (y (22 ((6 x-25) x+9) y-73 x+517)-51)-7 (29 y+2) y+30)+41 y-33\right)\\ & +6\Big)-3 s (x-1) (y-1) y \left(y \left(4 x^2 y^5 \left(2 (2 x-3) (x-1)^2 c_p+\left(x \left(6 x^2-32 x+63\right)-62\right) x+15\right)-x y^4 \left(8 (2 x-3)\right.\right.\right.\\& \left. (x-1) ((x-1) x-1) c_p+(x ((27 x-94) x+61)-182) x+63\right)+y^3 \left(9-x \left(24 (x-2) (x-1) c_p+(62 x\right.\right.\\ & \left.\left.+141) x-60\right)\right)+y^2 \left(x \left(8 (x-4) (x-1) c_p+(21 x+80) x-33\right)-18\right)+y \left(x \left(8 (x-1) c_p-17 x+2\right)+19\right)\\ & \left.\left.+3 x-10\Big)+2\right)\right)+\frac{c_2 s x^2 y^3}{24576 \pi ^3 (x-1)^2 (y-1)} m_c^3 (x (y (x (y (22 ((x-1) x+1) y+32 x-17)-47)-13 y+20)\\ & +3)+y-1)\Bigg\},\\ \rho^{m_s\langle g_sG^2\rangle\langle \bar{s}\sigma\cdot Gs\rangle}_{4;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1}{110592 \pi ^3 (x-1)^2 (y-1)^2} m_c^2 \left(-8 x^2 y^5 \left((x (x ((3 x-29) x+101)-66)-54) c_p\right.\right.\\& \left.+(((126 x-407) x+245) x+48) x+105\right)+40 ((x-3) x+3) x^3 y^6 \left(2 \left(x^2-2\right) c_p+5 x (2-3 x)+6\right)\\ & +x y^4 \left(((x ((9 x+115) x+395)-1368) x+198) c_p+((2853-x (309 x+845)) x+828) x-24\right)+y^3 \left((x (x ((31\right.\\ & \left.x-443) x+1384)-372)-6) c_p+(x ((763 x-3247) x+414)-204) x\right)+y^2 \left((((60 x-601) x+253) x+12)\right.\\ & \left.\left. c_p+((702 x-295) x+157) x\right)+y \left((x (135 x-73)-8) c_p+61 (3 x-1) x\right)-3 x c_p+2 c_p-19 x\right)\\ & +\frac{c_2 x y}{49152 \pi ^3 (x-1)^2 (y-1)^2} m_c^2 \left(c_p \left(8 ((x ((x-4) x+6)-6) x+2) x y^4+(x (x ((5 x-14) x+60)-24)-2) y^3\right.\right.\\& \left.-(x (x (x+45)-15)-4) y^2+(2 x-1) (8 x+3) y-2 x+1\right)+x y \left(40 \left(x^2 ((x-4) x+6)-2\right) x y^4+4 ((x ((34 x\right.\\& \left.-111) x+81)-16) x+10) y^3+(3 x ((36 x-109) x+61)-68) y^2+((99 x-86) x+43) y+39 x-13\right)-10 x\\ & +6 y-6\Big)\Bigg\}+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{-\frac{c_1}{110592 \pi ^3 (x-1)^3 (y-1)^2} m_c^2 \left(6 x m_c^2 (y (y (x ((x-3) x+3) y-3)+3)-1) \Big(y \right.\\& \left(2 c_p (((x-4) x+2) y+3 x-2)-4 (1-2 x)^2 y+9 x-4\right)-1\Big)+s (x-1) (y-1) y \left(-2 x^2 y^5 \left((x (x (7 (9 x-67) x\right.\right.\\& \left.+1471)-906)-774) c_p+(((1401 x-4367) x+1595) x+1458) x+1410\right)+140 ((x-3) x+3) x^3 y^6 \left(2 \Big(x^2\right.\\ & \left.-2\Big) c_p+5 x (2-3 x)+6\right)+x y^4 \Big((2 (x (328 x+467)-2070) x+432) c_p+((9669-x (659 x+4243)) x+3216)\\ \end{aligned} $

$ \begin{aligned}[b] &\left.\left.x+12\Big)-y^3 \left(2 (((x (29 x+560)-1891) x+408) x+6) c_p+((5 x (1741-427 x)+708) x+252) x\right)+y^2 \left(2\right.\right.\right.\\ & \left.((11 (9 x-68) x+277) x+12) c_p+((2244 x-145) x+289) x\right)+y \left(2 (x (144 x-79)-8) c_p+(137 x-173) x\right)\\ & \left.\left.+(4-6 x) c_p+37 x\right)\right)+\frac{c_2 x y }{49152 \pi ^3 (x-1)^3 (y-1)^2}m_c^2 \left(2 m_c^2 \left(2 ((x ((x-4) x+10)-8) x+2) x y^4+(x (5 x (1-3 x).\right.\right.\\& \left.+4)-2) y^3+((x (7 x+11)-10) x+4) y^2+((3-8 x) x-3) y+x+1\right)+s (x-1) (y-1) y \left(2 c_p \left(11 ((x ((x-4) x\right.\right.\\& +6)-6) x+2) x y^4+(x (x ((8 x-23) x+87)-36)-2) y^3-(x (x (x+63)-24)-4) y^2+(2 x-1) (11 x+3) y\\ & \left.-2 x+1\right)+x \left(y \left(140 \left(x^2 ((x-4) x+6)-2\right) x y^4+2 ((((207 x-668) x+358) x+2) x+50) y^3+(x ((340 x\right.\right.\\ & \left.\left.\left.\left.-829) x+465)-180) y^2+((305 x-262) x+109) y+41 x-19\right)-8\right)-6 y+6\right)\right)\Bigg\}, \end{aligned} $

(25) where the coefficient
$ c_p=1 $ for current$ J_{3,\mu\nu}^{A(S)} $ and$ c_p=-1 $ for current$ J_{9,\mu\nu}^{A(S)} $ . The spectral functions for the$ (0,2\{1,1\}) $ structure with$ \mathbb{C}=+1 $ are given as$ \begin{aligned}[b] \rho^{pert}_{4;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{c_1 x^3}{12902400 \pi ^5 (y-1)^5} \left(c_p+1\right) F(s,x,y)^3 \left(-15 (y-1) F(s,x,y) \left(7 s x y m_c m_s (11 x y (2 x y+3)\right.\right.\\ & \left.-10) (x y-1)+14 m_c^2 m_s^2 (x y (2 x y-5)+5)-6 s^2 (x-1) x^2 (y-1) y^3 (5 x y (5 x y+7)-7)\right)+21 x F(s,x,y)^2 \Big(20 s\\ & (x-1) x (y-1) y^2 (x y+2) (3 x y-1)-m_c m_s (x y-1) (x y+3) (8 x y-5)\Big)+2 (x-1) x^2 y (10 x y (3 x y+7)-49)\\ & F(s,x,y)^3-60 s x (y-1)^2 y^2 \Big(7 s x y m_c m_s (x y-1) (4 x y+5)+14 m_c^2 m_s^2 (4 x y-5)-2 s^2 (x-1) x^2 (y-1) y^3 (6 x y\\ & +7)\Big)\Big),\\ \rho^{\langle\bar{s}s\rangle}_{4;A(S)}(s) =& 0,\\ \rho^{\langle m_s\bar{s}s\rangle}_{4;A(S)}(s) =& 0,\\ \rho^{\langle g_sG^2\rangle}_{4;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 x^3}{15482880 \pi ^5 (x-1)^3 (y-1)^5} m_c \left(c_p+1\right) \left(6 (y-1) F(s,x,y) \left(6 s (x-1) x (y-1) y^2 m_c \Big(s x y\right.\right.\\ & (x y-1) (5 x y (5 x y+7)-7) (y (((x-3) x+3) y+x-3)+1)-7 m_s^2 (4 x y-5) (y (((x-2) x+2) y-2)+1)\Big)\\ &-7 s x y m_c^2 m_s (x y-1)^2 (11 x y (2 x y+3)-10) (y (((x-3) x+3) y+x-3)+1)-14 m_c^3 m_s^2 (x y-1) (x y (2 x y-5)\\ & +5) (y (((x-3) x+3) y+x-3)+1)-21 s^2 (x-1) x^2 (y-1) y^3 m_s (x y-1) (4 x y+5) (y (((x-3) x+3) y+x-3)\\ & +1)\Big)-21 F(s,x,y)^2 \left(2 (x-1) (y-1) m_c \left(3 m_s^2 (x y (2 x y-5)+5) (y (((x-2) x+2) y-2)+1)-10 s x^2 y^2 (x y-1)\right.\right.\\ & \left.(x y+2) (3 x y-1) (y (((x-3) x+3) y+x-3)+1)\right)+x m_c^2 m_s (8 x y-5) (x y-1)^2 (y (((x-3) x+3) y+x-3)\\ & \left.+1) (x y+3)+3 s (x-1) x (y-1) y m_s (11 x y (2 x y+3)-10) (x y-1) (y (((x-3) x+3) y+x-3)+1)\right)+(x-1)\\ & x (y (y (x ((x-3) x+3) y-3)+3)-1) F(s,x,y)^3 \left(4 x y m_c (10 x y (3 x y+7)-49)-21 m_s (x y+3) (8 x y-5)\right)\\& -6 s x (y-1)^2 y^2 m_c (y (y (x ((x-3) x+3) y-3)+3)-1) \left(7 s x y m_c m_s (x y-1) (4 x y+5)+14 m_c^2 m_s^2 (4 x y-5)\right.\\ & \left.\left.-2 s^2 (x-1) x^2 (y-1) y^3 (6 x y+7)\right)\right)+\frac{c_3}{11796480 \pi ^5 (y-1)^3} (x-1) x^2 \left(c_p+1\right) F(s,x,y)^2 \left(4 s (y-1) y (293 x y\right.\\ & \left.-27) F(s,x,y)+(181 x y-36) F(s,x,y)^2+588 s^2 x (y-1)^2 y^3\right)\Bigg\},\\ \rho^{\langle\bar{s}\sigma\cdot Gs\rangle}_{4;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1 x^2}{512 \pi ^3 (y-1)^3} m_c \left(c_p+1\right) (x y-1) F(s,x,y) \left(s (y-1) y (5 x y-2) F(s,x,y)+(x y-1)\right. \\ & \left. F(s,x,y)^2+2 s^2 x (y-1)^2 y^3\right)\Bigg\},\\ \rho^{m_s\langle\bar{s}\sigma\cdot Gs\rangle}_{4;A(S)}(s) =& -\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1 x}{2304 \pi ^3 (y-1)^2} \left(c_p+1\right) \left(18 s x (y-1) y^2 F(s,x,y) \left(s (x-1) (y-1) y (5 x y-1)-m_c^2\right)\right. \end{aligned} $

$ \begin{aligned}[b]& \left.+3 F(s,x,y)^2 \left(m_c^2 (3-3 x y)+10 s (x-1) x (y-1) y^2 (5 x y-2)\right)+2 (x-1) x y (10 x y-7) F(s,x,y)^3+6 s^3 (x-1)\right.\\ & \left. x^2 (y-1)^3 y^5\right)\Bigg\},\\ \rho^{\langle\bar{s}s\bar{s}s\rangle}_{4;A(S)}(s) = &0,\\ \rho^{\langle\bar{s}s\rangle\langle\bar{s}\sigma\cdot Gs\rangle}_{4;A(S)}(s) = &0,\\ \rho^{\langle g_sG^2\rangle\langle \bar{s}s\rangle}_{4;A(S)}(s) =& 0,\\ \rho^{m_s\langle g_sG^2\rangle\langle \bar{s}s\rangle}_{4;A(S)}(s) = &0,\\ \rho^{\langle g_sG^2\rangle^2\;A(S)}_4(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{c_1 x^5 y^4 m_c^4 \left(c_p+1\right) (10 x y (3 x y+7)-49)}{46448640 \pi ^5 (x-1)^2 (y-1)^2}\\ & +\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\frac{c_1 s x^5 y^5 m_c^4 \left(c_p+1\right) (x y+2) (3 x y-1)}{1327104 \pi ^5 (x-1)^2 (y-1)},\\ \rho^{\langle g_sG^2\rangle\langle \bar{s}\sigma\cdot Gs\rangle}_{4;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1 x^2}{6144 \pi ^3 (x-1)^3 (y-1)^3} m_c \left(c_p+1\right) (y (y (x ((x-3) x+3) y-3)+3)-1) \Big(3 (x-1)\\ & (x y-1) F(s,x,y)+m_c^2 (x y-1)^2+s (x-1) (y-1) y (5 x y-2)\Big)\Bigg\}+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{-\frac{c_1 s x^2 y}{18432 \pi ^3 (x-1)^3 (y-1)^2}\\ & m_c \left(c_p+1\right) (y (y (x ((x-3) x+3) y-3)+3)-1) \left(m_c^2 (5 x y-2) (x y-1)+3 s (x-1) x (y-1) y^2\right)\Bigg\},\\ \rho^{m_s\langle g_sG^2\rangle\langle \bar{s}\sigma\cdot Gs\rangle}_{10;A(S)}(s) = &\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1 x }{27648 \pi ^3 (x-1)^2 (y-1)^2}m_c^2 \left(c_p+1\right) (x y-1)^2 \left(x y \left(20 ((x-3) x+3) y^2+26 (x-3) y\right.\right.\\ & \left.+23\Big)+18 (y-1) y+9\right)\Bigg\}+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{-\frac{c_1 x}{27648 \pi ^3 (x-1)^3 (y-1)^2} m_c^2 \left(c_p+1\right) \left(s (x-1) x (y-1) y^2 (x y (x\right.\\ & y(10 y (5 ((x-3) x+3) y-2 x+6)-9)+42 (4-5 y) y-50)+42 (y-1) y+11)-3 m_c^2 (x y-1)^2 (y (((x-3) x\\ & \left.+3) y+x-3)+1)\right)\Bigg\},\\ \end{aligned} $

(26) where the coefficient
$ c_p=1 $ . The spectral functions for the$ (0,2\{1,1\}) $ structure with$ \mathbb{C}=-1 $ are given as$ \begin{aligned}[b]\\ \rho^{pert}_{10;A(S)}(s) =& -\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{c_1 x^3}{614400 \pi ^5 (y-1)^5} \left(c_p-1\right) F(s,x,y)^3 \left(5 (y-1) F(s,x,y) \left(2 s^2 (x-1) x^2 (y-1) y^3 (11 x y\right.\right.\\ & \left.-3)-5 m_c m_s (x y-1) \left(s x y (5 x y-2)-2 m_c m_s\right)\right)+x F(s,x,y)^2 \left(8 s (x-1) x (y-1) y^2 (7 x y-4)-15 m_c m_s\right.\\ & \left.(x y-1)^2\right)+4 (x-1) x^2 y (x y-1) F(s,x,y)^3+20 s x (y-1)^2 y^2 \left(5 m_c m_s \left(2 m_c m_s+s x y (1-x y)\right)+2 s^2 (x-1)\right.\\ & \left.\left.x^2 (y-1) y^3\right)\right),\\ \rho^{\langle\bar{s}s\rangle}_{10;A(S)}(s) = &0,\\ \rho^{\langle m_s\bar{s}s\rangle}_{10;A(S)}(s) = &0,\\ \rho^{\langle g_sG^2\rangle}_{10;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1 x^3}{737280 \pi ^5 (x-1)^3 (y-1)^5} m_c \left(c_p-1\right) \left(2 (y-1) F(s,x,y) \left(2 s (x-1) x (y-1) y^2 m_c\right.\right. \\ & \left(15 m_s^2 (y (((x-2) x+2) y-2)+1)+s x y (11 x y-3) (x y-1) (y (((x-3) x+3) y+x-3)+1)\right)-5 s x y m_c^2 m_s\\ & (x y-1)^2 (5 x y-2) (y (((x-3) x+3) y+x-3)+1)+10 m_c^3 m_s^2 (x y-1)^2 (y (((x-3) x+3) y+x-3)+1)\\ & \left.-15 s^2 (x-1) x^2 (y-1) y^3 m_s (y (y (x ((x-3) x+3) y-3)+3)-1)\right)+(x y-1) F(s,x,y)^2 \left(2 (x-1) (y-1) m_c\right.\\ & \left(15 m_s^2 (y (((x-2) x+2) y-2)+1)+4 s x^2 y^2 (7 x y-4) (y (((x-3) x+3) y+x-3)+1)\right)-15 x m_c^2 m_s (x y-1)^2\\ \end{aligned} $

$ \begin{aligned}[b] & \left.(y (((x-3) x+3) y+x-3)+1)-15 s (x-1) x (y-1) y m_s (5 x y-2) (y (((x-3) x+3) y+x-3)+1)\right)\\ & +(x-1) x (x y-1)^2 (y (((x-3) x+3) y+x-3)+1) F(s,x,y)^3 \left(8 x y m_c-15 m_s\right)+2 s x (y-1)^2 y^2 m_c (y (y (x ((x\\ & \left.-3) x+3) y-3)+3)-1) \left(-5 s x y m_c m_s (x y-1)+10 m_c^2 m_s^2+2 s^2 (x-1) x^2 (y-1) y^3\right)\right)+\frac{c_3}{2359296 \pi ^5 (y-1)^3}\\ & (x-1) x^2 \left(c_p-1\right) F(s,x,y)^2 \left(4 s (y-1) y (15 x y-2) F(s,x,y)+(9 x y-3) F(s,x,y)^2+36 s^2 x (y-1)^2 y^3\right)\Bigg\},\\ \rho^{\langle\bar{s}\sigma\cdot Gs\rangle}_{10;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 x^2}{512 \pi ^3 (y-1)^3} m_c \left(c_p-1\right) (x y-1) F(s,x,y) \left(s (y-1) y (5 x y-2) F(s,x,y)+(x y-1)\right.\\ & \left. F(s,x,y)^2+2 s^2 x (y-1)^2 y^3\right)\Bigg\},\\ \rho^{m_s\langle\bar{s}\sigma\cdot Gs\rangle}_{10;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 x}{768 \pi ^3 (y-1)^2} \left(c_p-1\right) \left(2 s x (y-1) y^2 F(s,x,y) \left(s (x-1) (y-1) y (11 x y-3)-3 m_c^2\right)\right.\\ & +F(s,x,y)^2 \left(m_c^2 (3-3 x y)+4 s (x-1) x (y-1) y^2 (7 x y-4)\right)+4 (x-1) x y (x y-1) F(s,x,y)^3+2 s^3 (x-1) x^2\\ & \left. (y-1)^3 y^5\right)\Bigg\},\\ \rho^{\langle\bar{s}s\bar{s}s\rangle}_{10;A(S)}(s) =& 0,\\ \rho^{\langle\bar{s}s\rangle\langle\bar{s}\sigma\cdot Gs\rangle}_{10;A(S)}(s) =& 0,\\ \rho^{\langle g_sG^2\rangle\langle \bar{s}s\rangle}_{10;A(S)}(s) =& 0,\\ \rho^{m_s\langle g_sG^2\rangle\langle \bar{s}s\rangle}_{10;A(S)}(s) =& 0,\\ \rho^{\langle g_sG^2\rangle^2}_{10;A(S)}(s) = &-\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{c_1 x^5 y^4 m_c^4 \left(c_p-1\right) (x y-1)}{1105920 \pi ^5 (x-1)^2 (y-1)^2}-\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\frac{i c_1 s x^5 y^5 m_c^4 \left(c_p-1\right) (7 x y-4)}{3317760 \pi ^5 (x-1)^2 (y-1)},\\ \rho^{\langle g_sG^2\rangle\langle \bar{s}\sigma\cdot Gs\rangle}_{10;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 x^2}{6144 \pi ^3 (x-1)^3 (y-1)^3} m_c \left(c_p-1\right) (y (y (x ((x-3) x+3) y-3)+3)-1) \left(3 (x-1) (x y\right.\\ & \left.-1) F(s,x,y)+m_c^2 (x y-1)^2+s (x-1) (y-1) y (5 x y-2)\right)\Bigg\}+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{\frac{c_1 s x^2 y}{18432 \pi ^3 (x-1)^3 (y-1)^2} m_c\\ & \left(c_p-1\right) (y (y (x ((x-3) x+3) y-3)+3)-1) \left(m_c^2 (5 x y-2) (x y-1)+3 s (x-1) x (y-1) y^2\right)\Bigg\},\\ \rho^{m_s\langle g_sG^2\rangle\langle \bar{s}\sigma\cdot Gs\rangle}_{5,11;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 x}{9216 \pi ^3 (x-1)^2 (y-1)^2} m_c^2 \left(c_p-1\right) (x y-1) \left(y \left(x \left(y \Big(x \left(4 ((x-3) x+3) y^2-3\right)-12 y\right.\right.\right.\\ & \left.\left.\left.+18\Big)-4\right)-6 y+6\right)-3\right)\Bigg\}+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{\frac{c_1 x}{27648 \pi ^3 (x-1)^3 (y-1)^2} m_c^2 \left(c_p-1\right) \left(s (x-1) x (y-1) y^2\right.\\ & \left(x y \left(y \left(28 ((x-3) x+3) x y^2-4 (4 (x-3) x+33) y-9 x+102\right)-28\right)+30 (y-1) y+7\right)-3 m_c^2 (x y-1)^2\\ & \left.(y (((x-3) x+3) y+x-3)+1)\right)\Bigg\},\\ \end{aligned} $

(27) where the coefficient
$ c_p=-1 $ . The spectral functions for the$ (0,2\{2,0\}) $ structure are given as$ \begin{aligned}[b] \\[-4pt]\rho^{pert}_{5,11;A(S)}(s) = &-\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{c_1 x^3}{6451200 \pi ^5 (y-1)^5} F(s,x,y)^3 \left(15 (y-1) F(s,x,y) \left(7 s x y m_c m_s (x y-1) \left(x y \left(11 x y \left(c_p+6\right)\right.\right.\right.\right.\\ & \left.\left.+4 c_p+99\right)-25\right)+14 m_c^2 m_s^2 \left(x y \left(x y \left(c_p+6\right)-15\right)+10\right)-s^2 (x-1) x^2 (y-1) y^3 \left(x y \left(25 x y \left(3 c_p+10\right)+28\right.\right.\\ & \left.\left.\left.\left(c_p+13\right)\right)-63\right)\right)+21 x F(s,x,y)^2 \left(m_c m_s (x y-1) \left(x y \left(4 x y \left(c_p+6\right)+2 c_p+57\right)-35\right)-2 s (x-1) x (y-1) y^2\right.\\ & \left.\left(5 x^2 y^2 \left(3 c_p+10\right)+x y \left(11 c_p+78\right)-2 \left(c_p+12\right)\right)\right)-(x-1) x^2 y \left(10 x^2 y^2 \left(3 c_p+10\right)+28 x y \left(c_p+8\right)\right.\\ \end{aligned} $

$ \begin{aligned}[b]& \left.-7 \left(c_p+18\right)\right) F(s,x,y)^3+60 s x (y-1)^2 y^2 \left(7 s x y m_c m_s (x y-1) \left(2 x y \left(c_p+6\right)+15\right)+14 m_c^2 m_s^2 \left(2 x y \left(c_p+6\right)\right.\right.\\ & \left.\left.\left.-15\right)-2 s^2 (x-1) x^2 (y-1) y^3 \left(x y \left(3 c_p+10\right)+14\right)\right)\right),\\ \rho^{\langle\bar{s}s\rangle}_{5,11;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{c_1 x^3}{384 \pi ^3 (y-1)^4} m_c (x y-1) F(s,x,y)^2 \Big(s (y-1) y (x y (11 x y+14)-3) F(s,x,y)+(x y (x y+2)\\ & -1) F(s,x,y)^2+6 s^2 x (y-1)^2 y^3 (x y+1)\Big),\\ \rho^{\langle m_s\bar{s}s\rangle}_{5,11;A(S)}(s) =& -\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\frac{c_1 x^2}{384 \pi ^3 (y-1)^3} F(s,x,y) \left(-3 s x (y-1) y^2 F(s,x,y) \left(4 m_c^2 (x y-1)+s (x-1) (y-1) y (x y (25\right.\right.\\ & \left.x y+26)-3)\right)-2 F(s,x,y)^2 \left(m_c^2 (x y-1)^2+s (x-1) x (y-1) y^2 (x y (35 x y+39)-8)\right)-(x-1) x y (x y (5 x y+8)\\ & \left.-3) F(s,x,y)^3-12 s^3 (x-1) x^2 (y-1)^3 y^5 (x y+1)\right),\\ \rho^{\langle g_sG^2\rangle}_{5,11;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1 x^3}{7741440 \pi ^5 (x-1)^3 (y-1)^5} m_c \left(-6 (y-1) F(s,x,y) \left(-21 s^2 (x-1) x^2 (y-1) y^3 m_s (y (y (x\right.\right.\\ & ((x-3) x+3) y-3)+3)-1) \left(2 x y \left(c_p+6\right)+15\right)+s (x-1) x (y-1) y^2 m_c \left(s x y (y (y (x ((x-3) x+3) y-3)\right.\\ & \left.+3)-1) \left(x y \left(25 x y \left(3 c_p+10\right)+28 \left(c_p+13\right)\right)-63\right)-42 m_s^2 (y (((x-2) x+2) y-2)+1) \left(2 x y \left(c_p+6\right)-15\right)\right)\\ & -7 s x y m_c^2 m_s (x y-1)^2 (y (((x-3) x+3) y+x-3)+1) \left(x y \left(11 x y \left(c_p+6\right)+4 c_p+99\right)-25\right)-14 m_c^3 m_s^2 (y (y (x\\ & \left.((x-3) x+3) y-3)+3)-1) \left(x y \left(x y \left(c_p+6\right)-15\right)+10\right)\right)+(x-1) x (y (y (x ((x-3) x+3) y-3)+3)-1)\\& F(s,x,y)^3 \left(21 m_s \left(x y \left(4 x y \left(c_p+6\right)+2 c_p+57\right)-35\right)+2 x y m_c \left(-10 x^2 y^2 \left(3 c_p+10\right)-28 x y \left(c_p+8\right)\right.\right.\\ & \left.\left.+7 \left(c_p+18\right)\right)\right)+21 F(s,x,y)^2 \left(2 (x-1) (y-1) m_c \left(3 m_s^2 (y (((x-2) x+2) y-2)+1) \Big(x y \left(x y \left(c_p+6\right)-15\right)\right.\right.\\ & \left.+10\Big)-s x^2 y^2 (y (y (x ((x-3) x+3) y-3)+3)-1) \left(5 x^2 y^2 \left(3 c_p+10\right)+x y \left(11 c_p+78\right)-2 \left(c_p+12\right)\right)\right)\\& +x m_c^2 m_s (x y-1)^2 (y (((x-3) x+3) y+x-3)+1) \left(x y \left(4 x y \left(c_p+6\right)+2 c_p+57\right)-35\right)+3 s (x-1) x (y-1) y\\ & \left. m_s (y (y (x ((x-3) x+3) y-3)+3)-1) \left(x y \left(11 x y \left(c_p+6\right)+4 c_p+99\right)-25\right)\right)+6 s x (y-1)^2 y^2 m_c (y (y (x ((x\\ & -3) x+3) y-3)+3)-1) \left(7 s x y m_c m_s (x y-1) \left(2 x y \left(c_p+6\right)+15\right)+14 m_c^2 m_s^2 \left(2 x y \left(c_p+6\right)-15\right)-2 s^2\right.\\ & \left.\left.(x-1) x^2 (y-1) y^3 \left(x y \left(3 c_p+10\right)+14\right)\right)\right)\Bigg\},\\ \rho^{\langle\bar{s}\sigma\cdot Gs\rangle}_{5,11;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 x^2}{1536 \pi ^3 (y-1)^3} m_c (x y-1) F(s,x,y) \Big(3 s (y-1) y (x y(55 x y+56)-9) F(s,x,y)+4 (x y\\ & (5 x y+8)-3) F(s,x,y)^2+12 s^2 x (y-1)^2 y^3 (5 x y+4)\Big)+\frac{c_2 x^3}{1024 \pi ^3 (x-1) (y-1)^4} m_c (y (((x-2) x+2) y-2)\\ & +1) F(s,x,y) \Big(3 s (y-1) y (x y (11 x y+14)-3) F(s,x,y)+4 (x y (x y+2)-1) F(s,x,y)^2+12 s^2 x (y-1)^2 y^3\\ & (x y+1)\Big)\Bigg\},\\ \rho^{m_s\langle\bar{s}\sigma\cdot Gs\rangle}_{5,11;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{-\frac{c_1 x}{1152 \pi ^3 (y-1)^2} \left(-3 s x (y-1) y^2 F(s,x,y) \left(6 m_c^2 (4 x y-3)+s (x-1) (y-1) y \left(x y \left(-4 c_p\right.\right.\right.\right.\\& +125 x y+104\Big)-9\Big)\Big)-3 F(s,x,y)^2 \left(3 m_c^2 (x y-1) (2 x y-1)+s (x-1) x (y-1) y^2 \left(c_p (2-11 x y)+x y (175 x y\right.\right.\\ & \left.\left.+156)-24\right)\right)-(x-1) x y \left(-4 x y c_p+c_p+2 x y (25 x y+32)-18\right) F(s,x,y)^3-6 s^3 (x-1) x^2 (y-1)^3 y^5 (5 x y\\ & +4)\Big)+\frac{c_2 x^2 (x y-1)}{1024 \pi ^3 (x-1) (y-1)^3} \left(3 s x (y-1) y^2 F(s,x,y) \left(4 m_c^2 (x y-1)+s (x-1) (y-1) y (x y (25 x y+26)-3)\right)\right.\\ & +3 F(s,x,y)^2 \left(m_c^2 (x y-1)^2+s (x-1) x (y-1) y^2 (x y (35 x y+39)-8)\right)+2 (x-1) x y (x y (5 x y+8)-3)\\ & \left.F(s,x,y)^3+6 s^3 (x-1) x^2 (y-1)^3 y^5 (x y+1)\right)\Bigg\},\\ \end{aligned} $

$ \begin{aligned}[b]\rho^{\langle\bar{s}s\bar{s}s\rangle}_{5,11;A(S)}(s) =& 0,\\ \rho^{\langle\bar{s}s\rangle\langle\bar{s}\sigma\cdot Gs\rangle}_{5,11;A(S)}(s) =& 0,\\ \rho^{\langle g_sG^2\rangle\langle \bar{s}s\rangle}_{5,11;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 x^3}{4608 \pi ^3 (x-1)^3 (y-1)^4} m_c (y (y (x ((x-3) x+3) y-3)+3)-1) \left(F(s,x,y) \left(4 m_c^2 (x y (x y\right.\right.\\ & \left.+2)-1) (x y-1)+3 s (x-1) (y-1) y (x y (11 x y+14)-3)\right)+6 (x-1) (x y (x y+2)-1) F(s,x,y)^2+s (y-1) y\\& \left.\left(m_c^2 (x y (11 x y+14)-3) (x y-1)+6 s (x-1) x (y-1) y^2 (x y+1)\right)\right)\Bigg\}+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\frac{c_1 s^2 x^4 y^3}{2304 \pi ^3 (x-1)^3 (y-1)^2}\\ & m_c^3 \left(x^2 y^2-1\right) (y (y (x ((x-3) x+3) y-3)+3)-1),\\ \rho^{m_s\langle g_sG^2\rangle\langle \bar{s}s\rangle}_{5,11;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 x^2}{2304 \pi ^3 (x-1)^3 (y-1)^3} m_c^2 (x y-1) \left((x-1) \Big(y \Big(x \Big(y \Big(x \Big(y \Big(x \Big(10 ((x-3) x+3) y^2+26 (x\right.\\ & -3) y+23\Big)+48 y-36\Big)+7\Big)-12 y+18\Big)-3\Big)-6 y+6\Big)-3\Big) F(s,x,y)+m_c^2 (x y-1)^2 (y (((x-3) x+3) y+x\\ & -3)+1)+s (x-1) x (y-1) y^2 \left(x y \left(y \left(35 ((x-3) x+3) x y^2+(74 (x-3) x+117) y+72 x-105\right)+31\right)-12 (y\right.\\ & \left.-1) y-2\right)\Big)\Bigg\}+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{\frac{c_1 s x^3 y^2}{4608 \pi ^3 (x-1)^3 (y-1)^2} m_c^2 (x y-1) (y (((x-3) x+3) y+x-3)+1) \Big(4 m_c^2 (x y\\& -1)+s (x-1) (y-1) y (x y (25 x y+26)-3)\Big)\Bigg\},\\ \rho^{\langle g_sG^2\rangle^2}_{5,11;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 x^5 y^4}{46448640 \pi ^5 (x-1)^2 (y-1)^2}m_c^4 \left(c_p (2 x y (15 x y+14)-7)+4 x y (25 x y+56)-126\right)\Bigg\}\\ & +\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{\frac{c_1 s x^5 y^5}{6635520 \pi ^5 (x-1)^2 (y-1)} m_c^4 \left(c_p (x y (15 x y+11)-2)+2 x y (25 x y+39)-24\right)\Bigg\},\\ \rho^{\langle g_sG^2\rangle\langle \bar{s}\sigma\cdot Gs\rangle}_{5,11;A(S)}(s) = &-\int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 x^2}{18432 \pi ^3 (x-1)^3 (y-1)^3} m_c (y (y (x ((x-3) x+3) y-3)+3)-1) \Big(12 (x-1) (x y (5 x y\\ & +8)-3) F(s,x,y)+4 m_c^2 (x y (5 x y+8)-3) (x y-1)+3 s (x-1) (y-1) y (x y (55 x y+56)-9)\Big)\\ & +\frac{c_2 x^3 y^2}{3072 \pi ^3 (x-1)^2 (y-1)^2} m_c^3 (x y-1) (x y (x y+2)-1)\Bigg\}+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{\frac{c_1 s x^2 y}{18432 \pi ^3 (x-1)^3 (y-1)^2} m_c (y (y (x\\ & ((x-3) x+3) y-3)+3)-1) \Big(m_c^2 (x y (55 x y+56)-9)(x y-1)+6 s (x-1) x (y-1) y^2 (5 x y+4)\Big)\\ & +\frac{c_2 s x^3 y^3}{12288 \pi ^3 (x-1)^2 (y-1)} m_c^3 (x y-1) (x y (11 x y+14)-3)\Bigg\},\\ \rho^{m_s\langle g_sG^2\rangle\langle \bar{s}\sigma\cdot Gs\rangle}_{5,11;A(S)}(s) =& \int^{x_{\max}}_{0}{\rm d}x\int^{y_{\max}}_{y_{\min}}{\rm d}y\Bigg\{\frac{c_1 x}{13824 \pi ^3 (x-1)^2 (y-1)^2} m_c^2 (x y-1) \left(y \left(-x c_p (4 x y-1) (y (((x-3) x+3) y+x-3)+1)\right.\right.\\ & \left.\left.+y \left(x \left(x \left(50 ((x-3) x+3) x y^3+6 (19 (x-3) x+32) y^2+6 (19 x-29) y+37\right)-18 (y-2)\right)-18\right)+18\right)-9\right)\\ & +\frac{c_2 x^2 y }{12288 \pi ^3 (x-1)^2 (y-1)^2}m_c^2 (x y (x y (x y (2 y (5 ((x-2) x+2) y+8 x-26)+7)+4 (8 y-5) y+7)-12 (y-1) y\\ & +3)-3)\Bigg\}+\int^{1}_{0}{\rm d}x\int^{1}_{0}{\rm d}y\Bigg\{\frac{c_1 x}{13824 \pi ^3 (x-1)^3 (y-1)^2} m_c^2 \left(3 m_c^2 (x y-1)^2 (2 x y-1) (y (((x-3) x+3) y+x-3)\right.\\ & +1)+s (x-1) x (y-1) y^2 \Big(-c_p (x y-1) (11 x y-2) (y (((x-3) x+3) y+x-3)+1)+x y \Big(y \Big(x \Big(y \Big(x \Big(175 ((x\\ & \left.-3) x+3) y^2+156 (x-3) y+12\Big)-57 y+525\Big)-202\Big)-468 y+450\Big)-120\Big)+18 (y-1) y-3\Big)\right)\\ & +\frac{c_2 x^2 y}{12288 \pi ^3 (x-1)^3 (y-1)^2} m_c^2 \Big(m_c^2 (x y-1)^2 (y (((x-2) x+2) y-2)+1)+s (x-1) x (y-1) y^2 (x y (y (x (y (35 ((x\\ & -2) x+2) y+39 x-148)+33)+78 y-62)+27)-16 (y-1) y-2)\Big)\Bigg\},\\ \end{aligned} $

(28) where the coefficient
$ c_p=1 $ for current$ J_{5,\mu\nu}^{A(S)} $ and$ c_p=-1 $ for current$ J_{11,\mu\nu}^{A(S)} $ .
D-wave excited ${\boldsymbol{ cs}\bar{\boldsymbol c}\bar{\boldsymbol s}} $ tetraquark states with ${ \boldsymbol{J^{PC}}=\bf 1^{\bf ++}} $ and ${\bf 1^{\bf +-}} $
- Received Date: 2022-12-28
- Available Online: 2023-05-15
Abstract: We study the mass spectra of D-wave excited









 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF
















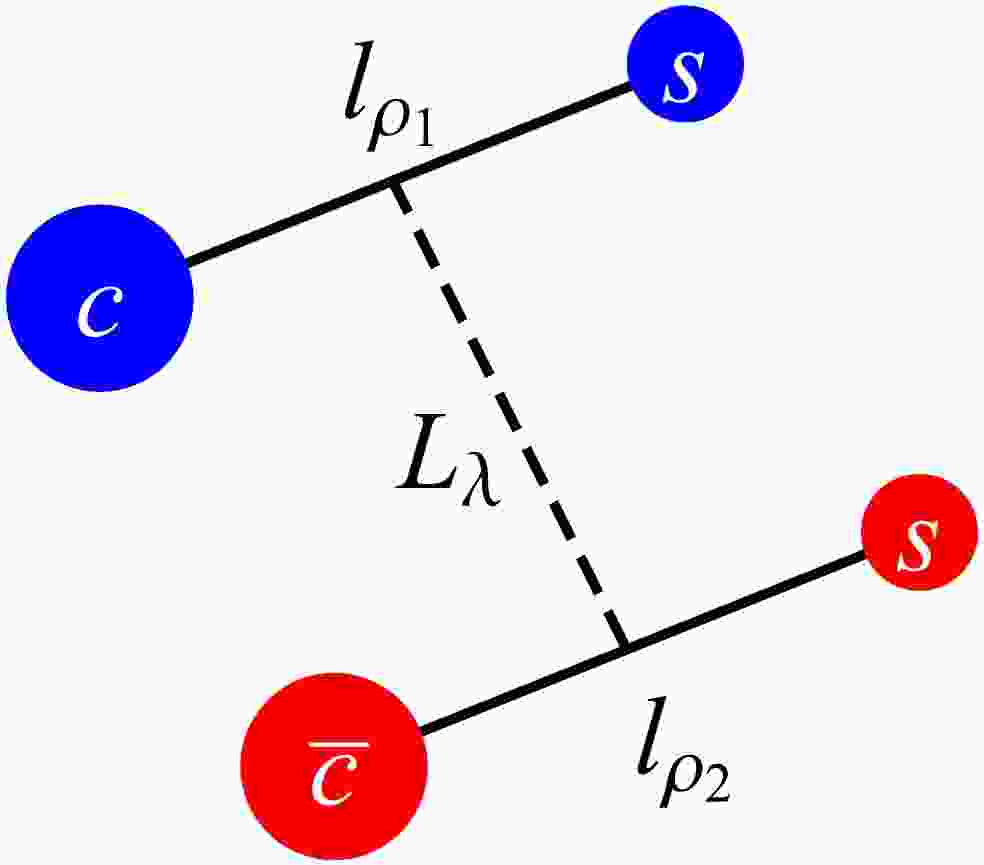














 DownLoad:
DownLoad: