-
α decay is a significant decay process of unstable nuclei and was observed in the early days of nuclear physics. In 1896, Becquerel first observed this process as an unknown radiation. It was not defined until 1899 [1]. Subsequently, Rutherford explained it as the process of a parent nucleus releasing a
$ ^{4}{\rm{He}} $ particle. In 1928, Gamow [2] and Condon and Gurney [3] independently explained α decay as a quantum tunneling process. Since then, α decay has been a popular topic in nuclear physics [4–29]. It is often studied to investigate nuclear forces, obtain information of nuclear structure including shell effects [22, 23], low-lying states [24], nuclear shape coexistences [25, 26], ground states [8], and energy levels [4] and particularly identify new superheavy elements and/or isotopes through the observation of α decay chains [14, 18, 27–29].With the advancement of experimental technology, significant progress have been achieved in the experimental [30–36] and theoretical [37–66] fields for α decay. Experimentally, in recent years, a series of superheavy elements and/or isotopes have been successfully synthesized through warm, hot, and cold fusion reactions. For instance, in 2011, the superheavy element
$ Z = 118 $ was successfully synthesized by conducting$ ^{48}{\rm{Ca}} $ -induced hot fusion reactions using the actinide element$ {\rm{Cf}} $ as the target material [36]. More recently, a new α-emitting isotope,$ ^{214}{\rm{U}} $ , was successfully identified in the fusion-evaporation reaction of$ ^{182}{\rm{W}} $ ($ ^{36}{\rm{Ar}} $ , 4n) [34]. Theoretically, multiple models have been proposed to study α decay, including effective liquid drop model [38, 39], fission-like model [52], generalized liquid drop model (GLDM) [48, 49], density dependent M3Y (DDM3Y) effective interaction [43, 44], and several others [58–61]. Furthermore, many empirical formulas have been adopted to investigate α decay based on the Geiger-Nuttall (G-N) law and/or the quantum tunneling effect [67], such as the universal decay law (UDL) [68, 69], Viola-Seaborg-Sobiczewski (VSS) formula [70], Royer formula [45], Hatsukawa formula [71], Deng-Zhang-Royer formula (DUR) [72], Sobiczewski-Parkhomenko (SP) formula [73], and so forth [74–82].In 2020, Bayrak proposed a phenomenological harmonic oscillator potential model (HOPM) [83] based on the Wentzel-Kramers-Brillouin (WKB) method and Bohr-Sommerfeld quantization condition. With this model, taking the α preformation probability
$ P_{\alpha} $ as a constant and obtaining the depth parameter of nuclear potential$ V_0 $ by fitting the experimental data for the even-even nuclei of nuclear property table NUBASE2016 [84], the favored α decay half-lives of 263 nuclei including 136 even-even, 48 even-odd, 49 odd-even, and 30 odd-odd nuclei were calculated. Considering the increasing volume of experimental data and taking the preformation probabilities$ P_{\alpha} $ from Xu and Ren [77], which were derived by fitting experimental α decay half-lives, based on the HOPM, we obtain the parameter$ V_0=162.6 $ MeV by refitting 178 α decay half-lives of even-even nuclei obtained from the latest nuclear property table NUBASE2020 [85] with a corresponding root-mean-square (rms) deviation of$ \sigma=0.322 $ . However, when applying the HOPM to calculate unfavored α decay half-lives, the emitted α particle carries a non-zero orbital angular momentum, i.e.,$ l \neq 0 $ , which means that the effect of centrifugal potential is introduced in the total interaction potential between the emitted α particle and the daughter nucleus. In favored α decay, the orbital angular momentum carried away by the emitted α particle is$ l=0 $ ; thus, the centrifugal potential is equal to zero. Under this condition, an analytical expression can be obtained for the logarithmic form of favored α decay half-lives with only one parameter. To extend the applicability of the analytical expression within the HOPM framework to consider the contribution of the centrifugal potential, adding a new term$ d\sqrt{l(l+1)} $ (d is the adjustable parameter) to the logarithmic form of favored α decay half-lives, we generalize this model to unfavored α decay and propose an improved simple model (ISM) for calculating favored and unfavored α decay half-lives. Fitting the experimental data of 205 unfavored α decay half-lives, we obtain$ d=0.381 $ . Compared with the HOPM, the rms deviations of 128 unfavored odd-A and 77 unfavored odd-odd nuclei through the ISM are reduced from 1.292 and 1.339 to 0.592 and 0.621, respectively. Meanwhile, the rms deviation for all 693 nuclei is only 0.452. In addition, we further extend the ISM to predicting the α decay half-lives of 144 even-even, odd-A, and odd-odd nuclei with$ Z = 117,118,119, $ and$ 120 $ . For comparison, the DUR and MUDL are also used. The corresponding predictions of these formulas are mostly consistent.The remainder of this article is organized as follows. Section II briefly introduces the theoretical framework. Detailed numerical results and discussion are given in Section III. Finally, a summary is provided in Section IV.
-
The α decay half-life
$ T_{1/2} $ is related to the decay width Γ as$ T_{1/2}=\frac{\hbar \,{\rm ln}2}{\Gamma}, $

(1) where
$ \hbar $ is the reduced Planck constant. Γ is the α-decay width including the α particle preformation probability$ P_\alpha $ , the normalization factor F, and the penetration probability P. In the HOPM, it can be expressed as [57]$ \Gamma=P_{\alpha} F \frac{\hbar^2}{4\,\mu}P, $

(2) where
$\mu={m_d m_{\alpha}}/(m_d +m_{\alpha})$ is the reduced mass of the α particle and daughter nucleus in the center-of-mass system with$ m_d $ and$ m_{\alpha} $ being the masses of the daughter nucleus and α particle, respectively, obtained from NUBASE2020 [85]. Owing to the complexity of nuclear potential and the many-body problem in nuclear physics,$ P_{\alpha} $ is a quantity that is challenging to ascertain. Referring the study of Xu and Ren [77], in this paper, the probability of α particle preformation is set as$ P_\alpha=0.43 $ for even-even nuclei,$ P_\alpha=0.35 $ for odd-A nuclei, and$ P_\alpha=0.18 $ for odd-odd nuclei.The normalization factor F, describing the assault frequency or the collision probability of the α particle, can be expressed as
$ F\, \int_{0}^{r_1}\frac{1}{2\,k(r)}{\rm d} r=1. $

(3) $P={\rm e}^{-2S}$ , the barrier penetration probability of the α particle, is calculated using the semi-classical WKB approximation. S denotes the action integral. It is given by [83]$ S=\int_{r_1}^{r_2}k(r)\,{\rm d}r, $

(4) where
$ k(r)=\sqrt{\dfrac{2\mu}{\hbar^2}(V(r)-Q_{\alpha})} $ is the wave number of the α particle, and r is the center-of-mass distance between the preformed α particle and the daughter nucleus.$ Q_{\alpha} $ and$ V(r) $ denote the α decay energy and total interaction potential between the emitted α particle and daughter nucleus, respectively.$ r_1 $ and$ r_2 $ denote the classical turning points, which satisfy the conditions$V(r_1)=V(r_2)= Q_{\alpha}$ .$ Q_{\alpha} $ is calculated using$ Q_{\alpha}=M(A,Z)-M(A-4,Z-2)-M(^{4}{\rm He}), $

(5) where
$ M(A,Z) $ ,$ M(A-4,Z-2) $ , and$M(^{4}{\rm He}$ ) represent the mass excesses of the parent nucleus, daughter nucleus, and α particle, respectively. The total interaction potential$ V(r) $ is expressed as$ V(r)=V_N(r)+V_C(r)+V_l(r), $

(6) where
$ V_N(r) $ is the nuclear potential. In this paper, it is selected as the modified harmonic oscillator form and can be expressed as [20, 83]$ V_N(r)=-V_0+V_1\,r^2, $

(7) where
$ V_1 $ and$ V_0 $ are the diffusivity and depth of nuclear potential, respectively.$ V_C $ , the Coulomb potential, is taken as the potential of a uniformly charged sphere with sharp radius R. It is expressed as$ V_C(r) = \left\{\begin{array}{*{20}{l}} {\dfrac{Z_{\alpha} Z_d e^2}{2R}\left(3-\dfrac{r^2}{R^2}\right), }& {r\leq r_1, }\\ {\dfrac{Z_{\alpha Z_d} e^2}{r},} & {r> r_1,} \end{array}\right. $

(8) where
$ e^{2}=1.4399652 $ MeV$ \cdot $ fm represents the square of the electronic elementary charge.$ Z_d $ and$ Z_{\alpha} $ are the proton numbers of the daughter nucleus and preformed α particle, respectively. The sharp radius R is calculated using the empirical radius formula$R=1.28A_d^{1/3}- 0.76+ 0.8A_d^{-1/3}$ , where$ A_d $ is the mass numbers of the daughter nucleus [45].$ V_l $ is the centrifugal potential and can be expressed as$ V_l(r)=\frac{\hbar^2\,l(l+1)}{2\mu r^2}, $

(9) where l denotes the orbital angular momentum carried away by the emitted α particle. For favored α decay, the total interaction potential
$ V(r) $ can be expressed as [83]$ V(r) = \left\{\begin{array}{*{20}{l}} {C_0-V_0+(V_1-C_1)r^2,} & {r\leq r_1, }\\ {\dfrac{C_2}{r},} & {r> r_1, }\end{array}\right. $

(10) where
$ C_0=\dfrac{3Z_d\,Z_{\alpha}\,e^2}{2R} $ ,$ C_1=\dfrac{Z_d\,Z_{\alpha}\,e^2}{2R^3} $ , and$ C_2=Z_d\,Z_{\alpha}e^2 $ . Using$ ^{148}{\rm{Sm}} \to ^{144}{\rm{Nd}} + ^{4}{\rm{He}} $ as an example, the schematic diagram of the total interaction potential$ V(r) $ is shown in Fig. 1. Using the conditions$ V(r_1)=V(r_2)=Q_{\alpha} $ , we obtain$ r_1=\sqrt{\dfrac{Q_{\alpha}+V_0-C_0}{V_1-C_1}} $ and$ r_2=\dfrac{C_2}{Q_{\alpha}} $ .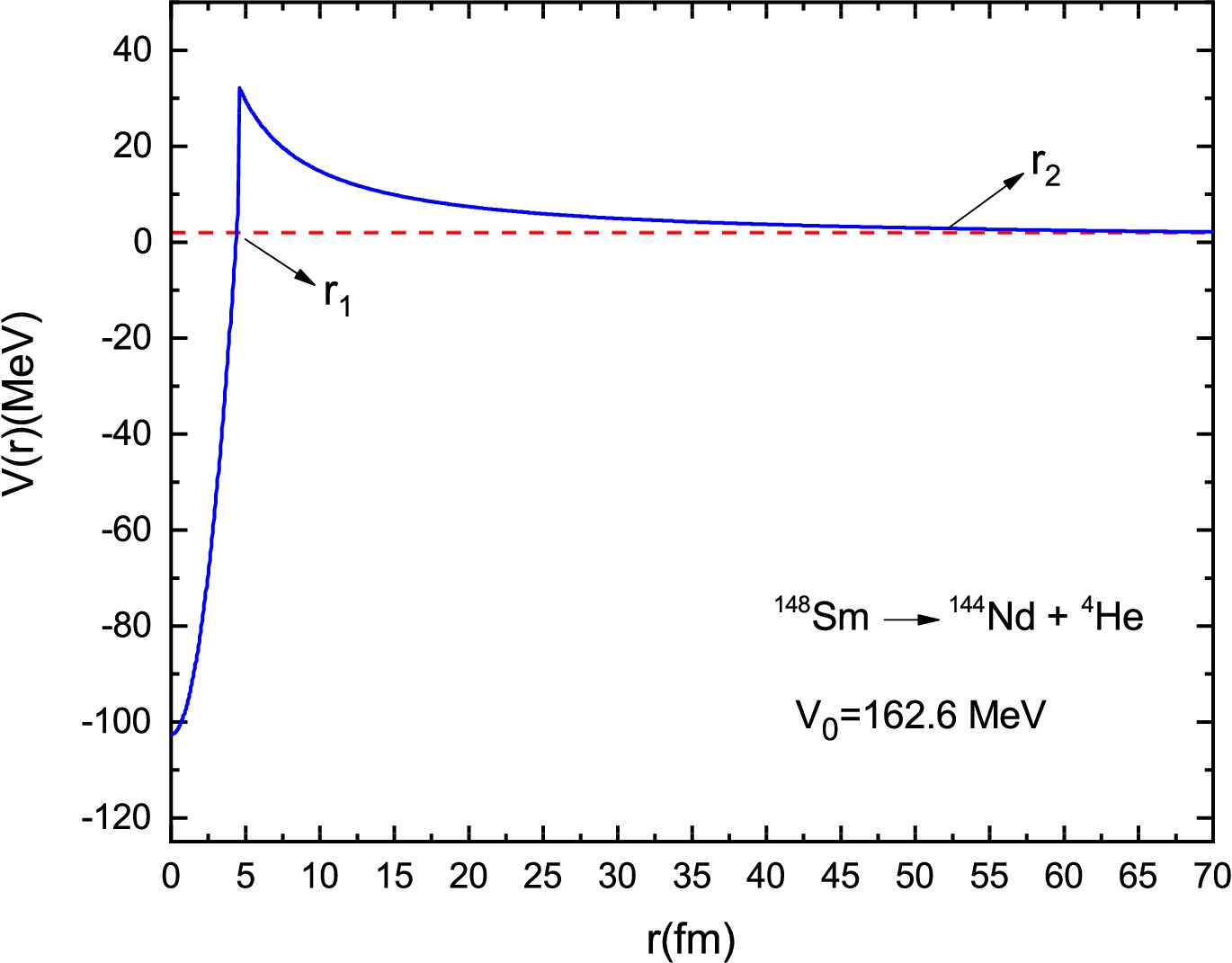
Figure 1. (color online) Variation in the total interaction potential
$ V(r) $ for the parent nucleus$ ^{148}{\rm{Sm}} $ as a function of r.The Bohr-Sommerfeld quantization condition, a crucial component of the WKB approximation, is employed to reduce the system's degrees of freedom. It is expressed as
$ \int_{0}^{r_1}\sqrt{\frac{2\mu}{\hbar^2}(V(r)-Q_{\alpha})}{\rm d}r=(G_{\alpha}-l+1)\frac{\pi}{2}, $

(11) where
$ G_{\alpha} = 2n_r + l $ is the main quantum number of α decay, with$ n_r $ and l being the radial and angular momentum quantum numbers, respectively. It is given by [77]$ G_\alpha = \left\{\begin{array}{*{20}{l}} {20,} & {N> 126,} \\ {18,} & {82 < N \leq 126,} \\ {16,} & {N \leq 82,} \end{array}\right. $

(12) where N is the neutron number of the parent nucleus.
Through the integral operation of Eq. (11), the analytical expression between
$ V_0 $ and$ V_1 $ is obtained as follows:$ V_1=C_1+\frac{\mu}{2\hbar^2}\bigg(\frac{Q_{\alpha}+V_0-C_0}{1+G_{\alpha}}\bigg)^2, $

(13) where
$ C_0<(Q_{\alpha}+V_0) $ and$ C_1< V_1 $ . Using Eq. (13), the normalization factor F and action integral S can be analytical expressed as$ F=\frac{4}{\pi}\,\frac{\mu}{\hbar^2}\bigg(\frac{Q_{\alpha}+V_0-C_0}{1+G_{\alpha}}\bigg), $

(14) $ S=\frac{\sqrt{2\mu}}{\hbar}\frac{C_2}{\sqrt{Q_{\alpha}}}\bigg [{\rm{arccos}}\bigg (\sqrt{\frac{Q_{\alpha}r_1}{C_2}}\bigg) - \sqrt{\frac{Q_{\alpha}r_1}{C_2} - \bigg (\frac{Q_{\alpha}r_1}{C_2}\bigg)^2}\bigg]. $

(15) Subsequently, the logarithmic form of favored α decay half-lives can be expressed as
$ {{\rm{log}}}_{10}T_{1/2}= {{\rm{log}}}_{10}\bigg(\frac{\pi\, \hbar \,ln2}{P_{\alpha}}\frac{1+G_{\alpha}}{Q_{\alpha}+V_0-C_0}\bigg)+2\,S\,{{\rm{log}}}_{10}(e). $

(16) Similar to the unfavored α decay, the emitted α particle carries a non-zero orbital angular momentum (
$ l \neq 0 $ ); thus, the effect of centrifugal potential must be considered in the total interaction potential, which means that the total barrier is higher, resulting in a reduced penetration probability and consequently a longer α decay half-life. Under this condition, this effect must be considered for unfavored α decay. Generally, two methods can be used to solve this problem: directly adding the terms of orbital angular momentum$ d\,l(l+1) $ [61, 72, 78] or$ d\,\sqrt{l(l+1)} $ [74] to the corresponding models or empirical formulas. In this paper, if$ l \neq 0 $ , the wave number$ k(r) $ under integration can not be determined using analytical solutions in the HOPM framework, then Eqs. (4), (11), and (14) can not be calculated analytically. Consequently, to generalize the applicability of the analytic expression within the framework of HOPM to study unfavored α decay half-lives, we have augmented the original analytic Eq. (16) with an additional term$ d\sqrt{l(l+1)} $ , thereby proposing the ISM for calculating α decay half-lives. It can be expressed as$ \begin{aligned}[b] {{\rm{log}}}_{10}T_{1/2}=\;& {{\rm{log}}}_{10}\bigg( \frac{\pi\, \hbar \,ln2}{P_{\alpha}}\frac{1+G_{\alpha}}{Q_{\alpha}+V_0-C_0}\bigg)\\&+2{{\rm{log}}}_{10}(e)S + d\sqrt{l(l+1)}. \end{aligned} $

(17) -
In 2020, Deng et al. presented an unitary Royer formula (DUR) for α decay half-lives. It can be expressed as [72]
$ {{\rm{log}}}_{10}T_{1/2}= a+bA^{1/6}\sqrt{Z}+cZ/\sqrt{Q_{\alpha}}+dl(l+1)+h, $

(18) where A and Z are the mass and proton numbers of the parent nucleus, respectively. The adjustable parameters are
$ a=-26.8125 $ ,$ b=-1.1255 $ ,$ c=1.6057 $ , and$d= 0.0513$ , respectively. h is given by$ h = \left\{\begin{array}{*{20}{l}} {0,} & {\rm{ for\,\, even-even \,\,nuclei,} }\\ {0.2812,} &{\rm{for \, \,odd\,\, {\it Z}-even \,\,{\it N} \,\,nuclei,}} \\{ 0.3625,} & {\rm{for \,\,even\,\, {\it Z}-odd \,\,{\it N} \,\,nuclei,}}\\ {0.7486,} & {\rm{for \,\,odd-odd \,\,nuclei.}} \end{array}\right. $

(19) -
In 2021, considering the effects of the orbital angular momentum and isospin, Soylu et al. modified the UDL formula for α decay and cluster radioactivity (MUDL). It can be expressed as [74]
$ \begin{aligned}[b]{{\rm{log}}}_{10}T_{1/2}=\;& aZ_{\alpha}Z_d\sqrt{\mathcal{A}/Q_{\alpha}}+b\sqrt{\mathcal{A}Z_{\alpha}Z_d(A_d^{1/3}+A_{\alpha}^{1/3})}\\ & +c+d\sqrt{\mathcal{A}Z_{\alpha}Z_d(A_d^{1/3}+A_{\alpha}^{1/3})}\sqrt{l(l+1)}, \end{aligned} $

(20) where
$ \mathcal{A}=A_{\alpha}A_d/(A_{\alpha}+A_d) $ , with$ A_{\alpha} $ and$ A_d $ being the mass numbers of the emitted α particle and the daughter nucleus, respectively. The adjustable parameters are$ a=0.4392 $ ,$ b=-0.3944 $ ,$ c=-27.0649 $ , and$ d=0.0052 $ , respectively. -
Based on WKB method and Bohr-Sommerfeld quantization condition, Bayrak proposed the HOPM to calculate the favored α decay half-lives of 263 nuclei. Considering the increase in experimental data and the effect of centrifugal potential, we generalize the HOPM to unfavored α decay and propose an ISM to calculate α decay half-lives. In this paper, to redetermine the only one adjustable parameter
$ V_0 $ in HOPM, we use a genetic algorithm with the optimal solution of the standard deviation σ (The detailed definition of σ is given in following) as the objective function to obtain it. Fitting experimental α decay half-lives of 178 even-even nuclei, which are obtained from the latest nuclear property table NUBASE2020, we obtain the ideal value as$ V_0=162.6 $ MeV. Using the obtained$ V_0 $ , based on Eq. (16), we first systemically calculate the favored α decay half-lives. The detail results for even-even, odd-A, and odd-odd nuclei are listed in Tables 2–4, respectively. These three tables share the same frameworks, the first three columns denote the α decay, experimental α decay energy, and minimum angular momentum carried away by the emitted α particle, respectively. The fourth and fifth columns represent the experimental α decay half-lives and those calculated using the HOPM in the logarithmic form, denoted as$ {\rm{log}}_{10}{T}_{1/2}^{\rm{\,exp}} $ and$ {\rm{log}}_{10}{T}_{1/2}^{\rm{\,HOPM}} $ , respectively. These three tables show that our calculated results agree closely with the experimental data for most nuclei, with some exceptions, such as$ ^{268}{\rm{Hs}} $ ,$ ^{282}{\rm{Ds}} $ ,$ ^{185}{\rm{Pt}} $ ,$ ^{197}{\rm{Bi^{m}}} $ ,$ ^{231}{\rm{Pa}} $ ,$ ^{225}{\rm{Np}} $ ,$ ^{263}{\rm{Hs^{m}}} $ ,$ ^{196}{\rm{At^{m}}} $ , and$ ^{198}{\rm{Fr^{m}}} $ . For$ ^{268}{\rm{Hs}} $ and$ ^{282}{\rm{Ds}} $ , the deformation around the region with$ N=184 $ and$ Z=108 $ may result in large deviations for these two nuclei [78, 88, 89]. For$ ^{197}{\rm{Bi^{m}}} $ ,$ ^{263}{\rm{Hs^{m}}} $ ,$ ^{196}{\rm{At^{m}}} $ , and$ ^{198}{\rm{Fr^{m}}} $ in these excited state, the corresponding experimental data may have some uncertainty [90]. For a intuitive comparison, the differences between the experimental and calculated half-lives for even-even, odd-A, and odd-odd nuclei in the logarithmic form are plotted in Fig. 2. The figure clearly shows that the deviations between the experimental and calculated data are mostly within the range of$ \pm 0.5 $ . This means our calculated results can reproduce the experimental data well.α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{\,exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{\,HOPM}} $ 

146Sm $ \to {}^{142}{\rm{Nd}} $ 

2.5288 ± 0.0028 0 $ 15.3316 ^{+ 0.0426 }_{- 0.0472 } $ 

$ 15.3856 ^{+ 0.0346 }_{- 0.0446 } $ 

148Sm $ \to {}^{144}{\rm{Nd}} $ 

1.9870 ± 0.0004 0 $ 23.2984 ^{+ 0.0815 }_{- 0.1004 } $ 

$ 23.4501 ^{+ 0.0072 }_{- 0.0173 } $ 

148Gd $ \to {}^{144}{\rm{Sm}} $ 

3.2713 ± 0.0003 0 $ 9.3522 ^{+ 0.0060 }_{- 0.0061 } $ 

$ 9.2179 ^{+ 0.0025 }_{- 0.0127 } $ 

150Gd $ \to {}^{146}{\rm{Sm}} $ 

2.8070 ± 0.0060 0 $ 13.7519 ^{+ 0.0190 }_{- 0.0199 } $ 

$ 13.7319 ^{+ 0.0653 }_{- 0.0757 } $ 

152Gd $ \to {}^{148}{\rm{Sm}} $ 

2.2038 ± 0.0010 0 $ 21.5325 ^{+ 0.0310 }_{- 0.0334 } $ 

$ 21.6542 ^{+ 0.0158 }_{- 0.0262 } $ 

150Dy $ \to {}^{146}{\rm{Gd}} $ 

4.3513 ± 0.0015 0 $ 2.6337 ^{+ 0.0030 }_{- 0.0030 } $ 

$ 2.8945 ^{+ 0.0086 }_{- 0.0185 } $ 

152Dy $ \to {}^{148}{\rm{Gd}} $ 

3.7270 ± 0.0040 0 $ 6.9329 ^{+ 0.0036 }_{- 0.0037 } $ 

$ 6.9305 ^{+ 0.0290 }_{- 0.0392 } $ 

154Dy $ \to {}^{150}{\rm{Gd}} $ 

2.9450 ± 0.0050 0 $ 13.9762 ^{+ 0.1761 }_{- 0.3010 } $ 

$ 13.7609 ^{+ 0.0523 }_{- 0.0628 } $ 

152Er $ \to {}^{148}{\rm{Dy}} $ 

4.9343 ± 0.0016 0 $ 1.0567 ^{+ 0.0042 }_{- 0.0042 } $ 

$ 0.8946 ^{+ 0.0077 }_{- 0.0178 } $ 

154Er $ \to {}^{150}{\rm{Dy}} $ 

4.2797 ± 0.0026 0 $ 4.6778 ^{+ 0.0104 }_{- 0.0106 } $ 

$ 4.4508 ^{+ 0.0157 }_{- 0.0260 } $ 

156Er $ \to {}^{152}{\rm{Dy}} $ 

3.4810 ± 0.0025 0 $ 9.9890 ^{+ 0.0217 }_{- 0.0229 } $ 

$ 10.1396 ^{+ 0.0209 }_{- 0.0314 } $ 

154Yb $ \to {}^{150}{\rm{Er}} $ 

5.4743 ± 0.0017 0 $ -0.3549 ^{+ 0.0021 }_{- 0.0021 } $ 

$ -0.5701 ^{+ 0.0072 }_{- 0.0173 } $ 

156Yb $ \to {}^{152}{\rm{Er}} $ 

4.8100 ± 0.0040 0 $ 2.4166 ^{+ 0.0115 }_{- 0.0118 } $ 

$ 2.5614 ^{+ 0.0208 }_{- 0.0312 } $ 

156Hf $ \to {}^{152}{\rm{Yb}} $ 

6.0260 ± 0.0030 0 $ -1.6383 ^{+ 0.0185 }_{- 0.0193 } $ 

$ -1.8568 ^{+ 0.0112 }_{- 0.0215 } $ 

158Hf $ \to {}^{154}{\rm{Yb}} $ 

5.4048 ± 0.0027 0 $ 0.8084 ^{+ 0.0105 }_{- 0.0108 } $ 

$ 0.6970 ^{+ 0.0120 }_{- 0.0225 } $ 

160Hf $ \to {}^{156}{\rm{Yb}} $ 

4.9019 ± 0.0026 0 $ 3.2884 ^{+ 0.0063 }_{- 0.0064 } $ 

$ 3.1354 ^{+ 0.0135 }_{- 0.0242 } $ 

162Hf $ \to {}^{158}{\rm{Yb}} $ 

4.4160 ± 0.0050 0 $ 5.6924 ^{+ 0.0098 }_{- 0.0100 } $ 

$ 5.8968 ^{+ 0.0306 }_{- 0.0415 } $ 

158W $ \to {}^{154}{\rm{Hf}} $ 

6.6125 ± 0.0026 0 $ -2.8447 ^{+ 0.0515 }_{- 0.0584 } $ 

$ -3.0775 ^{+ 0.0086 }_{- 0.0190 } $ 

160W $ \to {}^{156}{\rm{Hf}} $ 

6.0660 ± 0.0050 0 $ -0.9853 ^{+ 0.0235 }_{- 0.0248 } $ 

$ -1.1138 ^{+ 0.0191 }_{- 0.0296 } $ 

162W $ \to {}^{158}{\rm{Hf}} $ 

5.6783 ± 0.0024 0 $ 0.4204 ^{+ 0.0417 }_{- 0.0462 } $ 

$ 0.4647 ^{+ 0.0102 }_{- 0.0209 } $ 

164W $ \to {}^{160}{\rm{Hf}} $ 

5.2783 ± 0.0020 0 $ 2.2196 ^{+ 0.0136 }_{- 0.0140 } $ 

$ 2.2821 ^{+ 0.0095 }_{- 0.0203 } $ 

166W $ \to {}^{162}{\rm{Hf}} $ 

4.8560 ± 0.0040 0 $ 4.7392 ^{+ 0.0134 }_{- 0.0138 } $ 

$ 4.4509 ^{+ 0.0218 }_{- 0.0327 } $ 

168W $ \to {}^{164}{\rm{Hf}} $ 

4.5010 ± 0.0110 0 $ 6.2016 ^{+ 0.0159 }_{- 0.0165 } $ 

$ 6.5191 ^{+ 0.0673 }_{- 0.0786 } $ 

180W $ \to {}^{176}{\rm{Hf}} $ 

2.5153 ± 0.0010 0 $ 25.7005 ^{+ 0.1187 }_{- 0.1640 } $ 

$ 25.5606 ^{+ 0.0151 }_{- 0.0268 } $ 

162Os $ \to {}^{158}{\rm{W}} $ 

6.7678 ± 0.0029 0 $ -2.6778 ^{+ 0.0202 }_{- 0.0212 } $ 

$ -2.7616 ^{+ 0.0096 }_{- 0.0201 } $ 

164Os $ \to {}^{160}{\rm{W}} $ 

6.4790 ± 0.0050 0 $ -1.6619 ^{+ 0.0202 }_{- 0.0212 } $ 

$ -1.7578 ^{+ 0.0177 }_{- 0.0284 } $ 

166Os $ \to {}^{162}{\rm{W}} $ 

6.1430 ± 0.0030 0 $ -0.5928 ^{+ 0.0101 }_{- 0.0103 } $ 

$ -0.4993 ^{+ 0.0116 }_{- 0.0224 } $ 

Continued on next page Table 2-continued from previous page α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{\,exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{\,HOPM}} $ 

168Os $ \to {}^{164}{\rm{W}} $ 

5.8156 ± 0.0027 0 $ 0.6888 ^{+ 0.0202 }_{- 0.0212 } $ 

$ 0.8372 ^{+ 0.0114 }_{- 0.0223 } $ 

170Os $ \to {}^{166}{\rm{W}} $ 

5.5369 ± 0.0027 0 $ 1.8897 ^{+ 0.0105 }_{- 0.0107 } $ 

$ 2.0747 ^{+ 0.0123 }_{- 0.0233 } $ 

172Os $ \to {}^{168}{\rm{W}} $ 

5.2240 ± 0.0070 0 $ 3.2078 ^{+ 0.0199 }_{- 0.0209 } $ 

$ 3.5841 ^{+ 0.0349 }_{- 0.0461 } $ 

174Os $ \to {}^{170}{\rm{W}} $ 

4.8710 ± 0.0100 0 $ 5.2632 ^{+ 0.0378 }_{- 0.0414 } $ 

$ 5.4643 ^{+ 0.0557 }_{- 0.0671 } $ 

186Os $ \to {}^{182}{\rm{W}} $ 

2.8212 ± 0.0009 0 $ 22.8001 ^{+ 0.1903 }_{- 0.3468 } $ 

$ 22.9093 ^{+ 0.0117 }_{- 0.0236 } $ 

166Pt $ \to {}^{162}{\rm{Os}} $ 

7.2920 ± 0.0070 0 $ -3.6716 ^{+ 0.0831 }_{- 0.1029 } $ 

$ -3.6097 ^{+ 0.0210 }_{- 0.0318 } $ 

168Pt $ \to {}^{164}{\rm{Os}} $ 

6.9900 ± 0.0030 0 $ -2.6946 ^{+ 0.0210 }_{- 0.0221 } $ 

$ -2.6525 ^{+ 0.0097 }_{- 0.0205 } $ 

170Pt $ \to {}^{166}{\rm{Os}} $ 

6.7070 ± 0.0030 0 $ -1.8560 ^{+ 0.0050 }_{- 0.0050 } $ 

$ -1.6924 ^{+ 0.0103 }_{- 0.0212 } $ 

172Pt $ \to {}^{168}{\rm{Os}} $ 

6.4630 ± 0.0040 0 $ -0.9928 ^{+ 0.0057 }_{- 0.0058 } $ 

$ -0.8089 ^{+ 0.0146 }_{- 0.0256 } $ 

174Pt $ \to {}^{170}{\rm{Os}} $ 

6.1830 ± 0.0030 0 $ 0.0610 ^{+ 0.0040 }_{- 0.0040 } $ 

$ 0.2698 ^{+ 0.0118 }_{- 0.0228 } $ 

176Pt $ \to {}^{172}{\rm{Os}} $ 

5.8848 ± 0.0020 0 $ 1.1993 ^{+ 0.0102 }_{- 0.0104 } $ 

$ 1.5059 ^{+ 0.0085 }_{- 0.0196 } $ 

178Pt $ \to {}^{174}{\rm{Os}} $ 

5.5730 ± 0.0220 0 $ 2.4295 ^{+ 0.0144 }_{- 0.0149 } $ 

$ 2.9079 ^{+ 0.1018 }_{- 0.1136 } $ 

180Pt $ \to {}^{176}{\rm{Os}} $ 

5.2760 ± 0.0050 0 $ 4.0322 ^{+ 0.0227 }_{- 0.0239 } $ 

$ 4.3638 ^{+ 0.0253 }_{- 0.0366 } $ 

182Pt $ \to {}^{178}{\rm{Os}} $ 

4.9510 ± 0.0050 0 $ 5.6249 ^{+ 0.0191 }_{- 0.0200 } $ 

$ 6.1098 ^{+ 0.0280 }_{- 0.0394 } $ 

184Pt $ \to {}^{180}{\rm{Os}} $ 

4.5990 ± 0.0080 0 $ 7.7857 ^{+ 0.0050 }_{- 0.0050 } $ 

$ 8.2131 ^{+ 0.0502 }_{- 0.0619 } $ 

186Pt $ \to {}^{182}{\rm{Os}} $ 

4.3200 ± 0.0180 0 $ 9.7282 ^{+ 0.0103 }_{- 0.0106 } $ 

$ 10.0701 ^{+ 0.1243 }_{- 0.1367 } $ 

188Pt $ \to {}^{184}{\rm{Os}} $ 

4.0070 ± 0.0050 0 $ 12.5284 ^{+ 0.0076 }_{- 0.0078 } $ 

$ 12.3854 ^{+ 0.0389 }_{- 0.0507 } $ 

190Pt $ \to {}^{186}{\rm{Os}} $ 

3.2686 ± 0.0006 0 $ 20.1830 ^{+ 0.0027 }_{- 0.0027 } $ 

$ 19.1251 ^{+ 0.0064 }_{- 0.0184 } $ 

170Hg $ \to {}^{166}{\rm{Pt}} $ 

7.7700 ± 0.0300 0 $ -3.5086 ^{+ 0.2568 }_{- 0.7132 } $ 

$ -4.2313 ^{+ 0.0835 }_{- 0.0948 } $ 

172Hg $ \to {}^{168}{\rm{Pt}} $ 

7.5240 ± 0.0060 0 $ -3.6364 ^{+ 0.0166 }_{- 0.0173 } $ 

$ -3.5099 ^{+ 0.0176 }_{- 0.0285 } $ 

174Hg $ \to {}^{170}{\rm{Pt}} $ 

7.2330 ± 0.0060 0 $ -2.6990 ^{+ 0.0792 }_{- 0.0969 } $ 

$ -2.6091 ^{+ 0.0188 }_{- 0.0298 } $ 

176Hg $ \to {}^{172}{\rm{Pt}} $ 

6.8970 ± 0.0060 0 $ -1.6467 ^{+ 0.0290 }_{- 0.0310 } $ 

$ -1.4965 ^{+ 0.0203 }_{- 0.0314 } $ 

178Hg $ \to {}^{174}{\rm{Pt}} $ 

6.5773 ± 0.0030 0 $ -0.5237 ^{+ 0.0039 }_{- 0.0039 } $ 

$ -0.3535 ^{+ 0.0110 }_{- 0.0222 } $ 

180Hg $ \to {}^{176}{\rm{Pt}} $ 

6.2585 ± 0.0023 0 $ 0.7321 ^{+ 0.0017 }_{- 0.0017 } $ 

$ 0.8778 ^{+ 0.0091 }_{- 0.0204 } $ 

182Hg $ \to {}^{178}{\rm{Pt}} $ 

5.9960 ± 0.0050 0 $ 1.8947 ^{+ 0.0024 }_{- 0.0024 } $ 

$ 1.9716 ^{+ 0.0212 }_{- 0.0326 } $ 

184Hg $ \to {}^{180}{\rm{Pt}} $ 

5.6600 ± 0.0040 0 $ 3.4442 ^{+ 0.0036 }_{- 0.0037 } $ 

$ 3.4823 ^{+ 0.0186 }_{- 0.0301 } $ 

186Hg $ \to {}^{182}{\rm{Pt}} $ 

5.2040 ± 0.0100 0 $ 5.7139 ^{+ 0.0185 }_{- 0.0193 } $ 

$ 5.7677 ^{+ 0.0531 }_{- 0.0649 } $ 

188Hg $ \to {}^{184}{\rm{Pt}} $ 

4.7090 ± 0.0150 0 $ 8.7218 ^{+ 0.0196 }_{- 0.0205 } $ 

$ 8.6312 ^{+ 0.0931 }_{- 0.1054 } $ 

178Pb $ \to {}^{174}{\rm{Hg}} $ 

7.7890 ± 0.0130 0 $ -3.6021 ^{+ 0.1206 }_{- 0.1675 } $ 

$ -3.5051 ^{+ 0.0371 }_{- 0.0483 } $ 

180Pb $ \to {}^{176}{\rm{Hg}} $ 

7.4190 ± 0.0050 0 $ -2.3872 ^{+ 0.0307 }_{- 0.0330 } $ 

$ -2.3876 ^{+ 0.0155 }_{- 0.0267 } $ 

182Pb $ \to {}^{178}{\rm{Hg}} $ 

7.0660 ± 0.0060 0 $ -1.2596 ^{+ 0.0378 }_{- 0.0414 } $ 

$ -1.2337 ^{+ 0.0201 }_{- 0.0314 } $ 

184Pb $ \to {}^{180}{\rm{Hg}} $ 

6.7740 ± 0.0030 0 $ -0.2129 ^{+ 0.0216 }_{- 0.0227 } $ 

$ -0.2043 ^{+ 0.0108 }_{- 0.0222 } $ 

186Pb $ \to {}^{182}{\rm{Hg}} $ 

6.4710 ± 0.0050 0 $ 1.0810 ^{+ 0.0027 }_{- 0.0027 } $ 

$ 0.9406 ^{+ 0.0193 }_{- 0.0308 } $ 

188Pb $ \to {}^{184}{\rm{Hg}} $ 

6.1090 ± 0.0030 0 $ 2.4703 ^{+ 0.0017 }_{- 0.0017 } $ 

$ 2.4218 ^{+ 0.0127 }_{- 0.0243 } $ 

190Pb $ \to {}^{186}{\rm{Hg}} $ 

5.6980 ± 0.0050 0 $ 4.2492 ^{+ 0.0061 }_{- 0.0062 } $ 

$ 4.2790 ^{+ 0.0237 }_{- 0.0354 } $ 

192Pb $ \to {}^{188}{\rm{Hg}} $ 

5.2220 ± 0.0050 0 $ 6.5462 ^{+ 0.0122 }_{- 0.0126 } $ 

$ 6.7095 ^{+ 0.0272 }_{- 0.0391 } $ 

194Pb $ \to {}^{190}{\rm{Hg}} $ 

4.7380 ± 0.0170 0 $ 9.9442 ^{+ 0.0237 }_{- 0.0251 } $ 

$ 9.5642 ^{+ 0.1073 }_{- 0.1200 } $ 

210Pb $ \to {}^{206}{\rm{Pb}} $ 

3.7920 ± 0.0200 0 $ 16.5667 ^{+ 0.0043 }_{- 0.0043 } $ 

$ 15.3018 ^{+ 0.1776 }_{- 0.1919 } $ 

186Po $ \to {}^{182}{\rm{Pb}} $ 

8.5010 ± 0.0140 0 $ -4.4685 ^{+ 0.1313 }_{- 0.1891 } $ 

$ -4.6712 ^{+ 0.0356 }_{- 0.0467 } $ 

188Po $ \to {}^{184}{\rm{Pb}} $ 

8.0820 ± 0.0150 0 $ -3.5686 ^{+ 0.0458 }_{- 0.0512 } $ 

$ -3.5430 ^{+ 0.0415 }_{- 0.0528 } $ 

190Po $ \to {}^{186}{\rm{Pb}} $ 

7.6930 ± 0.0070 0 $ -2.6108 ^{+ 0.0088 }_{- 0.0090 } $ 

$ -2.4058 ^{+ 0.0210 }_{- 0.0323 } $ 

Continued on next page Table 2-continued from previous page α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{\,exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{\,HOPM}} $ 

192Po $ \to {}^{188}{\rm{Pb}} $ 

7.3200 ± 0.0030 0 $ -1.4921 ^{+ 0.0040 }_{- 0.0041 } $ 

$ -1.2241 ^{+ 0.0098 }_{- 0.0212 } $ 

194Po $ \to {}^{190}{\rm{Pb}} $ 

6.9870 ± 0.0030 0 $ -0.4067 ^{+ 0.0044 }_{- 0.0045 } $ 

$ -0.0828 ^{+ 0.0105 }_{- 0.0221 } $ 

196Po $ \to {}^{192}{\rm{Pb}} $ 

6.6582 ± 0.0024 0 $ 0.7774 ^{+ 0.0054 }_{- 0.0054 } $ 

$ 1.1328 ^{+ 0.0091 }_{- 0.0207 } $ 

198Po $ \to {}^{194}{\rm{Pb}} $ 

6.3097 ± 0.0014 0 $ 2.2678 ^{+ 0.0059 }_{- 0.0060 } $ 

$ 2.5289 ^{+ 0.0058 }_{- 0.0175 } $ 

200Po $ \to {}^{196}{\rm{Pb}} $ 

5.9816 ± 0.0018 0 $ 3.7939 ^{+ 0.0030 }_{- 0.0030 } $ 

$ 3.9603 ^{+ 0.0081 }_{- 0.0200 } $ 

202Po $ \to {}^{198}{\rm{Pb}} $ 

5.7010 ± 0.0050 0 $ 5.1442 ^{+ 0.0039 }_{- 0.0039 } $ 

$ 5.2884 ^{+ 0.0243 }_{- 0.0363 } $ 

204Po $ \to {}^{200}{\rm{Pb}} $ 

5.4849 ± 0.0014 0 $ 6.2766 ^{+ 0.0015 }_{- 0.0015 } $ 

$ 6.3867 ^{+ 0.0072 }_{- 0.0192 } $ 

206Po $ \to {}^{202}{\rm{Pb}} $ 

5.3270 ± 0.0013 0 $ 7.1446 ^{+ 0.0049 }_{- 0.0050 } $ 

$ 7.2368 ^{+ 0.0070 }_{- 0.0191 } $ 

208Po $ \to {}^{204}{\rm{Pb}} $ 

5.2157 ± 0.0013 0 $ 7.9612 ^{+ 0.0003 }_{- 0.0003 } $ 

$ 7.8642 ^{+ 0.0073 }_{- 0.0193 } $ 

210Po $ \to {}^{206}{\rm{Pb}} $ 

5.4075 ± 0.0001 0 $ 7.0776 ^{+ 0.0000 }_{- 0.0000 } $ 

$ 6.8360 ^{+ 0.0005 }_{- 0.0122 } $ 

212Po $ \to {}^{208}{\rm{Pb}} $ 

8.9542 ± 0.0001 0 $ -6.5311 ^{+ 0.0117 }_{- 0.0120 } $ 

$ -6.9453 ^{+ 0.0002 }_{- 0.0112 } $ 

214Po $ \to {}^{210}{\rm{Pb}} $ 

7.8335 ± 0.0001 0 $ -3.7866 ^{+ 0.0000 }_{- 0.0000 } $ 

$ -4.0399 ^{+ 0.0003 }_{- 0.0114 } $ 

216Po $ \to {}^{212}{\rm{Pb}} $ 

6.9063 ± 0.0005 0 $ -0.8416 ^{+ 0.0018 }_{- 0.0018 } $ 

$ -1.0594 ^{+ 0.0018 }_{- 0.0135 } $ 

218Po $ \to {}^{214}{\rm{Pb}} $ 

6.1148 ± 0.0001 0 $ 2.2692 ^{+ 0.0017 }_{- 0.0017 } $ 

$ 2.0525 ^{+ 0.0002 }_{- 0.0127 } $ 

194Rn $ \to {}^{190}{\rm{Po}} $ 

7.8620 ± 0.0100 0 $ -3.1079 ^{+ 0.0810 }_{- 0.0997 } $ 

$ -2.1543 ^{+ 0.0297 }_{- 0.0413 } $ 

196Rn $ \to {}^{192}{\rm{Po}} $ 

7.6170 ± 0.0090 0 $ -2.3279 ^{+ 0.0913 }_{- 0.1158 } $ 

$ -1.3906 ^{+ 0.0282 }_{- 0.0398 } $ 

198Rn $ \to {}^{194}{\rm{Po}} $ 

7.3490 ± 0.0040 0 $ -1.1596 ^{+ 0.0107 }_{- 0.0109 } $ 

$ -0.5105 ^{+ 0.0133 }_{- 0.0250 } $ 

200Rn $ \to {}^{196}{\rm{Po}} $ 

7.0434 ± 0.0021 0 $ 0.0736 ^{+ 0.0595 }_{- 0.0689 } $ 

$ 0.5556 ^{+ 0.0075 }_{- 0.0193 } $ 

202Rn $ \to {}^{198}{\rm{Po}} $ 

6.7738 ± 0.0018 0 $ 1.0947 ^{+ 0.0045 }_{- 0.0045 } $ 

$ 1.5610 ^{+ 0.0068 }_{- 0.0187 } $ 

204Rn $ \to {}^{200}{\rm{Po}} $ 

6.5467 ± 0.0018 0 $ 2.0125 ^{+ 0.0080 }_{- 0.0081 } $ 

$ 2.4606 ^{+ 0.0072 }_{- 0.0191 } $ 

206Rn $ \to {}^{202}{\rm{Po}} $ 

6.3837 ± 0.0016 0 $ 2.7393 ^{+ 0.0128 }_{- 0.0132 } $ 

$ 3.1415 ^{+ 0.0067 }_{- 0.0187 } $ 

208Rn $ \to {}^{204}{\rm{Po}} $ 

6.2607 ± 0.0017 0 $ 3.3723 ^{+ 0.0025 }_{- 0.0025 } $ 

$ 3.6771 ^{+ 0.0073 }_{- 0.0193 } $ 

210Rn $ \to {}^{206}{\rm{Po}} $ 

6.1590 ± 0.0022 0 $ 3.9542 ^{+ 0.0177 }_{- 0.0185 } $ 

$ 4.1349 ^{+ 0.0097 }_{- 0.0217 } $ 

212Rn $ \to {}^{208}{\rm{Po}} $ 

6.3851 ± 0.0026 0 $ 3.1565 ^{+ 0.0036 }_{- 0.0036 } $ 

$ 3.1794 ^{+ 0.0108 }_{- 0.0228 } $ 

214Rn $ \to {}^{210}{\rm{Po}} $ 

9.2080 ± 0.0090 0 $ -6.5867 ^{+ 0.0050 }_{- 0.0051 } $ 

$ -6.8968 ^{+ 0.0203 }_{- 0.0315 } $ 

216Rn $ \to {}^{212}{\rm{Po}} $ 

8.1980 ± 0.0060 0 $ -4.5376 ^{+ 0.0561 }_{- 0.0645 } $ 

$ -4.3646 ^{+ 0.0165 }_{- 0.0280 } $ 

218Rn $ \to {}^{214}{\rm{Po}} $ 

7.2625 ± 0.0019 0 $ -1.4717 ^{+ 0.0019 }_{- 0.0019 } $ 

$ -1.5091 ^{+ 0.0064 }_{- 0.0183 } $ 

220Rn $ \to {}^{216}{\rm{Po}} $ 

6.4047 ± 0.0001 0 $ 1.7451 ^{+ 0.0008 }_{- 0.0008 } $ 

$ 1.6924 ^{+ 0.0006 }_{- 0.0125 } $ 

222Rn $ \to {}^{218}{\rm{Po}} $ 

5.5904 ± 0.0003 0 $ 5.5187 ^{+ 0.0000 }_{- 0.0000 } $ 

$ 5.4409 ^{+ 0.0015 }_{- 0.0142 } $ 

202Ra $ \to {}^{198}{\rm{Rn}} $ 

7.8800 ± 0.0070 0 $ -2.3872 ^{+ 0.1032 }_{- 0.1357 } $ 

$ -1.4246 ^{+ 0.0213 }_{- 0.0331 } $ 

204Ra $ \to {}^{200}{\rm{Rn}} $ 

7.6370 ± 0.0070 0 $ -1.2218 ^{+ 0.0607 }_{- 0.0706 } $ 

$ -0.6507 ^{+ 0.0224 }_{- 0.0343 } $ 

206Ra $ \to {}^{202}{\rm{Rn}} $ 

7.4150 ± 0.0040 0 $ -0.6198 ^{+ 0.0348 }_{- 0.0378 } $ 

$ 0.0928 ^{+ 0.0134 }_{- 0.0254 } $ 

208Ra $ \to {}^{204}{\rm{Rn}} $ 

7.2730 ± 0.0050 0 $ 0.1058 ^{+ 0.0173 }_{- 0.0180 } $ 

$ 0.5925 ^{+ 0.0173 }_{- 0.0293 } $ 

210Ra $ \to {}^{206}{\rm{Rn}} $ 

7.1510 ± 0.0030 0 $ 0.6021 ^{+ 0.0107 }_{- 0.0110 } $ 

$ 1.0362 ^{+ 0.0107 }_{- 0.0227 } $ 

214Ra $ \to {}^{210}{\rm{Rn}} $ 

7.2726 ± 0.0026 0 $ 0.3871 ^{+ 0.0028 }_{- 0.0029 } $ 

$ 0.6370 ^{+ 0.0090 }_{- 0.0209 } $ 

216Ra $ \to {}^{212}{\rm{Rn}} $ 

9.5260 ± 0.0070 0 $ -6.7645 ^{+ 0.0173 }_{- 0.0180 } $ 

$ -6.9906 ^{+ 0.0153 }_{- 0.0267 } $ 

218Ra $ \to {}^{214}{\rm{Rn}} $ 

8.5400 ± 0.0120 0 $ -4.5865 ^{+ 0.0023 }_{- 0.0024 } $ 

$ -4.6056 ^{+ 0.0316 }_{- 0.0434 } $ 

220Ra $ \to {}^{216}{\rm{Rn}} $ 

7.5940 ± 0.0050 0 $ -1.7423 ^{+ 0.0279 }_{- 0.0298 } $ 

$ -1.8429 ^{+ 0.0160 }_{- 0.0281 } $ 

222Ra $ \to {}^{218}{\rm{Rn}} $ 

6.6780 ± 0.0040 0 $ 1.5263 ^{+ 0.0051 }_{- 0.0052 } $ 

$ 1.4268 ^{+ 0.0158 }_{- 0.0283 } $ 

224Ra $ \to {}^{220}{\rm{Rn}} $ 

5.7889 ± 0.0002 0 $ 5.4966 ^{+ 0.0002 }_{- 0.0002 } $ 

$ 5.3737 ^{+ 0.0008 }_{- 0.0135 } $ 

226Ra $ \to {}^{222}{\rm{Rn}} $ 

4.8707 ± 0.0003 0 $ 10.7032 ^{+ 0.0019 }_{- 0.0019 } $ 

$ 10.6053 ^{+ 0.0016 }_{- 0.0149 } $ 

Continued on next page Table 2-continued from previous page α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{\,exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{\,HOPM}} $ 

208Th $ \to {}^{204}{\rm{Ra}} $ 

8.2000 ± 0.0300 0 $ -2.6198 ^{+ 0.1761 }_{- 0.3010 } $ 

$ -1.6203 ^{+ 0.0876 }_{- 0.1000 } $ 

210Th $ \to {}^{206}{\rm{Ra}} $ 

8.0960 ± 0.0060 0 $ -1.7959 ^{+ 0.0881 }_{- 0.1107 } $ 

$ -1.2980 ^{+ 0.0179 }_{- 0.0299 } $ 

212Th $ \to {}^{208}{\rm{Ra}} $ 

7.9580 ± 0.0050 0 $ -1.4989 ^{+ 0.0175 }_{- 0.0182 } $ 

$ -0.8651 ^{+ 0.0154 }_{- 0.0274 } $ 

214Th $ \to {}^{210}{\rm{Ra}} $ 

7.8270 ± 0.0050 0 $ -1.0605 ^{+ 0.0473 }_{- 0.0530 } $ 

$ -0.4425 ^{+ 0.0158 }_{- 0.0278 } $ 

216Th $ \to {}^{212}{\rm{Ra}} $ 

8.0720 ± 0.0040 0 $ -1.5804 ^{+ 0.0026 }_{- 0.0027 } $ 

$ -1.1834 ^{+ 0.0120 }_{- 0.0239 } $ 

218Th $ \to {}^{214}{\rm{Ra}} $ 

9.8490 ± 0.0090 0 $ -6.9136 ^{+ 0.0174 }_{- 0.0182 } $ 

$ -7.0891 ^{+ 0.0191 }_{- 0.0307 } $ 

220Th $ \to {}^{216}{\rm{Ra}} $ 

8.9730 ± 0.0110 0 $ -4.9914 ^{+ 0.0126 }_{- 0.0130 } $ 

$ -5.0586 ^{+ 0.0274 }_{- 0.0393 } $ 

222Th $ \to {}^{218}{\rm{Ra}} $ 

8.1326 ± 0.0029 0 $ -2.6498 ^{+ 0.0058 }_{- 0.0059 } $ 

$ -2.7726 ^{+ 0.0085 }_{- 0.0207 } $ 

224Th $ \to {}^{220}{\rm{Ra}} $ 

7.2990 ± 0.0060 0 $ 0.0170 ^{+ 0.0083 }_{- 0.0084 } $ 

$ -0.0859 ^{+ 0.0210 }_{- 0.0336 } $ 

226Th $ \to {}^{222}{\rm{Ra}} $ 

6.4525 ± 0.0010 0 $ 3.2653 ^{+ 0.0004 }_{- 0.0004 } $ 

$ 3.2039 ^{+ 0.0043 }_{- 0.0172 } $ 

228Th $ \to {}^{224}{\rm{Ra}} $ 

5.5202 ± 0.0002 0 $ 7.7807 ^{+ 0.0002 }_{- 0.0002 } $ 

$ 7.7299 ^{+ 0.0009 }_{- 0.0148 } $ 

230Th $ \to {}^{226}{\rm{Ra}} $ 

4.7700 ± 0.0015 0 $ 12.3765 ^{+ 0.0017 }_{- 0.0017 } $ 

$ 12.3551 ^{+ 0.0103 }_{- 0.0240 } $ 

232Th $ \to {}^{228}{\rm{Ra}} $ 

4.0816 ± 0.0014 0 $ 18.6452 ^{+ 0.0031 }_{- 0.0031 } $ 

$ 17.7276 ^{+ 0.0123 }_{- 0.0262 } $ 

218U $ \to {}^{214}{\rm{Th}} $ 

8.7750 ± 0.0090 0 $ -3.4510 ^{+ 0.0994 }_{- 0.1290 } $ 

$ -2.4735 ^{+ 0.0242 }_{- 0.0362 } $ 

222U $ \to {}^{218}{\rm{Th}} $ 

9.4800 ± 0.0500 0 $ -5.3279 ^{+ 0.0355 }_{- 0.0386 } $ 

$ -5.6497 ^{+ 0.1160 }_{- 0.1290 } $ 

224U $ \to {}^{220}{\rm{Th}} $ 

8.6280 ± 0.0070 0 $ -3.4023 ^{+ 0.0183 }_{- 0.0191 } $ 

$ -3.4880 ^{+ 0.0191 }_{- 0.0314 } $ 

226U $ \to {}^{222}{\rm{Th}} $ 

7.7010 ± 0.0040 0 $ -0.5702 ^{+ 0.0096 }_{- 0.0098 } $ 

$ -0.6968 ^{+ 0.0132 }_{- 0.0259 } $ 

228U $ \to {}^{224}{\rm{Th}} $ 

6.8000 ± 0.0090 0 $ 2.7482 ^{+ 0.0094 }_{- 0.0097 } $ 

$ 2.5962 ^{+ 0.0362 }_{- 0.0494 } $ 

230U $ \to {}^{226}{\rm{Th}} $ 

5.9925 ± 0.0005 0 $ 6.2425 ^{+ 0.0004 }_{- 0.0004 } $ 

$ 6.2069 ^{+ 0.0025 }_{- 0.0159 } $ 

232U $ \to {}^{228}{\rm{Th}} $ 

5.4136 ± 0.0001 0 $ 9.3373 ^{+ 0.0025 }_{- 0.0025 } $ 

$ 9.3132 ^{+ 0.0007 }_{- 0.0140 } $ 

234U $ \to {}^{230}{\rm{Th}} $ 

4.8575 ± 0.0007 0 $ 12.8891 ^{+ 0.0011 }_{- 0.0011 } $ 

$ 12.8325 ^{+ 0.0048 }_{- 0.0187 } $ 

236U $ \to {}^{232}{\rm{Th}} $ 

4.5730 ± 0.0009 0 $ 14.8687 ^{+ 0.0007 }_{- 0.0007 } $ 

$ 14.8945 ^{+ 0.0068 }_{- 0.0208 } $ 

238U $ \to {}^{234}{\rm{Th}} $ 

4.2699 ± 0.0021 0 $ 18.1487 ^{+ 0.0003 }_{- 0.0003 } $ 

$ 17.3210 ^{+ 0.0176 }_{- 0.0318 } $ 

230Pu $ \to {}^{226}{\rm{U}} $ 

7.1780 ± 0.0200 0 $ 2.0212 ^{+ 0.0395 }_{- 0.0435 } $ 

$ 1.9240 ^{+ 0.0754 }_{- 0.0890 } $ 

232Pu $ \to {}^{228}{\rm{U}} $ 

6.7160 ± 0.0100 0 $ 4.0048 ^{+ 0.0082 }_{- 0.0084 } $ 

$ 3.7802 ^{+ 0.0420 }_{- 0.0556 } $ 

234Pu $ \to {}^{230}{\rm{U}} $ 

6.3100 ± 0.0050 0 $ 5.7226 ^{+ 0.0049 }_{- 0.0050 } $ 

$ 5.5898 ^{+ 0.0232 }_{- 0.0369 } $ 

236Pu $ \to {}^{232}{\rm{U}} $ 

5.8672 ± 0.0001 0 $ 7.9552 ^{+ 0.0012 }_{- 0.0012 } $ 

$ 7.7850 ^{+ 0.0002 }_{- 0.0145 } $ 

238Pu $ \to {}^{234}{\rm{U}} $ 

5.5933 ± 0.0002 0 $ 9.4421 ^{+ 0.0005 }_{- 0.0005 } $ 

$ 9.2841 ^{+ 0.0009 }_{- 0.0151 } $ 

240Pu $ \to {}^{236}{\rm{U}} $ 

5.2558 ± 0.0001 0 $ 11.3161 ^{+ 0.0005 }_{- 0.0005 } $ 

$ 11.2939 ^{+ 0.0010 }_{- 0.0148 } $ 

242Pu $ \to {}^{238}{\rm{U}} $ 

4.9842 ± 0.0010 0 $ 13.0731 ^{+ 0.0023 }_{- 0.0023 } $ 

$ 13.0667 ^{+ 0.0067 }_{- 0.0209 } $ 

244Pu $ \to {}^{240}{\rm{U}} $ 

4.6656 ± 0.0010 0 $ 15.4097 ^{+ 0.0016 }_{- 0.0016 } $ 

$ 15.3452 ^{+ 0.0075 }_{- 0.0217 } $ 

234Cm $ \to {}^{230}{\rm{Pu}} $ 

7.3650 ± 0.0090 0 $ 2.2846 ^{+ 0.0693 }_{- 0.0825 } $ 

$ 2.0180 ^{+ 0.0334 }_{- 0.0469 } $ 

236Cm $ \to {}^{232}{\rm{Pu}} $ 

7.0670 ± 0.0050 0 $ 3.3554 ^{+ 0.0483 }_{- 0.0544 } $ 

$ 3.1757 ^{+ 0.0198 }_{- 0.0335 } $ 

238Cm $ \to {}^{234}{\rm{Pu}} $ 

6.6700 ± 0.0100 0 $ 5.3144 ^{+ 0.0207 }_{- 0.0217 } $ 

$ 4.8399 ^{+ 0.0435 }_{- 0.0574 } $ 

240Cm $ \to {}^{236}{\rm{Pu}} $ 

6.3978 ± 0.0006 0 $ 6.4194 ^{+ 0.0499 }_{- 0.0564 } $ 

$ 6.0796 ^{+ 0.0028 }_{- 0.0167 } $ 

242Cm $ \to {}^{238}{\rm{Pu}} $ 

6.2156 ± 0.0001 0 $ 7.1482 ^{+ 0.0005 }_{- 0.0005 } $ 

$ 6.9618 ^{+ 0.0005 }_{- 0.0142 } $ 

244Cm $ \to {}^{240}{\rm{Pu}} $ 

5.9016 ± 0.0000 0 $ 8.7570 ^{+ 0.0007 }_{- 0.0007 } $ 

$ 8.5707 ^{+ 0.0002 }_{- 0.0142 } $ 

246Cm $ \to {}^{242}{\rm{Pu}} $ 

5.4751 ± 0.0009 0 $ 11.1719 ^{+ 0.0037 }_{- 0.0037 } $ 

$ 10.9793 ^{+ 0.0054 }_{- 0.0196 } $ 

248Cm $ \to {}^{244}{\rm{Pu}} $ 

5.1618 ± 0.0003 0 $ 13.0787 ^{+ 0.0074 }_{- 0.0076 } $ 

$ 12.9485 ^{+ 0.0017 }_{- 0.0159 } $ 

238Cf $ \to {}^{234}{\rm{Cm}} $ 

8.1300 ± 0.3000 0 $ -0.0737 ^{+ 0.0260 }_{- 0.0276 } $ 

$ 0.1277 ^{+ 0.9415 }_{- 1.0136 } $ 

240Cf $ \to {}^{236}{\rm{Cm}} $ 

7.7110 ± 0.0040 0 $ 1.6119 ^{+ 0.0096 }_{- 0.0098 } $ 

$ 1.5564 ^{+ 0.0141 }_{- 0.0277 } $ 

Continued on next page Table 2-continued from previous page α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{\,exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{\,HOPM}} $ 

242Cf $ \to {}^{238}{\rm{Cm}} $ 

7.5170 ± 0.0040 0 $ 2.5356 ^{+ 0.0183 }_{- 0.0191 } $ 

$ 2.2689 ^{+ 0.0147 }_{- 0.0284 } $ 

244Cf $ \to {}^{240}{\rm{Cm}} $ 

7.3290 ± 0.0018 0 $ 3.1931 ^{+ 0.0110 }_{- 0.0113 } $ 

$ 2.9882 ^{+ 0.0069 }_{- 0.0207 } $ 

246Cf $ \to {}^{242}{\rm{Cm}} $ 

6.8616 ± 0.0010 0 $ 5.1090 ^{+ 0.0060 }_{- 0.0061 } $ 

$ 4.8891 ^{+ 0.0043 }_{- 0.0182 } $ 

248Cf $ \to {}^{244}{\rm{Cm}} $ 

6.3610 ± 0.0050 0 $ 7.4596 ^{+ 0.0349 }_{- 0.0379 } $ 

$ 7.1669 ^{+ 0.0240 }_{- 0.0382 } $ 

250Cf $ \to {}^{246}{\rm{Cm}} $ 

6.1285 ± 0.0002 0 $ 8.6160 ^{+ 0.0030 }_{- 0.0030 } $ 

$ 8.3320 ^{+ 0.0010 }_{- 0.0152 } $ 

252Cf $ \to {}^{248}{\rm{Cm}} $ 

6.2170 ± 0.0000 0 $ 7.9352 ^{+ 0.0013 }_{- 0.0013 } $ 

$ 7.9004 ^{+ 0.0000 }_{- 0.0146 } $ 

254Cf $ \to {}^{250}{\rm{Cm}} $ 

5.9270 ± 0.0050 0 $ 9.2269 ^{+ 0.0014 }_{- 0.0014 } $ 

$ 9.4157 ^{+ 0.0269 }_{- 0.0412 } $ 

244Fm $ \to {}^{240}{\rm{Cf}} $ 

8.5500 ± 0.2000 0 $ -0.5058 ^{+ 0.0110 }_{- 0.0113 } $ 

$ -0.4594 ^{+ 0.5977 }_{- 0.6347 } $ 

246Fm $ \to {}^{242}{\rm{Cf}} $ 

8.3790 ± 0.0050 0 $ 0.2181 ^{+ 0.0111 }_{- 0.0114 } $ 

$ 0.0843 ^{+ 0.0157 }_{- 0.0294 } $ 

248Fm $ \to {}^{244}{\rm{Cf}} $ 

7.9950 ± 0.0080 0 $ 1.5378 ^{+ 0.0148 }_{- 0.0154 } $ 

$ 1.3549 ^{+ 0.0272 }_{- 0.0410 } $ 

250Fm $ \to {}^{246}{\rm{Cf}} $ 

7.5570 ± 0.0080 0 $ 3.2695 ^{+ 0.0151 }_{- 0.0157 } $ 

$ 2.9279 ^{+ 0.0298 }_{- 0.0438 } $ 

252Fm $ \to {}^{248}{\rm{Cf}} $ 

7.1537 ± 0.0010 0 $ 4.9610 ^{+ 0.0007 }_{- 0.0007 } $ 

$ 4.5128 ^{+ 0.0041 }_{- 0.0182 } $ 

254Fm $ \to {}^{250}{\rm{Cf}} $ 

7.3073 ± 0.0010 0 $ 4.0671 ^{+ 0.0003 }_{- 0.0003 } $ 

$ 3.9122 ^{+ 0.0039 }_{- 0.0180 } $ 

256Fm $ \to {}^{252}{\rm{Cf}} $ 

7.0253 ± 0.0019 0 $ 5.0658 ^{+ 0.0036 }_{- 0.0036 } $ 

$ 5.0715 ^{+ 0.0080 }_{- 0.0221 } $ 

252No $ \to {}^{248}{\rm{Fm}} $ 

8.5490 ± 0.0050 0 $ 0.5622 ^{+ 0.0028 }_{- 0.0028 } $ 

$ 0.2769 ^{+ 0.0156 }_{- 0.0294 } $ 

254No $ \to {}^{250}{\rm{Fm}} $ 

8.2260 ± 0.0080 0 $ 1.7550 ^{+ 0.0034 }_{- 0.0034 } $ 

$ 1.3296 ^{+ 0.0265 }_{- 0.0406 } $ 

256No $ \to {}^{252}{\rm{Fm}} $ 

8.5820 ± 0.0050 0 $ 0.4663 ^{+ 0.0074 }_{- 0.0075 } $ 

$ 0.2020 ^{+ 0.0155 }_{- 0.0293 } $ 

256Rf $ \to {}^{252}{\rm{No}} $ 

8.9260 ± 0.0150 0 $ 0.3282 ^{+ 0.0033 }_{- 0.0033 } $ 

$ -0.1738 ^{+ 0.0444 }_{- 0.0586 } $ 

258Rf $ \to {}^{254}{\rm{No}} $ 

9.1960 ± 0.0130 0 $ -0.5933 ^{+ 0.0170 }_{- 0.0177 } $ 

$ -0.9416 ^{+ 0.0367 }_{- 0.0506 } $ 

260Sg $ \to {}^{256}{\rm{Rf}} $ 

9.9010 ± 0.0100 0 $ -1.7678 ^{+ 0.0280 }_{- 0.0300 } $ 

$ -2.2001 ^{+ 0.0255 }_{- 0.0394 } $ 

266Hs $ \to {}^{262}{\rm{Sg}} $ 

10.3460 ± 0.0160 0 $ -2.4037 ^{+ 0.0792 }_{- 0.0969 } $ 

$ -2.6745 ^{+ 0.0387 }_{- 0.0528 } $ 

268Hs $ \to {}^{264}{\rm{Sg}} $ 

9.7600 ± 0.1000 0 $ 0.1461 ^{+ 0.2518 }_{- 0.6690 } $ 

$ -1.1695 ^{+ 0.2650 }_{- 0.2838 } $ 

270Hs $ \to {}^{266}{\rm{Sg}} $ 

9.0700 ± 0.0400 0 $ 0.9542 ^{+ 0.1597 }_{- 0.2553 } $ 

$ 0.8032 ^{+ 0.1202 }_{- 0.1356 } $ 

270Ds $ \to {}^{266}{\rm{Hs}} $ 

11.1170 ± 0.0280 0 $ -3.6882 ^{+ 0.0914 }_{- 0.1159 } $ 

$ -3.8611 ^{+ 0.0613 }_{- 0.0755 } $ 

282Ds $ \to {}^{278}{\rm{Hs}} $ 

9.1500 ± 0.4200 0 $ 2.4014 ^{+ 0.2518 }_{- 0.6690 } $ 

$ 1.2962 ^{+ 1.2293 }_{- 1.3403 } $ 

286Cn $ \to {}^{282}{\rm{Ds}} $ 

9.2400 ± 0.7600 0 $ 1.4771 ^{+ 0.3010 }_{- 0.3010 } $ 

$ 1.7006 ^{+ 2.1712 }_{- 2.5004 } $ 

286Fl $ \to {}^{282}{\rm{Cn}} $ 

10.3600 ± 0.0400 0 $ -0.6569 ^{+ 0.0099 }_{- 0.0101 } $ 

$ -0.8175 ^{+ 0.1026 }_{- 0.1181 } $ 

288Fl $ \to {}^{284}{\rm{Cn}} $ 

10.0760 ± 0.0120 0 $ -0.1851 ^{+ 0.0693 }_{- 0.0825 } $ 

$ -0.0558 ^{+ 0.0323 }_{- 0.0473 } $ 

290Lv $ \to {}^{286}{\rm{Fl}} $ 

11.0000 ± 0.0600 0 $ -2.0458 ^{+ 0.1249 }_{- 0.1761 } $ 

$ -1.7981 ^{+ 0.1419 }_{- 0.1580 } $ 

292Lv $ \to {}^{288}{\rm{Fl}} $ 

10.7910 ± 0.0120 0 $ -1.7959 ^{+ 0.1383 }_{- 0.2041 } $ 

$ -1.2802 ^{+ 0.0294 }_{- 0.0444 } $ 

294Og $ \to {}^{290}{\rm{Lv}} $ 

11.8700 ± 0.0300 0 $ -3.1549 ^{+ 0.1549 }_{- 0.2430 } $ 

$ -3.1857 ^{+ 0.0638 }_{- 0.0788 } $ 

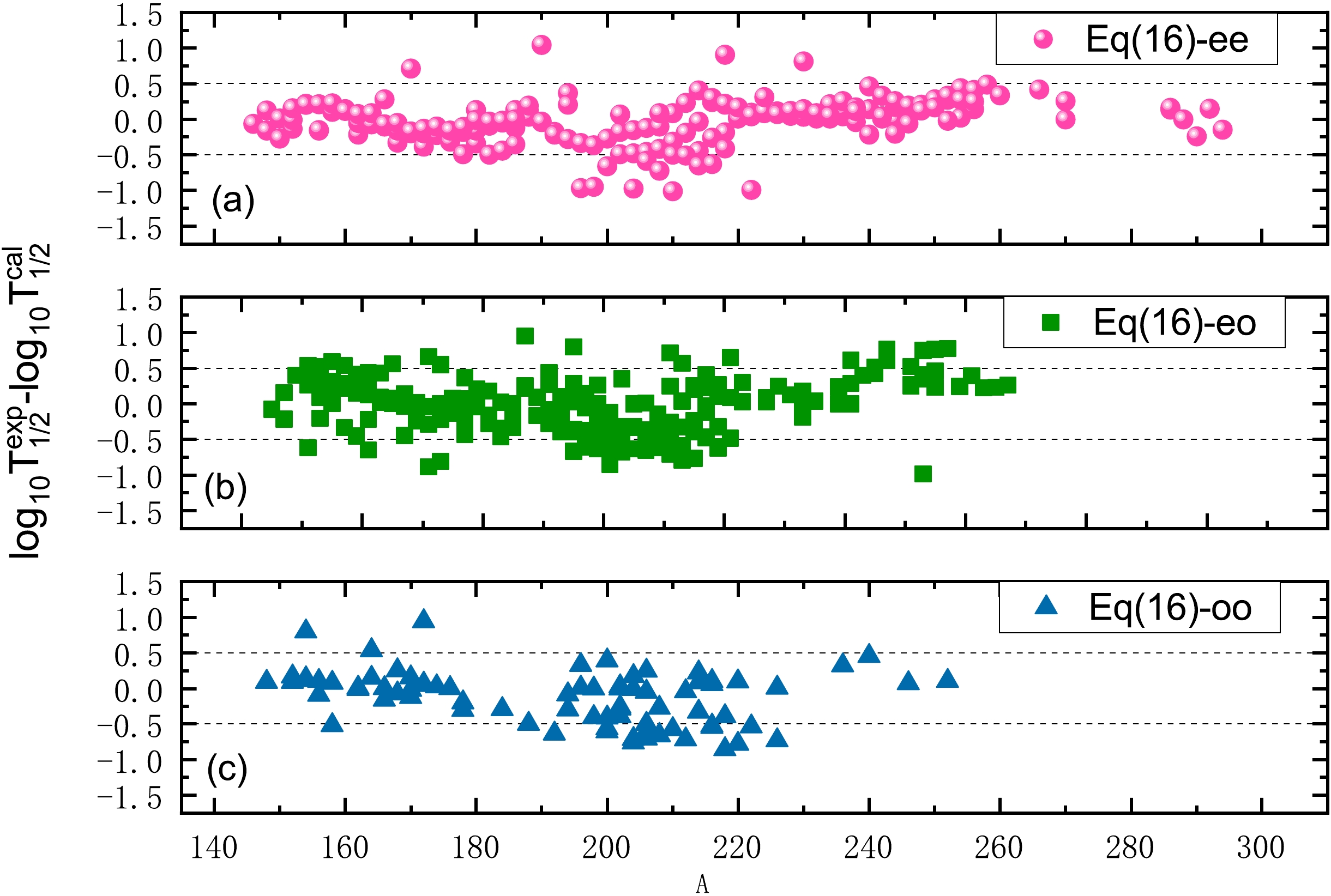
Figure 2. (color online) Deviations between the experimental half-lives and those calculated in logarithmic form using Eq. (16) for favored α decay for (a) even-even nuclei, (b) odd-A nuclei, and (c) odd-odd nuclei, respectively.
α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{\,exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{\,HOPM}} $ 

105Te $ \to {}^{101}{\rm{Nb}} $ 

5.0680 ± 0.0030 0 $ -6.1986 ^{+ 0.0431 }_{- 0.0478 } $ 

$ -6.5162 ^{+ 0.0103 }_{- 0.0103 } $ 

107Te $ \to {}^{103}{\rm{Nb}} $ 

4.0100 ± 0.0050 0 $ -2.3372 ^{+ 0.0120 }_{- 0.0123 } $ 

$ -2.1065 ^{+ 0.0250 }_{- 0.0250 } $ 

113I $ \to {}^{109}{\rm{Sb}} $ 

2.7070 ± 0.0100 0 $ 7.2997 ^{+ 0.0130 }_{- 0.0134 } $ 

$ 7.5353 ^{+ 0.0939 }_{- 0.0944 } $ 

109Xe $ \to {}^{105}{\rm{Te}} $ 

4.2170 ± 0.0210 0 $ -1.8861 ^{+ 0.0621 }_{- 0.0726 } $ 

$ -1.9885 ^{+ 0.1008 }_{- 0.1016 } $ 

111Xe $ \to {}^{107}{\rm{Te}} $ 

3.7100 ± 0.0600 0 $ 0.8439 ^{+ 0.1039 }_{- 0.1368 } $ 

$ 0.7345 ^{+ 0.3492 }_{- 0.3581 } $ 

145Pm $ \to {}^{141}{\rm{Pr}} $ 

2.3210 ± 0.0290 0 $ 17.2999 ^{+ 0.0097 }_{- 0.0099 } $ 

$ 17.3786 ^{+ 0.3981 }_{- 0.4058 } $ 

147Sm $ \to {}^{143}{\rm{Nd}} $ 

2.3113 ± 0.0150 0 $ 18.5269 ^{+ 0.0020 }_{- 0.0020 } $ 

$ 18.3762 ^{+ 0.2119 }_{- 0.2140 } $ 

147Eu $ \to {}^{143}{\rm{Pm}} $ 

2.9910 ± 0.0005 0 $ 10.9644 ^{+ 0.0107 }_{- 0.0109 } $ 

$ 11.1826 ^{+ 0.0049 }_{- 0.0049 } $ 

149Gd $ \to {}^{145}{\rm{Sm}} $ 

3.0990 ± 0.0030 0 $ 11.2706 ^{+ 0.0047 }_{- 0.0047 } $ 

$ 10.8732 ^{+ 0.0281 }_{- 0.0281 } $ 

151Gd $ \to {}^{147}{\rm{Sm}} $ 

2.6522 ± 0.0029 0 $ 14.9882 ^{+ 0.0035 }_{- 0.0035 } $ 

$ 15.6039 ^{+ 0.0345 }_{- 0.0345 } $ 

151Dy $ \to {}^{147}{\rm{Gd}} $ 

4.1796 ± 0.0026 0 $ 4.2828 ^{+ 0.0072 }_{- 0.0073 } $ 

$ 4.0136 ^{+ 0.0158 }_{- 0.0158 } $ 

153Dy $ \to {}^{149}{\rm{Gd}} $ 

3.5590 ± 0.0040 0 $ 8.3887 ^{+ 0.0067 }_{- 0.0068 } $ 

$ 8.3050 ^{+ 0.0312 }_{- 0.0313 } $ 

151Ho $ \to {}^{147}{\rm{Tb}} $ 

$ ^{\rm{m}} $ 

4.6950 ± 0.0018 0 $ 2.2041 ^{+ 0.0012 }_{- 0.0012 } $ 

$ 1.6715 ^{+ 0.0093 }_{- 0.0093 } $ 

151Ho $ ^{\rm{m}} $ 

$ \to {}^{147}{\rm{Tb}} $ 

4.7400 ± 0.0002 0 $ 1.7875 ^{+ 0.0118 }_{- 0.0121 } $ 

$ 1.4417 ^{+ 0.0010 }_{- 0.0010 } $ 

153Ho $ \to {}^{149}{\rm{Tb}} $ 

$ ^{\rm{m}} $ 

4.0250 ± 0.0040 0 $ 5.3717 ^{+ 0.0064 }_{- 0.0065 } $ 

$ 5.5780 ^{+ 0.0262 }_{- 0.0262 } $ 

153Ho $ ^{\rm{m}} $ 

$ \to {}^{149}{\rm{Tb}} $ 

4.1200 ± 0.0003 0 $ 5.4914 ^{+ 0.0227 }_{- 0.0240 } $ 

$ 4.9667 ^{+ 0.0019 }_{- 0.0019 } $ 

153Er $ \to {}^{149}{\rm{Dy}} $ 

4.8024 ± 0.0014 0 $ 1.8451 ^{+ 0.0023 }_{- 0.0023 } $ 

$ 1.6542 ^{+ 0.0071 }_{- 0.0071 } $ 

155Er $ \to {}^{151}{\rm{Dy}} $ 

4.1180 ± 0.0050 0 $ 6.1600 ^{+ 0.0239 }_{- 0.0253 } $ 

$ 5.5677 ^{+ 0.0321 }_{- 0.0322 } $ 

153Tm $ \to {}^{149}{\rm{Ho}} $ 

5.2483 ± 0.0015 0 $ 0.2112 ^{+ 0.0029 }_{- 0.0029 } $ 

$ 0.0330 ^{+ 0.0067 }_{- 0.0067 } $ 

155Tm $ \to {}^{151}{\rm{Ho}} $ 

4.5720 ± 0.0050 0 $ 3.4154 ^{+ 0.0040 }_{- 0.0040 } $ 

$ 3.4093 ^{+ 0.0277 }_{- 0.0277 } $ 

157Tm $ \to {}^{153}{\rm{Ho}} $ 

$ ^{\rm{m}} $ 

3.8780 ± 0.0280 0 $ 7.4630 ^{+ 0.0106 }_{- 0.0109 } $ 

$ 7.7996 ^{+ 0.1996 }_{- 0.2019 } $ 

153Tm $ ^{\rm{m}} $ 

$ \to {}^{149}{\rm{Ho}} $ 

$ ^{\rm{m}} $ 

5.2400 ± 0.0002 0 $ 0.4342 ^{+ 0.0334 }_{- 0.0362 } $ 

$ 0.0700 ^{+ 0.0009 }_{- 0.0009 } $ 

155Yb $ \to {}^{151}{\rm{Er}} $ 

5.3388 ± 0.0021 0 $ 0.3042 ^{+ 0.0048 }_{- 0.0049 } $ 

$ 0.1230 ^{+ 0.0092 }_{- 0.0093 } $ 

155Lu $ \to {}^{151}{\rm{Tm}} $ 

5.8016 ± 0.0022 0 $ -1.1217 ^{+ 0.0126 }_{- 0.0130 } $ 

$ -1.3425 ^{+ 0.0086 }_{- 0.0086 } $ 

155Lu $ ^{\rm{m}} $ 

$ \to {}^{151}{\rm{Tm}} $ 

$ ^{\rm{m}} $ 

5.7300 ± 0.0040 0 $ -0.7409 ^{+ 0.0274 }_{- 0.0293 } $ 

$ -1.0592 ^{+ 0.0160 }_{- 0.0160 } $ 

157Lu $ ^{\rm{m}} $ 

$ \to {}^{153}{\rm{Tm}} $ 

5.1300 ± 0.0020 0 $ 1.7938 ^{+ 0.0107 }_{- 0.0110 } $ 

$ 1.5764 ^{+ 0.0095 }_{- 0.0095 } $ 

157Hf $ \to {}^{153}{\rm{Yb}} $ 

5.8800 ± 0.0030 0 $ -0.9124 ^{+ 0.0038 }_{- 0.0038 } $ 

$ -1.1911 ^{+ 0.0117 }_{- 0.0117 } $ 

159Hf $ \to {}^{155}{\rm{Yb}} $ 

5.2251 ± 0.0027 0 $ 1.1719 ^{+ 0.0083 }_{- 0.0084 } $ 

$ 1.6282 ^{+ 0.0127 }_{- 0.0127 } $ 

161Hf $ \to {}^{157}{\rm{Yb}} $ 

4.6790 ± 0.0250 0 $ 3.8024 ^{+ 0.0093 }_{- 0.0095 } $ 

$ 4.4473 ^{+ 0.1394 }_{- 0.1406 } $ 

157Ta $ \to {}^{153}{\rm{Lu}} $ 

$ ^{\rm{m}} $ 

6.3550 ± 0.0060 0 $ -1.9820 ^{+ 0.0169 }_{- 0.0175 } $ 

$ -2.5163 ^{+ 0.0209 }_{- 0.0210 } $ 

157Ta $ ^{\rm{m}} $ 

$ \to {}^{153}{\rm{Lu}} $ 

6.3900 ± 0.0050 0 $ -2.3665 ^{+ 0.0100 }_{- 0.0102 } $ 

$ -2.6379 ^{+ 0.0173 }_{- 0.0173 } $ 

159Ta $ \to {}^{155}{\rm{Lu}} $ 

$ ^{\rm{m}} $ 

5.6810 ± 0.0240 0 $ 0.4856 ^{+ 0.0360 }_{- 0.0393 } $ 

$ 0.0744 ^{+ 0.1000 }_{- 0.1006 } $ 

159Ta $ ^{\rm{m}} $ 

$ \to {}^{155}{\rm{Lu}} $ 

5.7500 ± 0.0050 0 $ 0.0078 ^{+ 0.0442 }_{- 0.0492 } $ 

$ -0.2112 ^{+ 0.0205 }_{- 0.0205 } $ 

161Ta $ ^{\rm{m}} $ 

$ \to {}^{157}{\rm{Lu}} $ 

$ ^{\rm{m}} $ 

5.2800 ± 0.0230 0 $ 1.6435 ^{+ 0.0152 }_{- 0.0158 } $ 

$ 1.8681 ^{+ 0.1076 }_{- 0.1084 } $ 

159W $ \to {}^{155}{\rm{Hf}} $ 

6.4510 ± 0.0040 0 $ -2.0862 ^{+ 0.0356 }_{- 0.0388 } $ 

$ -2.4217 ^{+ 0.0138 }_{- 0.0138 } $ 

161W $ \to {}^{157}{\rm{Hf}} $ 

5.9230 ± 0.0040 0 $ -0.2516 ^{+ 0.0167 }_{- 0.0174 } $ 

$ -0.4484 ^{+ 0.0159 }_{- 0.0159 } $ 

163W $ \to {}^{159}{\rm{Hf}} $ 

5.5200 ± 0.0600 0 $ 1.2738 ^{+ 0.0146 }_{- 0.0151 } $ 

$ 1.2610 ^{+ 0.2643 }_{- 0.2689 } $ 

167W $ \to {}^{163}{\rm{Hf}} $ 

4.7510 ± 0.0300 0 $ 4.6968 ^{+ 0.0108 }_{- 0.0111 } $ 

$ 5.1415 ^{+ 0.1682 }_{- 0.1698 } $ 

163Re $ \to {}^{159}{\rm{Ta}} $ 

6.0120 ± 0.0080 0 $ 0.0859 ^{+ 0.0717 }_{- 0.0859 } $ 

$ -0.3462 ^{+ 0.0314 }_{- 0.0315 } $ 

165Re $ \to {}^{161}{\rm{Ta}} $ 

5.6940 ± 0.0060 0 $ 1.0580 ^{+ 0.1383 }_{- 0.2041 } $ 

$ 0.9777 ^{+ 0.0257 }_{- 0.0258 } $ 

Continued on next page Table 3. Same as Table 2 but for the favored α decay of odd-A nuclei. The superscripts "n," "m," or "p" denote excited isomeric states, which are defined as higher states with half-lives exceeding 100 ns. The superscript “p” also presents nonisomeric levels as used in AME2020.
Table 3-continued from previous page α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{\,exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{\,HOPM}} $ 

159Re $ ^{\rm{m}} $ 

$ \to {}^{155}{\rm{Ta}} $ 

6.9700 ± 0.0500 0 $ -3.5740 ^{+ 0.0792 }_{- 0.0969 } $ 

$ -3.7233 ^{+ 0.1539 }_{- 0.1557 } $ 

161Re $ ^{\rm{m}} $ 

$ \to {}^{157}{\rm{Ta}} $ 

$ ^{\rm{m}} $ 

6.4300 ± 0.0013 0 $ -1.8012 ^{+ 0.0088 }_{- 0.0090 } $ 

$ -1.9210 ^{+ 0.0046 }_{- 0.0046 } $ 

163Re $ ^{\rm{m}} $ 

$ \to {}^{159}{\rm{Ta}} $ 

$ ^{\rm{m}} $ 

6.0700 ± 0.0050 0 $ -0.4891 ^{+ 0.0100 }_{- 0.0103 } $ 

$ -0.5726 ^{+ 0.0194 }_{- 0.0194 } $ 

165Re $ ^{\rm{m}} $ 

$ \to {}^{161}{\rm{Ta}} $ 

$ ^{\rm{m}} $ 

5.6600 ± 0.0220 0 $ 1.1266 ^{+ 0.0147 }_{- 0.0152 } $ 

$ 1.1243 ^{+ 0.0950 }_{- 0.0956 } $ 

161Os $ \to {}^{157}{\rm{W}} $ 

7.0690 ± 0.0110 0 $ -3.1938 ^{+ 0.0389 }_{- 0.0428 } $ 

$ -3.6288 ^{+ 0.0338 }_{- 0.0338 } $ 

163Os $ \to {}^{159}{\rm{W}} $ 

6.6730 ± 0.0070 0 $ -2.2441 ^{+ 0.0365 }_{- 0.0399 } $ 

$ -2.3368 ^{+ 0.0236 }_{- 0.0236 } $ 

165Os $ \to {}^{161}{\rm{W}} $ 

6.3350 ± 0.0060 0 $ -1.1030 ^{+ 0.0180 }_{- 0.0187 } $ 

$ -1.1301 ^{+ 0.0220 }_{- 0.0220 } $ 

167Os $ \to {}^{163}{\rm{W}} $ 

5.9800 ± 0.0600 0 $ 0.2162 ^{+ 0.0026 }_{- 0.0026 } $ 

$ 0.2519 ^{+ 0.2397 }_{- 0.2436 } $ 

169Os $ \to {}^{165}{\rm{W}} $ 

5.7130 ± 0.0030 0 $ 1.4024 ^{+ 0.0202 }_{- 0.0212 } $ 

$ 1.3843 ^{+ 0.0130 }_{- 0.0130 } $ 

171Os $ \to {}^{167}{\rm{W}} $ 

5.3710 ± 0.0040 0 $ 2.6638 ^{+ 0.0103 }_{- 0.0106 } $ 

$ 2.9577 ^{+ 0.0191 }_{- 0.0191 } $ 

173Os $ \to {}^{169}{\rm{W}} $ 

5.0550 ± 0.0060 0 $ 3.7482 ^{+ 0.0171 }_{- 0.0178 } $ 

$ 4.5595 ^{+ 0.0315 }_{- 0.0316 } $ 

167Ir $ \to {}^{163}{\rm{Re}} $ 

6.5049 ± 0.0026 0 $ -1.1716 ^{+ 0.0088 }_{- 0.0090 } $ 

$ -1.3141 ^{+ 0.0093 }_{- 0.0093 } $ 

169Ir $ \to {}^{165}{\rm{Re}} $ 

6.1410 ± 0.0040 0 $ -0.1765 ^{+ 0.0049 }_{- 0.0049 } $ 

$ 0.0635 ^{+ 0.0157 }_{- 0.0157 } $ 

171Ir $ \to {}^{167}{\rm{Re}} $ 

$ ^{\rm{m}} $ 

5.9970 ± 0.0120 0 $ 1.3153 ^{+ 0.0401 }_{- 0.0442 } $ 

$ 0.6552 ^{+ 0.0487 }_{- 0.0489 } $ 

173Ir $ \to {}^{169}{\rm{Re}} $ 

$ ^{\rm{m}} $ 

5.7160 ± 0.0090 0 $ 2.4102 ^{+ 0.0370 }_{- 0.0404 } $ 

$ 1.8601 ^{+ 0.0395 }_{- 0.0396 } $ 

177Ir $ \to {}^{173}{\rm{Re}} $ 

5.0800 ± 0.0300 0 $ 4.6961 ^{+ 0.0241 }_{- 0.0255 } $ 

$ 4.9612 ^{+ 0.1582 }_{- 0.1597 } $ 

165Ir $ ^{\rm{m}} $ 

$ \to {}^{161}{\rm{Re}} $ 

$ ^{\rm{m}} $ 

6.8800 ± 0.0500 0 $ -2.5673 ^{+ 0.0389 }_{- 0.0428 } $ 

$ -2.6105 ^{+ 0.1620 }_{- 0.1639 } $ 

167Ir $ ^{\rm{m}} $ 

$ \to {}^{163}{\rm{Re}} $ 

$ ^{\rm{m}} $ 

6.5600 ± 0.0021 0 $ -1.4945 ^{+ 0.0076 }_{- 0.0077 } $ 

$ -1.5095 ^{+ 0.0074 }_{- 0.0074 } $ 

169Ir $ ^{\rm{m}} $ 

$ \to {}^{165}{\rm{Re}} $ 

$ ^{\rm{m}} $ 

6.2700 ± 0.0220 0 $ -0.4505 ^{+ 0.0015 }_{- 0.0016 } $ 

$ -0.4334 ^{+ 0.0831 }_{- 0.0836 } $ 

165Pt $ \to {}^{161}{\rm{Os}} $ 

7.4530 ± 0.0140 0 $ -3.4318 ^{+ 0.1722 }_{- 0.2894 } $ 

$ -3.9938 ^{+ 0.0406 }_{- 0.0407 } $ 

167Pt $ \to {}^{163}{\rm{Os}} $ 

7.1600 ± 0.0600 0 $ -3.0386 ^{+ 0.0548 }_{- 0.0627 } $ 

$ -3.0981 ^{+ 0.1847 }_{- 0.1873 } $ 

169Pt $ \to {}^{165}{\rm{Os}} $ 

6.8580 ± 0.0050 0 $ -2.1555 ^{+ 0.0056 }_{- 0.0056 } $ 

$ -2.1118 ^{+ 0.0166 }_{- 0.0166 } $ 

171Pt $ \to {}^{167}{\rm{Os}} $ 

6.6070 ± 0.0030 0 $ -1.2765 ^{+ 0.0232 }_{- 0.0245 } $ 

$ -1.2348 ^{+ 0.0106 }_{- 0.0106 } $ 

173Pt $ \to {}^{169}{\rm{Os}} $ 

6.3600 ± 0.0600 0 $ -0.3524 ^{+ 0.0023 }_{- 0.0023 } $ 

$ -0.3183 ^{+ 0.2237 }_{- 0.2272 } $ 

177Pt $ \to {}^{173}{\rm{Os}} $ 

5.6429 ± 0.0027 0 $ 2.2441 ^{+ 0.0170 }_{- 0.0177 } $ 

$ 2.6791 ^{+ 0.0123 }_{- 0.0123 } $ 

181Pt $ \to {}^{177}{\rm{Os}} $ 

5.1500 ± 0.0050 0 $ 4.8468 ^{+ 0.0180 }_{- 0.0188 } $ 

$ 5.1229 ^{+ 0.0263 }_{- 0.0263 } $ 

183Pt $ \to {}^{179}{\rm{Os}} $ 

4.8220 ± 0.0090 0 $ 6.6088 ^{+ 0.0621 }_{- 0.0726 } $ 

$ 6.9559 ^{+ 0.0524 }_{- 0.0526 } $ 

185Pt $ \to {}^{181}{\rm{Os}} $ 

$ ^{\rm{n}} $ 

4.2800 ± 0.0100 0 $ 7.9298 ^{+ 0.0145 }_{- 0.0150 } $ 

$ 10.4418 ^{+ 0.0701 }_{- 0.0704 } $ 

173Au $ \to {}^{169}{\rm{Ir}} $ 

6.8360 ± 0.0050 0 $ -1.5280 ^{+ 0.0134 }_{- 0.0138 } $ 

$ -1.6094 ^{+ 0.0170 }_{- 0.0170 } $ 

175Au $ \to {}^{171}{\rm{Ir}} $ 

6.5834 ± 0.0027 0 $ -0.6435 ^{+ 0.0065 }_{- 0.0066 } $ 

$ -0.7096 ^{+ 0.0097 }_{- 0.0097 } $ 

177Au $ \to {}^{173}{\rm{Ir}} $ 

6.2980 ± 0.0040 0 $ 0.5743 ^{+ 0.0057 }_{- 0.0058 } $ 

$ 0.3731 ^{+ 0.0155 }_{- 0.0155 } $ 

179Au $ \to {}^{175}{\rm{Ir}} $ 

5.9810 ± 0.0050 0 $ 1.5088 ^{+ 0.0180 }_{- 0.0187 } $ 

$ 1.6687 ^{+ 0.0210 }_{- 0.0210 } $ 

181Au $ \to {}^{177}{\rm{Ir}} $ 

5.7514 ± 0.0029 0 $ 2.7054 ^{+ 0.0423 }_{- 0.0468 } $ 

$ 2.6814 ^{+ 0.0130 }_{- 0.0130 } $ 

183Au $ \to {}^{179}{\rm{Ir}} $ 

5.4653 ± 0.0029 0 $ 3.8911 ^{+ 0.0100 }_{- 0.0103 } $ 

$ 4.0316 ^{+ 0.0141 }_{- 0.0141 } $ 

185Au $ \to {}^{181}{\rm{Ir}} $ 

5.1080 ± 0.0050 0 $ 4.9916 ^{+ 0.0061 }_{- 0.0062 } $ 

$ 5.8778 ^{+ 0.0270 }_{- 0.0270 } $ 

171Au $ ^{\rm{m}} $ 

$ \to {}^{167}{\rm{Ir}} $ 

$ ^{\rm{m}} $ 

7.1600 ± 0.0100 0 $ -2.7628 ^{+ 0.0067 }_{- 0.0068 } $ 

$ -2.6843 ^{+ 0.0314 }_{- 0.0315 } $ 

173Au $ ^{\rm{m}} $ 

$ \to {}^{169}{\rm{Ir}} $ 

$ ^{\rm{m}} $ 

6.9000 ± 0.0210 0 $ -1.8630 ^{+ 0.0035 }_{- 0.0036 } $ 

$ -1.8249 ^{+ 0.0700 }_{- 0.0703 } $ 

175Au $ ^{\rm{m}} $ 

$ \to {}^{171}{\rm{Ir}} $ 

$ ^{\rm{m}} $ 

6.5900 ± 0.0110 0 $ -0.7415 ^{+ 0.0032 }_{- 0.0032 } $ 

$ -0.7334 ^{+ 0.0395 }_{- 0.0396 } $ 

177Au $ ^{\rm{m}} $ 

$ \to {}^{173}{\rm{Ir}} $ 

$ ^{\rm{m}} $ 

6.2600 ± 0.0070 0 $ 0.2985 ^{+ 0.0049 }_{- 0.0050 } $ 

$ 0.5209 ^{+ 0.0273 }_{- 0.0274 } $ 

173Hg $ \to {}^{169}{\rm{Pt}} $ 

7.3780 ± 0.0040 0 $ -3.0969 ^{+ 0.0414 }_{- 0.0458 } $ 

$ -2.9648 ^{+ 0.0122 }_{- 0.0122 } $ 

175Hg $ \to {}^{171}{\rm{Pt}} $ 

7.0720 ± 0.0050 0 $ -1.9914 ^{+ 0.0126 }_{- 0.0130 } $ 

$ -1.9858 ^{+ 0.0163 }_{- 0.0163 } $ 

Continued on next page Table 3-continued from previous page α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{\,exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{\,HOPM}} $ 

179Hg $ \to {}^{175}{\rm{Pt}} $ 

6.3500 ± 0.0300 0 $ 0.1461 ^{+ 0.0122 }_{- 0.0126 } $ 

$ 0.6115 ^{+ 0.1158 }_{- 0.1167 } $ 

183Hg $ \to {}^{179}{\rm{Pt}} $ 

6.0390 ± 0.0040 0 $ 1.9049 ^{+ 0.0312 }_{- 0.0336 } $ 

$ 1.8988 ^{+ 0.0168 }_{- 0.0168 } $ 

185Hg $ \to {}^{181}{\rm{Pt}} $ 

5.7730 ± 0.0040 0 $ 2.9129 ^{+ 0.0088 }_{- 0.0089 } $ 

$ 3.0732 ^{+ 0.0180 }_{- 0.0181 } $ 

177Tl $ \to {}^{173}{\rm{Au}} $ 

7.0670 ± 0.0070 0 $ -1.6081 ^{+ 0.1065 }_{- 0.1413 } $ 

$ -1.5657 ^{+ 0.0231 }_{- 0.0232 } $ 

179Tl $ \to {}^{175}{\rm{Au}} $ 

6.7091 ± 0.0026 0 $ -0.1377 ^{+ 0.0089 }_{- 0.0090 } $ 

$ -0.3152 ^{+ 0.0093 }_{- 0.0094 } $ 

181Tl $ \to {}^{177}{\rm{Au}} $ 

6.3220 ± 0.0040 0 $ 1.5279 ^{+ 0.0147 }_{- 0.0152 } $ 

$ 1.1615 ^{+ 0.0158 }_{- 0.0158 } $ 

177Tl $ ^{\rm{m}} $ 

$ \to {}^{173}{\rm{Au}} $ 

$ ^{\rm{m}} $ 

7.6600 ± 0.0180 0 $ -3.3285 ^{+ 0.0696 }_{- 0.0830 } $ 

$ -3.4017 ^{+ 0.0521 }_{- 0.0523 } $ 

179Tl $ ^{\rm{m}} $ 

$ \to {}^{175}{\rm{Au}} $ 

$ ^{\rm{m}} $ 

7.3700 ± 0.0100 0 $ -2.8508 ^{+ 0.0061 }_{- 0.0062 } $ 

$ -2.5178 ^{+ 0.0309 }_{- 0.0309 } $ 

185Pb $ ^{\rm{m}} $ 

$ \to {}^{181}{\rm{Hg}} $ 

$ ^{\rm{m}} $ 

6.5600 ± 0.0500 0 $ 0.9106 ^{+ 0.0157 }_{- 0.0163 } $ 

$ 0.6931 ^{+ 0.1879 }_{- 0.1902 } $ 

187Pb $ ^{\rm{m}} $ 

$ \to {}^{183}{\rm{Hg}} $ 

$ ^{\rm{m}} $ 

6.2100 ± 0.0100 0 $ 2.1833 ^{+ 0.0071 }_{- 0.0072 } $ 

$ 2.0922 ^{+ 0.0412 }_{- 0.0413 } $ 

189Pb $ ^{\rm{m}} $ 

$ \to {}^{185}{\rm{Hg}} $ 

$ ^{\rm{m}} $ 

5.8500 ± 0.0040 0 $ 4.1012 ^{+ 0.0177 }_{- 0.0184 } $ 

$ 3.6672 ^{+ 0.0182 }_{- 0.0182 } $ 

191Pb $ ^{\rm{m}} $ 

$ \to {}^{187}{\rm{Hg}} $ 

$ ^{\rm{m}} $ 

5.4000 ± 0.0100 0 $ 5.8156 ^{+ 0.0157 }_{- 0.0162 } $ 

$ 5.8605 ^{+ 0.0515 }_{- 0.0516 } $ 

185Bi $ ^{\rm{m}} $ 

$ \to {}^{181}{\rm{Tl}} $ 

8.2200 ± 0.0800 0 $ -3.2366 ^{+ 0.0290 }_{- 0.0310 } $ 

$ -4.1870 ^{+ 0.2109 }_{- 0.2143 } $ 

187Bi $ ^{\rm{m}} $ 

$ \to {}^{183}{\rm{Tl}} $ 

7.8900 ± 0.0080 0 $ -3.4318 ^{+ 0.0229 }_{- 0.0241 } $ 

$ -3.2649 ^{+ 0.0227 }_{- 0.0228 } $ 

189Bi $ ^{\rm{m}} $ 

$ \to {}^{185}{\rm{Tl}} $ 

7.4500 ± 0.0050 0 $ -2.2201 ^{+ 0.0086 }_{- 0.0088 } $ 

$ -1.9388 ^{+ 0.0156 }_{- 0.0156 } $ 

191Bi $ \to {}^{187}{\rm{Tl}} $ 

$ ^{\rm{m}} $ 

6.4500 ± 0.0030 0 $ 1.3859 ^{+ 0.0104 }_{- 0.0106 } $ 

$ 1.5833 ^{+ 0.0118 }_{- 0.0118 } $ 

191Bi $ ^{\rm{m}} $ 

$ \to {}^{187}{\rm{Tl}} $ 

7.0200 ± 0.0250 0 $ -0.7356 ^{+ 0.0269 }_{- 0.0287 } $ 

$ -0.5146 ^{+ 0.0857 }_{- 0.0862 } $ 

193Bi $ \to {}^{189}{\rm{Tl}} $ 

$ ^{\rm{m}} $ 

6.0200 ± 0.0050 0 $ 3.2594 ^{+ 0.0200 }_{- 0.0210 } $ 

$ 3.3889 ^{+ 0.0220 }_{- 0.0220 } $ 

193Bi $ ^{\rm{m}} $ 

$ \to {}^{189}{\rm{Tl}} $ 

6.6100 ± 0.0060 0 $ 0.5809 ^{+ 0.0186 }_{- 0.0194 } $ 

$ 0.9806 ^{+ 0.0227 }_{- 0.0228 } $ 

195Bi $ \to {}^{191}{\rm{Tl}} $ 

$ ^{\rm{m}} $ 

5.5400 ± 0.0050 0 $ 5.7853 ^{+ 0.0094 }_{- 0.0096 } $ 

$ 5.6596 ^{+ 0.0251 }_{- 0.0251 } $ 

195Bi $ ^{\rm{m}} $ 

$ \to {}^{191}{\rm{Tl}} $ 

6.2300 ± 0.0060 0 $ 2.4210 ^{+ 0.0050 }_{- 0.0050 } $ 

$ 2.5056 ^{+ 0.0250 }_{- 0.0250 } $ 

197Bi $ ^{\rm{m}} $ 

$ \to {}^{193}{\rm{Tl}} $ 

5.9000 ± 0.0120 0 $ 2.7402 ^{+ 0.0136 }_{- 0.0140 } $ 

$ 3.9566 ^{+ 0.0544 }_{- 0.0546 } $ 

191Po $ \to {}^{187}{\rm{Pb}} $ 

7.4930 ± 0.0050 0 $ -1.6576 ^{+ 0.0193 }_{- 0.0202 } $ 

$ -1.6837 ^{+ 0.0157 }_{- 0.0157 } $ 

193Po $ \to {}^{189}{\rm{Pb}} $ 

7.0940 ± 0.0040 0 $ -0.3990 ^{+ 0.0355 }_{- 0.0387 } $ 

$ -0.3607 ^{+ 0.0137 }_{- 0.0137 } $ 

195Po $ \to {}^{191}{\rm{Pb}} $ 

6.7497 ± 0.0028 0 $ 0.6934 ^{+ 0.0083 }_{- 0.0085 } $ 

$ 0.8827 ^{+ 0.0104 }_{- 0.0104 } $ 

197Po $ \to {}^{193}{\rm{Pb}} $ 

6.4110 ± 0.0030 0 $ 2.0857 ^{+ 0.0072 }_{- 0.0074 } $ 

$ 2.2086 ^{+ 0.0121 }_{- 0.0121 } $ 

199Po $ \to {}^{195}{\rm{Pb}} $ 

6.0743 ± 0.0019 0 $ 3.6411 ^{+ 0.0117 }_{- 0.0121 } $ 

$ 3.6414 ^{+ 0.0083 }_{- 0.0084 } $ 

201Po $ \to {}^{197}{\rm{Pb}} $ 

5.7993 ± 0.0017 0 $ 4.9182 ^{+ 0.0028 }_{- 0.0028 } $ 

$ 4.9107 ^{+ 0.0080 }_{- 0.0080 } $ 

205Po $ \to {}^{201}{\rm{Pb}} $ 

5.3250 ± 0.0100 0 $ 7.1948 ^{+ 0.0195 }_{- 0.0204 } $ 

$ 7.3415 ^{+ 0.0541 }_{- 0.0542 } $ 

207Po $ \to {}^{203}{\rm{Pb}} $ 

5.2159 ± 0.0025 0 $ 7.9975 ^{+ 0.0015 }_{- 0.0015 } $ 

$ 7.9571 ^{+ 0.0140 }_{- 0.0140 } $ 

209Po $ \to {}^{205}{\rm{Pb}} $ 

$ ^{\rm{m}} $ 

4.9800 ± 0.0014 0 $ 9.5945 ^{+ 0.0104 }_{- 0.0106 } $ 

$ 9.3406 ^{+ 0.0084 }_{- 0.0084 } $ 

213Po $ \to {}^{209}{\rm{Pb}} $ 

8.5361 ± 0.0026 0 $ -5.4312 ^{+ 0.0001 }_{- 0.0001 } $ 

$ -5.8339 ^{+ 0.0065 }_{- 0.0065 } $ 

215Po $ \to {}^{211}{\rm{Pb}} $ 

7.5263 ± 0.0008 0 $ -2.7493 ^{+ 0.0012 }_{- 0.0012 } $ 

$ -3.0161 ^{+ 0.0025 }_{- 0.0025 } $ 

217Po $ \to {}^{213}{\rm{Pb}} $ 

6.6621 ± 0.0024 0 $ 0.1957 ^{+ 0.0140 }_{- 0.0144 } $ 

$ -0.0592 ^{+ 0.0090 }_{- 0.0090 } $ 

219Po $ \to {}^{215}{\rm{Pb}} $ 

5.9100 ± 0.0500 0 $ 3.3407 ^{+ 0.0402 }_{- 0.0444 } $ 

$ 3.0699 ^{+ 0.2266 }_{- 0.2297 } $ 

187Po $ ^{\rm{m}} $ 

$ \to {}^{183}{\rm{Pb}} $ 

$ ^{\rm{m}} $ 

7.8900 ± 0.0270 0 $ -3.3010 ^{+ 0.0000 }_{- 0.0000 } $ 

$ -2.9076 ^{+ 0.0776 }_{- 0.0780 } $ 

191Po $ ^{\rm{m}} $ 

$ \to {}^{187}{\rm{Pb}} $ 

$ ^{\rm{m}} $ 

7.5400 ± 0.0110 0 $ -1.0315 ^{+ 0.0138 }_{- 0.0142 } $ 

$ -1.8304 ^{+ 0.0341 }_{- 0.0342 } $ 

193Po $ ^{\rm{m}} $ 

$ \to {}^{189}{\rm{Pb}} $ 

$ ^{\rm{m}} $ 

7.1500 ± 0.0060 0 $ -0.6108 ^{+ 0.0191 }_{- 0.0200 } $ 

$ -0.5513 ^{+ 0.0203 }_{- 0.0203 } $ 

195Po $ ^{\rm{m}} $ 

$ \to {}^{191}{\rm{Pb}} $ 

$ ^{\rm{m}} $ 

6.8411 ± 0.0090 0 $ 0.2833 ^{+ 0.0045 }_{- 0.0045 } $ 

$ 0.5470 ^{+ 0.0327 }_{- 0.0327 } $ 

197Po $ ^{\rm{m}} $ 

$ \to {}^{193}{\rm{Pb}} $ 

$ ^{\rm{m}} $ 

6.5200 ± 0.0120 0 $ 1.4873 ^{+ 0.0017 }_{- 0.0017 } $ 

$ 1.7749 ^{+ 0.0470 }_{- 0.0472 } $ 

199Po $ ^{\rm{m}} $ 

$ \to {}^{195}{\rm{Pb}} $ 

$ ^{\rm{m}} $ 

6.1800 ± 0.0027 0 $ 3.0181 ^{+ 0.0052 }_{- 0.0052 } $ 

$ 3.1832 ^{+ 0.0115 }_{- 0.0115 } $ 

Continued on next page Table 3-continued from previous page α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{\,exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{\,HOPM}} $ 

201Po $ ^{\rm{m}} $ 

$ \to {}^{197}{\rm{Pb}} $ 

$ ^{\rm{m}} $ 

5.9000 ± 0.0024 0 $ 4.3370 ^{+ 0.0060 }_{- 0.0060 } $ 

$ 4.4408 ^{+ 0.0110 }_{- 0.0111 } $ 

191At $ \to {}^{187}{\rm{Bi}} $ 

$ ^{\rm{m}} $ 

7.8220 ± 0.0140 0 $ -2.6778 ^{+ 0.1402 }_{- 0.2083 } $ 

$ -2.3187 ^{+ 0.0414 }_{- 0.0415 } $ 

193At $ \to {}^{189}{\rm{Bi}} $ 

$ ^{\rm{m}} $ 

7.5720 ± 0.0070 0 $ -1.5376 ^{+ 0.0691 }_{- 0.0822 } $ 

$ -1.5429 ^{+ 0.0219 }_{- 0.0219 } $ 

195At $ \to {}^{191}{\rm{Bi}} $ 

$ ^{\rm{m}} $ 

7.3440 ± 0.0060 0 $ -0.5376 ^{+ 0.0290 }_{- 0.0310 } $ 

$ -0.7975 ^{+ 0.0197 }_{- 0.0197 } $ 

197At $ \to {}^{193}{\rm{Bi}} $ 

7.1040 ± 0.0030 0 $ -0.3937 ^{+ 0.0062 }_{- 0.0063 } $ 

$ 0.0271 ^{+ 0.0104 }_{- 0.0104 } $ 

199At $ \to {}^{195}{\rm{Bi}} $ 

6.7773 ± 0.0012 0 $ 0.8969 ^{+ 0.0074 }_{- 0.0075 } $ 

$ 1.2181 ^{+ 0.0045 }_{- 0.0045 } $ 

201At $ \to {}^{197}{\rm{Bi}} $ 

6.4728 ± 0.0016 0 $ 2.0792 ^{+ 0.0081 }_{- 0.0082 } $ 

$ 2.4142 ^{+ 0.0064 }_{- 0.0064 } $ 

203At $ \to {}^{199}{\rm{Bi}} $ 

6.2101 ± 0.0008 0 $ 3.1560 ^{+ 0.0116 }_{- 0.0119 } $ 

$ 3.5221 ^{+ 0.0034 }_{- 0.0034 } $ 

205At $ \to {}^{201}{\rm{Bi}} $ 

6.0196 ± 0.0017 0 $ 4.2079 ^{+ 0.0129 }_{- 0.0133 } $ 

$ 4.3768 ^{+ 0.0077 }_{- 0.0077 } $ 

207At $ \to {}^{203}{\rm{Bi}} $ 

5.8720 ± 0.0030 0 $ 4.8140 ^{+ 0.0071 }_{- 0.0073 } $ 

$ 5.0719 ^{+ 0.0141 }_{- 0.0141 } $ 

209At $ \to {}^{205}{\rm{Bi}} $ 

5.7511 ± 0.0020 0 $ 5.6992 ^{+ 0.0040 }_{- 0.0040 } $ 

$ 5.6645 ^{+ 0.0097 }_{- 0.0097 } $ 

211At $ \to {}^{207}{\rm{Bi}} $ 

5.9824 ± 0.0013 0 $ 4.7933 ^{+ 0.0004 }_{- 0.0004 } $ 

$ 4.5897 ^{+ 0.0059 }_{- 0.0059 } $ 

213At $ \to {}^{209}{\rm{Bi}} $ 

9.2540 ± 0.0050 0 $ -6.9031 ^{+ 0.0204 }_{- 0.0214 } $ 

$ -7.2094 ^{+ 0.0111 }_{- 0.0111 } $ 

215At $ \to {}^{211}{\rm{Bi}} $ 

8.1718 ± 0.0040 0 $ -4.4318 ^{+ 0.0339 }_{- 0.0367 } $ 

$ -4.5373 ^{+ 0.0109 }_{- 0.0109 } $ 

217At $ \to {}^{213}{\rm{Bi}} $ 

7.2014 ± 0.0012 0 $ -1.4867 ^{+ 0.0040 }_{- 0.0040 } $ 

$ -1.5864 ^{+ 0.0040 }_{- 0.0040 } $ 

219At $ \to {}^{215}{\rm{Bi}} $ 

6.3420 ± 0.0050 0 $ 1.7769 ^{+ 0.0227 }_{- 0.0239 } $ 

$ 1.6271 ^{+ 0.0206 }_{- 0.0207 } $ 

193At $ ^{\rm{n}} $ 

$ \to {}^{189}{\rm{Bi}} $ 

$ ^{\rm{n}} $ 

7.2600 ± 0.0090 0 $ -0.9331 ^{+ 0.0580 }_{- 0.0669 } $ 

$ -0.5345 ^{+ 0.0301 }_{- 0.0301 } $ 

197At $ ^{\rm{m}} $ 

$ \to {}^{193}{\rm{Bi}} $ 

$ ^{\rm{m}} $ 

6.8400 ± 0.0080 0 $ 0.3010 ^{+ 0.0414 }_{- 0.0458 } $ 

$ 0.9702 ^{+ 0.0294 }_{- 0.0295 } $ 

199At $ ^{\rm{m}} $ 

$ \to {}^{195}{\rm{Bi}} $ 

$ ^{\rm{m}} $ 

6.6200 ± 0.0010 0 $ 1.4362 ^{+ 0.0141 }_{- 0.0146 } $ 

$ 1.8173 ^{+ 0.0039 }_{- 0.0039 } $ 

195Rn $ \to {}^{191}{\rm{Po}} $ 

7.6900 ± 0.0500 0 $ -2.1549 ^{+ 0.1549 }_{- 0.2430 } $ 

$ -1.5243 ^{+ 0.1535 }_{- 0.1552 } $ 

197Rn $ \to {}^{193}{\rm{Po}} $ 

7.4110 ± 0.0070 0 $ -1.2676 ^{+ 0.0458 }_{- 0.0512 } $ 

$ -0.6216 ^{+ 0.0229 }_{- 0.0230 } $ 

199Rn $ \to {}^{195}{\rm{Po}} $ 

7.1320 ± 0.0040 0 $ -0.2291 ^{+ 0.0215 }_{- 0.0227 } $ 

$ 0.3370 ^{+ 0.0139 }_{- 0.0140 } $ 

203Rn $ \to {}^{199}{\rm{Po}} $ 

6.6299 ± 0.0021 0 $ 1.8259 ^{+ 0.0154 }_{- 0.0160 } $ 

$ 2.2247 ^{+ 0.0082 }_{- 0.0082 } $ 

207Rn $ \to {}^{203}{\rm{Po}} $ 

6.2512 ± 0.0016 0 $ 3.4221 ^{+ 0.0079 }_{- 0.0081 } $ 

$ 3.8120 ^{+ 0.0069 }_{- 0.0069 } $ 

209Rn $ \to {}^{205}{\rm{Po}} $ 

6.2607 ± 0.0020 0 $ 4.0071 ^{+ 0.0148 }_{- 0.0153 } $ 

$ 3.7859 ^{+ 0.0086 }_{- 0.0086 } $ 

215Rn $ \to {}^{211}{\rm{Po}} $ 

8.8390 ± 0.0060 0 $ -5.6383 ^{+ 0.0185 }_{- 0.0193 } $ 

$ -5.9255 ^{+ 0.0145 }_{- 0.0146 } $ 

217Rn $ \to {}^{213}{\rm{Po}} $ 

7.8872 ± 0.0029 0 $ -3.2269 ^{+ 0.0270 }_{- 0.0288 } $ 

$ -3.3745 ^{+ 0.0085 }_{- 0.0085 } $ 

195Rn $ ^{\rm{m}} $ 

$ \to {}^{191}{\rm{Po}} $ 

$ ^{\rm{m}} $ 

7.7100 ± 0.0500 0 $ -2.2218 ^{+ 0.1761 }_{- 0.3010 } $ 

$ -1.5859 ^{+ 0.1529 }_{- 0.1545 } $ 

197Rn $ ^{\rm{m}} $ 

$ \to {}^{193}{\rm{Po}} $ 

$ ^{\rm{m}} $ 

7.5100 ± 0.0110 0 $ -1.5918 ^{+ 0.0405 }_{- 0.0446 } $ 

$ -0.9427 ^{+ 0.0352 }_{- 0.0353 } $ 

199Rn $ ^{\rm{m}} $ 

$ \to {}^{195}{\rm{Po}} $ 

$ ^{\rm{m}} $ 

7.2000 ± 0.0110 0 $ -0.5086 ^{+ 0.0272 }_{- 0.0290 } $ 

$ 0.1016 ^{+ 0.0377 }_{- 0.0378 } $ 

203Rn $ ^{\rm{m}} $ 

$ \to {}^{199}{\rm{Po}} $ 

$ ^{\rm{m}} $ 

6.6800 ± 0.0040 0 $ 1.5547 ^{+ 0.0080 }_{- 0.0081 } $ 

$ 2.0293 ^{+ 0.0155 }_{- 0.0155 } $ 

197Fr $ \to {}^{193}{\rm{At}} $ 

$ ^{\rm{m}} $ 

7.9000 ± 0.0500 0 $ -2.6383 ^{+ 0.2615 }_{- 0.7597 } $ 

$ -1.7832 ^{+ 0.1489 }_{- 0.1504 } $ 

199Fr $ \to {}^{195}{\rm{At}} $ 

7.8170 ± 0.0100 0 $ -2.1805 ^{+ 0.1249 }_{- 0.1761 } $ 

$ -1.5175 ^{+ 0.0304 }_{- 0.0305 } $ 

201Fr $ \to {}^{197}{\rm{At}} $ 

7.5190 ± 0.0040 0 $ -1.2020 ^{+ 0.0129 }_{- 0.0133 } $ 

$ -0.5657 ^{+ 0.0130 }_{- 0.0130 } $ 

203Fr $ \to {}^{199}{\rm{At}} $ 

7.2750 ± 0.0040 0 $ -0.2596 ^{+ 0.0078 }_{- 0.0080 } $ 

$ 0.2624 ^{+ 0.0137 }_{- 0.0137 } $ 

205Fr $ \to {}^{201}{\rm{At}} $ 

7.0547 ± 0.0024 0 $ 0.5976 ^{+ 0.0077 }_{- 0.0079 } $ 

$ 1.0503 ^{+ 0.0086 }_{- 0.0086 } $ 

207Fr $ \to {}^{203}{\rm{At}} $ 

6.8890 ± 0.0200 0 $ 1.1925 ^{+ 0.0029 }_{- 0.0029 } $ 

$ 1.6728 ^{+ 0.0746 }_{- 0.0749 } $ 

209Fr $ \to {}^{205}{\rm{At}} $ 

6.7850 ± 0.0040 0 $ 1.7539 ^{+ 0.0060 }_{- 0.0061 } $ 

$ 2.0810 ^{+ 0.0153 }_{- 0.0153 } $ 

211Fr $ \to {}^{207}{\rm{At}} $ 

6.6620 ± 0.0030 0 $ 2.3300 ^{+ 0.0028 }_{- 0.0028 } $ 

$ 2.5737 ^{+ 0.0118 }_{- 0.0118 } $ 

213Fr $ \to {}^{209}{\rm{At}} $ 

6.9047 ± 0.0013 0 $ 1.5357 ^{+ 0.0008 }_{- 0.0008 } $ 

$ 1.6573 ^{+ 0.0048 }_{- 0.0048 } $ 

215Fr $ \to {}^{211}{\rm{At}} $ 

9.5400 ± 0.0070 0 $ -7.0458 ^{+ 0.0189 }_{- 0.0197 } $ 

$ -7.2234 ^{+ 0.0151 }_{- 0.0151 } $ 

Continued on next page Table 3-continued from previous page α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{\,exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{\,HOPM}} $ 

217Fr $ \to {}^{213}{\rm{At}} $ 

8.4690 ± 0.0040 0 $ -4.6576 ^{+ 0.0889 }_{- 0.1120 } $ 

$ -4.6529 ^{+ 0.0106 }_{- 0.0106 } $ 

219Fr $ \to {}^{215}{\rm{At}} $ 

7.4486 ± 0.0018 0 $ -1.6478 ^{+ 0.0316 }_{- 0.0341 } $ 

$ -1.6445 ^{+ 0.0059 }_{- 0.0059 } $ 

199Fr $ ^{\rm{m}} $ 

$ \to {}^{195}{\rm{At}} $ 

$ ^{\rm{m}} $ 

7.8300 ± 0.0130 0 $ -2.1871 ^{+ 0.0563 }_{- 0.0647 } $ 

$ -1.5570 ^{+ 0.0394 }_{- 0.0395 } $ 

199Fr $ ^{\rm{n}} $ 

$ \to {}^{195}{\rm{At}} $ 

$ ^{\rm{p}} $ 

7.9700 ± 0.0500 0 $ -2.6576 ^{+ 0.1891 }_{- 0.3424 } $ 

$ -1.9761 ^{+ 0.1468 }_{- 0.1483 } $ 

201Fr $ ^{\rm{m}} $ 

$ \to {}^{197}{\rm{At}} $ 

$ ^{\rm{m}} $ 

7.6000 ± 0.0100 0 $ -1.6198 ^{+ 0.0969 }_{- 0.1249 } $ 

$ -0.8262 ^{+ 0.0319 }_{- 0.0319 } $ 

203Fr $ ^{\rm{m}} $ 

$ \to {}^{199}{\rm{At}} $ 

$ ^{\rm{m}} $ 

7.3900 ± 0.0060 0 $ -0.6676 ^{+ 0.0386 }_{- 0.0424 } $ 

$ -0.1263 ^{+ 0.0200 }_{- 0.0200 } $ 

201Ra $ \to {}^{197}{\rm{Rn}} $ 

8.0020 ± 0.0120 0 $ -1.6990 ^{+ 0.3979 }_{- 0.3010 } $ 

$ -1.6978 ^{+ 0.0356 }_{- 0.0357 } $ 

203Ra $ \to {}^{199}{\rm{Rn}} $ 

7.7360 ± 0.0060 0 $ -1.4437 ^{+ 0.1339 }_{- 0.1946 } $ 

$ -0.8708 ^{+ 0.0188 }_{- 0.0188 } $ 

205Ra $ \to {}^{201}{\rm{Rn}} $ 

7.4860 ± 0.0200 0 $ -0.6576 ^{+ 0.0889 }_{- 0.1120 } $ 

$ -0.0501 ^{+ 0.0661 }_{- 0.0664 } $ 

209Ra $ \to {}^{205}{\rm{Rn}} $ 

7.2730 ± 0.0027 0 $ 0.6730 ^{+ 0.0073 }_{- 0.0074 } $ 

$ 0.7012 ^{+ 0.0094 }_{- 0.0094 } $ 

211Ra $ \to {}^{207}{\rm{Rn}} $ 

7.0417 ± 0.0029 0 $ 1.1004 ^{+ 0.0395 }_{- 0.0435 } $ 

$ 1.5394 ^{+ 0.0106 }_{- 0.0106 } $ 

217Ra $ \to {}^{213}{\rm{Rn}} $ 

9.1610 ± 0.0060 0 $ -5.7100 ^{+ 0.0259 }_{- 0.0276 } $ 

$ -6.0555 ^{+ 0.0141 }_{- 0.0141 } $ 

201Ra $ ^{\rm{m}} $ 

$ \to {}^{197}{\rm{Rn}} $ 

$ ^{\rm{m}} $ 

8.0700 ± 0.0260 0 $ -2.2218 ^{+ 0.2632 }_{- 0.7782 } $ 

$ -1.8984 ^{+ 0.0760 }_{- 0.0764 } $ 

203Ra $ ^{\rm{m}} $ 

$ \to {}^{199}{\rm{Rn}} $ 

$ ^{\rm{m}} $ 

7.7600 ± 0.0140 0 $ -1.6021 ^{+ 0.0792 }_{- 0.0969 } $ 

$ -0.9459 ^{+ 0.0437 }_{- 0.0438 } $ 

205Ra $ ^{\rm{m}} $ 

$ \to {}^{201}{\rm{Rn}} $ 

$ ^{\rm{m}} $ 

7.5100 ± 0.0250 0 $ -0.7447 ^{+ 0.1065 }_{- 0.1413 } $ 

$ -0.1294 ^{+ 0.0821 }_{- 0.0826 } $ 

207Ac $ \to {}^{203}{\rm{Fr}} $ 

7.8400 ± 0.0600 0 $ -1.5086 ^{+ 0.0997 }_{- 0.1296 } $ 

$ -0.7991 ^{+ 0.1855 }_{- 0.1878 } $ 

209Ac $ \to {}^{205}{\rm{Fr}} $ 

7.7300 ± 0.0600 0 $ -1.0269 ^{+ 0.0439 }_{- 0.0488 } $ 

$ -0.4383 ^{+ 0.1898 }_{- 0.1922 } $ 

211Ac $ \to {}^{207}{\rm{Fr}} $ 

7.5700 ± 0.0500 0 $ -0.6716 ^{+ 0.0482 }_{- 0.0542 } $ 

$ 0.0943 ^{+ 0.1638 }_{- 0.1656 } $ 

213Ac $ \to {}^{209}{\rm{Fr}} $ 

7.4980 ± 0.0040 0 $ -0.1319 ^{+ 0.0093 }_{- 0.0095 } $ 

$ 0.3477 ^{+ 0.0134 }_{- 0.0134 } $ 

215Ac $ \to {}^{211}{\rm{Fr}} $ 

7.7460 ± 0.0030 0 $ -0.7670 ^{+ 0.0247 }_{- 0.0262 } $ 

$ -0.4465 ^{+ 0.0095 }_{- 0.0095 } $ 

217Ac $ \to {}^{213}{\rm{Fr}} $ 

9.8320 ± 0.0100 0 $ -7.1612 ^{+ 0.0245 }_{- 0.0259 } $ 

$ -7.2482 ^{+ 0.0211 }_{- 0.0211 } $ 

219Ac $ \to {}^{215}{\rm{Fr}} $ 

8.8830 ± 0.0500 0 $ -5.0269 ^{+ 0.0439 }_{- 0.0488 } $ 

$ -5.0560 ^{+ 0.1245 }_{- 0.1257 } $ 

221Ac $ \to {}^{217}{\rm{Fr}} $ 

7.7900 ± 0.0600 0 $ -1.2840 ^{+ 0.0164 }_{- 0.0170 } $ 

$ -1.9931 ^{+ 0.1855 }_{- 0.1879 } $ 

227Ac $ \to {}^{223}{\rm{Fr}} $ 

5.0423 ± 0.0001 0 $ 10.6971 ^{+ 0.0001 }_{- 0.0001 } $ 

$ 10.1322 ^{+ 0.0006 }_{- 0.0013 } $ 

211Th $ \to {}^{207}{\rm{Ra}} $ 

7.9400 ± 0.0600 0 $ -1.3188 ^{+ 0.1513 }_{- 0.2341 } $ 

$ -0.7155 ^{+ 0.1841 }_{- 0.1863 } $ 

213Th $ \to {}^{209}{\rm{Ra}} $ 

7.8370 ± 0.0070 0 $ -0.8416 ^{+ 0.0591 }_{- 0.0685 } $ 

$ -0.3798 ^{+ 0.0221 }_{- 0.0221 } $ 

219Th $ \to {}^{215}{\rm{Ra}} $ 

9.5100 ± 0.0600 0 $ -5.9901 ^{+ 0.0076 }_{- 0.0077 } $ 

$ -6.2382 ^{+ 0.1349 }_{- 0.1363 } $ 

209Th $ ^{\rm{m}} $ 

$ \to {}^{205}{\rm{Ra}} $ 

$ ^{\rm{m}} $ 

8.2700 ± 0.1000 0 $ -2.5086 ^{+ 0.1421 }_{- 0.2126 } $ 

$ -1.7152 ^{+ 0.2859 }_{- 0.2917 } $ 

211Pa $ \to {}^{207}{\rm{Ac}} $ 

8.4800 ± 0.0400 0 $ -2.2218 ^{+ 0.1761 }_{- 0.3010 } $ 

$ -1.9513 ^{+ 0.1118 }_{- 0.1127 } $ 

213Pa $ \to {}^{209}{\rm{Ac}} $ 

8.3840 ± 0.0120 0 $ -2.1308 ^{+ 0.1220 }_{- 0.1703 } $ 

$ -1.6650 ^{+ 0.0343 }_{- 0.0344 } $ 

215Pa $ \to {}^{211}{\rm{Ac}} $ 

8.2400 ± 0.0600 0 $ -1.8539 ^{+ 0.0580 }_{- 0.0669 } $ 

$ -1.2330 ^{+ 0.1755 }_{- 0.1776 } $ 

217Pa $ \to {}^{213}{\rm{Ac}} $ 

8.4890 ± 0.0040 0 $ -2.4202 ^{+ 0.0223 }_{- 0.0235 } $ 

$ -1.9341 ^{+ 0.0112 }_{- 0.0112 } $ 

219Pa $ \to {}^{215}{\rm{Ac}} $ 

10.1300 ± 0.0700 0 $ -7.2518 ^{+ 0.0647 }_{- 0.0761 } $ 

$ -7.2831 ^{+ 0.1430 }_{- 0.1447 } $ 

221Pa $ \to {}^{217}{\rm{Ac}} $ 

9.2500 ± 0.0600 0 $ -5.2291 ^{+ 0.1100 }_{- 0.1476 } $ 

$ -5.3161 ^{+ 0.1431 }_{- 0.1447 } $ 

223Pa $ \to {}^{219}{\rm{Ac}} $ 

8.3400 ± 0.0600 0 $ -2.2757 ^{+ 0.0239 }_{- 0.0253 } $ 

$ -2.9230 ^{+ 0.1701 }_{- 0.1721 } $ 

227Pa $ \to {}^{223}{\rm{Ac}} $ 

6.5804 ± 0.0021 0 $ 3.4319 ^{+ 0.0034 }_{- 0.0034 } $ 

$ 3.1868 ^{+ 0.0088 }_{- 0.0088 } $ 

231Pa $ \to {}^{227}{\rm{Ac}} $ 

5.1499 ± 0.0008 0 $ 12.0130 ^{+ 0.0027 }_{- 0.0027 } $ 

$ 10.4972 ^{+ 0.0050 }_{- 0.0050 } $ 

221U $ \to {}^{217}{\rm{Th}} $ 

9.8900 ± 0.0700 0 $ -6.1805 ^{+ 0.0835 }_{- 0.1035 } $ 

$ -6.4768 ^{+ 0.1509 }_{- 0.1527 } $ 

229U $ \to {}^{225}{\rm{Th}} $ 

6.4760 ± 0.0030 0 $ 4.2390 ^{+ 0.0037 }_{- 0.0038 } $ 

$ 4.0626 ^{+ 0.0131 }_{- 0.0131 } $ 

233U $ \to {}^{229}{\rm{Th}} $ 

4.9087 ± 0.0012 0 $ 12.7010 ^{+ 0.0004 }_{- 0.0004 } $ 

$ 12.5796 ^{+ 0.0081 }_{- 0.0081 } $ 

223Np $ \to {}^{219}{\rm{Pa}} $ 

9.6500 ± 0.0400 0 $ -5.6021 ^{+ 0.1206 }_{- 0.1675 } $ 

$ -5.6347 ^{+ 0.0913 }_{- 0.0919 } $ 

Continued on next page Table 3-continued from previous page α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{\,exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{\,HOPM}} $ 

225Np $ \to {}^{221}{\rm{Pa}} $ 

8.8200 ± 0.0500 0 $ -2.1871 ^{+ 0.1871 }_{- 0.3358 } $ 

$ -3.5713 ^{+ 0.1325 }_{- 0.1338 } $ 

233Np $ \to {}^{229}{\rm{Pa}} $ 

5.6300 ± 0.0500 0 $ 8.4918 ^{+ 0.0012 }_{- 0.0012 } $ 

$ 8.6764 ^{+ 0.2733 }_{- 0.2772 } $ 

231Pu $ \to {}^{227}{\rm{U}} $ 

6.8390 ± 0.0200 0 $ 3.5987 ^{+ 0.0245 }_{- 0.0260 } $ 

$ 3.3658 ^{+ 0.0815 }_{- 0.0819 } $ 

235Pu $ \to {}^{231}{\rm{U}} $ 

5.9510 ± 0.0200 0 $ 7.7341 ^{+ 0.0085 }_{- 0.0087 } $ 

$ 7.4483 ^{+ 0.1018 }_{- 0.1023 } $ 

239Pu $ \to {}^{235}{\rm{U}} $ 

$ ^{\rm{m}} $ 

5.2445 ± 0.0002 0 $ 11.8813 ^{+ 0.0005 }_{- 0.0005 } $ 

$ 11.4601 ^{+ 0.0012 }_{- 0.0012 } $ 

233Cm $ \to {}^{229}{\rm{Pu}} $ 

7.4700 ± 0.0500 0 $ 2.1303 ^{+ 0.1368 }_{- 0.2009 } $ 

$ 1.7284 ^{+ 0.1803 }_{- 0.1823 } $ 

239Cf $ \to {}^{235}{\rm{Cm}} $ 

7.7700 ± 0.0600 0 $ 2.7482 ^{+ 0.0300 }_{- 0.0322 } $ 

$ 1.4455 ^{+ 0.2076 }_{- 0.2102 } $ 

241Cf $ \to {}^{237}{\rm{Cm}} $ 

$ ^{\rm{p}} $ 

7.4600 ± 0.1500 0 $ 2.9731 ^{+ 0.0321 }_{- 0.0346 } $ 

$ 2.5754 ^{+ 0.5490 }_{- 0.5671 } $ 

245Cf $ \to {}^{241}{\rm{Cm}} $ 

7.2585 ± 0.0018 0 $ 3.8836 ^{+ 0.0142 }_{- 0.0147 } $ 

$ 3.3706 ^{+ 0.0070 }_{- 0.0070 } $ 

253Cf $ \to {}^{249}{\rm{Cm}} $ 

$ ^{\rm{m}} $ 

6.1260 ± 0.0040 0 $ 9.0732 ^{+ 0.0022 }_{- 0.0022 } $ 

$ 8.4700 ^{+ 0.0204 }_{- 0.0204 } $ 

241Es $ \to {}^{237}{\rm{Bk}} $ 

8.2590 ± 0.0170 0 $ 0.7076 ^{+ 0.0633 }_{- 0.0741 } $ 

$ 0.1867 ^{+ 0.0541 }_{- 0.0543 } $ 

243Es $ \to {}^{239}{\rm{Bk}} $ 

8.0720 ± 0.0100 0 $ 1.5591 ^{+ 0.0267 }_{- 0.0284 } $ 

$ 0.8084 ^{+ 0.0331 }_{- 0.0331 } $ 

245Es $ \to {}^{241}{\rm{Bk}} $ 

$ ^{\rm{p}} $ 

7.9090 ± 0.0030 0 $ 2.1333 ^{+ 0.0229 }_{- 0.0241 } $ 

$ 1.3711 ^{+ 0.0103 }_{- 0.0103 } $ 

247Es $ \to {}^{243}{\rm{Bk}} $ 

$ ^{\rm{p}} $ 

7.4640 ± 0.0200 0 $ 3.5911 ^{+ 0.0241 }_{- 0.0256 } $ 

$ 2.9813 ^{+ 0.0750 }_{- 0.0753 } $ 

251Es $ \to {}^{247}{\rm{Bk}} $ 

6.5971 ± 0.0010 0 $ 7.3758 ^{+ 0.0130 }_{- 0.0134 } $ 

$ 6.6081 ^{+ 0.0046 }_{- 0.0046 } $ 

253Es $ \to {}^{249}{\rm{Bk}} $ 

6.7392 ± 0.0001 0 $ 6.2476 ^{+ 0.0006 }_{- 0.0006 } $ 

$ 5.9819 ^{+ 0.0004 }_{- 0.0000 } $ 

255Es $ \to {}^{251}{\rm{Bk}} $ 

$ ^{\rm{m}} $ 

6.4363 ± 0.0013 0 $ 7.6333 ^{+ 0.0129 }_{- 0.0133 } $ 

$ 7.3886 ^{+ 0.0062 }_{- 0.0062 } $ 

241Fm $ \to {}^{237}{\rm{Cf}} $ 

8.8600 ± 0.3200 0 $ -2.2828 ^{+ 0.0343 }_{- 0.0372 } $ 

$ -1.2946 ^{+ 0.8924 }_{- 0.9472 } $ 

247Fm $ ^{\rm{m}} $ 

$ \to {}^{243}{\rm{Cf}} $ 

8.3100 ± 0.0080 0 $ 0.7631 ^{+ 0.0167 }_{- 0.0174 } $ 

$ 0.4131 ^{+ 0.0255 }_{- 0.0256 } $ 

251No $ \to {}^{247}{\rm{Fm}} $ 

8.7520 ± 0.0040 0 $ -0.0160 ^{+ 0.0054 }_{- 0.0055 } $ 

$ -0.2474 ^{+ 0.0120 }_{- 0.0120 } $ 

251No $ ^{\rm{m}} $ 

$ \to {}^{247}{\rm{Fm}} $ 

$ ^{\rm{m}} $ 

8.8200 ± 0.0060 0 $ 0.0086 ^{+ 0.0126 }_{- 0.0130 } $ 

$ -0.4500 ^{+ 0.0178 }_{- 0.0178 } $ 

253Lr $ \to {}^{249}{\rm{Md}} $ 

8.9180 ± 0.3000 0 $ -0.1535 ^{+ 0.0306 }_{- 0.0329 } $ 

$ -0.3960 ^{+ 0.8580 }_{- 0.9069 } $ 

253Lr $ ^{\rm{m}} $ 

$ \to {}^{249}{\rm{Md}} $ 

$ ^{\rm{m}} $ 

8.8600 ± 0.1000 0 $ 0.1663 ^{+ 0.0438 }_{- 0.0487 } $ 

$ -0.2246 ^{+ 0.2944 }_{- 0.2999 } $ 

255Lr $ \to {}^{251}{\rm{Md}} $ 

$ ^{\rm{p}} $ 

8.5560 ± 0.0070 0 $ 1.4941 ^{+ 0.0151 }_{- 0.0156 } $ 

$ 0.7190 ^{+ 0.0220 }_{- 0.0220 } $ 

255Lr $ ^{\rm{m}} $ 

$ \to {}^{251}{\rm{Md}} $ 

8.6000 ± 0.0800 0 $ 0.8028 ^{+ 0.0085 }_{- 0.0086 } $ 

$ 0.5811 ^{+ 0.2477 }_{- 0.2515 } $ 

259Lr $ \to {}^{255}{\rm{Md}} $ 

$ ^{\rm{p}} $ 

8.5800 ± 0.0700 0 $ 0.9003 ^{+ 0.0021 }_{- 0.0021 } $ 

$ 0.6713 ^{+ 0.2179 }_{- 0.2208 } $ 

261Rf $ \to {}^{257}{\rm{No}} $ 

8.6500 ± 0.0700 0 $ 1.0669 ^{+ 0.0395 }_{- 0.0435 } $ 

$ 0.8048 ^{+ 0.2174 }_{- 0.2203 } $ 

257Db $ ^{\rm{m}} $ 

$ \to {}^{253}{\rm{Lr}} $ 

$ ^{\rm{m}} $ 

9.3100 ± 0.1100 0 $ -0.1134 ^{+ 0.0372 }_{- 0.0407 } $ 

$ -0.8470 ^{+ 0.3048 }_{- 0.3108 } $ 

263Sg $ \to {}^{259}{\rm{Rf}} $ 

9.4000 ± 0.0600 0 $ 0.0336 ^{+ 0.0603 }_{- 0.0700 } $ 

$ -0.7403 ^{+ 0.1662 }_{- 0.1680 } $ 

265Hs $ \to {}^{259}{\rm{Sg}} $ 

$ ^{\rm{m}} $ 

10.4700 ± 0.0800 0 $ -2.7077 ^{+ 0.0341 }_{- 0.0370 } $ 

$ -2.8756 ^{+ 0.1889 }_{- 0.1913 } $ 

263Hs $ ^{\rm{m}} $ 

$ \to {}^{261}{\rm{Sg}} $ 

10.4700 ± 0.1100 0 $ -3.0000 ^{+ 0.0000 }_{- 0.0000 } $ 

$ -2.8892 ^{+ 0.2591 }_{- 0.2637 } $ 

267Ds $ \to {}^{263}{\rm{Hs}} $ 

11.7800 ± 0.0500 0 $ -5.0000 ^{+ 0.2553 }_{- 0.6990 } $ 

$ -5.1623 ^{+ 0.0990 }_{- 0.0997 } $ 

α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{\,exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{\,HOPM}} $ 

148Eu $ \to {}^{144}{\rm{Pm}} $ 

2.6940 ± 0.0100 0 $ 14.6998 ^{+ 0.0040 }_{- 0.0040 } $ 

$ 14.6088 ^{+ 0.1138 }_{- 0.1145 } $ 

152Ho $ ^{\rm{m}} $ 

$ \to {}^{148}{\rm{Tb}} $ 

$ ^{\rm{m}} $ 

4.5800 ± 0.0030 0 $ 2.6638 ^{+ 0.0017 }_{- 0.0017 } $ 

$ 2.5724 ^{+ 0.0160 }_{- 0.0161 } $ 

152Ho $ \to {}^{148}{\rm{Tb}} $ 

4.5074 ± 0.0013 0 $ 3.1298 ^{+ 0.0008 }_{- 0.0008 } $ 

$ 2.9658 ^{+ 0.0071 }_{- 0.0071 } $ 

154Ho $ \to {}^{150}{\rm{Tb}} $ 

4.0410 ± 0.0040 0 $ 6.5698 ^{+ 0.0070 }_{- 0.0071 } $ 

$ 5.7712 ^{+ 0.0260 }_{- 0.0261 } $ 

154Tm $ ^{\rm{m}} $ 

$ \to {}^{150}{\rm{Ho}} $ 

$ ^{\rm{m}} $ 

5.1800 ± 0.0500 0 $ 0.7551 ^{+ 0.0091 }_{- 0.0093 } $ 

$ 0.6378 ^{+ 0.2257 }_{- 0.2292 } $ 

154Tm $ \to {}^{150}{\rm{Ho}} $ 

5.0938 ± 0.0026 0 $ 1.1761 ^{+ 0.0158 }_{- 0.0164 } $ 

$ 1.0351 ^{+ 0.0121 }_{- 0.0122 } $ 

156Tm $ \to {}^{152}{\rm{Ho}} $ 

4.3450 ± 0.0070 0 $ 5.1171 ^{+ 0.0092 }_{- 0.0094 } $ 

$ 5.0162 ^{+ 0.0420 }_{- 0.0421 } $ 

156Lu $ \to {}^{152}{\rm{Tm}} $ 

5.5960 ± 0.0030 0 $ -0.3063 ^{+ 0.0104 }_{- 0.0107 } $ 

$ -0.2161 ^{+ 0.0124 }_{- 0.0125 } $ 

158Lu $ \to {}^{154}{\rm{Tm}} $ 

4.7900 ± 0.0050 0 $ 3.0663 ^{+ 0.0121 }_{- 0.0125 } $ 

$ 3.5850 ^{+ 0.0265 }_{- 0.0266 } $ 

158Ta $ \to {}^{154}{\rm{Lu}} $ 

6.1240 ± 0.0040 0 $ -1.3098 ^{+ 0.0341 }_{- 0.0370 } $ 

$ -1.3884 ^{+ 0.0148 }_{- 0.0148 } $ 

162Re $ ^{\rm{m}} $ 

$ \to {}^{158}{\rm{Ta}} $ 

$ ^{\rm{m}} $ 

6.2800 ± 0.0090 0 $ -1.0726 ^{+ 0.0480 }_{- 0.0540 } $ 

$ -1.0839 ^{+ 0.0330 }_{- 0.0330 } $ 

164Re $ ^{\rm{m}} $ 

$ \to {}^{160}{\rm{Ta}} $ 

$ ^{\rm{m}} $ 

5.7700 ± 0.2500 0 $ 1.4723 ^{+ 0.0592 }_{- 0.0686 } $ 

$ 0.9351 ^{+ 1.0153 }_{- 1.0880 } $ 

162Re $ \to {}^{158}{\rm{Ta}} $ 

6.2400 ± 0.0050 0 $ -0.9437 ^{+ 0.0498 }_{- 0.0563 } $ 

$ -0.9365 ^{+ 0.0185 }_{- 0.0185 } $ 

164Ir $ ^{\rm{m}} $ 

$ \to {}^{160}{\rm{Re}} $ 

$ ^{\rm{m}} $ 

7.0600 ± 0.1000 0 $ -2.7570 ^{+ 0.0580 }_{- 0.0669 } $ 

$ -2.9047 ^{+ 0.3089 }_{- 0.3162 } $ 

166Ir $ ^{\rm{m}} $ 

$ \to {}^{162}{\rm{Re}} $ 

$ ^{\rm{m}} $ 

6.7300 ± 0.0060 0 $ -1.8131 ^{+ 0.0251 }_{- 0.0267 } $ 

$ -1.8155 ^{+ 0.0203 }_{- 0.0203 } $ 

168Ir $ ^{\rm{m}} $ 

$ \to {}^{164}{\rm{Re}} $ 

$ ^{\rm{m}} $ 

6.4800 ± 0.2500 0 $ -0.6743 ^{+ 0.0407 }_{- 0.0449 } $ 

$ -0.9277 ^{+ 0.8711 }_{- 0.9269 } $ 

172Ir $ ^{\rm{m}} $ 

$ \to {}^{168}{\rm{Re}} $ 

6.1300 ± 0.0100 0 $ 1.3627 ^{+ 0.0137 }_{- 0.0141 } $ 

$ 0.4205 ^{+ 0.0392 }_{- 0.0393 } $ 

166Ir $ \to {}^{162}{\rm{Re}} $ 

6.7220 ± 0.0060 0 $ -1.9473 ^{+ 0.0826 }_{- 0.1021 } $ 

$ -1.7884 ^{+ 0.0203 }_{- 0.0203 } $ 

168Ir $ \to {}^{164}{\rm{Re}} $ 

6.3810 ± 0.0090 0 $ -0.6383 ^{+ 0.0854 }_{- 0.1065 } $ 

$ -0.5676 ^{+ 0.0331 }_{- 0.0332 } $ 

170Ir $ \to {}^{166}{\rm{Re}} $ 

$ ^{\rm{p}} $ 

5.9600 ± 0.0500 0 $ 1.2430 ^{+ 0.0663 }_{- 0.0782 } $ 

$ 1.0868 ^{+ 0.2041 }_{- 0.2068 } $ 

170Au $ ^{\rm{m}} $ 

$ \to {}^{166}{\rm{Ir}} $ 

$ ^{\rm{m}} $ 

7.2900 ± 0.0130 0 $ -2.8309 ^{+ 0.0337 }_{- 0.0365 } $ 

$ -2.8068 ^{+ 0.0397 }_{- 0.0398 } $ 

172Au $ ^{\rm{m}} $ 

$ \to {}^{168}{\rm{Ir}} $ 

$ ^{\rm{m}} $ 

7.0400 ± 0.2500 0 $ -1.9586 ^{+ 0.0378 }_{- 0.0414 } $ 

$ -2.0045 ^{+ 0.7859 }_{- 0.8325 } $ 

178Au $ ^{\rm{m}} $ 

$ \to {}^{174}{\rm{Ir}} $ 

$ ^{\rm{m}} $ 

6.1200 ± 0.0002 0 $ 1.1761 ^{+ 0.0142 }_{- 0.0147 } $ 

$ 1.3753 ^{+ 0.0008 }_{- 0.0008 } $ 

170Au $ \to {}^{166}{\rm{Ir}} $ 

7.1770 ± 0.0150 0 $ -2.5790 ^{+ 0.0691 }_{- 0.0822 } $ 

$ -2.4572 ^{+ 0.0469 }_{- 0.0471 } $ 

172Au $ \to {}^{168}{\rm{Ir}} $ 

6.9230 ± 0.0100 0 $ -1.5528 ^{+ 0.0580 }_{- 0.0669 } $ 

$ -1.6210 ^{+ 0.0332 }_{- 0.0333 } $ 

174Au $ \to {}^{170}{\rm{Ir}} $ 

6.6990 ± 0.0070 0 $ -0.8112 ^{+ 0.0093 }_{- 0.0095 } $ 

$ -0.8400 ^{+ 0.0245 }_{- 0.0246 } $ 

176Au $ \to {}^{172}{\rm{Ir}} $ 

6.4330 ± 0.0070 0 $ 0.1461 ^{+ 0.0041 }_{- 0.0042 } $ 

$ 0.1398 ^{+ 0.0262 }_{- 0.0262 } $ 

178Au $ \to {}^{174}{\rm{Ir}} $ 

6.0580 ± 0.0050 0 $ 1.3274 ^{+ 0.0596 }_{- 0.0691 } $ 

$ 1.6288 ^{+ 0.0206 }_{- 0.0206 } $ 

184Au $ \to {}^{180}{\rm{Ir}} $ 

5.2340 ± 0.0050 0 $ 5.1999 ^{+ 0.0186 }_{- 0.0194 } $ 

$ 5.4906 ^{+ 0.0260 }_{- 0.0260 } $ 

188Bi $ ^{\rm{n}} $ 

$ \to {}^{184}{\rm{Tl}} $ 

$ ^{\rm{n}} $ 

6.9700 ± 0.0300 0 $ -0.5768 ^{+ 0.0239 }_{- 0.0253 } $ 

$ -0.0761 ^{+ 0.1040 }_{- 0.1047 } $ 

196Bi $ \to {}^{192}{\rm{Tl}} $ 

$ ^{\rm{p}} $ 

5.2600 ± 0.0400 0 $ 7.4276 ^{+ 0.0166 }_{- 0.0173 } $ 

$ 7.4200 ^{+ 0.2165 }_{- 0.2191 } $ 

194At $ ^{\rm{m}} $ 

$ \to {}^{190}{\rm{Bi}} $ 

$ ^{\rm{m}} $ 

7.3100 ± 0.0400 0 $ -0.4908 ^{+ 0.0093 }_{- 0.0095 } $ 

$ -0.4043 ^{+ 0.1317 }_{- 0.1329 } $ 

196At $ ^{\rm{m}} $ 

$ \to {}^{192}{\rm{Bi}} $ 

$ ^{\rm{m}} $ 

7.0200 ± 0.0400 0 $ -1.6990 ^{+ 0.0000 }_{- 0.0000 } $ 

$ 0.6022 ^{+ 0.1406 }_{- 0.1419 } $ 

198At $ ^{\rm{m}} $ 

$ \to {}^{194}{\rm{Bi}} $ 

$ ^{\rm{n}} $ 

6.9900 ± 0.0027 0 $ 0.1214 ^{+ 0.0173 }_{- 0.0180 } $ 

$ 0.7237 ^{+ 0.0096 }_{- 0.0096 } $ 

200At $ ^{\rm{m}} $ 

$ \to {}^{196}{\rm{Bi}} $ 

$ ^{\rm{m}} $ 

6.5400 ± 0.0029 0 $ 2.0386 ^{+ 0.0091 }_{- 0.0093 } $ 

$ 2.4272 ^{+ 0.0115 }_{- 0.0115 } $ 

202At $ ^{\rm{m}} $ 

$ \to {}^{198}{\rm{Bi}} $ 

$ ^{\rm{m}} $ 

6.2600 ± 0.0400 0 $ 3.3307 ^{+ 0.0072 }_{- 0.0073 } $ 

$ 3.5901 ^{+ 0.1689 }_{- 0.1707 } $ 

202At $ ^{\rm{n}} $ 

$ \to {}^{198}{\rm{Bi}} $ 

$ ^{\rm{n}} $ 

6.4000 ± 0.0400 0 $ 2.6805 ^{+ 0.0448 }_{- 0.0500 } $ 

$ 3.0062 ^{+ 0.1631 }_{- 0.1647 } $ 

214At $ ^{\rm{n}} $ 

$ \to {}^{210}{\rm{Bi}} $ 

$ ^{\rm{m}} $ 

8.9500 ± 0.0090 0 $ -6.1192 ^{+ 0.0085 }_{- 0.0087 } $ 

$ -6.2223 ^{+ 0.0211 }_{- 0.0211 } $ 

216At $ ^{\rm{m}} $ 

$ \to {}^{212}{\rm{Bi}} $ 

$ ^{\rm{m}} $ 

7.8600 ± 0.0110 0 $ -4.0000 ^{+ 0.0000 }_{- 0.0000 } $ 

$ -3.3635 ^{+ 0.0320 }_{- 0.0321 } $ 

192At $ \to {}^{188}{\rm{Bi}} $ 

7.6960 ± 0.0260 0 $ -1.9393 ^{+ 0.0221 }_{- 0.0233 } $ 

$ -1.6440 ^{+ 0.0789 }_{- 0.0793 } $ 

194At $ \to {}^{190}{\rm{Bi}} $ 

$ ^{\rm{n}} $ 

7.4540 ± 0.0110 0 $ -0.5436 ^{+ 0.0105 }_{- 0.0108 } $ 

$ -0.8731 ^{+ 0.0352 }_{- 0.0353 } $ 

Continued on next page Table 4-continued from previous page α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{\,exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{\,HOPM}} $ 

196At $ \to {}^{192}{\rm{Bi}} $ 

7.1964 ± 0.0027 0 $ -0.4127 ^{+ 0.0046 }_{- 0.0046 } $ 

$ -0.0085 ^{+ 0.0092 }_{- 0.0092 } $ 

198At $ \to {}^{194}{\rm{Bi}} $ 

6.8894 ± 0.0019 0 $ 0.6772 ^{+ 0.0048 }_{- 0.0049 } $ 

$ 1.0857 ^{+ 0.0069 }_{- 0.0069 } $ 

200At $ \to {}^{196}{\rm{Bi}} $ 

6.5962 ± 0.0013 0 $ 1.9195 ^{+ 0.0090 }_{- 0.0091 } $ 

$ 2.2063 ^{+ 0.0051 }_{- 0.0051 } $ 

202At $ \to {}^{198}{\rm{Bi}} $ 

6.3538 ± 0.0013 0 $ 3.1856 ^{+ 0.0024 }_{- 0.0024 } $ 

$ 3.1966 ^{+ 0.0054 }_{- 0.0054 } $ 

204At $ \to {}^{200}{\rm{Bi}} $ 

6.0704 ± 0.0012 0 $ 4.1584 ^{+ 0.0052 }_{- 0.0053 } $ 

$ 4.4300 ^{+ 0.0053 }_{- 0.0054 } $ 

208At $ \to {}^{204}{\rm{Bi}} $ 

5.7511 ± 0.0022 0 $ 6.0281 ^{+ 0.0079 }_{- 0.0081 } $ 

$ 5.9459 ^{+ 0.0107 }_{- 0.0107 } $ 

214At $ \to {}^{210}{\rm{Bi}} $ 

8.9880 ± 0.0040 0 $ -6.2534 ^{+ 0.0077 }_{- 0.0079 } $ 

$ -6.3110 ^{+ 0.0093 }_{- 0.0093 } $ 

216At $ \to {}^{212}{\rm{Bi}} $ 

7.9500 ± 0.0030 0 $ -3.5229 ^{+ 0.0414 }_{- 0.0458 } $ 

$ -3.6230 ^{+ 0.0086 }_{- 0.0086 } $ 

220At $ \to {}^{216}{\rm{Bi}} $ 

$ ^{\rm{m}} $ 

6.0770 ± 0.0180 0 $ 3.4444 ^{+ 0.0047 }_{- 0.0047 } $ 

$ 3.0546 ^{+ 0.0794 }_{- 0.0798 } $ 

198Fr $ ^{\rm{m}} $ 

$ \to {}^{194}{\rm{At}} $ 

$ ^{\rm{m}} $ 

7.9000 ± 0.0500 0 $ -2.9586 ^{+ 0.2139 }_{- 0.4393 } $ 

$ -1.4868 ^{+ 0.1489 }_{- 0.1504 } $ 

200Fr $ ^{\rm{m}} $ 

$ \to {}^{196}{\rm{At}} $ 

$ ^{\rm{m}} $ 

7.7100 ± 0.0600 0 $ -0.7212 ^{+ 0.2126 }_{- 0.4337 } $ 

$ -0.8916 ^{+ 0.1857 }_{- 0.1881 } $ 

202Fr $ ^{\rm{m}} $ 

$ \to {}^{198}{\rm{At}} $ 

$ ^{\rm{m}} $ 

7.3800 ± 0.0060 0 $ -0.5719 ^{+ 0.0206 }_{- 0.0216 } $ 

$ 0.1885 ^{+ 0.0201 }_{- 0.0201 } $ 

204Fr $ ^{\rm{m}} $ 

$ \to {}^{200}{\rm{At}} $ 

$ ^{\rm{m}} $ 

7.1100 ± 0.0040 0 $ 0.4278 ^{+ 0.0330 }_{- 0.0357 } $ 

$ 1.1340 ^{+ 0.0142 }_{- 0.0142 } $ 

204Fr $ ^{\rm{n}} $ 

$ \to {}^{200}{\rm{At}} $ 

$ ^{\rm{n}} $ 

7.1500 ± 0.0040 0 $ 0.4932 ^{+ 0.0378 }_{- 0.0414 } $ 

$ 0.9926 ^{+ 0.0141 }_{- 0.0141 } $ 

206Fr $ ^{\rm{m}} $ 

$ \to {}^{202}{\rm{At}} $ 

$ ^{\rm{m}} $ 

6.9300 ± 0.0400 0 $ 1.2762 ^{+ 0.0000 }_{- 0.0000 } $ 

$ 1.8016 ^{+ 0.1474 }_{- 0.1488 } $ 

206Fr $ ^{\rm{n}} $ 

$ \to {}^{202}{\rm{At}} $ 

$ ^{\rm{n}} $ 

7.0700 ± 0.0400 0 $ 0.7312 ^{+ 0.0580 }_{- 0.0669 } $ 

$ 1.2916 ^{+ 0.1428 }_{- 0.1441 } $ 

216Fr $ ^{\rm{m}} $ 

$ \to {}^{212}{\rm{At}} $ 

$ ^{\rm{m}} $ 

9.1700 ± 0.0060 0 $ -6.0706 ^{+ 0.0151 }_{- 0.0156 } $ 

$ -6.1018 ^{+ 0.0139 }_{- 0.0139 } $ 

200Fr $ \to {}^{196}{\rm{At}} $ 

7.6220 ± 0.0040 0 $ -1.3233 ^{+ 0.0249 }_{- 0.0264 } $ 

$ -0.6149 ^{+ 0.0127 }_{- 0.0127 } $ 

202Fr $ \to {}^{198}{\rm{At}} $ 

7.3850 ± 0.0040 0 $ -0.4295 ^{+ 0.0138 }_{- 0.0142 } $ 

$ 0.1718 ^{+ 0.0134 }_{- 0.0134 } $ 

204Fr $ \to {}^{200}{\rm{At}} $ 

7.1703 ± 0.0024 0 $ 0.2608 ^{+ 0.0602 }_{- 0.0699 } $ 

$ 0.9213 ^{+ 0.0084 }_{- 0.0084 } $ 

206Fr $ \to {}^{202}{\rm{At}} $ 

6.9230 ± 0.0030 0 $ 1.2577 ^{+ 0.0000 }_{- 0.0000 } $ 

$ 1.8276 ^{+ 0.0111 }_{- 0.0111 } $ 

208Fr $ \to {}^{204}{\rm{At}} $ 

6.7850 ± 0.0250 0 $ 1.8222 ^{+ 0.0022 }_{- 0.0022 } $ 

$ 2.3625 ^{+ 0.0955 }_{- 0.0960 } $ 

210Fr $ \to {}^{206}{\rm{At}} $ 

6.6710 ± 0.0050 0 $ 2.4293 ^{+ 0.0081 }_{- 0.0083 } $ 

$ 2.8198 ^{+ 0.0197 }_{- 0.0197 } $ 

216Fr $ \to {}^{212}{\rm{At}} $ 

9.1740 ± 0.0030 0 $ -6.1549 ^{+ 0.0122 }_{- 0.0126 } $ 

$ -6.1111 ^{+ 0.0069 }_{- 0.0069 } $ 

218Fr $ \to {}^{214}{\rm{At}} $ 

8.0137 ± 0.0014 0 $ -2.8539 ^{+ 0.1326 }_{- 0.1919 } $ 

$ -3.0991 ^{+ 0.0041 }_{- 0.0041 } $ 

206Ac $ ^{\rm{m}} $ 

$ \to {}^{202}{\rm{Fr}} $ 

$ ^{\rm{m}} $ 

7.9100 ± 0.0700 0 $ -1.3872 ^{+ 0.1431 }_{- 0.2148 } $ 

$ -0.7339 ^{+ 0.2131 }_{- 0.2162 } $ 

206Ac $ \to {}^{202}{\rm{Fr}} $ 

7.9600 ± 0.0600 0 $ -1.6021 ^{+ 0.1072 }_{- 0.1427 } $ 

$ -0.8864 ^{+ 0.1810 }_{- 0.1832 } $ 

208Ac $ \to {}^{204}{\rm{Fr}} $ 

7.7300 ± 0.0600 0 $ -1.0132 ^{+ 0.0624 }_{- 0.0730 } $ 

$ -0.1568 ^{+ 0.1898 }_{- 0.1922 } $ 

212Ac $ \to {}^{208}{\rm{Fr}} $ 

7.5400 ± 0.0240 0 $ -0.0482 ^{+ 0.0134 }_{- 0.0138 } $ 

$ 0.4894 ^{+ 0.0794 }_{- 0.0798 } $ 

218Ac $ \to {}^{214}{\rm{Fr}} $ 

9.3800 ± 0.0600 0 $ -6.0000 ^{+ 0.0170 }_{- 0.0177 } $ 

$ -5.9616 ^{+ 0.1362 }_{- 0.1377 } $ 

222Ac $ \to {}^{218}{\rm{Fr}} $ 

7.1374 ± 0.0020 0 $ 0.7033 ^{+ 0.0414 }_{- 0.0458 } $ 

$ 0.4831 ^{+ 0.0072 }_{- 0.0072 } $ 

212Pa $ \to {}^{208}{\rm{Ac}} $ 

8.4100 ± 0.0600 0 $ -2.2366 ^{+ 0.1231 }_{- 0.1724 } $ 

$ -1.4575 ^{+ 0.1697 }_{- 0.1717 } $ 

214Pa $ \to {}^{210}{\rm{Ac}} $ 

8.2700 ± 0.0500 0 $ -1.7696 ^{+ 0.0706 }_{- 0.0843 } $ 

$ -1.0393 ^{+ 0.1455 }_{- 0.1470 } $ 

220Pa $ \to {}^{216}{\rm{Ac}} $ 

9.7040 ± 0.0110 0 $ -6.0706 ^{+ 0.0296 }_{- 0.0318 } $ 

$ -6.0796 ^{+ 0.0243 }_{- 0.0244 } $ 

226Pa $ \to {}^{222}{\rm{Ac}} $ 

6.9870 ± 0.0100 0 $ 2.1642 ^{+ 0.0458 }_{- 0.0512 } $ 

$ 1.8437 ^{+ 0.0380 }_{- 0.0381 } $ 

226Np $ \to {}^{222}{\rm{Pa}} $ 

8.3300 ± 0.0500 0 $ -1.4559 ^{+ 0.1091 }_{- 0.1461 } $ 

$ -1.9072 ^{+ 0.1457 }_{- 0.1471 } $ 

236Am $ \to {}^{232}{\rm{Np}} $ 

6.2600 ± 0.0600 0 $ 6.7324 ^{+ 0.0119 }_{- 0.0122 } $ 

$ 6.6619 ^{+ 0.2835 }_{- 0.2879 } $ 

240Am $ \to {}^{236}{\rm{Np}} $ 

$ ^{\rm{p}} $ 

5.4700 ± 0.0500 0 $ 10.9834 ^{+ 0.0026 }_{- 0.0026 } $ 

$ 10.8768 ^{+ 0.2927 }_{- 0.2970 } $ 

246Es $ \to {}^{242}{\rm{Bk}} $ 

$ ^{\rm{p}} $ 

7.5000 ± 0.1000 0 $ 3.6576 ^{+ 0.0280 }_{- 0.0300 } $ 

$ 3.1282 ^{+ 0.3690 }_{- 0.3770 } $ 

252Lr $ \to {}^{248}{\rm{Md}} $ 

9.1640 ± 0.0170 0 $ -0.4242 ^{+ 0.0804 }_{- 0.0987 } $ 

$ -0.8211 ^{+ 0.0477 }_{- 0.0478 } $ 

The above discussion focuses primarily on the favored α decay half-lives. For unfavored α decay, as shown in Eq. (17), we introduce another term
$ d\sqrt{l(l+1)} $ to consider the effect of the centrifugal potential. Using the genetic algorithm with the optimal solution of σ as the objective function and fitting the experimental data of 205 unfavored α decay half-lives, we obtain the value as$ d=0.381 $ . In the following, based on the Eq. (17), we calculate the unfavored α decay half-lives. The detailed calculated α decay half-lives for odd-A and odd-odd nuclei are listed in Tables 5 and 6, respectively. In these two tables, the first four columns denote α decay, the corresponding decay energy, spin and parity transition, and the minimum angular momentum carried away by the emitted α particle, respectively. The fifth and sixth columns represent the experimental α decay half-lives and those calculated using Eq. (17) in logarithmic form, denoted as$ {\rm{log}}_{10}{T}_{1/2}^{\rm{\,exp}} $ and$ {\rm{log}}_{10}{T}_{1/2}^{\rm{\,ISM}} $ , respectively. These two tables intuitively show that the reults calculated using the ISM can reproduce most of the experimental data well, except for a few nuclei such as$ ^{171}{\rm{Hg}} $ ,$ ^{193}{\rm{Rn}} $ ,$ ^{205}{\rm{Rn}} $ ,$ ^{213}{\rm{Ra}}^{m} $ ,$ ^{207}{\rm{Ra}} $ ,$ ^{205}{\rm{Ac}} $ ,$ ^{247}{\rm{Cm}} $ ,$ ^{245}{\rm{Bk}} $ ,$ ^{249}{\rm{Cf}} $ ,$ ^{251}{\rm{Fm}} $ ,$ ^{198}{\rm{Fr}} $ , and$ ^{216}{\rm{Pa}} $ . Among these nuclei,$ ^{247}{\rm{Cm}} $ ,$ ^{245}{\rm{Bk}} $ ,$ ^{249}{\rm{Cf}} $ ,$ ^{251}{\rm{Fm}} $ ,$ ^{255}{\rm{Md}} $ are probably influenced by the shell effect at$ N=152 $ ,$ ^{213}{\rm{Ra}}^{\rm{m}} $ ,$ ^{217}{\rm{Ac}}^{\rm{m}} $ ,$ ^{216}{\rm{Pa}} $ are affected by the shell effect at$ N = 126 $ , and$ ^{171}{\rm{Hg}} $ is probably influenced by shell effect at$ Z=82 $ [85, 91]. For a more clear comparison, the deviations between the experimental half-lives and those calculated using Eqs. (16) and (17) for odd-A and odd-odd nuclei in logarithmic form are plotted in Figs. 3 and 4, respectively. These two figures share the same framework, with the red hollow and blue solid symbols representing the deviations between the experimental data and calculated data using Eqs. (16) and (17), respectively. These two figures show that the original HOPM, i.e., Eq. (16) is generalized to calculate unfavored α decay half-lives with the corresponding deviations being significantly large. Additionally, the deviations between experimental data and calculations for the orbital angular momentum$ l = 1, ~2 $ are relatively close for both equations. However, the deviations generally increase as l increases. Specifically, the deviations between the experimental data and calculated data using HOPM can differ by 6 to 8.5 orders of magnitude for odd-A nuclei with$ l = 11, ~13 $ . Meanwhile, the calculated results using the ISM have a significant improvement. These results indicate that the influence of centrifugal potential is crucial to calculating the unfavored α decay half-lives [61, 72, 74, 83].α decay $ Q_{\alpha} $ 

$ j_p^{\pi}\to{j_d}^{\pi} $ 

l $ \,\log_{10}{T}_{1/2}^{\rm{exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{ISM}} $ 

109I $ \to {}^{105}{\rm{Sb}} $ 

3.9180 ± 0.0210 $ (1/2^{+},3/2^{+}) \to (5/2^{+}) $ 

2 $ -0.1786 ^{+ 0.0037 }_{- 0.0038 } $ 

$ -0.1068 ^{+ 0.1108 }_{- 0.1118 } $ 

151Eu $ \to {}^{147}{\rm{Pm}} $ 

1.9640 ± 0.0010 $ 5/2^{+}* \to 7/2^{+} $ 

2 $ 26.1619 ^{+ 0.1007 }_{- 0.1313 } $ 

$ 25.8688 ^{+ 0.0185 }_{- 0.0185 } $ 

149Tb $ \to {}^{145}{\rm{Eu}} $ 

4.0780 ± 0.0022 $ 1/2^{+} \to 5/2^{+} $ 

2 $ 4.9483 ^{+ 0.0026 }_{- 0.0026 } $ 

$ 4.9868 ^{+ 0.0137 }_{- 0.0137 } $ 

151Tb $ \to {}^{147}{\rm{Eu}} $ 

3.4960 ± 0.0040 $ 1/2^{+}* \to 5/2^{+} $ 

2 $ 8.8243 ^{+ 0.0000 }_{- 0.0000 } $ 

$ 9.0793 ^{+ 0.0316 }_{- 0.0316 } $ 

155Lu $ ^{\rm{m}} $ 

$ \to {}^{151}{\rm{Tm}} $ 

7.5800 ± 0.0040 $ 25/2^{-} \# \to (11/2)^{-} $ 

8 $ -0.7409 ^{+ 0.0274 }_{- 0.0293 } $ 

$ -3.7099 ^{+ 0.0101 }_{- 0.0101 } $ 

157Ta $ ^{\rm{n}} $ 

$ \to {}^{153}{\rm{Lu}} $ 

7.9600 ± 0.0090 $ 25/2^{-} \# \to (11/2)^{-} $ 

8 $ -2.7696 ^{+ 0.0248 }_{- 0.0263 } $ 

$ -3.9390 ^{+ 0.0217 }_{- 0.0217 } $ 

169Re $ \to {}^{165}{\rm{Ta}} $ 

5.0140 ± 0.0130 $ (9/2^{-}) \to 1/2^{+},3/2^{+} $ 

3 $ 5.2095 ^{+ 0.0260 }_{- 0.0277 } $ 

$ 5.5596 ^{+ 0.0681 }_{- 0.0684 } $ 

171Ir $ ^{\rm{m}} $ 

$ \to {}^{167}{\rm{Re}} $ 

6.1600 ± 0.0110 $ (11/2^{-}) \to (9/2^{-}) $ 

2 $ 0.4349 ^{+ 0.0174 }_{- 0.0181 } $ 

$ 0.9393 ^{+ 0.0428 }_{- 0.0429 } $ 

173Ir $ ^{\rm{m}} $ 

$ \to {}^{169}{\rm{Re}} $ 

5.9400 ± 0.0090 $ 11/2^{-} \to (9/2^{-}) $ 

2 $ 1.2632 ^{+ 0.0098 }_{- 0.0100 } $ 

$ 1.8389 ^{+ 0.0371 }_{- 0.0372 } $ 

175Ir $ \to {}^{171}{\rm{Re}} $ 

5.4300 ± 0.0300 $ 5/2^{-} \# \to 9/2^{-} $ 

2 $ 3.0248 ^{+ 0.0872 }_{- 0.1091 } $ 

$ 4.1190 ^{+ 0.1424 }_{- 0.1436 } $ 

175Pt $ \to {}^{171}{\rm{Os}} $ 

6.1640 ± 0.0040 $ (7/2^{-}) \to (5/2^{-}) $ 

2 $ 0.5794 ^{+ 0.0071 }_{- 0.0072 } $ 

$ 1.3866 ^{+ 0.0158 }_{- 0.0158 } $ 

179Pt $ \to {}^{175}{\rm{Os}} $ 

5.4120 ± 0.0090 $ 1/2^{-} \to 5/2^{-} $ 

2 $ 3.9461 ^{+ 0.0081 }_{- 0.0083 } $ 

$ 4.7148 ^{+ 0.0437 }_{- 0.0438 } $ 

185Hg $ ^{\rm{m}} $ 

$ \to {}^{181}{\rm{Pt}} $ 

5.8800 ± 0.0004 $ 13/2^{+}* \to 1/2^{-} $ 

7 $ 4.8573 ^{+ 0.0292 }_{- 0.0313 } $ 

$ 5.4483 ^{+ 0.0018 }_{- 0.0018 } $ 

171Hg $ \to {}^{167}{\rm{Pt}} $ 

7.6680 ± 0.0150 $ 3/2^{-} \# \to 7/2^{-} \# $ 

2 $ -4.1549 ^{+ 0.1549 }_{- 0.2430 } $ 

$ -2.9018 ^{+ 0.0427 }_{- 0.0429 } $ 

177Hg $ \to {}^{173}{\rm{Pt}} $ 

6.7400 ± 0.0600 $ 7/2^{-}* \to (5/2^{-}) $ 

2 $ -0.9318 ^{+ 0.0252 }_{- 0.0268 } $ 

$ 0.0879 ^{+ 0.2097 }_{- 0.2128 } $ 

181Hg $ \to {}^{177}{\rm{Pt}} $ 

6.2840 ± 0.0040 $ 1/2^{-}* \to 5/2^{-} $ 

2 $ 1.1249 ^{+ 0.0119 }_{- 0.0122 } $ 

$ 1.8190 ^{+ 0.0158 }_{- 0.0158 } $ 

181Tl $ ^{\rm{m}} $ 

$ \to {}^{177}{\rm{Au}} $ 

$ ^{\rm{m}} $ 

6.9700 ± 0.0004 $ (9/2^{-}) \to 11/2^{-} \# $ 

2 $ -0.4559 ^{+ 0.0092 }_{- 0.0094 } $ 

$ -0.2757 ^{+ 0.0014 }_{- 0.0014 } $ 

183Tl $ ^{\rm{m}} $ 

$ \to {}^{179}{\rm{Au}} $ 

6.6100 ± 0.0005 $ (9/2^{-}) \to (1/2^{+},3/2^{+}) $ 

3 $ 0.5506 ^{+ 0.0024 }_{- 0.0025 } $ 

$ 1.3978 ^{+ 0.0018 }_{- 0.0018 } $ 

187Tl $ ^{\rm{m}} $ 

$ \to {}^{183}{\rm{Au}} $ 

5.6600 ± 0.0030 $ 9/2^{-}* \to 5/2^{-} $ 

2 $ 4.0170 ^{+ 0.0033 }_{- 0.0034 } $ 

$ 5.0048 ^{+ 0.0142 }_{- 0.0142 } $ 

183Pb $ ^{\rm{m}} $ 

$ \to {}^{179}{\rm{Hg}} $ 

7.0200 ± 0.0080 $ 13/2^{+}* \to 7/2^{-} $ 

3 $ -0.3820 ^{+ 0.0204 }_{- 0.0215 } $ 

$ 0.3500 ^{+ 0.0271 }_{- 0.0271 } $ 

Continued on next page Table 5. Calculations of unfavored α decay half-lives for odd-A nuclei. The α decay energy, spin, and parity and experimental half-lives are obtained from the latest evaluated nuclear properties table NUBASE2020 [85–87]. The symbols (), *, and # denote uncertain spin and/or parity based on weak experimental arguments, directly measured spin, and non-experimental value estimated from trends in neighboring nuclei or from theoretical predictions, respectively.
Table 5-continued from previous page α decay $ Q_{\alpha} $ 

$ j_p^{\pi}\to{j_d}^{\pi} $ 

l $ \,\log_{10}{T}_{1/2}^{\rm{exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{ISM}} $ 

179Pb $ \to {}^{175}{\rm{Hg}} $ 

7.5960 ± 0.0050 $ (9/2^{-}) \to (7/2^{-}) $ 

2 $ -2.5686 ^{+ 0.0310 }_{- 0.0334 } $ 

$ -1.8997 ^{+ 0.0149 }_{- 0.0149 } $ 

181Pb $ \to {}^{177}{\rm{Hg}} $ 

7.2400 ± 0.0070 $ (9/2^{-}) \to 7/2^{-}* $ 

2 $ -1.4089 ^{+ 0.0088 }_{- 0.0090 } $ 

$ -0.7799 ^{+ 0.0226 }_{- 0.0226 } $ 

183Pb $ \to {}^{179}{\rm{Hg}} $ 

6.9280 ± 0.0070 $ 3/2^{-}* \to 7/2^{-} $ 

2 $ -0.2716 ^{+ 0.0237 }_{- 0.0251 } $ 

$ 0.2786 ^{+ 0.0242 }_{- 0.0243 } $ 

185Pb $ \to {}^{181}{\rm{Hg}} $ 

6.6950 ± 0.0050 $ 3/2^{-}* \to 1/2^{-}* $ 

2 $ 1.2679 ^{+ 0.0267 }_{- 0.0285 } $ 

$ 1.1242 ^{+ 0.0183 }_{- 0.0183 } $ 

187Pb $ \to {}^{183}{\rm{Hg}} $ 

6.3930 ± 0.0060 $ 3/2^{-}* \to 1/2^{-}* $ 

2 $ 2.2041 ^{+ 0.0085 }_{- 0.0087 } $ 

$ 2.2872 ^{+ 0.0236 }_{- 0.0237 } $ 

189Pb $ \to {}^{185}{\rm{Hg}} $ 

5.9150 ± 0.0040 $ 3/2^{-}* \to 1/2^{-}* $ 

2 $ 3.9678 ^{+ 0.0810 }_{- 0.0997 } $ 

$ 4.3079 ^{+ 0.0178 }_{- 0.0179 } $ 

187Bi $ \to {}^{183}{\rm{Tl}} $ 

7.7790 ± 0.0040 $ (9/2^{-}) \to 1/2^{+} $ 

5 $ -1.4318 ^{+ 0.0229 }_{- 0.0241 } $ 

$ -0.8588 ^{+ 0.0116 }_{- 0.0116 } $ 

189Bi $ \to {}^{185}{\rm{Tl}} $ 

7.2682 ± 0.0027 $ 9/2^{-}* \to 1/2^{+} $ 

5 $ -0.1624 ^{+ 0.0031 }_{- 0.0032 } $ 

$ 0.7272 ^{+ 0.0088 }_{- 0.0088 } $ 

209Bi $ \to {}^{205}{\rm{Tl}} $ 

3.1373 ± 0.0008 $ 9/2^{-}* \to 1/2^{+} $ 

5 $ 26.8023 ^{+ 0.0170 }_{- 0.0176 } $ 

$ 26.4780 ^{+ 0.0097 }_{- 0.0097 } $ 

211Bi $ \to {}^{207}{\rm{Tl}} $ 

6.7504 ± 0.0005 $ 9/2^{-}* \to 1/2^{+} $ 

5 $ 2.1086 ^{+ 0.0040 }_{- 0.0041 } $ 

$ 1.2617 ^{+ 0.0018 }_{- 0.0018 } $ 

213Bi $ \to {}^{209}{\rm{Tl}} $ 

5.9880 ± 0.0030 $ 9/2^{-}* \to 1/2^{+} $ 

5 $ 4.9472 ^{+ 0.0004 }_{- 0.0004 } $ 

$ 4.3256 ^{+ 0.0132 }_{- 0.0132 } $ 

211Po $ ^{\rm{m}} $ 

$ \to {}^{207}{\rm{Pb}} $ 

9.0600 ± 0.0050 $ (25/2^{+}) \to 1/2^{-} $ 

13 $ 1.4015 ^{+ 0.0102 }_{- 0.0105 } $ 

$ -1.9529 ^{+ 0.0113 }_{- 0.0113 } $ 

187Po $ \to {}^{183}{\rm{Pb}} $ 

7.9790 ± 0.0150 $ 1/2^{-},5/2^{-} \to 3/2^{-}* $ 

2 $ -2.8539 ^{+ 0.0714 }_{- 0.0854 } $ 

$ -2.2285 ^{+ 0.0424 }_{- 0.0425 } $ 

189Po $ \to {}^{185}{\rm{Pb}} $ 

7.6940 ± 0.0150 $ (5/2^{-}) \to 3/2^{-}* $ 

2 $ -2.4559 ^{+ 0.0580 }_{- 0.0669 } $ 

$ -1.3825 ^{+ 0.0450 }_{- 0.0451 } $ 

203Po $ \to {}^{199}{\rm{Pb}} $ 

5.4960 ± 0.0050 $ 5/2^{-}* \to 3/2^{-} $ 

2 $ 6.3014 ^{+ 0.0059 }_{- 0.0060 } $ 

$ 7.3565 ^{+ 0.0257 }_{- 0.0258 } $ 

211Po $ \to {}^{207}{\rm{Pb}} $ 

7.5946 ± 0.0005 $ 9/2^{+}* \to 1/2^{-} $ 

5 $ -0.2874 ^{+ 0.0025 }_{- 0.0025 } $ 

$ -1.1641 ^{+ 0.0015 }_{- 0.0015 } $ 

191At $ ^{\rm{m}} $ 

$ \to {}^{187}{\rm{Bi}} $ 

7.8800 ± 0.0200 $ (7/2^{-}) \to 9/2^{-} $ 

2 $ -2.6576 ^{+ 0.0726 }_{- 0.0872 } $ 

$ -1.5562 ^{+ 0.0584 }_{- 0.0587 } $ 

193At $ ^{\rm{m}} $ 

$ \to {}^{189}{\rm{Bi}} $ 

7.5800 ± 0.0090 $ 7/2^{-} \to (9/2^{-}) $ 

2 $ -1.6778 ^{+ 0.0928 }_{- 0.1181 } $ 

$ -0.6346 ^{+ 0.0280 }_{- 0.0281 } $ 

195At $ ^{\rm{m}} $ 

$ \to {}^{191}{\rm{Bi}} $ 

7.3700 ± 0.0070 $ 7/2^{-}* \to 9/2^{-}* $ 

2 $ -0.7891 ^{+ 0.0090 }_{- 0.0092 } $ 

$ 0.0506 ^{+ 0.0228 }_{- 0.0229 } $ 

193Rn $ \to {}^{189}{\rm{Po}} $ 

8.0400 ± 0.0120 $ (3/2^{-}) \to (5/2^{-}) $ 

2 $ -2.9393 ^{+ 0.0916 }_{- 0.1162 } $ 

$ -1.6480 ^{+ 0.0344 }_{- 0.0345 } $ 

205Rn $ \to {}^{201}{\rm{Po}} $ 

6.3865 ± 0.0180 $ 5/2^{-}* \to 3/2^{-}* $ 

2 $ 2.8395 ^{+ 0.0101 }_{- 0.0103 } $ 

$ 4.1570 ^{+ 0.0748 }_{- 0.0751 } $ 

211Rn $ \to {}^{207}{\rm{Po}} $ 

5.9655 ± 0.0001 $ 1/2^{-}* \to 5/2^{-}* $ 

2 $ 5.2829 ^{+ 0.0059 }_{- 0.0060 } $ 

$ 6.0531 ^{+ 0.0005 }_{- 0.0005 } $ 

213Rn $ \to {}^{209}{\rm{Po}} $ 

8.2452 ± 0.0029 $ 9/2^{+} \# \to 1/2^{-}* $ 

5 $ -1.7100 ^{+ 0.0022 }_{- 0.0022 } $ 

$ -2.3254 ^{+ 0.0079 }_{- 0.0079 } $ 

219Rn $ \to {}^{215}{\rm{Po}} $ 

6.9462 ± 0.0003 $ 5/2^{+}* \to 9/2^{+} $ 

2 $ 0.5670 ^{+ 0.0012 }_{- 0.0012 } $ 

$ 0.6334 ^{+ 0.0011 }_{- 0.0011 } $ 

221Rn $ \to {}^{217}{\rm{Po}} $ 

6.1630 ± 0.0030 $ 7/2^{+}* \to (9/2^{+})* $ 

2 $ 3.8457 ^{+ 0.0084 }_{- 0.0085 } $ 

$ 3.7602 ^{+ 0.0131 }_{- 0.0131 } $ 

221Fr $ \to {}^{217}{\rm{At}} $ 

6.4577 ± 0.0014 $ 5/2^{-}* \to 9/2^{-}* $ 

2 $ 2.4595 ^{+ 0.0005 }_{- 0.0005 } $ 

$ 2.9325 ^{+ 0.0058 }_{- 0.0058 } $ 

223Fr $ \to {}^{219}{\rm{At}} $ 

5.5614 ± 0.0028 $ 3/2^{-}* \to (9/2^{-})* $ 

4 $ 7.3424 ^{+ 0.0014 }_{- 0.0014 } $ 

$ 7.8699 ^{+ 0.0146 }_{- 0.0146 } $ 

213Ra $ ^{\rm{m}} $ 

$ \to {}^{209}{\rm{Rn}} $ 

$ ^{\rm{m}} $ 

7.4600 ± 0.0040 $ (17/2^{-}) \to 13/2^{+} $ 

3 $ -0.4357 ^{+ 0.0098 }_{- 0.0100 } $ 

$ 1.4137 ^{+ 0.0133 }_{- 0.0133 } $ 

219Ra $ ^{\rm{m}} $ 

$ \to {}^{215}{\rm{Rn}} $ 

8.1600 ± 0.0008 $ (11/2)^{+} \to 9/2^{+} $ 

2 $ -2.0000 ^{+ 0.1139 }_{- 0.1549 } $ 

$ -2.5235 ^{+ 0.0023 }_{- 0.0023 } $ 

207Ra $ \to {}^{203}{\rm{Rn}} $ 

7.2700 ± 0.0600 $ 5/2^{-} \to 3/2^{-} $ 

2 $ 0.2054 ^{+ 0.0532 }_{- 0.0607 } $ 

$ 1.6302 ^{+ 0.2069 }_{- 0.2097 } $ 

213Ra $ \to {}^{209}{\rm{Rn}} $ 

6.8617 ± 0.0023 $ 1/2^{-}* \to 5/2^{-}* $ 

2 $ 2.2748 ^{+ 0.0079 }_{- 0.0080 } $ 

$ 3.1590 ^{+ 0.0088 }_{- 0.0088 } $ 

215Ra $ \to {}^{211}{\rm{Rn}} $ 

8.8624 ± 0.0023 $ 9/2^{+} \# \to 1/2^{-}* $ 

5 $ -2.7775 ^{+ 0.0023 }_{- 0.0023 } $ 

$ -3.2617 ^{+ 0.0057 }_{- 0.0057 } $ 

219Ra $ \to {}^{215}{\rm{Rn}} $ 

8.1380 ± 0.0030 $ (7/2)^{+} \to 9/2^{+} $ 

2 $ -2.0458 ^{+ 0.0872 }_{- 0.1091 } $ 

$ -2.4608 ^{+ 0.0086 }_{- 0.0086 } $ 

221Ra $ \to {}^{217}{\rm{Rn}} $ 

6.8804 ± 0.0020 $ 5/2^{+}* \to 9/2^{+} $ 

2 $ 1.3979 ^{+ 0.0645 }_{- 0.0757 } $ 

$ 1.6752 ^{+ 0.0075 }_{- 0.0075 } $ 

223Ra $ \to {}^{219}{\rm{Rn}} $ 

5.9790 ± 0.0002 $ 3/2^{+}* \to 5/2^{+}* $ 

2 $ 5.9948 ^{+ 0.0000 }_{- 0.0000 } $ 

$ 5.4830 ^{+ 0.0010 }_{- 0.0009 } $ 

205Ac $ \to {}^{201}{\rm{Fr}} $ 

$ ^{\rm{n}} $ 

8.0900 ± 0.0600 $ 9/2^{-} \to 13/2^{+} $ 

3 $ -1.0969 ^{+ 0.2430 }_{- 0.6021 } $ 

$ -0.2520 ^{+ 0.1762 }_{- 0.1784 } $ 

217Ac $ ^{\rm{m}} $ 

$ \to {}^{213}{\rm{Fr}} $ 

11.8000 ± 0.0200 $ 29/2^{+} \to 9/2^{-} $ 

11 $ -4.7849 ^{+ 0.0229 }_{- 0.0241 } $ 

$ -6.4096 ^{+ 0.0306 }_{- 0.0307 } $ 

223Ac $ \to {}^{219}{\rm{Fr}} $ 

6.7832 ± 0.0010 $ (5/2^{-}) \to 9/2^{-}* $ 

2 $ 2.1047 ^{+ 0.0102 }_{- 0.0105 } $ 

$ 2.4591 ^{+ 0.0039 }_{- 0.0039 } $ 

225Ac $ \to {}^{221}{\rm{Fr}} $ 

5.9351 ± 0.0014 $ 3/2^{-} \to 5/2^{-}* $ 

2 $ 5.9330 ^{+ 0.0001 }_{- 0.0001 } $ 

$ 6.1508 ^{+ 0.0068 }_{- 0.0068 } $ 

215Th $ \to {}^{211}{\rm{Ra}} $ 

7.6650 ± 0.0040 $ (1/2^{-}) \to 5/2^{-}* $ 

2 $ 0.1303 ^{+ 0.0429 }_{- 0.0475 } $ 

$ 1.1200 ^{+ 0.0131 }_{- 0.0131 } $ 

217Th $ \to {}^{213}{\rm{Ra}} $ 

9.4350 ± 0.0040 $ 9/2^{+} \# \to 1/2^{-}* $ 

5 $ -3.6055 ^{+ 0.0069 }_{- 0.0071 } $ 

$ -3.9939 ^{+ 0.0092 }_{- 0.0092 } $ 

Continued on next page Table 5-continued from previous page α decay $ Q_{\alpha} $ 

$ j_p^{\pi}\to{j_d}^{\pi} $ 

l $ \,\log_{10}{T}_{1/2}^{\rm{exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{ISM}} $ 

221Th $ \to {}^{217}{\rm{Ra}} $ 

8.6250 ± 0.0040 $ 7/2^{+} \# \to (9/2^{+}) $ 

2 $ -2.7570 ^{+ 0.0049 }_{- 0.0050 } $ 

$ -3.1210 ^{+ 0.0106 }_{- 0.0107 } $ 

223Th $ \to {}^{219}{\rm{Ra}} $ 

7.5670 ± 0.0040 $ (5/2)^{+} \to (7/2)^{+} $ 

2 $ -0.2218 ^{+ 0.0142 }_{- 0.0147 } $ 

$ 0.0305 ^{+ 0.0132 }_{- 0.0132 } $ 

225Th $ \to {}^{221}{\rm{Ra}} $ 

6.9214 ± 0.0021 $ 3/2^{+} \to 5/2^{+}* $ 

2 $ 2.7659 ^{+ 0.0020 }_{- 0.0020 } $ 

$ 2.3376 ^{+ 0.0080 }_{- 0.0080 } $ 

227Th $ \to {}^{223}{\rm{Ra}} $ 

6.1466 ± 0.0001 $ (1/2^{+}) \to 3/2^{+}* $ 

2 $ 6.2069 ^{+ 0.0001 }_{- 0.0001 } $ 

$ 5.6064 ^{+ 0.0005 }_{- 0.0005 } $ 

229Th $ \to {}^{225}{\rm{Ra}} $ 

5.1676 ± 0.0010 $ 5/2^{+}* \to 1/2^{+} $ 

2 $ 11.3976 ^{+ 0.0009 }_{- 0.0009 } $ 

$ 10.8090 ^{+ 0.0061 }_{- 0.0061 } $ 

217Pa $ ^{\rm{m}} $ 

$ \to {}^{213}{\rm{Ac}} $ 

10.3500 ± 0.0070 $ (23/2^{-}) \to 9/2^{-}* $ 

8 $ -2.8299 ^{+ 0.0119 }_{- 0.0122 } $ 

$ -3.1270 ^{+ 0.0141 }_{- 0.0141 } $ 

225Pa $ \to {}^{221}{\rm{Ac}} $ 

7.4000 ± 0.0600 $ 5/2^{-} \# \to 9/2^{-} \# $ 

2 $ 0.2330 ^{+ 0.0247 }_{- 0.0262 } $ 

$ 0.9801 ^{+ 0.2068 }_{- 0.2095 } $ 

229Pa $ \to {}^{225}{\rm{Ac}} $ 

5.8350 ± 0.0040 $ 5/2^{+} \to 3/2^{-} $ 

1 $ 7.4366 ^{+ 0.0111 }_{- 0.0114 } $ 

$ 7.1804 ^{+ 0.0203 }_{- 0.0203 } $ 

219U $ \to {}^{215}{\rm{Th}} $ 

9.9500 ± 0.0120 $ 9/2^{+} \# \to (1/2^{-}) $ 

5 $ -4.2218 ^{+ 0.0479 }_{- 0.0539 } $ 

$ -4.5326 ^{+ 0.0257 }_{- 0.0258 } $ 

223U $ \to {}^{219}{\rm{Th}} $ 

9.1580 ± 0.0170 $ 7/2^{+} \# \to 9/2^{+} \# $ 

2 $ -4.1871 ^{+ 0.0736 }_{- 0.0886 } $ 

$ -3.8358 ^{+ 0.0419 }_{- 0.0421 } $ 

225U $ \to {}^{221}{\rm{Th}} $ 

8.0070 ± 0.0060 $ 5/2^{+} \to 7/2^{+} \# $ 

2 $ -1.2076 ^{+ 0.0272 }_{- 0.0290 } $ 

$ -0.6438 ^{+ 0.0185 }_{- 0.0185 } $ 

227U $ \to {}^{223}{\rm{Th}} $ 

7.2350 ± 0.0030 $ (3/2^{+}) \to (5/2)^{+} $ 

2 $ 1.8195 ^{+ 0.0378 }_{- 0.0414 } $ 

$ 1.9589 ^{+ 0.0109 }_{- 0.0109 } $ 

231U $ \to {}^{227}{\rm{Th}} $ 

5.5763 ± 0.0017 $ 5/2^{+} \# \to (1/2^{+}) $ 

2 $ 9.9577 ^{+ 0.0102 }_{- 0.0105 } $ 

$ 9.4219 ^{+ 0.0094 }_{- 0.0094 } $ 

227Np $ \to {}^{223}{\rm{Pa}} $ 

7.8160 ± 0.0140 $ 5/2^{+} \# \to 9/2^{-} $ 

3 $ -0.2924 ^{+ 0.0483 }_{- 0.0544 } $ 

$ 0.7166 ^{+ 0.0454 }_{- 0.0456 } $ 

229Np $ \to {}^{225}{\rm{Pa}} $ 

7.0200 ± 0.0600 $ 5/2^{+} \to 5/2^{-} \# $ 

1 $ 2.5477 ^{+ 0.0191 }_{- 0.0200 } $ 

$ 2.7734 ^{+ 0.2307 }_{- 0.2339 } $ 

231Np $ \to {}^{227}{\rm{Pa}} $ 

6.3700 ± 0.0500 $ 5/2^{+} \# \to (5/2^{-}) $ 

1 $ 5.1655 ^{+ 0.0018 }_{- 0.0018 } $ 

$ 5.5079 ^{+ 0.2248 }_{- 0.2276 } $ 

235Np $ \to {}^{231}{\rm{Pa}} $ 

5.1938 ± 0.0015 $ 5/2^{+} \to 3/2^{-}* $ 

1 $ 12.1193 ^{+ 0.0013 }_{- 0.0013 } $ 

$ 11.7891 ^{+ 0.0094 }_{- 0.0094 } $ 

237Np $ \to {}^{233}{\rm{Pa}} $ 

4.9573 ± 0.0007 $ 5/2^{+}* \to 3/2^{-} $ 

1 $ 13.8303 ^{+ 0.0014 }_{- 0.0014 } $ 

$ 13.3379 ^{+ 0.0047 }_{- 0.0047 } $ 

229Pu $ \to {}^{225}{\rm{U}} $ 

7.6000 ± 0.0600 $ 3/2^{+} \# \to 5/2^{+} $ 

2 $ 2.2601 ^{+ 0.1091 }_{- 0.1461 } $ 

$ 1.4304 ^{+ 0.2053 }_{- 0.2080 } $ 

233Pu $ \to {}^{229}{\rm{U}} $ 

6.4100 ± 0.0500 $ 5/2^{+} \# \to 3/2^{+} $ 

2 $ 6.0191 ^{+ 0.0082 }_{- 0.0084 } $ 

$ 6.1597 ^{+ 0.2252 }_{- 0.2280 } $ 

241Pu $ \to {}^{237}{\rm{U}} $ 

5.1401 ± 0.0500 $ 5/2^{+}* \to 1/2^{+} $ 

2 $ 13.2661 ^{+ 0.0009 }_{- 0.0009 } $ 

$ 13.0691 ^{+ 0.3190 }_{- 0.3239 } $ 

229Am $ \to {}^{225}{\rm{Np}} $ 

8.1400 ± 0.0500 $ 5/2^{-} \# \to 9/2^{-} \# $ 

2 $ 0.2553 ^{+ 0.2632 }_{- 0.7782 } $ 

$ 0.0197 ^{+ 0.1550 }_{- 0.1565 } $ 

233Am $ \to {}^{229}{\rm{Np}} $ 

7.0700 ± 0.3200 $ 5/2^{-} \# \to 5/2^{+} $ 

1 $ 3.6301 ^{+ 0.0969 }_{- 0.1249 } $ 

$ 3.3917 ^{+ 1.2097 }_{- 1.3012 } $ 

235Am $ \to {}^{231}{\rm{Np}} $ 

6.5760 ± 0.0130 $ 5/2^{-} \# \to 5/2^{+} \# $ 

1 $ 5.1889 ^{+ 0.0246 }_{- 0.0261 } $ 

$ 5.4578 ^{+ 0.0571 }_{- 0.0573 } $ 

237Am $ \to {}^{233}{\rm{Np}} $ 

6.2000 ± 0.0300 $ 5/2^{-} \to 5/2^{+} \# $ 

1 $ 7.2471 ^{+ 0.0047 }_{- 0.0047 } $ 

$ 7.2073 ^{+ 0.1445 }_{- 0.1456 } $ 

239Am $ \to {}^{235}{\rm{Np}} $ 

5.9224 ± 0.0014 $ 5/2^{-} \to 5/2^{+} $ 

1 $ 8.6318 ^{+ 0.0036 }_{- 0.0037 } $ 

$ 8.6143 ^{+ 0.0073 }_{- 0.0073 } $ 

241Am $ \to {}^{237}{\rm{Np}} $ 

5.6378 ± 0.0001 $ 5/2^{-}* \to 5/2^{+}* $ 

1 $ 10.1352 ^{+ 0.0006 }_{- 0.0006 } $ 

$ 10.1681 ^{+ 0.0005 }_{- 0.0007 } $ 

243Am $ \to {}^{239}{\rm{Np}} $ 

5.4391 ± 0.0009 $ 5/2^{-}* \to 5/2^{+} $ 

1 $ 11.3654 ^{+ 0.0005 }_{- 0.0005 } $ 

$ 11.3324 ^{+ 0.0054 }_{- 0.0054 } $ 

235Cm $ \to {}^{231}{\rm{Pu}} $ 

7.2800 ± 0.1000 $ 5/2^{+} \# \to (3/2^{+}) $ 

2 $ 4.0212 ^{+ 0.1549 }_{- 0.2430 } $ 

$ 3.3798 ^{+ 0.3739 }_{- 0.3823 } $ 

241Cm $ \to {}^{237}{\rm{Pu}} $ 

6.1852 ± 0.0006 $ 1/2^{+} \to 7/2^{-} $ 

3 $ 8.4524 ^{+ 0.0026 }_{- 0.0027 } $ 

$ 8.5263 ^{+ 0.0029 }_{- 0.0029 } $ 

243Cm $ \to {}^{239}{\rm{Pu}} $ 

6.1688 ± 0.0010 $ 5/2^{+}* \to 1/2^{+}* $ 

2 $ 8.9630 ^{+ 0.0015 }_{- 0.0015 } $ 

$ 8.2351 ^{+ 0.0049 }_{- 0.0049 } $ 

245Cm $ \to {}^{241}{\rm{Pu}} $ 

5.6245 ± 0.0005 $ 7/2^{+}* \to 5/2^{+}* $ 

2 $ 11.4155 ^{+ 0.0037 }_{- 0.0037 } $ 

$ 11.1376 ^{+ 0.0029 }_{- 0.0029 } $ 

247Cm $ \to {}^{243}{\rm{Pu}} $ 

5.3540 ± 0.0030 $ 9/2^{-}* \to 7/2^{+} $ 

1 $ 14.6922 ^{+ 0.0137 }_{- 0.0141 } $ 

$ 12.3633 ^{+ 0.0185 }_{- 0.0185 } $ 

245Bk $ \to {}^{241}{\rm{Am}} $ 

6.4545 ± 0.0014 $ 3/2^{-} \to 5/2^{-}* $ 

2 $ 8.5519 ^{+ 0.0026 }_{- 0.0026 } $ 

$ 7.3138 ^{+ 0.0065 }_{- 0.0065 } $ 

247Bk $ \to {}^{243}{\rm{Am}} $ 

5.8900 ± 0.0050 $ 3/2^{-} \to 5/2^{-}* $ 

2 $ 10.6390 ^{+ 0.0723 }_{- 0.0868 } $ 

$ 10.1457 ^{+ 0.0268 }_{- 0.0269 } $ 

249Bk $ \to {}^{245}{\rm{Am}} $ 

5.5210 ± 0.0014 $ 7/2^{+}* \to 5/2^{+} $ 

2 $ 12.2900 ^{+ 0.0004 }_{- 0.0004 } $ 

$ 12.2448 ^{+ 0.0083 }_{- 0.0083 } $ 

237Cf $ \to {}^{233}{\rm{Cm}} $ 

8.2200 ± 0.0500 $ 5/2^{+} \# \to 3/2^{+} \# $ 

2 $ 0.0580 ^{+ 0.0969 }_{- 0.1249 } $ 

$ 0.8682 ^{+ 0.1580 }_{- 0.1596 } $ 

243Cf $ \to {}^{239}{\rm{Cm}} $ 

7.4200 ± 0.1000 $ (1/2^{+}) \to 7/2^{-} \# $ 

3 $ 3.6654 ^{+ 0.0119 }_{- 0.0122 } $ 

$ 4.0592 ^{+ 0.3712 }_{- 0.3794 } $ 

247Cf $ \to {}^{243}{\rm{Cm}} $ 

6.5030 ± 0.0140 $ (7/2^{+}) \to 5/2^{+}* $ 

2 $ 7.5050 ^{+ 0.0042 }_{- 0.0042 } $ 

$ 7.5262 ^{+ 0.0648 }_{- 0.0650 } $ 

249Cf $ \to {}^{245}{\rm{Cm}} $ 

6.2933 ± 0.0005 $ 9/2^{-} \to 7/2^{+}* $ 

1 $ 10.0444 ^{+ 0.0025 }_{- 0.0025 } $ 

$ 8.1446 ^{+ 0.0024 }_{- 0.0024 } $ 

251Cf $ \to {}^{247}{\rm{Cm}} $ 

6.1770 ± 0.0009 $ 1/2^{+} \to 9/2^{-}* $ 

5 $ 10.4524 ^{+ 0.0208 }_{- 0.0218 } $ 

$ 10.2840 ^{+ 0.0045 }_{- 0.0045 } $ 

Continued on next page Table 5-continued from previous page α decay $ Q_{\alpha} $ 

$ j_p^{\pi}\to{j_d}^{\pi} $ 

l $ \,\log_{10}{T}_{1/2}^{\rm{exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{ISM}} $ 

249Es $ \to {}^{245}{\rm{Bk}} $ 

6.9400 ± 0.0300 $ 7/2^{+} \to 3/2^{-} $ 

3 $ 6.0317 ^{+ 0.0025 }_{- 0.0026 } $ 

$ 6.4041 ^{+ 0.1264 }_{- 0.1272 } $ 

243Fm $ \to {}^{239}{\rm{Cf}} $ 

8.6900 ± 0.0500 $ 7/2^{-} \# \to 5/2^{+} \# $ 

1 $ -0.5954 ^{+ 0.0166 }_{- 0.0173 } $ 

$ -0.2455 ^{+ 0.1476 }_{- 0.1490 } $ 

245Fm $ \to {}^{241}{\rm{Cf}} $ 

8.4400 ± 0.1000 $ 1/2^{+} \# \to 7/2^{-} \# $ 

3 $ 0.6232 ^{+ 0.1171 }_{- 0.1609 } $ 

$ 1.3091 ^{+ 0.3082 }_{- 0.3142 } $ 

247Fm $ \to {}^{243}{\rm{Cf}} $ 

8.2580 ± 0.0100 $ (7/2^{+}) \to (1/2^{+}) $ 

4 $ 1.6852 ^{+ 0.0138 }_{- 0.0142 } $ 

$ 2.2839 ^{+ 0.0322 }_{- 0.0323 } $ 

251Fm $ \to {}^{247}{\rm{Cf}} $ 

7.4255 ± 0.0010 $ 9/2^{-} \to (7/2^{+}) $ 

1 $ 6.0253 ^{+ 0.0065 }_{- 0.0066 } $ 

$ 4.0742 ^{+ 0.0038 }_{- 0.0038 } $ 

253Fm $ \to {}^{249}{\rm{Cf}} $ 

$ ^{\rm{m}} $ 

7.1979 ± 0.0010 $ 1/2^{+} \to 5/2^{+} $ 

5 $ 6.3345 ^{+ 0.0170 }_{- 0.0177 } $ 

$ 6.5312 ^{+ 0.0040 }_{- 0.0040 } $ 

257Fm $ \to {}^{253}{\rm{Cf}} $ 

6.8637 ± 0.0009 $ 9/2^{+} \to (7/2^{+}) $ 

2 $ 6.9396 ^{+ 0.0009 }_{- 0.0009 } $ 

$ 6.8056 ^{+ 0.0039 }_{- 0.0039 } $ 

247Md $ ^{\rm{m}} $ 

$ \to {}^{243}{\rm{Es}} $ 

9.0300 ± 0.0400 $ 1/2^{-} \# \to 7/2^{+} $ 

3 $ -0.4997 ^{+ 0.0645 }_{- 0.0757 } $ 

$ -0.0911 ^{+ 0.1122 }_{- 0.1130 } $ 

249Md $ ^{\rm{m}} $ 

$ \to {}^{245}{\rm{Es}} $ 

8.5400 ± 0.1000 $ (1/2^{-}) \to 3/2^{-} $ 

2 $ 0.2788 ^{+ 0.1684 }_{- 0.2788 } $ 

$ 0.9810 ^{+ 0.3058 }_{- 0.3117 } $ 

245Md $ \to {}^{241}{\rm{Es}} $ 

9.0100 ± 0.1200 $ (7/2^{-}) \to 3/2^{-} \# $ 

2 $ -0.4202 ^{+ 0.1015 }_{- 0.1326 } $ 

$ -0.4352 ^{+ 0.3354 }_{- 0.3428 } $ 

247Md $ \to {}^{243}{\rm{Es}} $ 

8.7640 ± 0.0100 $ 7/2^{-} \# \to (7/2^{+}) $ 

1 $ 0.0755 ^{+ 0.0317 }_{- 0.0342 } $ 

$ -0.1044 ^{+ 0.0296 }_{- 0.0296 } $ 

249Md $ \to {}^{245}{\rm{Es}} $ 

8.4410 ± 0.0180 $ (7/2^{-}) \to (3/2^{-}) $ 

2 $ 1.5332 ^{+ 0.0150 }_{- 0.0155 } $ 

$ 1.2896 ^{+ 0.0565 }_{- 0.0567 } $ 

251Md $ \to {}^{247}{\rm{Es}} $ 

7.9630 ± 0.0040 $ (7/2^{-}) \to (7/2^{+}) $ 

1 $ 3.4024 ^{+ 0.0231 }_{- 0.0244 } $ 

$ 2.4867 ^{+ 0.0138 }_{- 0.0138 } $ 

253Md $ \to {}^{249}{\rm{Es}} $ 

7.5730 ± 0.0080 $ (7/2^{-}) \to 7/2^{+} $ 

1 $ 5.0122 ^{+ 0.2218 }_{- 0.4771 } $ 

$ 3.9068 ^{+ 0.0300 }_{- 0.0301 } $ 

255Md $ \to {}^{251}{\rm{Es}} $ 

7.9056 ± 0.0017 $ 7/2^{-} \to 3/2^{-} $ 

2 $ 4.3644 ^{+ 0.0310 }_{- 0.0334 } $ 

$ 3.1090 ^{+ 0.0060 }_{- 0.0060 } $ 

257Md $ \to {}^{253}{\rm{Es}} $ 

7.5571 ± 0.0009 $ (7/2^{-}) \to 7/2^{+}* $ 

1 $ 5.1222 ^{+ 0.0039 }_{- 0.0040 } $ 

$ 3.9947 ^{+ 0.0034 }_{- 0.0034 } $ 

253No $ \to {}^{249}{\rm{Fm}} $ 

8.4150 ± 0.0040 $ 9/2^{-}* \to 7/2^{+} $ 

1 $ 2.2337 ^{+ 0.0055 }_{- 0.0056 } $ 

$ 1.3490 ^{+ 0.0128 }_{- 0.0128 } $ 

255No $ \to {}^{251}{\rm{Fm}} $ 

$ ^{\rm{m}} $ 

8.2320 ± 0.0030 $ (1/2^{+}) \to 5/2^{+} $ 

2 $ 2.8476 ^{+ 0.0217 }_{- 0.0228 } $ 

$ 2.3533 ^{+ 0.0099 }_{- 0.0100 } $ 

257No $ \to {}^{253}{\rm{Fm}} $ 

8.4770 ± 0.0060 $ (3/2^{+}) \to 1/2^{+} $ 

2 $ 1.4597 ^{+ 0.0088 }_{- 0.0090 } $ 

$ 1.5739 ^{+ 0.0190 }_{- 0.0190 } $ 

259No $ \to {}^{255}{\rm{Fm}} $ 

7.8540 ± 0.0050 $ 9/2^{+} \to 7/2^{+} $ 

2 $ 3.6665 ^{+ 0.0359 }_{- 0.0392 } $ 

$ 3.6834 ^{+ 0.0179 }_{- 0.0179 } $ 

257Lr $ \to {}^{253}{\rm{Md}} $ 

$ ^{\rm{p}} $ 

9.0700 ± 0.0300 $ 7/2^{-} \# \to 1/2^{-} \# $ 

4 $ 0.7782 ^{+ 0.0280 }_{- 0.0300 } $ 

$ 0.8948 ^{+ 0.0856 }_{- 0.0860 } $ 

255Lr $ ^{\rm{p}} $ 

$ \to {}^{251}{\rm{Md}} $ 

10.0200 ± 0.0220 $ (25/2^{+}) \to 7/2^{-} $ 

9 $ 0.0743 ^{+ 0.0120 }_{- 0.0124 } $ 

$ 0.2914 ^{+ 0.0532 }_{- 0.0534 } $ 

257Rf $ ^{\rm{m}} $ 

$ \to {}^{253}{\rm{No}} $ 

9.1600 ± 0.0110 $ 11/2^{-} \# \to (9/2^{-}) $ 

2 $ 0.7063 ^{+ 0.0189 }_{- 0.0197 } $ 

$ 0.1901 ^{+ 0.0312 }_{- 0.0313 } $ 

261Rf $ ^{\rm{m}} $ 

$ \to {}^{257}{\rm{No}} $ 

$ ^{\rm{p}} $ 

8.4200 ± 0.1000 $ 11/2^{-} \# \to 9/2^{+} \# $ 

1 $ 1.8692 ^{+ 0.0498 }_{- 0.0563 } $ 

$ 2.0785 ^{+ 0.3235 }_{- 0.3298 } $ 

255Rf $ \to {}^{251}{\rm{No}} $ 

9.0550 ± 0.0040 $ (9/2^{-}) \to (7/2^{+}) $ 

1 $ 0.4896 ^{+ 0.0131 }_{- 0.0135 } $ 

$ 0.0831 ^{+ 0.0116 }_{- 0.0116 } $ 

257Rf $ \to {}^{253}{\rm{No}} $ 

$ ^{\rm{m}} $ 

9.0830 ± 0.0080 $ (1/2^{+}) \to 5/2^{+} $ 

2 $ 0.7481 ^{+ 0.0170 }_{- 0.0177 } $ 

$ 0.4106 ^{+ 0.0231 }_{- 0.0231 } $ 

259Rf $ \to {}^{255}{\rm{No}} $ 

9.1300 ± 0.0700 $ 3/2^{+} \# \to (1/2^{+}) $ 

2 $ 0.4905 ^{+ 0.0409 }_{- 0.0452 } $ 

$ 0.2893 ^{+ 0.1989 }_{- 0.2015 } $ 

257Db $ \to {}^{253}{\rm{Lr}} $ 

9.2060 ± 0.0200 $ 9/2^{+} \# \to (7/2^{-}) $ 

1 $ 0.3886 ^{+ 0.0362 }_{- 0.0395 } $ 

$ -0.0145 ^{+ 0.0569 }_{- 0.0571 } $ 

259Db $ \to {}^{255}{\rm{Lr}} $ 

$ ^{\rm{m}} $ 

9.6200 ± 0.0500 $ 9/2^{+} \# \to (7/2^{-}) $ 

1 $ -0.2924 ^{+ 0.1185 }_{- 0.1635 } $ 

$ -1.1386 ^{+ 0.1320 }_{- 0.1331 } $ 

263Db $ \to {}^{259}{\rm{Lr}} $ 

8.8300 ± 0.1500 $ 9/2^{+} \# \to 1/2^{-} \# $ 

5 $ 1.8942 ^{+ 0.1174 }_{- 0.1614 } $ 

$ 2.6846 ^{+ 0.4519 }_{- 0.4646 } $ 

259Sg $ ^{\rm{m}} $ 

$ \to {}^{255}{\rm{Rf}} $ 

$ ^{\rm{m}} $ 

9.7100 ± 0.0220 $ (1/2^{+}) \to (5/2^{+}) $ 

2 $ -0.6327 ^{+ 0.0490 }_{- 0.0553 } $ 

$ -0.6748 ^{+ 0.0579 }_{- 0.0581 } $ 

263Sg $ ^{\rm{m}} $ 

$ \to {}^{259}{\rm{Rf}} $ 

$ ^{\rm{m}} $ 

9.4600 ± 0.0190 $ 7/2^{+} \# \to 3/2^{+} \# $ 

2 $ -0.3768 ^{+ 0.0928 }_{- 0.1181 } $ 

$ 0.0267 ^{+ 0.0523 }_{- 0.0524 } $ 

259Sg $ \to {}^{255}{\rm{Rf}} $ 

9.7650 ± 0.0080 $ (11/2^{-}) \to (9/2^{-}) $ 

2 $ -0.3958 ^{+ 0.0566 }_{- 0.0651 } $ 

$ -0.8192 ^{+ 0.0209 }_{- 0.0209 } $ 

261Sg $ \to {}^{257}{\rm{Rf}} $ 

9.7140 ± 0.0150 $ (3/2^{+}) \to (1/2^{+}) $ 

2 $ -0.7292 ^{+ 0.0117 }_{- 0.0120 } $ 

$ -0.6715 ^{+ 0.0395 }_{- 0.0396 } $ 

265Sg $ \to {}^{261}{\rm{Rf}} $ 

9.0500 ± 0.1200 $ 11/2^{-} \# \to 3/2^{+} \# $ 

5 $ 1.2648 ^{+ 0.0696 }_{- 0.0830 } $ 

$ 2.3659 ^{+ 0.3519 }_{- 0.3597 } $ 

261Bh $ \to {}^{257}{\rm{Db}} $ 

10.5000 ± 0.0700 $ (5/2^{-}) \to 9/2^{+} \# $ 

3 $ -1.8928 ^{+ 0.0969 }_{- 0.1249 } $ 

$ -1.9406 ^{+ 0.1629 }_{- 0.1647 } $ 

263Hs $ \to {}^{259}{\rm{Sg}} $ 

$ ^{\rm{m}} $ 

10.7300 ± 0.0800 $ 3/2^{+} \# \to (1/2^{+}) $ 

2 $ -3.0458 ^{+ 0.1597 }_{- 0.2553 } $ 

$ -2.5611 ^{+ 0.1813 }_{- 0.1835 } $ 

269Hs $ \to {}^{265}{\rm{Sg}} $ 

9.2700 ± 0.1700 $ 9/2^{+} \# \to 11/2^{-} \# $ 

1 $ 1.1761 ^{+ 0.1663 }_{- 0.2730 } $ 

$ 0.8464 ^{+ 0.4874 }_{- 0.5023 } $ 

α decay $ Q_{\alpha} $ 

$ j_p^{\pi}\to{j_d}^{\pi} $ 

l $ \,\log_{10}{T}_{1/2}^{\rm{exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{ISM}} $ 

154Ho $ ^{\rm{m}} $ 

$ \to {}^{150}{\rm{Tb}} $ 

$ ^{\rm{m}} $ 

3.8200 ± 0.0280 $ 8^{+}* \to 9^{+} $ 

2 $ 7.2695 ^{+ 0.0192 }_{- 0.0201 } $ 

$ 8.2084 ^{+ 0.1980 }_{- 0.2002 } $ 

156Lu $ ^{\rm{m}} $ 

$ \to {}^{152}{\rm{Tm}} $ 

$ ^{\rm{m}} $ 

5.7200 ± 0.2500 $ 10^{+} \to (9^{+}) $ 

2 $ -0.7033 ^{+ 0.0044 }_{- 0.0044 } $ 

$ 0.2115 ^{+ 0.9681 }_{- 1.0383 } $ 

162Ta $ \to {}^{158}{\rm{Lu}} $ 

5.0100 ± 0.0600 $ 3^{-} \# \to (2)^{-} $ 

2 $ 3.6834 ^{+ 0.0144 }_{- 0.0148 } $ 

$ 4.4207 ^{+ 0.3033 }_{- 0.3091 } $ 

158Ta $ ^{\rm{m}} $ 

$ \to {}^{154}{\rm{Lu}} $ 

$ ^{\rm{m}} $ 

6.2000 ± 0.0110 $ (9)^{+} \to 10^{+} $ 

2 $ -1.4214 ^{+ 0.0095 }_{- 0.0098 } $ 

$ -0.7339 ^{+ 0.0399 }_{- 0.0400 } $ 

160Re $ \to {}^{156}{\rm{Ta}} $ 

6.6980 ± 0.0040 $ (4^{-}) \to 2^{-} $ 

2 $ -2.2554 ^{+ 0.0049 }_{- 0.0050 } $ 

$ -1.6228 ^{+ 0.0132 }_{- 0.0132 } $ 

168Re $ \to {}^{164}{\rm{Ta}} $ 

5.0630 ± 0.0130 $ (7^{+}) \to 3^{+} $ 

4 $ 4.9445 ^{+ 0.0098 }_{- 0.0100 } $ 

$ 5.9684 ^{+ 0.0671 }_{- 0.0674 } $ 

172Ir $ \to {}^{168}{\rm{Re}} $ 

5.9910 ± 0.0100 $ (3^{-},4^{-}) \to (7^{+}) $ 

3 $ 2.3424 ^{+ 0.0286 }_{- 0.0307 } $ 

$ 2.2966 ^{+ 0.0407 }_{- 0.0408 } $ 

174Ir $ \to {}^{170}{\rm{Re}} $ 

5.6930 ± 0.0160 $ (2^{+},3^{-}) \to 8^{-},9^{-} $ 

2 $ 3.1987 ^{+ 0.0318 }_{- 0.0343 } $ 

$ 3.1919 ^{+ 0.0706 }_{- 0.0709 } $ 

170Ir $ ^{\rm{m}} $ 

$ \to {}^{166}{\rm{Re}} $ 

6.2800 ± 0.0500 $ (8^{+}) \to (7^{+}) $ 

2 $ 0.3292 ^{+ 0.0095 }_{- 0.0097 } $ 

$ 0.7592 ^{+ 0.1878 }_{- 0.1902 } $ 

174Ir $ ^{\rm{m}} $ 

$ \to {}^{170}{\rm{Re}} $ 

5.8200 ± 0.0160 $ (6,7,8,9) \to (5^{+}) $ 

2 $ 2.2923 ^{+ 0.0258 }_{- 0.0274 } $ 

$ 2.6400 ^{+ 0.0682 }_{- 0.0685 } $ 

180Au $ \to {}^{176}{\rm{Ir}} $ 

5.8310 ± 0.0070 $ (1^{+})* \to (3^{+}) $ 

2 $ 3.1342 ^{+ 0.0162 }_{- 0.0168 } $ 

$ 3.5428 ^{+ 0.0306 }_{- 0.0307 } $ 

182Au $ \to {}^{178}{\rm{Ir}} $ 

5.5250 ± 0.0040 $ (2^{+})* \to 3^{+} $ 

2 $ 4.0764 ^{+ 0.0111 }_{- 0.0114 } $ 

$ 4.9581 ^{+ 0.0191 }_{- 0.0191 } $ 

186Au $ \to {}^{182}{\rm{Ir}} $ 

4.9120 ± 0.0140 $ 3^{-}* \to 3^{+} $ 

1 $ 7.9044 ^{+ 0.0198 }_{- 0.0208 } $ 

$ 7.8056 ^{+ 0.0803 }_{- 0.0806 } $ 

184Au $ ^{\rm{m}} $ 

$ \to {}^{180}{\rm{Ir}} $ 

5.3000 ± 0.0000 $ 2^{+}* \to 5^{+} $ 

4 $ 5.5637 ^{+ 0.0126 }_{- 0.0130 } $ 

$ 6.8548 ^{+ 0.0000 }_{- 0.0000 } $ 

178Tl $ \to {}^{174}{\rm{Au}} $ 

7.0200 ± 0.0100 $ (4^{-},5^{-}) \to (3^{-}) $ 

2 $ -0.3859 ^{+ 0.0151 }_{- 0.0156 } $ 

$ -0.1792 ^{+ 0.0334 }_{- 0.0335 } $ 

184Tl $ ^{\rm{m}} $ 

$ \to {}^{180}{\rm{Au}} $ 

6.2700 ± 0.0300 $ (7^{+}) \to (1^{+})* $ 

6 $ 3.3532 ^{+ 0.0200 }_{- 0.0210 } $ 

$ 4.1511 ^{+ 0.1199 }_{- 0.1208 } $ 

186Tl $ ^{\rm{m}} $ 

$ \to {}^{182}{\rm{Au}} $ 

6.0200 ± 0.0400 $ 7^{+}* \to (2^{+}) $ 

6 $ 5.6612 ^{+ 0.0155 }_{- 0.0161 } $ 

$ 5.2031 ^{+ 0.1703 }_{- 0.1721 } $ 

186Bi $ \to {}^{182}{\rm{Tl}} $ 

7.7570 ± 0.0120 $ (3^{+}) \to (4^{-})* $ 

1 $ -1.8297 ^{+ 0.0201 }_{- 0.0210 } $ 

$ -2.0615 ^{+ 0.0350 }_{- 0.0351 } $ 

188Bi $ \to {}^{184}{\rm{Tl}} $ 

7.2640 ± 0.0050 $ (3^{+}) \to 2^{-}* $ 

1 $ -1.2218 ^{+ 0.0212 }_{- 0.0223 } $ 

$ -0.5260 ^{+ 0.0163 }_{- 0.0163 } $ 

190Bi $ \to {}^{186}{\rm{Tl}} $ 

6.8620 ± 0.0030 $ (3^{+})* \to 2^{-} $ 

1 $ 0.9128 ^{+ 0.0068 }_{- 0.0069 } $ 

$ 0.8587 ^{+ 0.0107 }_{- 0.0107 } $ 

192Bi $ \to {}^{188}{\rm{Tl}} $ 

6.3770 ± 0.0040 $ (3^{+})* \to 2^{-} $ 

1 $ 2.4599 ^{+ 0.0112 }_{- 0.0114 } $ 

$ 2.7090 ^{+ 0.0160 }_{- 0.0161 } $ 

194Bi $ \to {}^{190}{\rm{Tl}} $ 

5.9180 ± 0.0050 $ 3^{+}* \to 2^{-} $ 

1 $ 4.3150 ^{+ 0.0135 }_{- 0.0139 } $ 

$ 4.6792 ^{+ 0.0226 }_{- 0.0226 } $ 

212Bi $ \to {}^{208}{\rm{Tl}} $ 

6.2073 ± 0.0090 $ 1^{-}* \to 5^{+} $ 

5 $ 4.0047 ^{+ 0.0004 }_{- 0.0004 } $ 

$ 3.6685 ^{+ 0.0374 }_{- 0.0375 } $ 

214Bi $ \to {}^{210}{\rm{Tl}} $ 

5.6210 ± 0.0140 $ 1^{-}* \to 5^{+} $ 

5 $ 6.7548 ^{+ 0.0086 }_{- 0.0088 } $ 

$ 6.3226 ^{+ 0.0681 }_{- 0.0684 } $ 

184Bi $ ^{\rm{m}} $ 

$ \to {}^{180}{\rm{Tl}} $ 

8.3700 ± 0.1000 $ 10^{-} \# \to (4^{-})* $ 

6 $ -1.8861 ^{+ 0.0621 }_{- 0.0726 } $ 

$ -1.8293 ^{+ 0.2554 }_{- 0.2605 } $ 

186Bi $ ^{\rm{m}} $ 

$ \to {}^{182}{\rm{Tl}} $ 

$ ^{\rm{m}} $ 

7.8800 ± 0.1000 $ (10^{-}) \to (7^{+}) $ 

3 $ -2.0088 ^{+ 0.0174 }_{- 0.0181 } $ 

$ -1.6354 ^{+ 0.2819 }_{- 0.2878 } $ 

190Bi $ ^{\rm{m}} $ 

$ \to {}^{186}{\rm{Tl}} $ 

$ ^{\rm{m}} $ 

6.9700 ± 0.0400 $ 10^{-}* \to (7^{+}) $ 

3 $ 0.9473 ^{+ 0.0069 }_{- 0.0071 } $ 

$ 1.2593 ^{+ 0.1385 }_{- 0.1398 } $ 

192Bi $ ^{\rm{m}} $ 

$ \to {}^{188}{\rm{Tl}} $ 

$ ^{\rm{m}} $ 

6.4900 ± 0.0300 $ 10^{-}* \to (7^{+}) $ 

3 $ 2.5977 ^{+ 0.0044 }_{- 0.0044 } $ 

$ 3.0429 ^{+ 0.1166 }_{- 0.1175 } $ 

194Bi $ ^{\rm{n}} $ 

$ \to {}^{190}{\rm{Tl}} $ 

$ ^{\rm{m}} $ 

6.0100 ± 0.0040 $ 10^{-}* \to 7(^{+} \#) $ 

3 $ 4.7597 ^{+ 0.0148 }_{- 0.0154 } $ 

$ 5.0495 ^{+ 0.0176 }_{- 0.0177 } $ 

196Bi $ ^{\rm{n}} $ 

$ \to {}^{192}{\rm{Tl}} $ 

$ ^{\rm{n}} $ 

5.2600 ± 0.0030 $ (10^{-}) \to (8^{-}) $ 

2 $ 7.8004 ^{+ 0.0054 }_{- 0.0055 } $ 

$ 8.3532 ^{+ 0.0163 }_{- 0.0163 } $ 

212Bi $ ^{\rm{m}} $ 

$ \to {}^{208}{\rm{Tl}} $ 

6.4600 ± 0.0300 $ (8^{-},9^{-}) \to 5^{+} $ 

5 $ 3.3500 ^{+ 0.0035 }_{- 0.0035 } $ 

$ 2.6505 ^{+ 0.1166 }_{- 0.1175 } $ 

206At $ \to {}^{202}{\rm{Bi}} $ 

5.8870 ± 0.0050 $ (6)^{+}* \to 5^{+}* $ 

2 $ 5.3096 ^{+ 0.0112 }_{- 0.0115 } $ 

$ 6.2162 ^{+ 0.0234 }_{- 0.0234 } $ 

210At $ \to {}^{206}{\rm{Bi}} $ 

5.6312 ± 0.0010 $ (5)^{+}* \to 6^{+} $ 

2 $ 7.2217 ^{+ 0.0209 }_{- 0.0220 } $ 

$ 7.4863 ^{+ 0.0050 }_{- 0.0050 } $ 

212At $ \to {}^{208}{\rm{Bi}} $ 

7.8171 ± 0.0006 $ (1^{-}) \to 5^{+} $ 

5 $ -0.5031 ^{+ 0.0041 }_{- 0.0042 } $ 

$ -1.1771 ^{+ 0.0018 }_{- 0.0018 } $ 

218At $ \to {}^{214}{\rm{Bi}} $ 

6.8761 ± 0.0026 $ (2^{-},3^{-})* \to 1^{-} $ 

2 $ 0.1072 ^{+ 0.0199 }_{- 0.0209 } $ 

$ 0.7772 ^{+ 0.0094 }_{- 0.0094 } $ 

192At $ ^{\rm{m}} $ 

$ \to {}^{188}{\rm{Bi}} $ -m

$ $ 

7.6300 ± 0.0400 $ (9^{-},10^{-}) \to 7(^{+} \#) $ 

3 $ -1.0555 ^{+ 0.0286 }_{- 0.0307 } $ 

$ -0.1220 ^{+ 0.1229 }_{- 0.1239 } $ 

200At $ ^{\rm{n}} $ 

$ \to {}^{196}{\rm{Bi}} $ 

$ ^{\rm{m}} $ 

6.7700 ± 0.0030 $ (10^{-})* \to (7^{+}) $ 

3 $ 1.8819 ^{+ 0.1012 }_{- 0.1322 } $ 

$ 2.8615 ^{+ 0.0112 }_{- 0.0112 } $ 

198Fr $ \to {}^{194}{\rm{At}} $ 

7.8690 ± 0.0200 $ 3^{+} \# \to (5^{-}) $ 

3 $ -1.8239 ^{+ 0.0792 }_{- 0.0969 } $ 

$ -0.0739 ^{+ 0.0601 }_{- 0.0604 } $ 

212Fr $ \to {}^{208}{\rm{At}} $ 

6.5290 ± 0.0016 $ 5^{+}* \to 6^{+}* $ 

2 $ 3.4457 ^{+ 0.0128 }_{- 0.0132 } $ 

$ 4.3362 ^{+ 0.0065 }_{- 0.0065 } $ 

214Fr $ \to {}^{210}{\rm{At}} $ 

8.5890 ± 0.0040 $ (1^{-})* \to (5)^{+}* $ 

5 $ -2.2588 ^{+ 0.0101 }_{- 0.0104 } $ 

$ -2.6098 ^{+ 0.0103 }_{- 0.0103 } $ 

220Fr $ \to {}^{216}{\rm{At}} $ 

6.8007 ± 0.0019 $ 1^{+}* \to 1^{-} $ 

1 $ 1.4378 ^{+ 0.0047 }_{- 0.0048 } $ 

$ 1.4667 ^{+ 0.0072 }_{- 0.0072 } $ 

Continued on next page Table 6. Same as Table 5 but for unfavored α decay of odd-odd nuclei.
Table 6-continued from previous page α decay $ Q_{\alpha} $ 

$ j_p^{\pi}\to{j_d}^{\pi} $ 

l $ \,\log_{10}{T}_{1/2}^{\rm{exp}} $ 

$ \log_{10}{T}_{1/2}^{\rm{ISM}} $ 

214Fr $ ^{\rm{m}} $ 

$ \to {}^{210}{\rm{At}} $ 

8.7100 ± 0.0050 $ (8^{-}) \to 5^{+} $ 

3 $ -2.4750 ^{+ 0.0064 }_{- 0.0065 } $ 

$ -3.6851 ^{+ 0.0126 }_{- 0.0126 } $ 

218Fr $ ^{\rm{m}} $ 

$ \to {}^{214}{\rm{At}} $ 

$ ^{\rm{n}} $ 

7.8700 ± 0.0040 $ (8^{-}) \to ^{-}9 $ 

2 $ -1.6596 ^{+ 0.0098 }_{- 0.0100 } $ 

$ -1.7437 ^{+ 0.0119 }_{- 0.0119 } $ 

214Ac $ \to {}^{210}{\rm{Fr}} $ 

7.3519 ± 0.0025 $ 5^{+}* \to 6^{+}* $ 

2 $ 0.9453 ^{+ 0.0105 }_{- 0.0107 } $ 

$ 2.0735 ^{+ 0.0086 }_{- 0.0086 } $ 

216Ac $ \to {}^{212}{\rm{Fr}} $ 

9.2408 ± 0.0029 $ (1^{-}) \to 5^{+}* $ 

5 $ -3.3565 ^{+ 0.0155 }_{- 0.0161 } $ 

$ -3.5659 ^{+ 0.0068 }_{- 0.0068 } $ 

220Ac $ \to {}^{216}{\rm{Fr}} $ 

8.3480 ± 0.0040 $ (3^{-}) \to (1^{-}) $ 

2 $ -1.5791 ^{+ 0.0031 }_{- 0.0031 } $ 

$ -2.4175 ^{+ 0.0111 }_{- 0.0111 } $ 

224Ac $ \to {}^{220}{\rm{Fr}} $ 

6.3269 ± 0.0007 $ (0^{-}) \to 1^{+}* $ 

1 $ 5.0226 ^{+ 0.0243 }_{- 0.0257 } $ 

$ 4.2410 ^{+ 0.0031 }_{- 0.0031 } $ 

226Ac $ \to {}^{222}{\rm{Fr}} $ 

5.5060 ± 0.0080 $ (1^{-}) \to 2^{-} $ 

2 $ 9.2461 ^{+ 0.0018 }_{- 0.0018 } $ 

$ 8.6450 ^{+ 0.0434 }_{- 0.0435 } $ 

208Ac $ ^{\rm{m}} $ 

$ \to {}^{204}{\rm{Fr}} $ 

$ ^{\rm{m}} $ 

8.1800 ± 0.0600 $ 10^{-} \to 7^{+}* $ 

3 $ -1.5528 ^{+ 0.0969 }_{- 0.1249 } $ 

$ -0.2050 ^{+ 0.1732 }_{- 0.1753 } $ 

216Ac $ ^{\rm{m}} $ 

$ \to {}^{212}{\rm{Fr}} $ 

9.2800 ± 0.0050 $ (9^{-}) \to 5^{+}* $ 

5 $ -3.3556 ^{+ 0.0068 }_{- 0.0069 } $ 

$ -3.6574 ^{+ 0.0116 }_{- 0.0116 } $ 

222Ac $ ^{\rm{m}} $ 

$ \to {}^{218}{\rm{Fr}} $ 

$ ^{\rm{m}} $ 

7.1300 ± 0.0210 $ 5^{+} \# \to 8^{-} $ 

3 $ 1.8055 ^{+ 0.0202 }_{- 0.0212 } $ 

$ 1.8295 ^{+ 0.0753 }_{- 0.0757 } $ 

216Pa $ \to {}^{212}{\rm{Ac}} $ 

8.0990 ± 0.0110 $ 5^{+} \# \to 7^{+}* $ 

2 $ -0.9788 ^{+ 0.0580 }_{- 0.0669 } $ 

$ 0.4170 ^{+ 0.0333 }_{- 0.0333 } $ 

224Pa $ \to {}^{220}{\rm{Ac}} $ 

7.6940 ± 0.0040 $ (5^{-}) \to (3^{-}) $ 

2 $ -0.0737 ^{+ 0.0097 }_{- 0.0099 } $ 

$ 0.2740 ^{+ 0.0130 }_{- 0.0130 } $ 

228Pa $ \to {}^{224}{\rm{Ac}} $ 

6.2645 ± 0.0015 $ 3^{+} \to (0^{-}) $ 

3 $ 6.6316 ^{+ 0.0193 }_{- 0.0202 } $ 

$ 6.1807 ^{+ 0.0068 }_{- 0.0068 } $ 

230Pa $ \to {}^{226}{\rm{Ac}} $ 

5.4394 ± 0.0007 $ 2^{-} \to (1^{-}) $ 

2 $ 10.6719 ^{+ 0.0123 }_{- 0.0127 } $ 

$ 9.9957 ^{+ 0.0040 }_{- 0.0040 } $ 

218Pa $ ^{\rm{m}} $ 

$ \to {}^{214}{\rm{Ac}} $ 

9.8700 ± 0.0190 $ 1^{-} \# \to 5^{+}* $ 

5 $ -3.8239 ^{+ 0.1249 }_{- 0.1761 } $ 

$ -4.3677 ^{+ 0.0408 }_{- 0.0409 } $ 

224Np $ \to {}^{220}{\rm{Pa}} $ 

9.3290 ± 0.0300 $ 2^{-} \# \to 1^{-} \# $ 

2 $ -4.3188 ^{+ 0.1448 }_{- 0.2188 } $ 

$ -3.6495 ^{+ 0.0725 }_{- 0.0729 } $ 

228Np $ \to {}^{224}{\rm{Pa}} $ 

7.5400 ± 0.1000 $ 4^{+} \# \to (5^{-}) $ 

1 $ 2.1754 ^{+ 0.0098 }_{- 0.0100 } $ 

$ 1.1555 ^{+ 0.3411 }_{- 0.3485 } $ 

230Np $ \to {}^{226}{\rm{Pa}} $ 

6.7800 ± 0.0500 $ 4^{+} \# \to 1^{-} \# $ 

3 $ 3.9638 ^{+ 0.0274 }_{- 0.0293 } $ 

$ 4.8058 ^{+ 0.2035 }_{- 0.2059 } $ 

236Np $ \to {}^{232}{\rm{Pa}} $ 

5.0100 ± 0.0500 $ (6^{-}) \to 2^{-} $ 

4 $ 15.4797 ^{+ 0.0140 }_{- 0.0144 } $ 

$ 14.4326 ^{+ 0.3282 }_{- 0.3334 } $ 

234Am $ \to {}^{230}{\rm{Np}} $ 

6.8000 ± 0.1500 $ 0^{-} \# \to 4^{+} \# $ 

5 $ 5.5526 ^{+ 0.0147 }_{- 0.0152 } $ 

$ 6.3274 ^{+ 0.6148 }_{- 0.6370 } $ 

242Am $ ^{\rm{m}} $ 

$ \to {}^{238}{\rm{Np}} $ 

5.6400 ± 0.0800 $ 5^{-} \to 2^{+} $ 

3 $ 11.9951 ^{+ 0.0061 }_{- 0.0062 } $ 

$ 11.2331 ^{+ 0.4446 }_{- 0.4547 } $ 

234Bk $ \to {}^{230}{\rm{Am}} $ 

8.1000 ± 0.0500 $ 3^{-} \# \to 1^{-} $ 

2 $ 1.3979 ^{+ 0.0969 }_{- 0.1249 } $ 

$ 1.1697 ^{+ 0.1599 }_{- 0.1616 } $ 

240Es $ \to {}^{236}{\rm{Bk}} $ 

8.2600 ± 0.0600 $ 4^{-} \# \to 4^{+} $ 

1 $ 0.9331 ^{+ 0.1083 }_{- 0.1447 } $ 

$ 1.0039 ^{+ 0.1901 }_{- 0.1923 } $ 

242Es $ \to {}^{238}{\rm{Bk}} $ 

8.1600 ± 0.0200 $ 2^{+} \# \to 1 $ 

1 $ 1.4945 ^{+ 0.0374 }_{- 0.0409 } $ 

$ 1.3402 ^{+ 0.0649 }_{- 0.0652 } $ 

244Es $ \to {}^{240}{\rm{Bk}} $ 

7.9400 ± 0.1000 $ 6^{+} \# \to 7^{-} \# $ 

1 $ 2.8692 ^{+ 0.0446 }_{- 0.0497 } $ 

$ 2.0859 ^{+ 0.3365 }_{- 0.3435 } $ 

246Es $ \to {}^{242}{\rm{Bk}} $ 

7.6400 ± 0.1000 $ 4^{-} \# \to 3^{+} $ 

1 $ 3.6576 ^{+ 0.0280 }_{- 0.0300 } $ 

$ 3.1526 ^{+ 0.3582 }_{- 0.3659 } $ 

248Es $ \to {}^{244}{\rm{Bk}} $ 

7.1600 ± 0.0500 $ 2^{-} \# \to 4^{-} \# $ 

2 $ 5.7604 ^{+ 0.0512 }_{- 0.0580 } $ 

$ 5.3923 ^{+ 0.1999 }_{- 0.2021 } $ 

252Es $ \to {}^{248}{\rm{Bk}} $ 

6.7386 ± 0.0005 $ (4^{+}) \to 6^{+} $ 

2 $ 7.7181 ^{+ 0.0017 }_{- 0.0018 } $ 

$ 7.1995 ^{+ 0.0022 }_{- 0.0022 } $ 

254Es $ ^{\rm{m}} $ 

$ \to {}^{250}{\rm{Bk}} $ 

6.7000 ± 0.0011 $ 2^{+}* \to ^{-}2 $ 

1 $ 7.6455 ^{+ 0.0022 }_{- 0.0022 } $ 

$ 6.9912 ^{+ 0.0049 }_{- 0.0049 } $ 

244Md $ \to {}^{240}{\rm{Es}} $ 

8.9500 ± 0.0800 $ 3^{+} \# \to 4^{-} \# $ 

1 $ -0.4437 ^{+ 0.1427 }_{- 0.2139 } $ 

$ -0.3775 ^{+ 0.2269 }_{- 0.2302 } $ 

246Md $ \to {}^{242}{\rm{Es}} $ 

8.8900 ± 0.0400 $ 1^{-} \# \to 2^{+} \# $ 

1 $ -0.0362 ^{+ 0.0776 }_{- 0.0946 } $ 

$ -0.1909 ^{+ 0.1151 }_{- 0.1160 } $ 

250Md $ \to {}^{246}{\rm{Es}} $ 

8.1550 ± 0.2800 $ 2^{-} \# \to 4^{-} \# $ 

2 $ 2.8873 ^{+ 0.0310 }_{- 0.0334 } $ 

$ 2.5111 ^{+ 0.9068 }_{- 0.9590 } $ 

256Md $ \to {}^{252}{\rm{Es}} $ 

7.7400 ± 0.1100 $ (1^{-}) \to (4^{+}) $ 

3 $ 4.7048 ^{+ 0.0099 }_{- 0.0102 } $ 

$ 4.3815 ^{+ 0.3943 }_{- 0.4035 } $ 

258Md $ \to {}^{254}{\rm{Es}} $ 

7.2713 ± 0.0019 $ 8^{-} \# \to 7^{+} $ 

1 $ 6.6491 ^{+ 0.0024 }_{- 0.0024 } $ 

$ 5.4016 ^{+ 0.0076 }_{- 0.0076 } $ 

246Md $ ^{\rm{m}} $ 

$ \to {}^{242}{\rm{Es}} $ 

8.9500 ± 0.0600 $ 4^{-} \# \to 2^{+} \# $ 

3 $ 0.8876 ^{+ 0.0726 }_{- 0.0872 } $ 

$ 0.4177 ^{+ 0.1705 }_{- 0.1724 } $ 

258Md $ ^{\rm{m}} $ 

$ \to {}^{254}{\rm{Es}} $ 

$ ^{\rm{m}} $ 

7.1900 ± 0.2000 $ 1^{-} \# \to 2^{+}* $ 

1 $ 5.4548 ^{+ 0.0068 }_{- 0.0069 } $ 

$ 5.7306 ^{+ 0.7989 }_{- 0.8354 } $ 

254Lr $ \to {}^{250}{\rm{Md}} $ 

8.8200 ± 0.0080 $ 4^{+} \# \to 2^{-} \# $ 

3 $ 1.2237 ^{+ 0.0314 }_{- 0.0339 } $ 

$ 1.5103 ^{+ 0.0239 }_{- 0.0240 } $ 

256Lr $ \to {}^{252}{\rm{Md}} $ 

8.8500 ± 0.1200 $ (0^{-},3^{-}) \# \to 1^{+} $ 

1 $ 1.5162 ^{+ 0.0153 }_{- 0.0159 } $ 

$ 0.6536 ^{+ 0.3533 }_{- 0.3613 } $ 

256Db $ \to {}^{252}{\rm{Lr}} $ 

9.3400 ± 0.0300 $ 9^{-} \# \to 7^{-} \# $ 

2 $ 0.3854 ^{+ 0.0918 }_{- 0.1165 } $ 

$ 0.2843 ^{+ 0.0833 }_{- 0.0837 } $ 

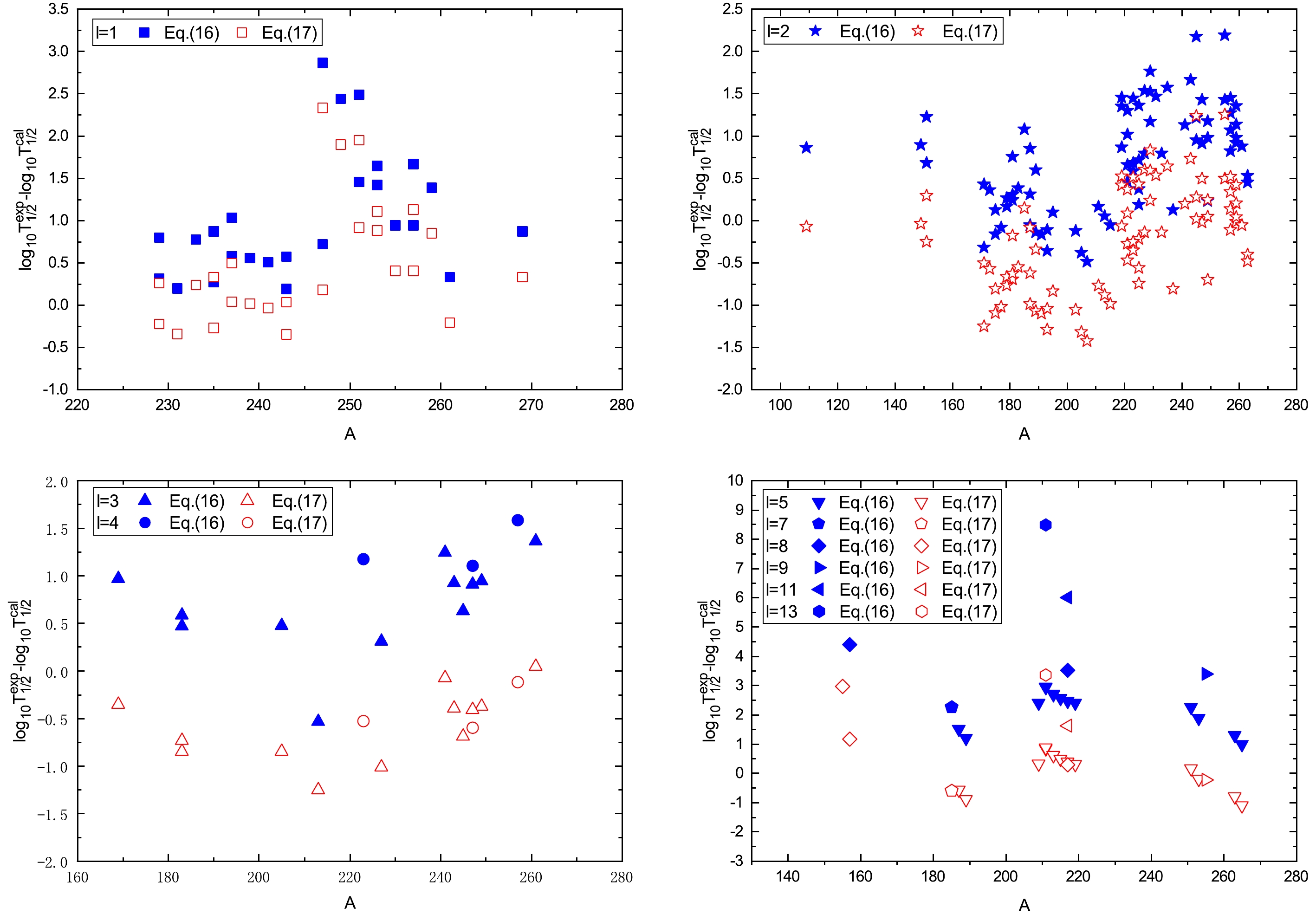
Figure 3. (color online) Deviations between the experimental data of α decay half-lives and calculated data in logarithmic form for unfavored α decay of odd-A nuclei. The different shapes represent the different angular momentums carried away by the α particle. For each angular momentums cases, the blue solid symbols represent the differences calculated using Eq. (16), whereas the red hollow symbol denote those calculated using Eq. (17).
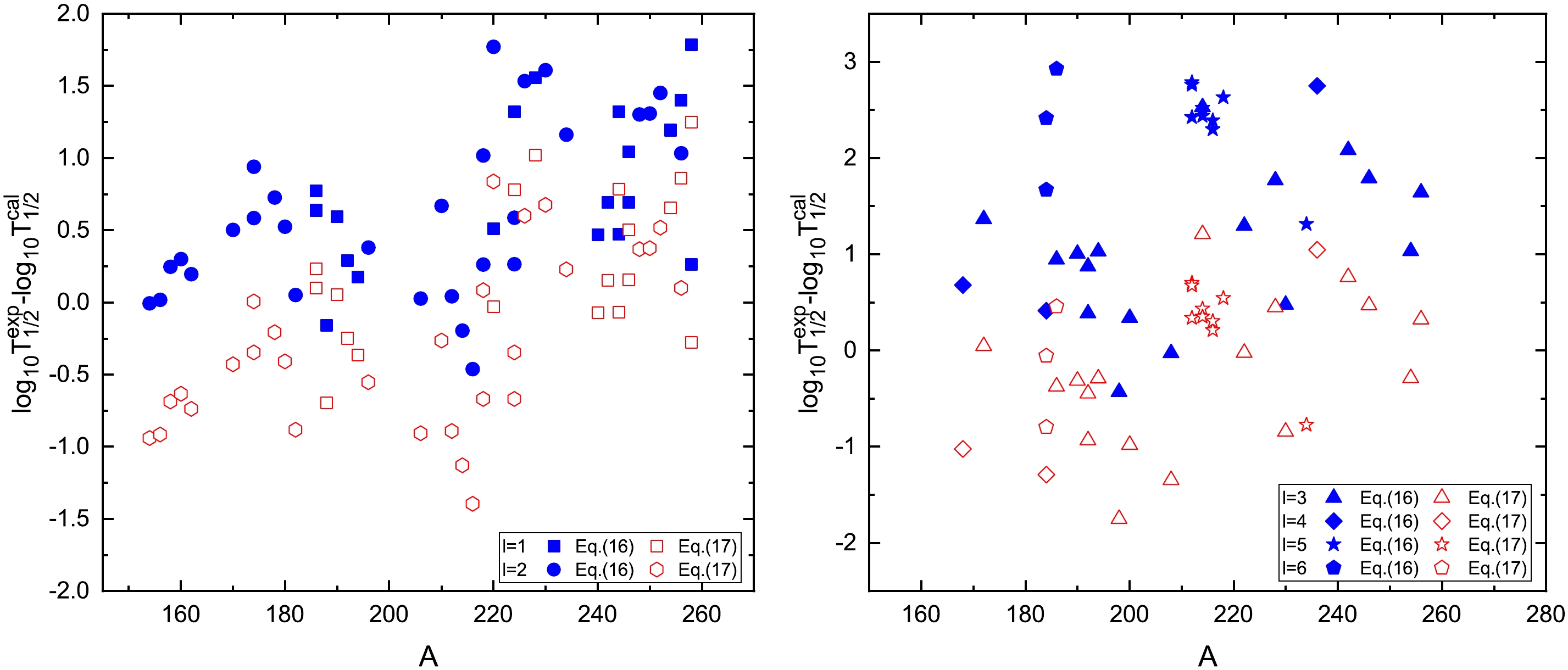
Figure 4. (color online) Same as Fig. 3 but for odd-odd nuclei.
The standard deviation σ frequently reflects the consistency between the experimental and calculated α decay half-lives, and it can be defined as
$ \sigma=\sqrt{\frac{1}{n} \sum\bigg({{\rm{log}}}_{10}T_{1/2}^{\rm{{exp}}}-{{\rm{log}}}_{10}T_{1/2}^{\rm{{cal}}}\bigg)^2}, $

(21) where n is the number of nuclei involved for each case,
$ {\rm{log}}_{10}T_{1/2}^{{\rm{exp}}} $ and$ {\rm{log}}_{10}T_{1/2}^{{\rm{cal}}} $ are the logarithmic form of experimental and calculated α decay half-lives, respectively. Subsequently, using Eq. (21), we calculate σ values for favored and unfavored α decay. The detailly calculated results are listed in the upper and bottom parts of Table 1, respectively. For favored α decay, the detailed results for 178 even-even, 231 odd-A, and 79 odd-odd nuclei are 0.322, 0.390, and 0.402 respectively. For unfavored α decay, the deviations between the experimental half-lives and those calculated using the HOPM and ISM are denoted as$ \sigma_2 $ and$ \sigma_3 $ , respectively. Compared with the original HOPM, the standard deviations obtained through our ISM for 128 odd-A and 77 odd-odd nuclei improve by$(1.292-0.592)/{1.292}=54.1$ % and$(1.339-0.621)/ {1.339}=53.6$ %, respectively, whereas the overall standard deviations for 693 nuclei is only 0.452. These results indicate that our improve model can reproduce experimental data well.Favored α decay Nuclei Even-even Odd-A Odd-odd All Cases 178 231 79 488 $ \sigma_1 $ 

0.322 0.390 0.402 0.369 Unfavored α decay Nuclei – Odd-A Odd-odd All Cases – 128 77 693 $ \sigma_2 $ 

– 1.292 1.339 – $ \sigma_3 $ 

– 0.592 0.621 0.452 Table 1. Standard deviations between experimental α decay half-lives and calculated ones using Eqs. (16) and (17) for even-even, odd-A, and odd-odd nuclei.
In the following, we extend the ISM to predict the α decay half-lives of isotope chains with
$ Z = 117,118,119 $ , and 120. For comparison, DUR [72] and MUDL [74] are also used. The predicted results are shown in Table 7. The first three columns denote α decay, the decay energy predicted using the WS3+ model [92], and the minimum angular momentum, respectively. The last three columns represent the predicted α decay half-lives in logarithmic form by using the ISM, DUR [72], and MUDL [74], denoted as$ {\rm{log}}_{10}T_{1/2}^{{\rm{ISM}}} $ ,$ {\rm{log}}_{10}T_{1/2}^{{\rm{DUR}}} $ , and$ {\rm{log}}_{10}T_{1/2}^{{\rm{MUDL}}} $ , respectively. This table shows that these predicted results are consistent with each other. For a better comparison, the predicted α decay half-lives in logarithmic form for$ Z = 117,118,119 $ , and 120 are plotted in Fig. 5. As shown in Fig. 5, when the neutron number of the daughter nucleus$ N_d <176 $ , the half-lives of α decay exhibits a slow upward trend and then gradually decreases to a minimum value at$ N_d = 178 $ . Meanwhile, the similar trend occurs at$ N_d = 184 $ . These results reflect that$ N_d= 184 $ is the possible neutron magic number, whereas$ N_d= 178 $ can be anticipated as the neutron submagic number.α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{ISM}} $ 

$ \log_{10}{T}_{1/2}^{\rm{DUR}} $ 

$ \log_{10}{T}_{1/2}^{\rm{MUDL}} $ 

α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{ISM}} $ 

$ \log_{10}{T}_{1/2}^{\rm{DUR}} $ 

$ \log_{10}{T}_{1/2}^{\rm{MUDL}} $ 

${{\bf Nuclei~ with}~ {\boldsymbol Z} {\bf = 117 }}$ 

281Ts $ \to {}^{277}{\rm{Mc}} $ 

13.52 2 $ -5.61 $ 

$ -6.29 $ 

$ -5.24 $ 

300Ts $ \to {}^{296}{\rm{Mc}} $ 

11.5 2 $ -1.28 $ 

$ -1.86 $ 

$ -0.92 $ 

282Ts $ \to {}^{278}{\rm{Mc}} $ 

13.33 2 $ -4.99 $ 

$ -5.48 $ 

$ -4.87 $ 

301Ts $ \to {}^{297}{\rm{Mc}} $ 

11.54 2 $ -1.65 $ 

$ -2.44 $ 

$ -1.04 $ 

283Ts $ \to {}^{279}{\rm{Mc}} $ 

13 2 $ -4.69 $ 

$ -5.31 $ 

$ -4.19 $ 

302Ts $ \to {}^{298}{\rm{Mc}} $ 

12.16 1 $ -3.07 $ 

$ -3.62 $ 

$ -3.05 $ 

284Ts $ \to {}^{280}{\rm{Mc}} $ 

12.64 2 $ -3.73 $ 

$ -4.13 $ 

$ -3.42 $ 

303Ts $ \to {}^{299}{\rm{Mc}} $ 

12.73 2 $ -4.07 $ 

$ -5.12 $ 

$ -3.90 $ 

285Ts $ \to {}^{281}{\rm{Mc}} $ 

12.39 2 $ -3.53 $ 

$ -4.08 $ 

$ -2.87 $ 

304Ts $ \to {}^{300}{\rm{Mc}} $ 

12.55 1 $ -3.82 $ 

$ -4.50 $ 

$ -3.98 $ 

286Ts $ \to {}^{282}{\rm{Mc}} $ 

12.19 2 $ -2.83 $ 

$ -3.20 $ 

$ -2.42 $ 

305Ts $ \to {}^{301}{\rm{Mc}} $ 

12.13 2 $ -2.88 $ 

$ -3.87 $ 

$ -2.55 $ 

287Ts $ \to {}^{283}{\rm{Mc}} $ 

11.92 2 $ -2.56 $ 

$ -3.08 $ 

$ -1.78 $ 

306Ts $ \to {}^{302}{\rm{Mc}} $ 

11.64 1 $ -1.95 $ 

$ -2.50 $ 

$ -1.83 $ 

288Ts $ \to {}^{284}{\rm{Mc}} $ 

11.81 2 $ -2.03 $ 

$ -2.37 $ 

$ -1.53 $ 

307Ts $ \to {}^{303}{\rm{Mc}} $ 

11 1 $ -0.76 $ 

$ -1.40 $ 

$ -0.16 $ 

289Ts $ \to {}^{285}{\rm{Mc}} $ 

11.79 2 $ -2.27 $ 

$ -2.81 $ 

$ -1.49 $ 

308Ts $ \to {}^{304}{\rm{Mc}} $ 

10.45 0 $ 0.37 $ 

$ 0.41 $ 

$ 0.76 $ 

290Ts $ \to {}^{286}{\rm{Mc}} $ 

11.62 2 $ -1.61 $ 

$ -1.97 $ 

$ -1.08 $ 

309Ts $ \to {}^{305}{\rm{Mc}} $ 

10.01 3 $ 2.60 $ 

$ 1.81 $ 

$ 3.66 $ 

291Ts $ \to {}^{287}{\rm{Mc}} $ 

11.46 2 $ -1.54 $ 

$ -2.07 $ 

$ -0.69 $ 

310Ts $ \to {}^{306}{\rm{Mc}} $ 

9.6 2 $ 3.70 $ 

$ 3.21 $ 

$ 4.53 $ 

292Ts $ \to {}^{288}{\rm{Mc}} $ 

11.51 2 $ -1.36 $ 

$ -1.74 $ 

$ -0.83 $ 

311Ts $ \to {}^{307}{\rm{Mc}} $ 

8.84 2 $ 5.87 $ 

$ 5.27 $ 

$ 7.24 $ 

293Ts $ \to {}^{289}{\rm{Mc}} $ 

11.33 2 $ -1.23 $ 

$ -1.79 $ 

$ -0.38 $ 

312Ts $ \to {}^{308}{\rm{Mc}} $ 

9.06 2 $ 5.42 $ 

$ 4.95 $ 

$ 6.40 $ 

294Ts $ \to {}^{290}{\rm{Mc}} $ 

11.19 2 $ -0.61 $ 

$ -0.99 $ 

$ -0.02 $ 

313Ts $ \to {}^{309}{\rm{Mc}} $ 

8.31 0 $ 6.87 $ 

$ 6.92 $ 

$ 8.23 $ 

295Ts $ \to {}^{291}{\rm{Mc}} $ 

11.12 2 $ -0.73 $ 

$ -1.30 $ 

$ 0.15 $ 

314Ts $ \to {}^{310}{\rm{Mc}} $ 

8.28 1 $ 7.82 $ 

$ 7.59 $ 

$ 8.98 $ 

296Ts $ \to {}^{292}{\rm{Mc}} $ 

11.4 2 $ -1.08 $ 

$ -1.54 $ 

$ -0.61 $ 

315Ts $ \to {}^{311}{\rm{Mc}} $ 

8.91 5 $ 6.81 $ 

$ 6.19 $ 

$ 8.29 $ 

297Ts $ \to {}^{293}{\rm{Mc}} $ 

11.54 2 $ -1.68 $ 

$ -2.37 $ 

$ -0.98 $ 

316Ts $ \to {}^{312}{\rm{Mc}} $ 

8.47 5 $ 8.66 $ 

$ 8.25 $ 

$ 10.00 $ 

298Ts $ \to {}^{294}{\rm{Mc}} $ 

11.43 2 $ -1.14 $ 

$ -1.65 $ 

$ -0.71 $ 

317Ts $ \to {}^{313}{\rm{Mc}} $ 

8.49 5 $ 8.31 $ 

$ 7.69 $ 

$ 9.90 $ 

299Ts $ \to {}^{295}{\rm{Mc}} $ 

11.39 2 $ -1.33 $ 

$ -2.04 $ 

$ -0.62 $ 

${{\bf Nuclei~ with}~ {\boldsymbol Z} {\bf = 118 }}$ 

282Og $ \to {}^{278}{\rm{Lv}} $ 

13.75 0 $ -6.78 $ 

$ -7.02 $ 

$ -6.48 $ 

301Og $ \to {}^{297}{\rm{Lv}} $ 

11.98 2 $ -2.34 $ 

$ -3.05 $ 

$ -1.77 $ 

283Og $ \to {}^{279}{\rm{Lv}} $ 

13.59 7 $ -3.56 $ 

$ -3.51 $ 

$ -2.83 $ 

302Og $ \to {}^{298}{\rm{Lv}} $ 

11.99 0 $ -3.37 $ 

$ -3.76 $ 

$ -2.92 $ 

284Og $ \to {}^{280}{\rm{Lv}} $ 

13.28 0 $ -5.96 $ 

$ -6.16 $ 

$ -5.55 $ 

303Og $ \to {}^{299}{\rm{Lv}} $ 

12.55 4 $ -2.70 $ 

$ -3.63 $ 

$ -2.24 $ 

285Og $ \to {}^{281}{\rm{Lv}} $ 

13.07 0 $ -5.49 $ 

$ -5.40 $ 

$ -5.12 $ 

304Og $ \to {}^{300}{\rm{Lv}} $ 

13.1 0 $ -5.52 $ 

$ -6.17 $ 

$ -5.48 $ 

286Og $ \to {}^{282}{\rm{Lv}} $ 

12.89 0 $ -5.25 $ 

$ -5.42 $ 

$ -4.75 $ 

305Og $ \to {}^{301}{\rm{Lv}} $ 

12.93 2 $ -4.18 $ 

$ -5.17 $ 

$ -4.02 $ 

287Og $ \to {}^{283}{\rm{Lv}} $ 

12.73 0 $ -4.85 $ 

$ -4.75 $ 

$ -4.41 $ 

306Og $ \to {}^{302}{\rm{Lv}} $ 

12.53 0 $ -4.44 $ 

$ -5.02 $ 

$ -4.25 $ 

288Og $ \to {}^{284}{\rm{Lv}} $ 

12.52 0 $ -4.53 $ 

$ -4.68 $ 

$ -3.95 $ 

307Og $ \to {}^{303}{\rm{Lv}} $ 

11.98 2 $ -2.30 $ 

$ -3.16 $ 

$ -1.86 $ 

289Og $ \to {}^{285}{\rm{Lv}} $ 

12.44 0 $ -4.28 $ 

$ -4.17 $ 

$ -3.79 $ 

308Og $ \to {}^{304}{\rm{Lv}} $ 

11.2 0 $ -1.58 $ 

$ -1.97 $ 

$ -0.98 $ 

290Og $ \to {}^{286}{\rm{Lv}} $ 

12.41 0 $ -4.31 $ 

$ -4.48 $ 

$ -3.73 $ 

309Og $ \to {}^{305}{\rm{Lv}} $ 

10.67 2 $ 0.75 $ 

$ 0.07 $ 

$ 1.60 $ 

291Og $ \to {}^{287}{\rm{Lv}} $ 

12.22 2 $ -2.90 $ 

$ -3.41 $ 

$ -2.21 $ 

310Og $ \to {}^{306}{\rm{Lv}} $ 

10.29 0 $ 0.73 $ 

$ 0.45 $ 

$ 1.61 $ 

292Og $ \to {}^{288}{\rm{Lv}} $ 

12.01 0 $ -3.48 $ 

$ -3.63 $ 

$ -2.81 $ 

311Og $ \to {}^{307}{\rm{Lv}} $ 

9.39 2 $ 4.39 $ 

$ 3.87 $ 

$ 5.66 $ 

293Og $ \to {}^{289}{\rm{Lv}} $ 

12.02 2 $ -2.47 $ 

$ -3.00 $ 

$ -1.76 $ 

312Og $ \to {}^{308}{\rm{Lv}} $ 

9.06 0 $ 4.44 $ 

$ 4.30 $ 

$ 5.73 $ 

294Og $ \to {}^{290}{\rm{Lv}} $ 

11.87 0 $ -3.17 $ 

$ -3.34 $ 

$ -2.50 $ 

313Og $ \to {}^{309}{\rm{Lv}} $ 

8.76 0 $ 5.57 $ 

$ 5.71 $ 

$ 6.86 $ 

295Og $ \to {}^{291}{\rm{Lv}} $ 

11.7 0 $ -2.71 $ 

$ -2.60 $ 

$ -2.09 $ 

314Og $ \to {}^{310}{\rm{Lv}} $ 

8.5 0 $ 6.43 $ 

$ 6.30 $ 

$ 7.88 $ 

296Og $ \to {}^{292}{\rm{Lv}} $ 

11.56 0 $ -2.48 $ 

$ -2.65 $ 

$ -1.75 $ 

315Og $ \to {}^{311}{\rm{Lv}} $ 

8.4 3 $ 8.22 $ 

$ 7.65 $ 

$ 9.85 $ 

297Og $ \to {}^{293}{\rm{Lv}} $ 

12 0 $ -3.34 $ 

$ -3.33 $ 

$ -2.87 $ 

316Og $ \to {}^{312}{\rm{Lv}} $ 

8.47 0 $ 6.55 $ 

$ 6.38 $ 

$ 7.97 $ 

298Og $ \to {}^{294}{\rm{Lv}} $ 

12.12 0 $ -3.67 $ 

$ -3.99 $ 

$ -3.17 $ 

317Og $ \to {}^{313}{\rm{Lv}} $ 

8.09 3 $ 9.44 $ 

$ 8.86 $ 

$ 11.15 $ 

299Og $ \to {}^{295}{\rm{Lv}} $ 

11.99 2 $ -2.37 $ 

$ -3.04 $ 

$ -1.77 $ 

318Og $ \to {}^{314}{\rm{Lv}} $ 

8.48 0 $ 6.53 $ 

$ 6.31 $ 

$ 7.91 $ 

300Og $ \to {}^{296}{\rm{Lv}} $ 

11.91 0 $ -3.22 $ 

$ -3.54 $ 

$ -2.69 $ 

Continued on next page Table 7-continued from previous page α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{ISM}} $ 

$ \log_{10}{T}_{1/2}^{\rm{DUR}} $ 

$ \log_{10}{T}_{1/2}^{\rm{MUDL}} $ 

α decay $ Q_{\alpha} $ 

l $ \log_{10}{T}_{1/2}^{\rm{ISM}} $ 

$ \log_{10}{T}_{1/2}^{\rm{DUR}} $ 

$ \log_{10}{T}_{1/2}^{\rm{MUDL}} $ 

${{\bf Nuclei~ with}~ {\boldsymbol Z} {\bf = 119 } }$ 

284119 $ \to {}^{280}{\rm{Ts}} $ 

13.77 4 $ -4.49 $ 

$ -5.02 $ 

$ -4.22 $ 

302119 $ \to {}^{298}{\rm{Ts}} $ 

12.4 0 $ -3.58 $ 

$ -3.60 $ 

$ -3.54 $ 

285119 $ \to {}^{281}{\rm{Ts}} $ 

13.65 2 $ -5.34 $ 

$ -6.00 $ 

$ -4.90 $ 

303119 $ \to {}^{299}{\rm{Ts}} $ 

12.37 2 $ -2.87 $ 

$ -3.71 $ 

$ -2.38 $ 

286119 $ \to {}^{282}{\rm{Ts}} $ 

13.46 2 $ -4.72 $ 

$ -5.19 $ 

$ -4.53 $ 

304119 $ \to {}^{300}{\rm{Ts}} $ 

12.9 1 $ -3.99 $ 

$ -4.60 $ 

$ -4.07 $ 

287119 $ \to {}^{283}{\rm{Ts}} $ 

13.28 2 $ -4.69 $ 

$ -5.32 $ 

$ -4.17 $ 

305119 $ \to {}^{301}{\rm{Ts}} $ 

13.45 2 $ -4.87 $ 

$ -5.98 $ 

$ -4.79 $ 

288119 $ \to {}^{284}{\rm{Ts}} $ 

13.17 2 $ -4.19 $ 

$ -4.65 $ 

$ -3.95 $ 

306119 $ \to {}^{302}{\rm{Ts}} $ 

13.25 0 $ -5.16 $ 

$ -5.44 $ 

$ -5.50 $ 

289119 $ \to {}^{285}{\rm{Ts}} $ 

13.08 2 $ -4.31 $ 

$ -4.96 $ 

$ -3.77 $ 

307119 $ \to {}^{303}{\rm{Ts}} $ 

12.82 0 $ -4.65 $ 

$ -5.05 $ 

$ -4.58 $ 

290119 $ \to {}^{286}{\rm{Ts}} $ 

12.92 2 $ -3.72 $ 

$ -4.18 $ 

$ -3.44 $ 

308119 $ \to {}^{304}{\rm{Ts}} $ 

12.07 0 $ -2.86 $ 

$ -2.97 $ 

$ -2.85 $ 

291119 $ \to {}^{287}{\rm{Ts}} $ 

12.87 2 $ -3.91 $ 

$ -4.57 $ 

$ -3.34 $ 

309119 $ \to {}^{305}{\rm{Ts}} $ 

11.34 3 $ -0.22 $ 

$ -1.10 $ 

$ 0.57 $ 

292119 $ \to {}^{288}{\rm{Ts}} $ 

12.71 2 $ -3.31 $ 

$ -3.78 $ 

$ -3.00 $ 

310119 $ \to {}^{306}{\rm{Ts}} $ 

10.83 3 $ 1.31 $ 

$ 0.67 $ 

$ 1.97 $ 

293119 $ \to {}^{289}{\rm{Ts}} $ 

12.51 2 $ -3.21 $ 

$ -3.84 $ 

$ -2.56 $ 

311119 $ \to {}^{307}{\rm{Ts}} $ 

10.6 0 $ 0.30 $ 

$ 0.20 $ 

$ 1.05 $ 

294119 $ \to {}^{290}{\rm{Ts}} $ 

12.52 2 $ -2.93 $ 

$ -3.41 $ 

$ -2.60 $ 

312119 $ \to {}^{308}{\rm{Ts}} $ 

10.44 2 $ 1.95 $ 

$ 1.41 $ 

$ 2.63 $ 

295119 $ \to {}^{291}{\rm{Ts}} $ 

12.57 2 $ -3.31 $ 

$ -4.01 $ 

$ -2.73 $ 

313119 $ \to {}^{309}{\rm{Ts}} $ 

9.5 2 $ 4.38 $ 

$ 3.78 $ 

$ 5.67 $ 

296119 $ \to {}^{292}{\rm{Ts}} $ 

12.31 2 $ -2.50 $ 

$ -2.99 $ 

$ -2.14 $ 

314119 $ \to {}^{310}{\rm{Ts}} $ 

9.12 1 $ 5.51 $ 

$ 5.30 $ 

$ 6.55 $ 

297119 $ \to {}^{293}{\rm{Ts}} $ 

12.3 2 $ -2.76 $ 

$ -3.45 $ 

$ -2.13 $ 

315119 $ \to {}^{311}{\rm{Ts}} $ 

9.31 1 $ 4.60 $ 

$ 4.17 $ 

$ 5.84 $ 

298119 $ \to {}^{294}{\rm{Ts}} $ 

12.59 2 $ -3.04 $ 

$ -3.64 $ 

$ -2.82 $ 

316119 $ \to {}^{312}{\rm{Ts}} $ 

8.77 2 $ 7.12 $ 

$ 6.72 $ 

$ 8.33 $ 

299119 $ \to {}^{295}{\rm{Ts}} $ 

12.73 2 $ -3.60 $ 

$ -4.42 $ 

$ -3.15 $ 

317119 $ \to {}^{313}{\rm{Ts}} $ 

9.25 3 $ 5.59 $ 

$ 4.85 $ 

$ 6.97 $ 

300119 $ \to {}^{296}{\rm{Ts}} $ 

12.53 2 $ -2.91 $ 

$ -3.54 $ 

$ -2.71 $ 

318119 $ \to {}^{314}{\rm{Ts}} $ 

9.18 0 $ 4.80 $ 

$ 4.92 $ 

$ 5.63 $ 

301119 $ \to {}^{297}{\rm{Ts}} $ 

12.37 0 $ -3.81 $ 

$ -3.99 $ 

$ -3.46 $ 

319119 $ \to {}^{315}{\rm{Ts}} $ 

8.48 6 $ 9.45 $ 

$ 9.15 $ 

$ 11.30 $ 

${{\bf Nuclei~ with}~ {\boldsymbol Z} \bf{= 120} }$ 

287120 $ \to {}^{283}{\rm{Og}} $ 

13.91 4 $ -4.76 $ 

$ -5.43 $ 

$ -4.21 $ 

304120 $ \to {}^{300}{\rm{Og}} $ 

12.75 0 $ -4.38 $ 

$ -4.82 $ 

$ -4.03 $ 

288120 $ \to {}^{284}{\rm{Og}} $ 

13.75 0 $ -6.28 $ 

$ -6.53 $ 

$ -5.92 $ 

305120 $ \to {}^{301}{\rm{Og}} $ 

13.27 2 $ -4.32 $ 

$ -5.24 $ 

$ -4.08 $ 

289120 $ \to {}^{285}{\rm{Og}} $ 

13.68 0 $ -6.07 $ 

$ -6.06 $ 

$ -5.80 $ 

306120 $ \to {}^{302}{\rm{Og}} $ 

13.82 0 $ -6.29 $ 

$ -6.99 $ 

$ -6.34 $ 

290120 $ \to {}^{286}{\rm{Og}} $ 

13.63 0 $ -6.07 $ 

$ -6.34 $ 

$ -5.71 $ 

307120 $ \to {}^{303}{\rm{Og}} $ 

13.58 4 $ -4.08 $ 

$ -5.16 $ 

$ -3.83 $ 

291120 $ \to {}^{287}{\rm{Og}} $ 

13.41 2 $ -4.66 $ 

$ -5.26 $ 

$ -4.16 $ 

308120 $ \to {}^{304}{\rm{Og}} $ 

13.04 0 $ -4.90 $ 

$ -5.49 $ 

$ -4.74 $ 

292120 $ \to {}^{288}{\rm{Og}} $ 

13.31 0 $ -5.49 $ 

$ -5.75 $ 

$ -5.08 $ 

309120 $ \to {}^{305}{\rm{Og}} $ 

12.17 0 $ -3.10 $ 

$ -3.27 $ 

$ -2.75 $ 

293120 $ \to {}^{289}{\rm{Og}} $ 

13.24 2 $ -4.34 $ 

$ -4.96 $ 

$ -3.83 $ 

310120 $ \to {}^{306}{\rm{Og}} $ 

11.48 0 $ -1.67 $ 

$ -2.02 $ 

$ -1.02 $ 

294120 $ \to {}^{290}{\rm{Og}} $ 

13.07 0 $ -5.05 $ 

$ -5.31 $ 

$ -4.59 $ 

311120 $ \to {}^{307}{\rm{Og}} $ 

11.1 2 $ 0.26 $ 

$ -0.40 $ 

$ 1.12 $ 

295120 $ \to {}^{291}{\rm{Og}} $ 

13.1 2 $ -4.07 $ 

$ -4.72 $ 

$ -3.56 $ 

312120 $ \to {}^{308}{\rm{Og}} $ 

11.05 0 $ -0.63 $ 

$ -0.96 $ 

$ 0.13 $ 

296120 $ \to {}^{292}{\rm{Og}} $ 

13.19 0 $ -5.25 $ 

$ -5.59 $ 

$ -4.88 $ 

313120 $ \to {}^{309}{\rm{Og}} $ 

10.92 2 $ 0.72 $ 

$ 0.04 $ 

$ 1.60 $ 

297120 $ \to {}^{293}{\rm{Og}} $ 

13.02 0 $ -4.84 $ 

$ -4.90 $ 

$ -4.53 $ 

314120 $ \to {}^{310}{\rm{Og}} $ 

9.97 0 $ 2.26 $ 

$ 2.07 $ 

$ 3.37 $ 

298120 $ \to {}^{294}{\rm{Og}} $ 

12.9 0 $ -4.70 $ 

$ -5.03 $ 

$ -4.28 $ 

315120 $ \to {}^{311}{\rm{Og}} $ 

10.15 0 $ 1.84 $ 

$ 1.87 $ 

$ 2.77 $ 

299120 $ \to {}^{295}{\rm{Og}} $ 

13.19 0 $ -5.14 $ 

$ -5.28 $ 

$ -4.93 $ 

316120 $ \to {}^{312}{\rm{Og}} $ 

9.97 0 $ 2.28 $ 

$ 2.03 $ 

$ 3.34 $ 

300120 $ \to {}^{296}{\rm{Og}} $ 

13.29 0 $ -5.41 $ 

$ -5.86 $ 

$ -5.16 $ 

317120 $ \to {}^{313}{\rm{Og}} $ 

9.96 3 $ 3.72 $ 

$ 3.03 $ 

$ 4.94 $ 

301120 $ \to {}^{297}{\rm{Og}} $ 

13.03 2 $ -3.90 $ 

$ -4.68 $ 

$ -3.50 $ 

318120 $ \to {}^{314}{\rm{Og}} $ 

9.99 0 $ 2.23 $ 

$ 1.94 $ 

$ 3.24 $ 

302120 $ \to {}^{298}{\rm{Og}} $ 

12.88 0 $ -4.64 $ 

$ -5.06 $ 

$ -4.30 $ 

319120 $ \to {}^{315}{\rm{Og}} $ 

9.89 3 $ 3.94 $ 

$ 3.21 $ 

$ 5.14 $ 

303120 $ \to {}^{299}{\rm{Og}} $ 

12.8 2 $ -3.46 $ 

$ -4.24 $ 

$ -3.02 $ 

320120 $ \to {}^{316}{\rm{Og}} $ 

9.71 0 $ 3.08 $ 

$ 2.78 $ 

$ 4.15 $ 

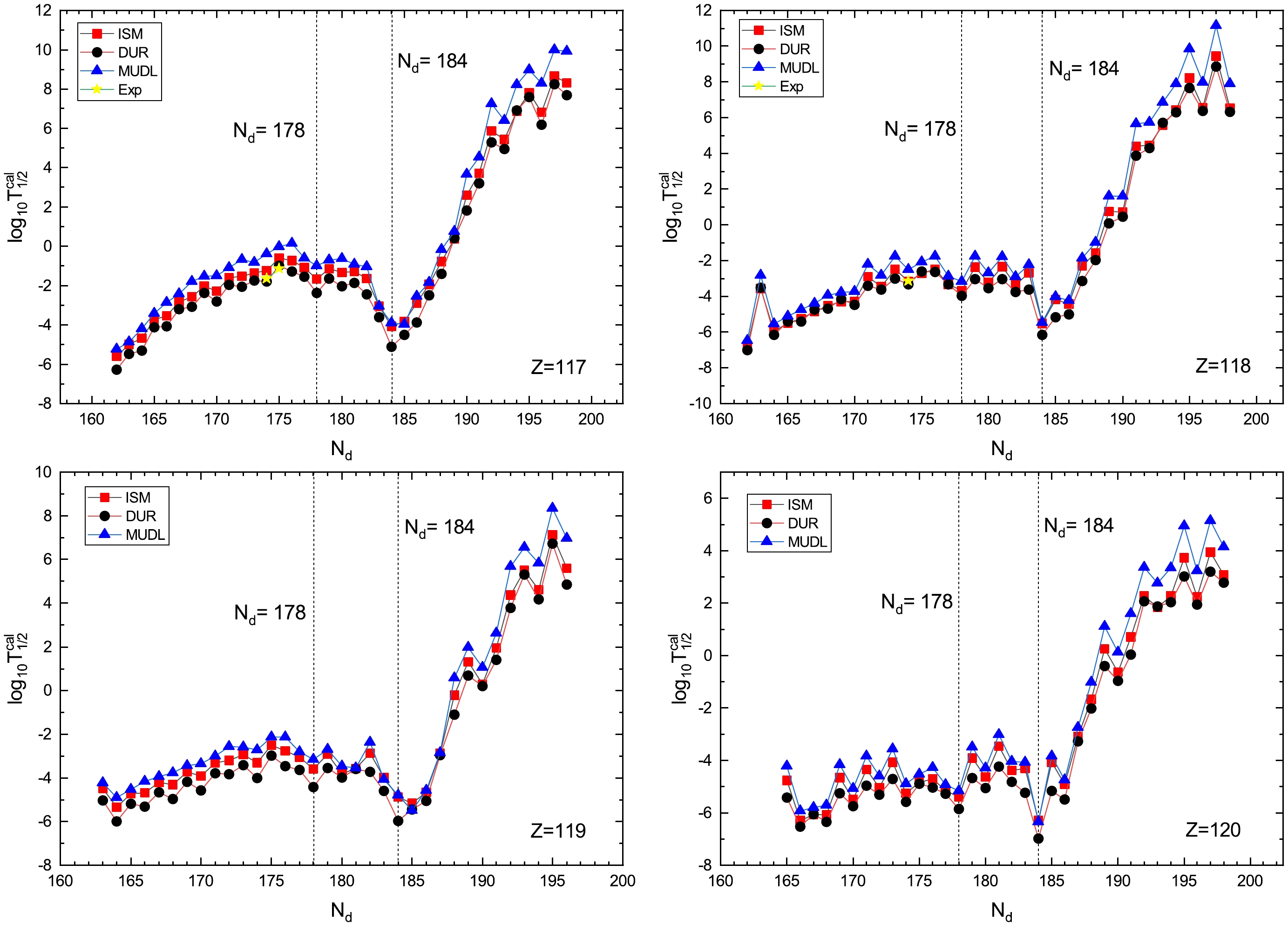
Figure 5. (color online) Predicted α decay half-lives in logarithmic form for 144 nuclei with
$ Z = 117,118,119 $ , and 120 using the ISM, DUR, and MUDL, with$ Q_{\alpha} $ obtained using WS3+. The red squares, green circles, blue triangles, and purple stars denote the predictions using the ISM, DUR, and MUDL, and the experimental ones, respectively. -
In this paper, taking the α particle preformation probability and reobtaining only one adjustable parameter in the HOPM, we calculate the favored α decay half-lives of even-even, odd-A, and odd-odd nuclei. The corresponding results can reproduce experimental data well. To consider the contribution of centrifugal potential to unfavored α decay half-lives, we propose an improved simple model (ISM) to uniformly study α decay half-lives. Compared with the original HOPM, the results calculated using the ISM are more consistent with experimental half-lives, and the corresponding deviations is only 0.452 for all 693 nuclei. As an application, the improved model ISM is also used to predict the α decay half-lives of 144 nuclei with
$ Z = 117,118,119 $ , and 120. The DUR and MUDL are also used for comparison; the corresponding predictions are generally consistent with each other. Meanwhile, the predicted results show that$ N=178 $ and$ N=184 $ are the probable neutron submagic and magic numbers, respectively. The predictions can provide useful references for experiments on synthesising new isotopes and elements in the future.
An improved simple model for α decay half-lives
- Received Date: 2024-01-29
- Available Online: 2024-07-15
Abstract: In this paper, using the α particle preformation probabilities





 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF






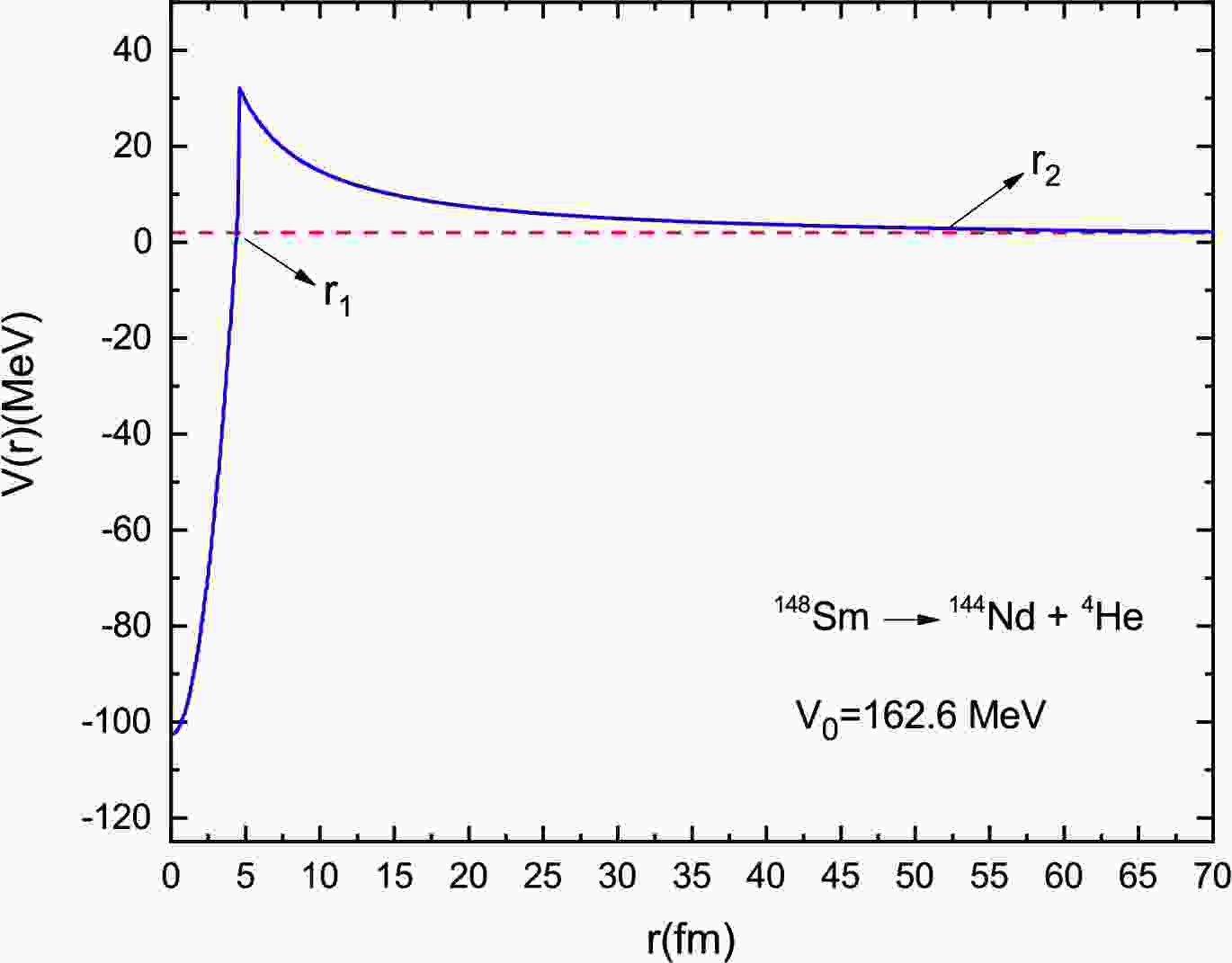













 DownLoad:
DownLoad: