Measurement of differential cross sections of neutron-induced deuteron production reactions on carbon from 25 to 52 MeV

The neutron-induced light-charged particle emitting reactions of carbon in several tens of MeV neutron energy, i.e., ¹²C(n, lcp) reactions, can offer information about the nuclear structure, and their angle-differential cross section (hereinafter referred to as differential cross section) data are needed to test the nuclear reaction models [1, 2]. Among these (n, lcp) reactions, deuteron production (¹²C(n, xd)) reactions are rather crucial because their differential cross sections are required for dose calculations in human tissues for fast neutron cancer therapy and cosmic-ray-induced neutrons considering the large amount of carbon in human tissues [1, 3-8]. Meanwhile, charged particle production reactions are of great significance to nuclear detection. Taking carbon-based neutron detectors such as plastic scintillator detectors as an example [9], precise cross sections and differential cross sections of ¹²C(n, xd) reactions are required in the simulation of the response of the detectors to fast neutrons. In addition, the cross sections of the ¹²C(n, d₀)¹¹B and ¹²C(n, d₁)¹¹B reactions were measured by Pillon et al. [10], and the cross section of the ¹²C(n, d₀)¹¹B reaction was recently measured by Majerle et al. [11]. Diamond detectors were used in both measurements, which suggests that deuteron production reactions can contribute to the response of diamond detectors. This importance necessitates the study of the differential cross sections of ¹³C(n, xd) reactions, which are referred to as ¹²C(n, xd) reactions hereinafter in accordance with literature because of the dominance of ¹²C over ¹³C in abundance (98.93% vs 1.07%) [12].

However, the cross sections of the ¹²C (n, xd) reactions are rather small, which creates difficulties in their measurement [13]. There are nine previous measurements [14], seven of which are in the several tens of MeV range: the works by Slypen et al. at E_n = 29.5 to 72.8 MeV [13, 15], as well as those by Subramanian et al. at E_n = 27.4 to 60.7 MeV [16], Tippawan et al. at E_n = 95.6 MeV [1], Bergenwall et al. at E_n = 95.6 MeV [2], McNaughton et al. at E_n = 56.0 MeV [9], and Nauchi et al. at E_n = 64.5 and 75.0 MeV [17] were all aimed at the measurement of the double differential cross sections of ¹²C (n, xd) reactions, while the work by Young et al. for E_n = 60.0 MeV [18] was aimed in particular at the measurement of the differential cross sections of the ¹²C(n, d)¹¹B reaction. All seven measurements under 100 MeV were carried out on ⁷Li(p, n) mono-energetic neutron sources. The results from previous measurements agree in trend but relatively large discrepancies in magnitude exist in some of them. For example, McNaughton’s experimental data at E_n = 56.0 MeV [9] are considerably larger than Slypen’s at E_n = 55.3 [13] MeV and at forward deuteron angles. Brenner et al. carried out comprehensive theoretical calculations of double differential cross sections by using intranuclear cascade (INCA) code [19], and there are some discrepancies between the theoretical calculations and experimental results [13]. Therefore, more measurements are needed, especially measurements using a white neutron source, which can provide systematicness. The China Spallation Neutron Source (CSNS) Back-n white neutron source provides neutrons of a wide energy range, which is suitable for measurements of neutron-induced nuclear reaction data [20].

In the present work, differential cross sections of ¹²C(n, xd) reactions relative to those of n-p elastic scattering were measured at six neutron energies from 25 to 52 MeV. A graphite foil and a polyethylene foil were used as samples for the ¹²C(n, xd) reactions and the n-p scattering measurements, respectively. The ΔE-E telescopes of the Light-charged Particle Detector Array (LPDA) system, each consisting of a Si-PIN detector (ΔE unit) and a CsI detector (E unit) placed in the forward direction from 10.1° to 55.0° with respect to the incident neutron, were employed. Measurements of the carbon sample for the ¹²C(n, xd) reactions and the polyethylene sample for the n-p elastic scattering were performed. Ratios of the differential cross sections of the ¹²C(n, xd) reactions to those of the n-p scattering were measured, and then the differential cross sections of the ¹²C(n, xd) reactions were obtained using the differential cross sections of the n-p scattering [21] from the JENDL-4.0/HE-2015 library [22] as the standard.

The measurements were performed at Endstation #1 (ES#1) of the CSNS Back-n white neutron source [23]. Protons accelerated up to 1.6 GeV in the Rapid Cycling Synchrotron (RCS) impinged on a tungsten target with a repetition rate of 25 Hz and thus produce spallation neutrons. The Back-n beamline provided neutrons of a wide energy spectrum (from ~eV to higher than 100 MeV) while the interference of the charged particles emitted from the tungsten target was eliminated by the bending magnet. The neutron source operated in double-bunch mode, with the time width of each proton bunch ~43 ns, and the separation duration between the two proton bunches was 410 ns [24]. The beam power during the present experiment was 50 kW. The neutron flight path was 57.99 m from the source to the sample. The diameter of the neutron beam spot was approximately 15 mm, and the neutron flux was ~ 9.35×10⁵ n/cm²/s. More details about the facility are presented in Ref. [25].

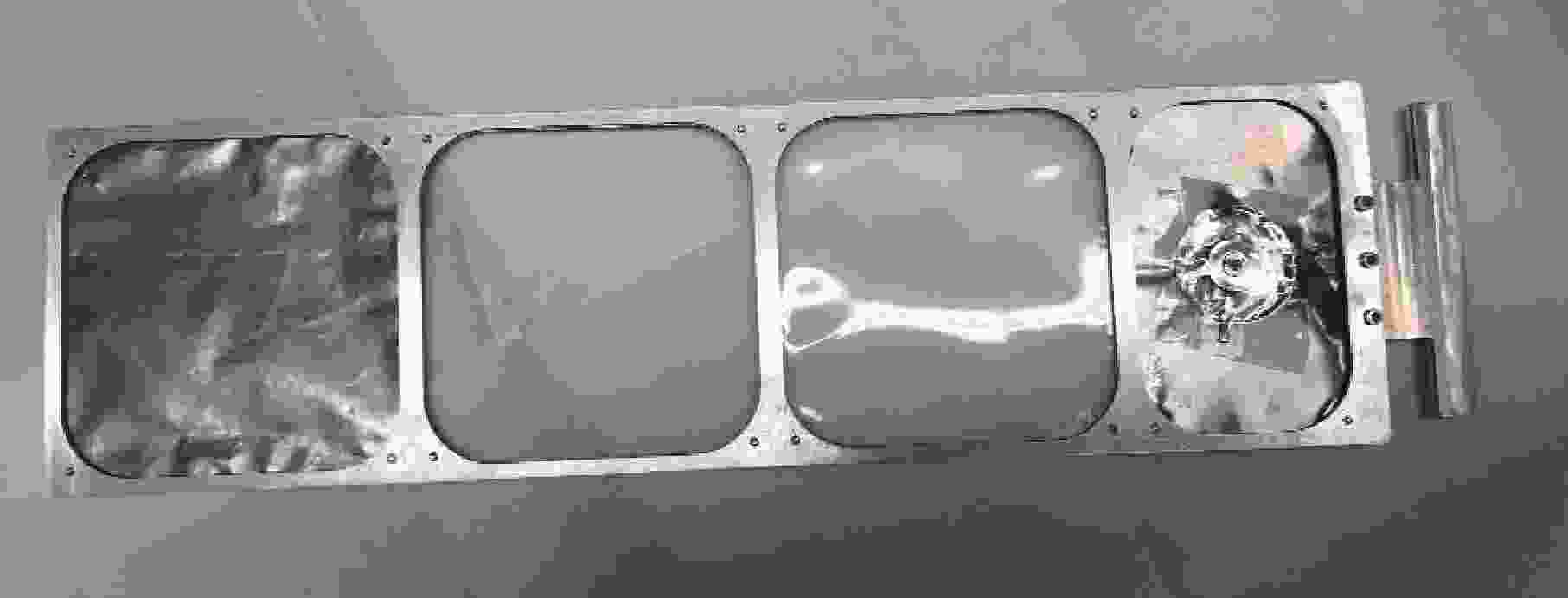
A picture of the samples and the sample holder is shown in Fig. 1.

Figure 1.  (color online) Samples and sample holder. From left to right: carbon sample, empty target, CH₂ sample, and α sources.

Four samples were used for the measurement: a high-purity graphite foil (carbon sample) of ~8.82 μg/cm² thickness for the foreground measurement of the ¹²C(n, xd) reactions, a low-density polyethylene (CH₂) sample of ~9.48 μg/cm² thickness for the n-p scattering measurement, an empty target for instrumental and neutron beam background measurement, and α sources for signal tests for the detectors, their electronics, and the data acquisition (DAQ) system. The α sources were back-to-back ²⁴¹Am sources, each with ~33 kBq activity. All the samples were mounted onto a customized aluminum holder, which has four sample positions. Three of them are rounded squares of 76.8 mm in side length for the graphite foil, empty target, and CH₂ sample, and one is a rectangle for the α sources. Therefore, the incident neutron beam spot was well covered by the sample (15 mm vs 76.8 mm).

The measurement of the charged particles was achieved using the LPDA detector system. A picture of the interior of the LPDA vacuum chamber and the configuration of the detectors are presented in Fig. 2 (a) and (b), respectively. Ten sets of ΔE-E telescopes of the LPDA system were used in the vacuum chamber. Each telescope is composed of a 300 μm-thick Si-PIN detector (ΔE unit) and a 30 mm-thick CsI scintillator (E unit). The signals from each Si-PIN detector were amplified and pre-processed by a Mesytec MSI-8 amplifier [26], while the signals from each CsI detector were multiplied by a silicon photomultiplier (SiPM) [27] and then processed by a main amplifier. Detailed descriptions of the LPDA system and the electronics can be found in Refs. [28, 29]. The telescopes were placed in the forward direction from 10.1° to 55.0° with respect to the incident neutron beamline. The telescopes at the angles θ_L = 10.1°, 20.0°, …, 50.0° (denoted as L1, L2, …, L5) were placed on the left side of the neutron beamline while the other five sets of telescopes at θ_L = 15.1°, 25.1°, …, 55.0° (denoted as R1, R2, …, R5) were placed on the right. The distances between the centers of the detectors and the center of the sample are listed in Table 1. The detectors at θ_L = 10.1° and θ_L = 15.1° were located further away from the sample than the others to avoid the irradiation by the neutron beam. The solid angle and the average detection angle for each telescope were calculated from Monte Carlo simulation [30] and are listed in Table 1. Note that the surface of each sample was perpendicular to the direction of the incident neutron beam throughout the experiment.

Figure 2.  (color online) (a) Picture of the interior of the LPDA vacuum chamber. (b) Schematic of the experimental setup.

The total beam duration for the measurement was approximately 210 h, split into seven rounds each consisting of ~18 h of CH₂ measurement, ~6 h of graphite measurement, and ~0.5 h of empty target measurement. Since the experiment was originally intended for the measurement of n-p scattering and the graphite measurement served as the background run [21], the measurement duration for the CH₂ sample was much longer than that for the carbon sample. Moreover, ²⁴¹Am sources were used to test the detectors and the DAQ system before, during, and after the experiment.

The experimental data were recorded using the Back-n DAQ system [31], which contained binary data of waveforms from all the telescopes, including two kinds of signals: a) those from the Si detectors that were only pre-processed by preamplifiers, and b) those from the CsI detectors that were multiplied by SiPMs and then amplified by the main amplifiers.

The ratios of the differential cross sections of the ¹²C(n, xd) reactions to those of the n-p elastic scattering in the corresponding neutron energy bin E_n and at the outgoing angle θ_L were measured first, and the principle for the measurement is described in the following equation:

where R(θ_L, E_n) is the ratio of the differential cross section of the ¹²C(n, xd) reactions to those of the n-p scattering in the corresponding neutron energy bin E_n and at the outgoing angle θ_L. The determination of neutron energy intervals will be explained in detail in section 4.5. C_d(θ_L, E_n) is the net count of deuteron events from the ¹²C(n, xd) reactions detected by the telescope at laboratory angle θ_L for neutron energy E_n. A correction of the influence from the ¹³C on C_d(θ_L, E_n) was carried out. However, it was rather insignificant because the abundance of ¹²C (98.93%) is 92.46 times that of ¹³C (1.07%) [12], and the cross section of the ¹²C(n, d)¹¹B reaction is at least 1.80 times bigger than that of the ¹³C(n, d)¹²B reaction according to the EAF-2010 library [32], which suggests that the deuterons from the ¹²C(n, d)¹¹B reaction are at least 166 times more than those from the ¹³C(n, d)¹²B. C_p(θ_L, E_n) is the net count of proton events from the n-p scattering. $c_{d}^{{\rm{dead\_time}}}({\theta _{\rm{L}}},{E_{n}})$ and $c_{p}^{{\rm{dead\_time}}}({\theta _{\rm{L}}},{E_{n}})$ are the respective correction factors due to the DAQ dead time for the carbon measurement and the n-p scattering measurement. They are determined by the portion of the saturated signals over the total number of the signals, which will be given in section IVA. ε_d(θ_L, E_n) is the detection efficiency for deuterons from the carbon sample, and ε_p(θ_L, E_n) is the detection efficiency for protons from the CH₂ sample, which will be discussed in section IVD. f_beam is the ratio of the number of protons bombarding the tungsten target (and thus producing neutrons) during the ¹²C(n, xd) reactions measurement to that during the n-p scattering measurement. ${N_{{}^{12}{\rm{C}}}}$ and N_H are the numbers of ¹²C atoms in the graphite foil sample and hydrogen atoms in the CH₂ sample, respectively. For relative measurement, the neutron fluence term and the solid angle term are eliminated.

The study of n-p scattering was for the measurement of the relative differential cross sections of the n-p scattering, details of which can be found in Ref. [21]. The results were relative differential cross sections of the n-p scattering, but only the net counts of protons from the n-p scattering, C_p(θ_L, E_n), the dead time correction, $c_{p}^{{\rm{dead\_time}}}({\theta _{\rm{L}}},{E_{n}})$, and the detection efficiency for protons, ε_d(θ_L, E_n), were used in the present work to calculate R(θ_L, E_n) in Eq. (1).

As it is not convenient to compare the present results, R(θ_L, E_n), to the previous ones obtained directly, the differential cross sections of the ¹²C(n, xd) reactions were thus calculated using the differential cross section data of the n-p scattering from the JENDL-4.0/HE-2015 library as the standard [22].

where $\dfrac{{{\rm d}\sigma ({\theta _{\rm{L}}},{E_{{n}}})}}{{{\rm d}\Omega }}\Big|_{{}^{{\rm{12}}}{\rm{C(}}n, xd{\rm{)}}}^{{\rm{lab}}}$ are the differential cross sections of the ¹²C(n, xd) reactions to be calculated in the laboratory system. $\dfrac{{{\rm d}\sigma ({\theta _{\rm{L}}},{E_{{n}}})}}{{{\rm d}\Omega }}{\Big |}_{n - p\;{\rm{scattering}}}^{{\rm{JENDL - lab}}}$ are the evaluated data of n-p scattering differential cross sections (in the laboratory system) extracted from the JENDL-4.0/HE-2015 [22] (= FENDL-3.1d [33]) library. The n-p data extracted from the JENDL-4.0/HE-2015 library were originally given as Legendre polynomial coefficients in the center-of-mass (CM) system. Using the coefficients, the differential cross sections of the n-p scattering in the CM system can be calculated, and they are transformed to the data in the laboratory system, which are $\dfrac{{{\rm d}\sigma ({\theta _{\rm{L}}},{E_{{n}}})}}{{{\rm d}\Omega }}{\Big|}_{n - p\;{\rm{scattering}}}^{{\rm{JENDL - lab}}}$.

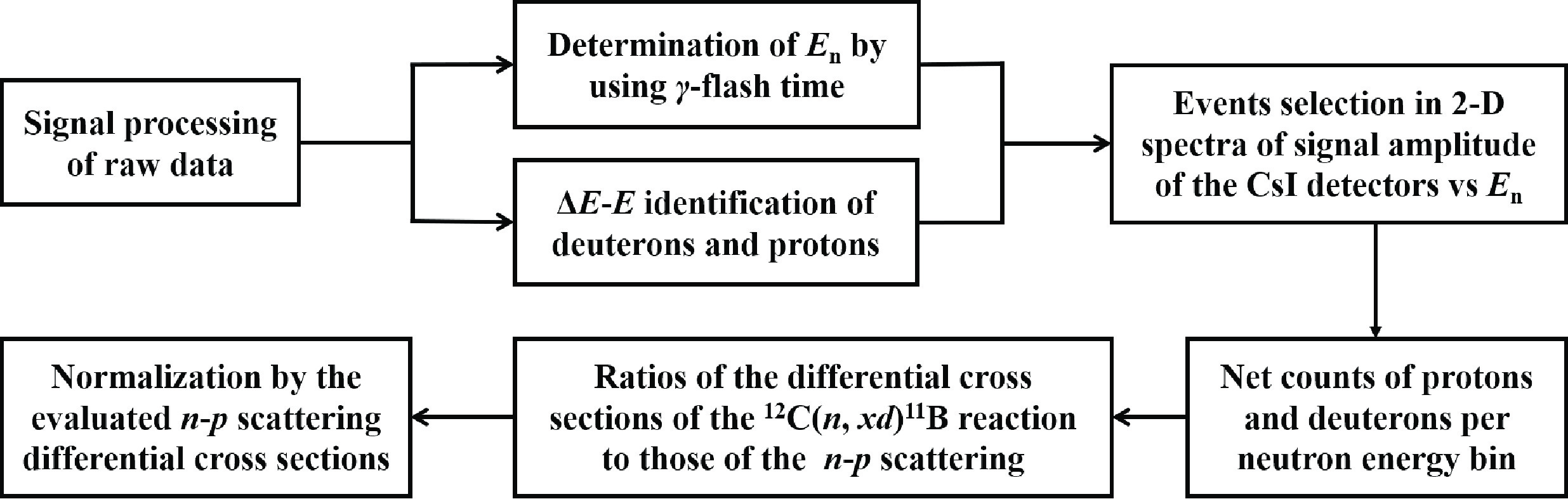
The method of the data analysis is similar to that for our n-p scattering measurement in 2019 [21]. A flowchart demonstrating the process of data analysis is shown in Fig. 3.

Figure 3.  Process of data analysis.

The recorded data of waveforms were processed and the amplitudes of the signals, the corresponding neutron TOF of each event, $c_{d}^{{\rm{dead\_time}}}({\theta _{\rm{L}}},{E_{n}})$, and $c_{p}^{{\rm{dead\_time}}}({\theta _{\rm{L}}},{E_{n}})$ in Eq. (1) were thus obtained. $c_{d}^{{\rm{dead\_time}}}({\theta _{\rm{L}}},{E_{n}})$ (<3‰) are the portions of the saturated signals over the total number of the signals for the carbon sample measurement and $c_{p}^{{\rm{dead\_time}}}({\theta _{\rm{L}}},{E_{n}})$ (<2%) is that for the CH₂ sample measurement.

The neutron energy for each event, E_n, is determined using the time-of-flight (TOF) method. γ-flash is produced in the spallation process and the γ-flash peak was used as the T₀ position. The TOF distributions of the γ-flash counts, i.e., the γ-flash event counts vs their TOFs for the Si-PIN detector and the CsI detector at θ_L = 15.1°, are presented in Fig. 4 (a) and (b), respectively, as examples. For each of the telescopes, the TOF distribution of the γ-flash events can be fitted by a double-peaked Gaussian distribution with a standard deviation of ~25 ns. This ~25 ns of standard deviation was caused by a) the width of the proton pulse that impinged on the tungsten target (main source), b) the time resolution of the detectors (several ns), and c) the time resolution of the DAQ system (<1 ns). The absolute TOF of the neutron for each event can be calculated:

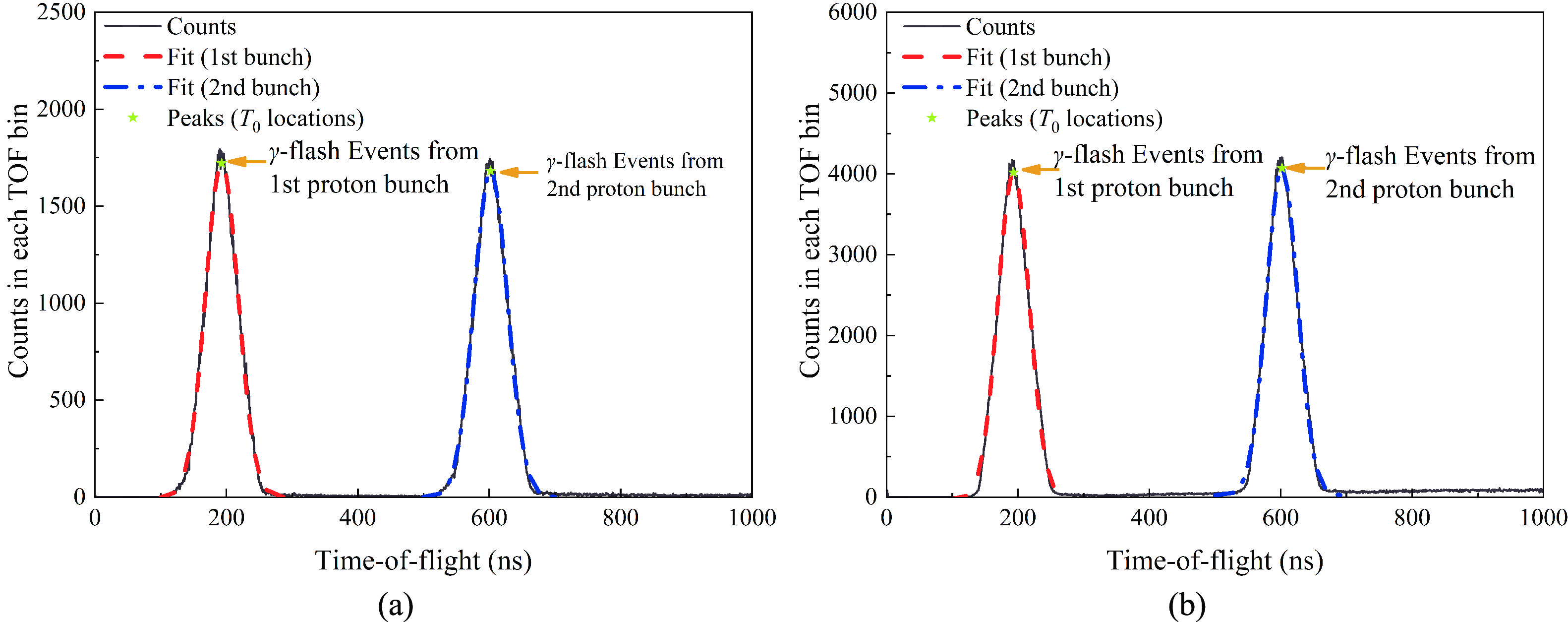
Figure 4.  (color online) TOF spectra of the γ-flash detected by the Si-PIN (a) and the CsI (b) detectors at θ_L = 15.1°. The semi-transparent red and blue lines are the fitting curves corresponding to the first and second proton bunches, respectively. The peak positions are shown as green stars.

where T_event is the starting time for the proton and deuteron events obtained from the Si-PIN signal because of its faster response time compared to the CsI signal. L is the length of the neuron flight path (57.99 m), and c is the velocity of light. The corresponding neutron energy for each event E_n can then be obtained from its TOF, where the relativistic effect is considered.

The ΔE-E distributions for all ten telescopes were obtained for the identification of the deuteron and proton events from the carbon sample and the CH₂ sample, respectively. The 2-D spectra of the ΔE-E signal amplitudes at θ_L = 15.1° obtained from the carbon sample and from the CH₂ sample are shown in Fig. 5 (a) and (b), respectively as examples. The same 2-D spectra obtained from the empty target were also analyzed and they were all blank, which proved that there was no charged particle background from the radiation on the aluminum sample holder by the neutron beam or the neutron beam itself.

Figure 5.  (color online) 2-D distributions of the ΔE-E signal amplitudes at θ_L = 15.1° from the carbon sample measurement (a) and the CH₂ sample measurement (b).

From Fig. 5 (a), it is clear that the number of proton events is much larger than the deuteron counts from the CH₂ sample. Therefore, the results from the CH₂ sample measurement are not suitable for obtaining the relative differential cross sections of the ¹²C(n, xd) reactions because of the strong interference from the protons, although there are plenty of carbon atoms in the CH₂ sample. Taking the counts at θ_L = 15.1° for instance, the number of proton events is 14.67 times that of deuteron events, which indicates that even a small number of protons misidentified by the boundaries (red lines and blue lines in Fig. 5 (b)) as deuterons will significantly affect the deuteron counts. This effect is particularly obvious for the area with high CsI detector signal amplitudes and low Si detector signal amplitudes, as can be seen in Fig. 5 (b). Consequently, a carbon sample (the graphite foil) was necessary for the present work for deuteron events measurement from the ¹²C(n, xd) reactions. As shown in Fig. 5, the interference from the proton events was much less for the carbon sample than those for the CH₂ sample and the interference is apparent only for CsI detector signal amplitudes greater than 2000 channels, where the corresponding neutron energy is larger than 60 MeV and out of the range of the present results.

With the knowledge of the neutron energy E_n for each deuteron or proton event and the signal amplitude of the CsI detectors, the 2-D distribution of the amplitude of the CsI detector output signal vs E_n (hereinafter referred to as the “CsI vs E_n spectrum”) is obtained. As the neutron source is double-bunched, the T₀ time for each of the two bunches is used to determine the corresponding neutron energy separately. The protons detected at θ_L = 15.1° from the CH₂ sample are plotted in Fig. 6 (a) and (b) as the 2-D distribution of the signal amplitude of the CsI detectors signals vs E_n as an example, which correspond to the first and second bunch, respectively.

Figure 6.  (color online) 2-D distribution of the signal amplitude of the CsI detector vs neutron energy E_n at θ_L = 15.1° for protons from the CH₂ sample. The neutron energy E_n corresponds to the first proton bunch in (a) and the second proton bunch in (b). The red line and the blue line are the upper and lower boundaries of the valid event area, respectively.

The identified deuterons using the ΔE-E method from the carbon sample are plotted as the 2-D distribution of the signal amplitude of the CsI detectors signals vs E_n. Fig. 7 (a) and (b) are the scatter plots of the detected deuteron distribution at θ_L = 15.1° corresponding to the first and second bunches, respectively.

Figure 7.  (color online) 2-D distribution of the signal amplitude of the CsI detector vs neutron energy E_n at θ_L = 15.1° for deuterons using the ΔE-E method from the carbon sample. The neutron energy, E_n, corresponds to the first proton bunch in (a) and the second proton bunch in (b). The red line and the blue line are the upper and lower boundaries of the valid event area.

As is shown in Fig. 7, the area of the deuteron events is not clear for both the first and second bunches, which creates considerable difficulty in selecting the boundaries of the deuteron events and in determining the detection efficiency for the deuterons. Consequently, the selection of the boundaries is a vital source of uncertainty of the result. The experimental results of the ¹²C(n, xd) reactions given in the present work include deuterons corresponding to different energy levels from multiple reactions such as ¹¹B (ground state, excited states of 2.12, 4.44, 5.02, 6.74, and 6.79 MeV) from the ¹²C(n, d) ¹¹B reaction. As a result, the boundaries of the deuterons were drawn noticeably wider than those of the protons (as shown in Fig. 6 and Fig. 7) to guarantee a high detection efficiency for deuterons, ε_d(θ_L, E_n), which was viewed as 100%. Although this approximation may introduce random events, it was reasonable and necessary. Furthermore, the boundaries were drawn narrower in the error analysis. The different counts between the narrow boundaries and the wide boundaries were counted as a source of uncertainty. The errors introduced by the random events and those by the approximation of viewing ε_d(θ_L, E_n) as 100% were thus included in the uncertainty of the boundaries. For the protons from the n-p scattering, it was found that ε_p(θ_L, E_n) > 98% by using the Monte Carlo simulation and comparing the experimental spectrum with that from the simulation. The method of the simulation is described in detail in Ref. [30]. The result ε_p(θ_L, E_n) > 98% was first reported in Ref. [21].

The neutron energy E_n for the Back-n white neutron source is split into six bins in the present work with a TOF interval of 50 ns according to the ~25 ns standard deviation of the Gaussian distribution of the γ-flash events, as described in section IVB. The E_n values in the present work, 25.2 MeV, 28.6 MeV, 32.7 MeV, 37.8 MeV, 44.2 MeV, and 52.5 MeV, are midpoints of their corresponding intervals. The differences between each midpoint and the boundaries of their intervals are thus viewed as the uncertainties of E_n. The Q-value of the ¹²C(n, d)¹¹B reaction is −13.732 MeV and the reaction threshold is 14.887 MeV [34]. The energies of the corresponding deuterons from the ¹²C(n, d)¹¹B reaction are 9.7 to 38.4 MeV for the ground state of ¹¹B according to binary reaction dynamics. Other channels, such as the ¹²C(n, n + d), etc., have Q-values lower than -13.732 MeV and therefore higher thresholds than 14.887 MeV. These higher thresholds, combined with the kinematics of the deuteron production reactions other than the ¹²C(n, d)¹¹B reaction, suggest that the emitting deuterons have lower energies than the deuterons from the ¹²C(n, d)¹¹B reaction for the ground state ¹¹B. The lower limit of the neutron energy for the present work is 25.2 MeV because of the negative Q-value of the ¹²C(n, d)¹¹B reaction [34] and the energy loss in the ΔE layer (Si-PIN detector) for particle identification. The upper limit of neutron energy is 52.5 MeV because the uncertainty of E_n increases and the number of recoil protons from the n-p scattering is not adequate due to the small cross sections of the n-p scattering and the weak neutron beam flux for E_n > 52.5 MeV [21]. The signal amplitudes caused by higher-energy protons are beyond the input voltage range of the DAQ system for the CsI detectors of the telescopes at θ_L = 10.1° and θ_L = 15.1°. As a result, there are no data for the ¹²C(n, xd) reactions θ_L = 10.1°, and the upper limit is 44.2 MeV at θ_L = 15.1° [21]. The numbers of protons from the CH₂ sample and those of the deuterons from the carbon sample within the boundaries drawn in section IVD, C_p(θ_L, E_n) and C_d (θ_L, E_n) in Eq. (1), are counted into the corresponding neutron energy bin according to the neutron energy. The numbers of protons from the CH₂ sample and those of the deuterons from the carbon sample within the boundaries drawn in section IVD, C_p(θ_L, E_n) and C_d (θ_L, E_n) in Eq. (1), are counted into the corresponding neutron energy bins according to the neutron energy.

C_d(θ_L, E_n) is the net count of the deuterons, which is the count for graphite foil measurement subtracted by the count for empty target measurement (normalized according to the beam of protons of the accelerator). However, there were no deuteron events in the empty target measurement run; therefore, the counts from the carbon sample measurement were the net counts. The net count of the protons from the CH₂ sample, C_p(θ_L, E_n), can be obtained after the background subtraction, which is described in detail in Ref. [21]. Because of the small cross sections and negative Q-values (-12.59 MeV for the ¹²C(n, p)¹²B reaction, for instance) [34] of the ¹²C(n, xp) reactions, the proton background from the ¹²C(n, xp) reactions is very weak compared to the protons from the n-p scattering. The spectrum of signal amplitude vs. net counts of protons from the CH₂ sample and that of deuterons from the carbon sample within the boundaries of the E_n = 44.2 MeV energy bin detected by the CsI detector at θ_L = 15.1° is presented in Fig. 8 as an example.

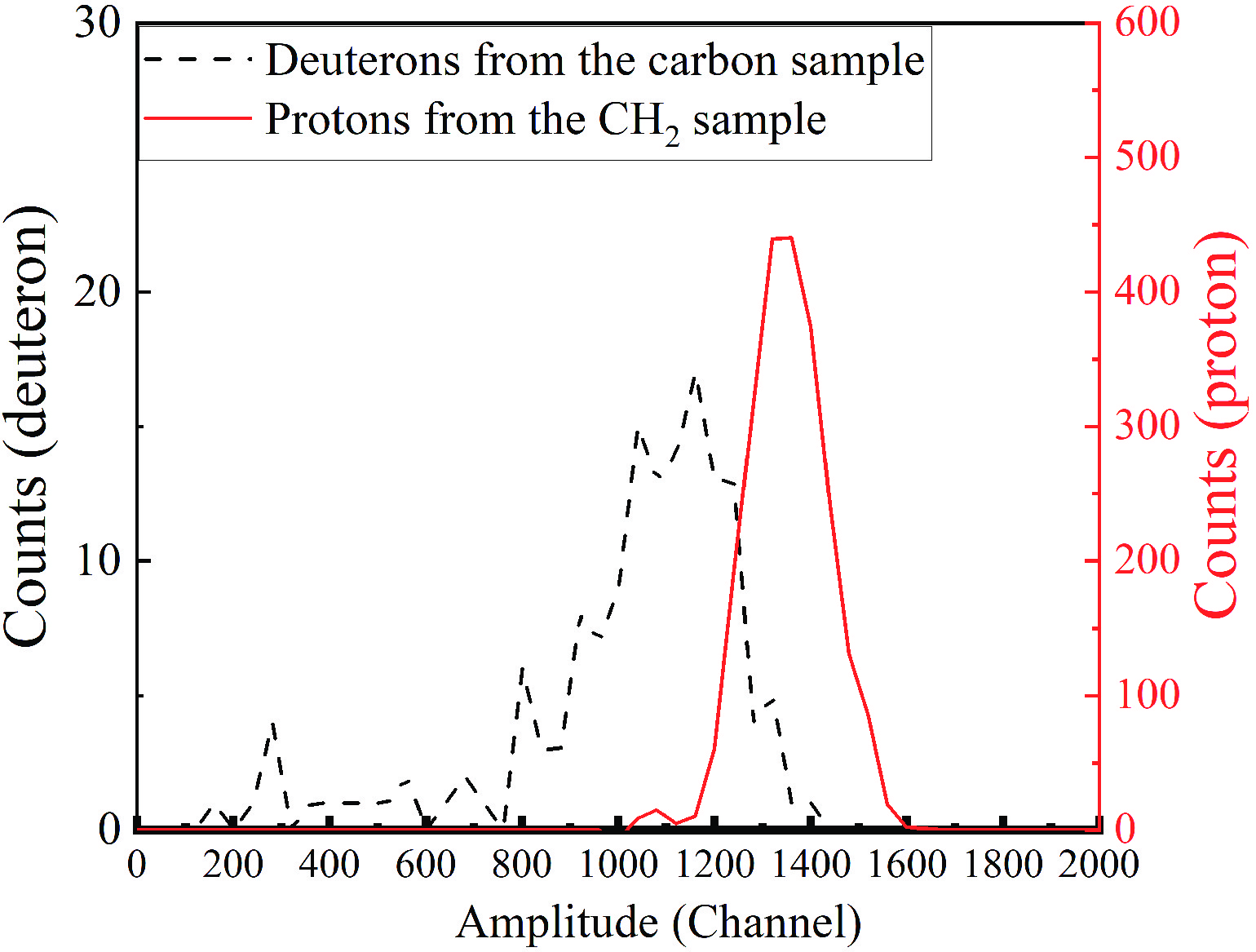
Figure 8.  (color online) Counts vs amplitude of the CsI detector signal at θ_L = 15.1° for the net deuterons from the carbon sample and the net protons from the CH₂ sample in the E_n = 44.2 MeV bin. Note that the scales for the deuteron counts (left black Y-axis) and the proton counts (right red Y-axis) are different. Counts in every 40 channels are added up.

The ratios of the differential cross sections of the ¹²C(n, xd) reactions to those of the n-p scattering, R(θ_L, E_n), can be calculated according to Eq. (1). As the present work is a relative measurement, ${N_{\rm{H}}}/{N_{{}^{12}{\rm{C}}}}$ can be calculated using the following equations:

where ρ_graphite = 1.80 g/cm³ and ${\rho _{{\rm{C}}{{\rm{H}}_2}}} = 0.92\;{\rm{ g/c}}{{\rm{m}}^3}$ are the densities of the carbon sample and the CH₂ sample, respectively, as provided by the manufacturer. d_graphite = 0.049 mm is the thickness of the carbon sample and ${d_{{\rm{C}}{{\rm{H}}_{\rm{2}}}}}$= 0.103 mm is the thickness of the CH₂ sample, both of which were measured using a micrometer. The areas of both samples were eliminated because the incident neutron beam spot was well covered by the samples. ${M_{{}^{{\rm{Nat}}}{\rm{C}}}} = 12.0\;{\rm{ g/mol}}$ is the molecular mass of natural carbon element, and ${M_{{\rm{C}}{{\rm{H}}_{\rm{2}}}}} = 14.0\;{\rm{ g/mol}}$ is the molecular mass of CH₂. ${a_{{}^{12}{\rm{C}}}} = 98.93$% is the abundance of ¹²C [12].

To compare the results from the present work with the previous ones from the literature [9, 13, 16-18] and the data from theoretical calculations [19], differential cross sections of the ¹²C(n, xd) reactions are obtained using the measured data R(θ_L, E_n) and the evaluated n-p scattering differential cross sections from the JENDL-4.0/HE-2015 library for normalization [22], as described in Eq. (2).

The measured differential cross sections in the laboratory system are presented in Fig. 9 and the tabulated data are given in Table 2. Brenner’s theoretical calculations using INCA code [16, 19] are also included in Fig. 9. The INCA code calculates the pre-equilibrium process using the intranuclear cascade model and describes the deexcitation of the compound nucleus based on the Fermi-breakup model [19, 35]. In addition, the data using Talys-1.9 code with default parameters [36] were calculated in the present work, and the results are presented in each plot. The data from the JENDL-4.0/HE-2015 library [22, 37] and the ENDF-B/VIII.0 library [38] are also included in Fig. 9, all of which were calculated based on GNASH code [39]. Furthermore, the results from previous measurements are also presented for comparison. There are only nine previous measurements [1, 2, 9, 13, 16-18, 40, 41] recorded in the EXFOR library, seven of which are in the several tens of MeV range [1, 2, 9, 13, 16-18] and five of which are within the neutron energy region of the present work. Those five measurement results are included in Fig. 9 for comparison. Some of the previous measurements were for the double differential cross sections of the deuteron production reactions on carbon, and they were energy-integrated to obtain angle-differential cross sections for comparison. Young’s original results were given for three energy levels of ¹¹B in the ¹²C(n, d)¹¹B reaction (ground state, level 1, and level 2 of ¹¹B) [18], and the differential cross sections of the three levels are added up in Fig. 9 (f) as “combined.”

Figure 9.  (color online) Differential cross sections of the ¹²C(n, xd) reactions measured at E_n = 25.2 MeV, 28.6 MeV, 32.7 MeV, 37.8 MeV, 44.2 MeV, and 52.5 MeV compared with results from previous measurements, the evaluated data, and the theoretical calculations. The previous experimental results were taken from the EXFOR library [14] and they include those of Subramanian et al. 1983 [16], Slypen et al. 2000, McNaughton et al. 1975 [9], Nauchi et al. 1999 [17], and Young et al. 1988 [18]. The evaluated data were taken from the JENDL-4.0/HE-2015 library [22, 37] and the ENDF-B/VIII.0 library [38], which were all calculated using GNASH code [39]. The theoretical calculations were carried out by Brenner et al. [19]. The calculations in the present work were based on the Talys-1.9 code [36]. The purple triangles in (f) are the combined data of Young (1988) at E_n = 60.0 MeV [18] for level 0, level 1, and level 2 of ¹¹B.

θL (°)	En = 25.2±1.5 MeV			En = 28.6±1.9 MeV			En = 32.7±2.3 MeV
	R(θL,En)	$\frac{ {{\rm d}\sigma ({\theta _{\rm{L} } },{E_{\rm{n} } })} }{ {{\rm d}\Omega } }|_{ {}^{ {\rm{12} } }{\rm{C(} }n, xd{\rm{)} } }^{ {\rm{lab} } }$		R(θL,En)	$\frac{ {{\rm d}\sigma ({\theta _{\rm{L} } },{E_{\rm{n} } })} }{ {{\rm d}\Omega } }|_{ {}^{ {\rm{12} } }{\rm{C(} }n, xd{\rm{)} } }^{ {\rm{lab} } }$		R(θL,En)	$\frac{ {{\rm d}\sigma ({\theta _{\rm{L} } },{E_{\rm{n} } })} }{ {{\rm d}\Omega } }|_{ {}^{ {\rm{12} } }{\rm{C(} }n, xd{\rm{)} } }^{ {\rm{lab} } }$
15.1	0.122±0.015	15.6±1.8		0.168±0.019	18.8±2.1		0.228±0.024	22.1±2.4
20.0	0.092±0.015	11.1±1.7		0.129±0.019	13.6±2.0		0.186±0.032	16.8±2.9
25.1	0.052±0.008	5.94±0.89		0.104±0.016	10.2±1.5		0.133±0.035	11.2±3.0
30.0	0.061±0.009	6.45±0.98		0.073±0.011	6.7±1.0		0.110±0.045	8.5±3.5
35.0	0.041±0.008	3.99±0.79		0.072±0.013	6.1±1.1		0.092±0.019	6.6±1.3
40.0	0.024±0.006	2.18±0.55		0.053±0.010	4.12±0.76		0.089±0.014	5.72±0.93
45.0	0.057±0.012	4.62±0.97		0.065±0.012	4.54±0.82		0.091±0.015	5.35±0.88
50.0	0.059±0.010	4.33±0.77		0.066±0.014	4.18±0.88		0.115±0.051	6.1±2.7
55.0	0.047±0.010	3.14±0.67		0.077±0.014	4.43±0.83		0.095±0.018	4.63±0.88
θL (°)	En = 37.8±2.8 MeV			En = 44.2±3.6 MeV			En = 52.5±4.7 MeV
	R(θL,En)	$\frac{ {{\rm d}\sigma ({\theta _{\rm{L} } },{E_{\rm{n} } })} }{ {{\rm d}\Omega } }|_{ {}^{ {\rm{12} } }{\rm{C(} }n, xd{\rm{)} } }^{ {\rm{lab} } }$		R(θL,En)	$\frac{ {{\rm d}\sigma ({\theta _{\rm{L} } },{E_{\rm{n} } })} }{ {{\rm d}\Omega } }|_{ {}^{ {\rm{12} } }{\rm{C(} }n, xd{\rm{)} } }^{ {\rm{lab} } }$		R(θL,En)	$\frac{ {{\rm d}\sigma ({\theta _{\rm{L} } },{E_{\rm{n} } })} }{ {{\rm d}\Omega } }|_{ {}^{ {\rm{12} } }{\rm{C(} }n, xd{\rm{)} } }^{ {\rm{lab} } }$
15.1	0.339±0.060	27.9±4.9		0.390±0.099	26.8±6.8		0.43±0.11	24.8±6.5
20.0	0.228±0.055	17.5±4.2		0.356±0.093	22.5±5.9		−	−
25.1	0.146±0.043	10.3±3.0		0.190±0.061	11.0±3.5		0.30±0.15	14.1±7.4
30.0	0.149±0.066	9.6±4.3		0.213±0.092	11.2±4.8		0.28±0.13	12.0±5.6
35.0	0.150±0.028	8.8±1.6		0.195±0.067	9.2±3.2		0.268±0.075	10.0±2.8
40.0	0.141±0.033	7.4±1.8		0.159±0.044	6.7±1.8		0.27±0.14	8.7±4.5
45.0	0.179±0.033	8.5±1.5		0.158±0.073	5.9±2.7		0.23±0.15	6.7±4.5
50.0	0.110±0.041	4.8±1.8		0.136±0.051	4.7±1.8		0.18±0.11	4.9±2.8
55.0	0.090±0.051	3.7±2.1		0.170±0.065	5.5±2.1		0.19±0.11	5.0±2.7

Table 2.  Measured results of the ¹²C(n, xd) reactions. R$ ({\theta _{\rm{L}}},{E_{n}}) $ are the ratios of the differential cross sections of the ¹²C(n, xd) reactions to those of the n-p scattering. $\frac{{{\rm d}\sigma ({\theta _{\rm{L}}},{E_{n}})}}{{{\rm d}\Omega }}|_{{}^{12}{\rm{C(}}n, xd{\rm{)}}}^{{\rm{lab}}}$ are the differential cross sections of the ¹²C(n, xd) reactions normalized by the JENDL-4.0/HE-2015 [22] data of the n-p scattering.

The sources of uncertainties and their magnitudes for the present work are listed in Table 3. Note that the uncertainties of the counts of the n-p scattering are included in the present work because the proton counts were used for the relative measurement [33].

The relatively short beam duration did not help obtain sufficiently good statistics and made it difficult to determine the valid area of the deuteron events in the 2-D spectrum scatter plots, including the ΔE-E spectra and the E_n-CsI spectra, as presented in Fig. 7 and Fig. 5 (a). This consequently brought about a rather large overall uncertainty. In addition, the uncertainty of E_n, i.e., the horizontal error shown in Table 3, is half the value of the width of the E_n interval, as explained in section IVE. The horizontal error increases as E_n increases.

As shown in Fig. 9, for all six energy regions, 25.3 ± 1.5, 28.6 ± 1.9, 32.7 ± 2.3, 37.8 ± 2.8, 44.2 ± 3.6, and 52.5 ± 4.7 MeV, the differential cross sections measured in the present work monotonically decline as θ_L increases. For E_n = 28.6 ± 1.9 MeV, the present results favor the data of Slypen et al. at E_n = 29.5 MeV [13], while the data by Subramanian et al. at E_n = 27.4 MeV [16] are noticeably larger with fluctuations in magnitude. From 32.7 MeV to 37.8 MeV, the present results agree well with the data of Slypen et al. [13] and Subramanian et al. [16]. The present results show a strong forward-peaked nature and fall drastically with the increase of θ_L, which is supported by all the results from the previous measurements. This effect is better characterized by the INCA code theoretical calculations by Brenner et al. [19], although the calculations predict results higher than the present ones and the previous measurement data at forward angles [13]. In addition, the decrease in the results as θ_L increases from the Talys-1.9 code, the JENDL-4.0/HE-2015 library, and the ENDF-B/VIII.0 is much milder.

The present data generally agree with the previous measurements except for the data of McNaughton at E_n = 56.0 MeV [9]. As shown in Fig. 9 (f), McNaughton’s data are larger than most of the other results, especially for θ_L $\leqslant $ 40°, while the present results are in better agreement with the data of Slypen et al. [13]. In contrast, Young’s data for the ¹²C(n, d)¹¹B reaction at 60.0 MeV [18] are significantly smaller than the rest, including the experimental data and all the theoretical calculations, which gives a scope of the contribution of the ¹²C(n, d)¹¹B reaction to the deuteron production from carbon. The data from the INCA code theoretical calculations at 40 MeV are larger than the present data and the previous measurements at the forward angles; however, they are in good accordance with the present results and the previous ones for θ_L $\geqslant $ 25°.

In general, despite the relatively large uncertainties, the present results agree with most of the previous experimental data relatively well considering the error bars in the whole energy region. The present results also agree with those of the theoretical calculations by Brenner et al. [19] at large emission angles and in higher neutron energy regions (θ_L > 30° and E_n > 40 MeV).

In the present paper, the angle-differential cross sections of the ¹²C(n, xd) reactions relative to those of the n-p scattering at six neutron energies from 25 MeV to 52 MeV are measured at the CSNS back-n white neutron source. Moreover, the present work includes theoretical calculations using the Talys-1.9 code with default parameters. Both the measured and the calculated results are compared with the data from the previous measurements, the evaluated data from the JENDL-4.0/HE-2015 library and the ENDF-B/VIII.0 library using GNASH code, and those from theoretical calculations based on INCA code. The measured results agree with most of the previous measurements in terms of the trend and magnitude (except for Young’s dedicated data for the ¹²C(n, d)¹¹B reaction) in the neutron energy region of the present work and are in accordance with the theoretical calculations by Brenner et al. at E_n = 50 and 60 MeV. The present work is the first white-neutron-source-based measurement of angular-differential cross sections of ¹²C(n, xd) reactions in the several tens of MeV range. The present results provide relative differential cross section data at a wider neutron energy range from 25 MeV to 52 MeV than the previous ones, all of which are obtained using mono-energetic neutrons.

The authors are grateful to the operating crew of the CSNS white neutron source. Senior Engineer Yongli Jin from the China Institute of Atomic Energy is appreciated for the support in data reading from the ENDF-B/VIII.0 library. The authors would also like to thank Prof. Zhenpeng Chen from Tsinghua University for valuable discussions.