-
In 2020, two charm-strange resonances,
$ X_0(2900) $ and$ X_1(2900) $ , which are presumably$ ud\bar{s}\bar{c} $ tetraquark candidates, were reported by the LHCb collaboration for$ B^+\rightarrow D^+D^-K^+ $ decays [1, 2]. In 2023, this collaboration announced two new resonant states,$ T^0_{c\bar{s}}(2900) $ and$ T^{++}_{c\bar{s}}(2900) $ , in a combined amplitude analysis of$B^0 \rightarrow \bar{D}^0 D^+_s \pi^-$ and$ B^+ \rightarrow D^- D^+_s \pi^+ $ decays [3, 4]. These observations indicated that the signals may be open-charm tetraquark candidates with minimal quark content$c\bar{s}q\bar{q} (q=u,\,d)$ . Without the ability to disentangle the masses and widths of these two resonances, the LHCb collaboration determined that they are$ 2.908\pm0.011\pm 0.020 $ GeV and$ 0.136\pm0.023\pm0.011 $ GeV in both cases; moreover, their quantum numbers$ I(J^P) $ were determined to be$ 1(0^+) $ .These observations have inspired numerous theoretical investigations. Generally, the
$ T_{c\bar{s}}(2900) $ states can be fully identified as a molecular structures in the$I(J^P)= 1(0^+)$ channel using phenomenological models such as those presented in Refs. [5−9]. However, interpretations of compact configurations [10, 11], threshold effects [12], and triangle anomalies [13] have also been proposed through various effective field theory approaches and quark models based on the color flux-tube. Additionally, the strong decay properties [14−16] and production mechanisms [17−20] of the exotic states have been investigated. In addition, more$ T_{c\bar{s}} $ resonances within an energy region of$ 2.1-3.0 $ GeV have been predicted theoretically [5, 7, 10].In addition to the mentioned
$ T_{cs} $ and$ T_{c\bar s} $ states, dozens of exotic hadrons, whose masses are generally near the threshold of two conventional heavy flavored hadrons, have been reported experimentally within the last 20 years. Owing to the rate at which they were being discovered, they have garnered considerable theoretical interest, and experiments through various approaches have been developed by theorists to reveal the nature of the unexpected exotic states, which are overall good candidates of multiquark systems. Particularly, many extensive reviews [21−38], which explain in detail particular theoretical methods and thus capture particular interpretations of exotic hadrons, can be found in the literature.Herein, we comprehensively investigate
$\bar{q}q\bar{s}Q \;\; (q=u, d;\,Q=c,\,b)$ tetraquark systems with spin-parities of$ J^P=0^+ $ ,$ 1^+ $ , and$ 2^+ $ in the isospin$ I=0 $ and$ 1 $ sectors. A variational formalism based on a highly efficient numerical approach called the Gaussian expansion method (GEM) [39] is used to solve the four-body Hamiltonian, which is based on a chiral quark model that has been used to describe reasonably well various tetra- and penta-quark systems [40−49]. Moreover, bound, resonant, and scattering states can be fully disentangled by solving the complex scaled Schrödinger equation formulated using the complex scaling method (CSM). Furthermore, the meson-meson, diquark-antidiquark, and K-type arrangements of quarks, as well as their couplings with all possible color wave functions, are considered.The remainder of this manuscript is organized as follows. Sec. II presents the theoretical framework and a brief description of the chiral quark model and
$ \bar{q}q\bar{s}Q $ tetraquark wave functions. Sec. III analyzes and discusses the calculated results. Finally, a summary is presented in Sec. IV. -
Phenomenological models continue to be the main tools used to study the nature of multiquark candidates observed experimentally. Hence,
$ \bar{q}q\bar{s}Q $ tetraquark systems are systematically investigated using a chiral quark model. Moreover, a highly accurate computing approach for the few-body system, the GEM, and an effective CSM are adopted to investigate the bound, resonant, and scattering states of the multiquark system. The theoretical formalism employed herein is described in detail in Ref. [26], and we shall focus on the most relevant features of the model and the numerical approach for$ \bar{q}q\bar{s}Q $ tetraquarks. -
The four-body problem is studied using a complex scaled Schrödinger equation:
$ \begin{array}{*{20}{l}} \left[ H(\theta)-E(\theta) \right] \Psi_{JM}(\theta)=0 \,, \end{array} $

(1) where the general form of the four-body Hamiltonian for a QCD-inspired chiral quark model is expressed as
$ H(\theta) = \sum\limits_{i=1}^{4}\left( m_i+\frac{\vec{p\,}^2_i}{2m_i}\right) - T_{\text{CM}} + \sum\limits_{j>i=1}^{4} V(\vec{r}_{ij} {\rm e}^{{\rm i}\theta}) \,, $

(2) where
$ m_{i} $ is the constituent quark mass,$ \vec{p}_i $ is the momentum of a quark,$ T_{\text{CM}} $ is the center-of-mass kinetic energy, and the last term is the two-body potential. By introducing an artificial parameter in the Hamiltonian, called the rotated angle θ, we find three types of complex eigenvalues, viz. bound, resonance, and scattering states can be simultaneously studied. In particular, the bound and resonance states are independent of θ, with the former always placed on the real-axis of the complex energy plane, and the latter located above the threshold line with a total decay width of$ \Gamma=-2\,\text{Im}(E) $ . Meanwhile, the energy dots corresponding to scattering states are unstable under rotations of θ and align along the threshold line, which also changes with θ.The dynamics of
$ \bar{q}q\bar{s}Q $ tetraquark systems is driven by two-body complex scaled potentials:$ \begin{array}{*{20}{l}} V(\vec{r}_{ij} {\rm e}^{{\rm i}\theta}) = V_{\chi}(\vec{r}_{ij} {\rm e}^{{\rm i}\theta}) + V_{\text{CON}}(\vec{r}_{ij} {\rm e}^{{\rm i}\theta}) + V_{\text{OGE}}(\vec{r}_{ij} {\rm e}^{{\rm i}\theta}) \,. \end{array} $

(3) In particular, dynamical chiral symmetry breaking, color-confinement, and perturbative one-gluon exchange interactions, which are considered the most relevant features of QCD at its low energy regime, are taken into account. Because the low-lying S-wave positive parity
$ \bar{q}q\bar{s}Q $ tetraquark states are investigated herein, only the central and spin-spin terms of the potential are considered.A consequence of the dynamical breaking of chiral symmetry is that Goldstone boson exchange interactions appear between constituent light quarks u, d, and s. Thus, the complex scaled chiral interaction can be expressed as
$ \begin{array}{*{20}{l}} V_{\chi}(\vec{r}_{ij} {\rm e}^{{\rm i}\theta}) = V_{\pi}(\vec{r}_{ij} {\rm e}^{{\rm i}\theta})+ V_{\sigma}(\vec{r}_{ij} {\rm e}^{{\rm i}\theta}) + V_{K}(\vec{r}_{ij} {\rm e}^{{\rm i}\theta}) + V_{\eta}(\vec{r}_{ij} {\rm e}^{{\rm i}\theta}) \,, \end{array} $

(4) where
$ \begin{aligned}[b] V_{\pi}\left( \vec{r}_{ij} {\rm e}^{{\rm i}\theta} \right) =\;& \frac{g_{ch}^{2}}{4\pi} \frac{m_{\pi}^2}{12m_{i}m_{j}} \frac{\Lambda_{\pi}^{2}}{\Lambda_{\pi}^{2}-m_{\pi} ^{2}}m_{\pi} \Bigg[ Y(m_{\pi}r_{ij} {\rm e}^{{\rm i}\theta}) \\ & - \frac{\Lambda_{\pi}^{3}}{m_{\pi}^{3}} Y(\Lambda_{\pi}r_{ij} {\rm e}^{{\rm i}\theta}) \bigg] (\vec{\sigma}_{i}\cdot\vec{\sigma}_{j})\sum\limits_{a=1}^{3}(\lambda_{i}^{a} \cdot\lambda_{j}^{a}) \,, \end{aligned} $

(5) $ \begin{aligned}[b] V_{\sigma}\left( \vec{r}_{ij} {\rm e}^{{\rm i}\theta} \right) =\;& - \frac{g_{ch}^{2}}{4\pi} \frac{\Lambda_{\sigma}^{2}}{\Lambda_{\sigma}^{2}-m_{\sigma}^{2}}m_{\sigma} \Bigg[Y(m_{\sigma}r_{ij} {\rm e}^{{\rm i}\theta}) \\ & - \frac{\Lambda_{\sigma}}{m_{\sigma}}Y(\Lambda_{\sigma}r_{ij} {\rm e}^{{\rm i}\theta}) \Bigg] \,, \end{aligned} $

(6) $ \begin{aligned}[b] V_{K}\left( \vec{r}_{ij} {\rm e}^{{\rm i}\theta} \right)=\;& \frac{g_{ch}^{2}}{4\pi} \frac{m_{K}^2}{12m_{i}m_{j}}\frac{\Lambda_{K}^{2}}{\Lambda_{K}^{2}-m_{K}^{2}}m_{ K} \Bigg[ Y(m_{K}r_{ij} {\rm e}^{{\rm i}\theta}) \\ & -\frac{\Lambda_{K}^{3}}{m_{K}^{3}}Y(\Lambda_{K}r_{ij} {\rm e}^{{\rm i}\theta}) \Bigg] (\vec{\sigma}_{i}\cdot\vec{\sigma}_{j}) \sum\limits_{a=4}^{7}(\lambda_{i}^{a} \cdot \lambda_{j}^{a}) \,, \end{aligned} $

(7) $ \begin{aligned} V_{\eta}\left( \vec{r}_{ij} {\rm e}^{{\rm i}\theta} \right) =\;& \frac{g_{ch}^{2}}{4\pi} \frac{m_{\eta}^2}{12m_{i}m_{j}} \frac{\Lambda_{\eta}^{2}}{\Lambda_{\eta}^{2}-m_{ \eta}^{2}}m_{\eta} \Bigg[ Y(m_{\eta}r_{ij} {\rm e}^{{\rm i}\theta}) \\ & -\frac{\Lambda_{\eta}^{3}}{m_{\eta}^{3} }Y(\Lambda_{\eta}r_{ij} {\rm e}^{{\rm i}\theta}) \Bigg] (\vec{\sigma}_{i}\cdot\vec{\sigma}_{j}) \Big[\cos\theta_{p} \left(\lambda_{i}^{8}\cdot\lambda_{j}^{8} \right) \\ & -\sin\theta_{p} \Big] \,, \end{aligned} $

(8) where
$Y(x)={\rm e}^{-x}/x$ is the Yukawa function. The physical η meson, instead of the octet one, is considered by introducing a model parameter of angle$ \theta_p $ .$ \lambda^{a} $ represents the$S U(3) $ flavor Gell-Mann matrices. Obtained from their experimental values,$ m_{\pi} $ ,$ m_{K} $ , and$ m_{\eta} $ are the masses of the$S U(3) $ Goldstone bosons. The value of$ m_{\sigma} $ is determined through the relation$ m_{\sigma}^{2}\simeq m_{\pi}^{2}+4m_{u,d}^{2} $ [50]. Finally, the chiral coupling constant,$ g_{ch} $ , is determined from the$ \pi NN $ coupling constant through$ \frac{g_{ch}^{2}}{4\pi}=\frac{9}{25}\frac{g_{\pi NN}^{2}}{4\pi} \frac{m_{u,d}^{2}}{m_{N}^2} \,, $

(9) which assumes that flavor
$S U(3) $ is an exact symmetry only broken by the different mass of the strange quark.Color confinement should be encoded in the non-Abelian character of QCD. On one hand, lattice-regularized QCD has demonstrated that multi-gluon exchanges produce an attractive linearly increasing potential proportional to the distance between infinite-heavy quarks [51]. On the other hand, the spontaneous creation of light-quark pairs from the QCD vacuum may result, at the same scale, in a breakup of the created color flux-tube [51]. We can phenomenologically describe the above two observations as
$ \begin{array}{*{20}{l}} V_{\text{CON}}(\vec{r}_{ij} {\rm e}^{{\rm i}\theta})=\left[-a_{c}(1-{\rm e}^{-\mu_{c}r_{ij} {\rm e}^{{\rm i}\theta}})+\Delta \right] (\lambda_{i}^{c}\cdot \lambda_{j}^{c}) \,, \end{array} $

(10) where
$ \lambda^c $ denotes the$S U(3) $ color Gell-Mann matrices, and$ a_{c} $ ,$ \mu_{c} $ , and Δ are model parameters. When the rotated angle θ is$ 0^\circ $ , the real-range potential in Eq. (10) is linear at short inter-quark distances with an effective confinement strength$ \sigma = -a_{c} \, \mu_{c} \, (\lambda^{c}_{i}\cdot \lambda^{c}_{j}) $ , whereas it becomes a constant at large distances,$ V_{\text{thr.}} = (\Delta-a_c) (\lambda^{c}_{i}\cdot \lambda^{c}_{j}) $ .Beyond the chiral symmetry breaking energy scale, we also expect the dynamics to be governed by perturbative effects of QCD. In particular, the one-gluon exchange potential, which includes the so-called Coulomb and color-magnetic interactions, is the leading order contribution:
$ \begin{aligned}[b] V_{\text{OGE}}(\vec{r}_{ij} {\rm e}^{{\rm i}\theta}) =\;& \frac{1}{4} \alpha_{s} (\lambda_{i}^{c}\cdot \lambda_{j}^{c}) \Bigg[\frac{1}{r_{ij} {\rm e}^{{\rm i}\theta}} \\ & - \frac{1}{6m_{i}m_{j}} (\vec{\sigma}_{i}\cdot\vec{\sigma}_{j}) \frac{{\rm e}^{-r_{ij} {\rm e}^{{\rm i}\theta} /r_{0}(\mu_{ij})}}{r_{ij} {\rm e}^{{\rm i}\theta} r_{0}^{2}(\mu_{ij})} \Bigg] \,, \end{aligned} $

(11) where
$ \vec{\sigma} $ denotes the Pauli matrices, and$ r_{0}(\mu_{ij})=\hat{r}_{0}/\mu_{ij} $ depends on the reduced mass of a$ q\bar{q} $ pair. Moreover, the regularized contact term is$ \delta(\vec{r}_{ij} {\rm e}^{{\rm i}\theta}) \sim \frac{1}{4\pi r_{0}^{2}(\mu_{ij})}\frac{{\rm e}^{-r_{ij} {\rm e}^{{\rm i}\theta} / r_{0}(\mu_{ij})}}{r_{ij} {\rm e}^{{\rm i}\theta} } \,. $

(12) An effective scale-dependent strong coupling constant,
$ \alpha_s(\mu_{ij}) $ , provides a consistent description of mesons and baryons from light to heavy quark sectors. The frozen coupling constant is expressed as (for instance, Ref. [52])$ \alpha_{s}(\mu_{ij})=\frac{\alpha_{0}}{\ln\left(\dfrac{\mu_{ij}^{2}+\mu_{0}^{2}}{\Lambda_{0}^{2}} \right)} \,, $

(13) where
$ \alpha_{0} $ ,$ \mu_{0} $ , and$ \Lambda_{0} $ are model parameters.All the discussed model parameters are summarized in Table 1. They have been fixed along the last two decades by thorough studies of hadron phenomenology such as meson [53−55] and baryon [56−58] spectra, hadron decays and reactions [59−61], the coupling between conventional hadrons and hadron-hadron thresholds [62−64], and molecular hadron-hadron formation [65−67]. Furthermore, for a later discussion, Table 2 lists theoretical and experimental (if available) masses of
$ 1S $ and$ 2S $ states of$ q\bar{q} $ and$ \bar{q}Q \;\; (q=u,\,d,\,s;\, Q=c,\,b) $ mesons.Quark masses $ m_q\,(q=u,\,d) $ /MeV

313 $ m_s $ /MeV

555 $ m_c $ /MeV

1752 $ m_b $ /MeV

5100 Goldstone bosons $ \Lambda_\pi=\Lambda_\sigma $ /fm

$ ^{-1} $ 

4.20 $ \Lambda_\eta=\Lambda_K $ fm

$ ^{-1} $ 

5.20 $ g^2_{ch}/(4\pi) $ 

0.54 $ \theta_P(^\circ) $ 

-15 Confinement $ a_c $ /MeV

430 $ \mu_c $ /fm

$^{-1}$ 

0.70 Δ /MeV 181.10 OGE $ \alpha_0 $ 

2.118 $ \Lambda_0 $ /fm

$ ^{-1} $ 

0.113 $ \mu_0 $ /MeV

36.976 $ \hat{r}_0 $ /MeV fm

28.17 Table 1. Chiral quark model parameters.
Meson $ nL $ 

$ M_{\text{The.}} $ 

$ M_{\text{Exp.}} $ 

Meson $ nL $ 

$ M_{\text{The.}} $ 

$ M_{\text{Exp.}} $ 

π $ 1S $ 

$ 149 $ 

$ 140 $ 

η $ 1S $ 

$ 689 $ 

$ 548 $ 

$ 2S $ 

$ 1291 $ 

$ 1300 $ 

$ 2S $ 

$ 1443 $ 

$ 1295 $ 

ρ $ 1S $ 

$ 772 $ 

$ 770 $ 

ω $ 1S $ 

$ 696 $ 

$ 782 $ 

$ 2S $ 

$ 1479 $ 

$ 1450 $ 

$ 2S $ 

$ 1449 $ 

$ 1420 $ 

K $ 1S $ 

$ 481 $ 

$ 494 $ 

$ K^* $ 

$ 1S $ 

$ 907 $ 

$ 892 $ 

$ 2S $ 

$ 1468 $ 

$ 1460 $ 

$ 2S $ 

$ 1621 $ 

$ 1630 $ 

D $ 1S $ 

$ 1897 $ 

$ 1870 $ 

$ D^* $ 

$ 1S $ 

$ 2017 $ 

$ 2007 $ 

$ 2S $ 

$ 2648 $ 

– $ 2S $ 

$ 2704 $ 

– $ D_s $ 

$ 1S $ 

$ 1989 $ 

$ 1968 $ 

$ D^*_s $ 

$ 1S $ 

$ 2115 $ 

$ 2112 $ 

$ 2S $ 

$ 2705 $ 

– $ 2S $ 

$ 2769 $ 

– B $ 1S $ 

$ 5278 $ 

$ 5280 $ 

$ B^* $ 

$ 1S $ 

$ 5319 $ 

$ 5325 $ 

$ 2S $ 

$ 5984 $ 

– $ 2S $ 

$ 6005 $ 

– $ B_s $ 

$ 1S $ 

$ 5355 $ 

$ 5367 $ 

$ B^*_s $ 

$ 1S $ 

$ 5400 $ 

$ 5415 $ 

$ 2S $ 

$ 6017 $ 

– $ 2S $ 

$ 6042 $ 

– Table 2. Theoretical and experimental (if available) masses of
$ 1S $ and$ 2S $ states of$ q\bar{q} $ and$ \bar{q}Q\,(q=u, d, s;\, Q=c,\,b) $ mesons (unit: MeV). -
The S-wave
$ \bar{q}q\bar{s}Q \;\; (q=u,\,d;\,Q=c,\,b) $ tetraquark configurations are shown in Fig. 1. Figs. 1(a) and (b) show the meson-meson structures, Fig. 1(c) shows the diquark-antidiquark arrangement, and (d) to (f) show the K-type configurations. For solving a manageable$ 4 $ -body problem for fully-coupled channels, the K-type configurations are occasionally restricted, as in our previous investigations [41, 42]. Furthermore, only one configuration would be sufficient for the calculation if all radial and orbital excited states are considered; however, this is inefficient. Therefore, a more economic method to use is the combination of the different mentioned structures.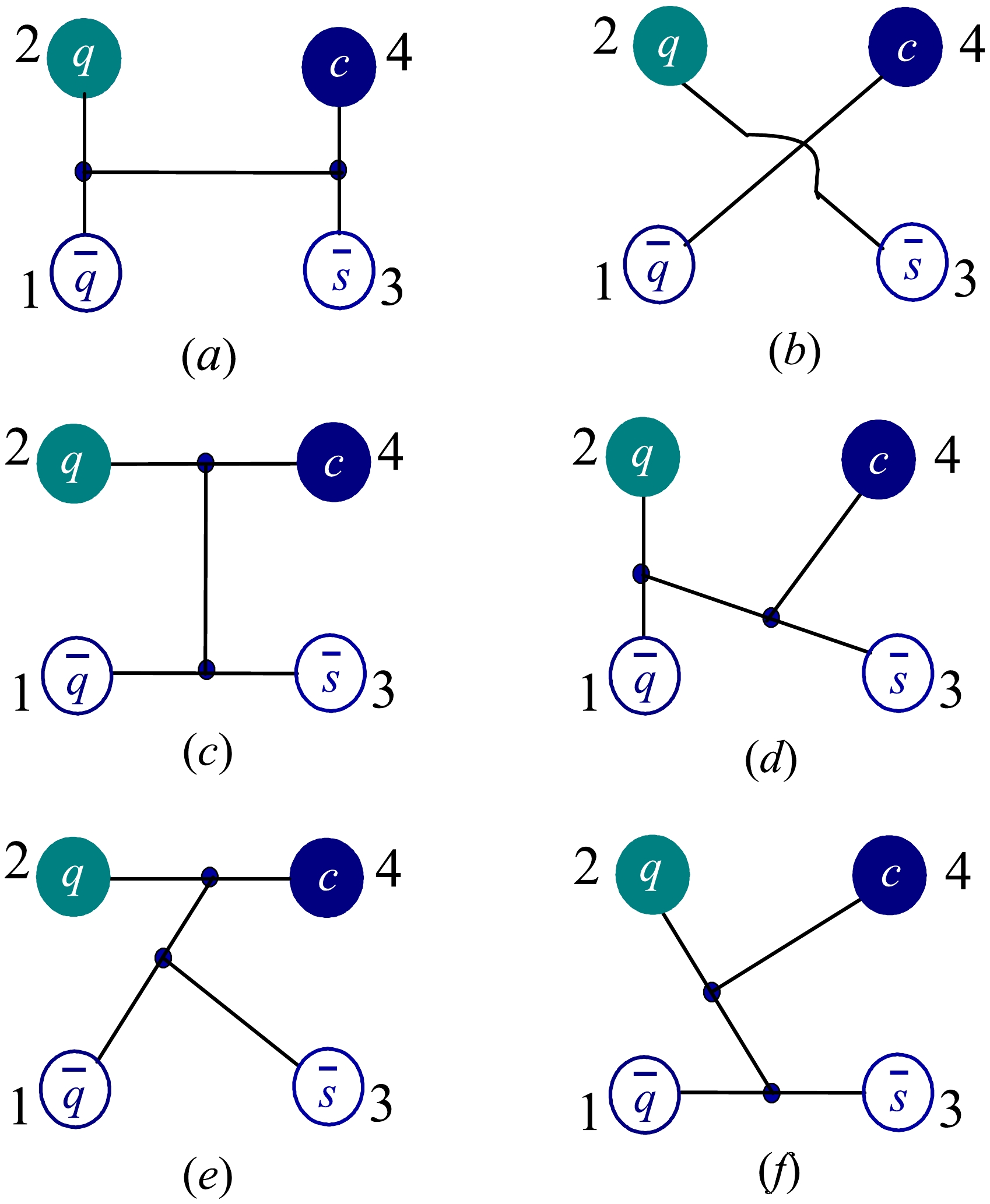
Figure 1. (color online) Six types of configurations are considered for the
$ \bar{q}q\bar{s}Q \;\; (q=u,\,d;\,Q=c,\,b) $ tetraquarks. Panels$(\rm a)$ and$(\rm b)$ are meson-meson structures, panel$(\rm c)$ is diquark-antidiquark arrangement, and the K-type configurations are shown in panels$(\rm d)$ to$(\rm f)$ .At the quark level, the total wave function of a tetraquark system is the internal product of color, spin, flavor, and space wave functions. First, regarding the color degree-of-freedom, the colorless wave function of a
$ 4 $ -quark system in meson-meson configuration can be obtained using either two coupled color-singlet clusters,$ 1\otimes 1 $ :$ \chi^c_1 = \frac{1}{3}(\bar{r}r+\bar{g}g+\bar{b}b)\times (\bar{r}r+\bar{g}g+\bar{b}b) \,, $

(14) or two coupled color-octet clusters,
$ 8\otimes 8 $ :$ \begin{aligned}[b] \chi^c_2 =\;& \frac{\sqrt{2}}{12}(3\bar{b}r\bar{r}b+3\bar{g}r\bar{r}g+3\bar{b}g\bar{g}b+3\bar{g}b\bar{b}g+3\bar{r}g\bar{g}r \\ &+3\bar{r}b\bar{b}r+2\bar{r}r\bar{r}r+2\bar{g}g\bar{g}g+2\bar{b}b\bar{b}b-\bar{r}r\bar{g}g \\ &-\bar{g}g\bar{r}r-\bar{b}b\bar{g}g-\bar{b}b\bar{r}r-\bar{g}g\bar{b}b-\bar{r}r\bar{b}b) \,. \end{aligned} $

(15) The first color state is the so-called color-singlet channel, and the second one is the named hidden-color channel.
The color wave functions associated to the diquark-antidiquark structure are the coupled color triplet-antitriplet clusters,
$ 3\otimes \bar{3} $ :$ \begin{aligned}[b] \chi^c_3 =\;& \frac{\sqrt{3}}{6}(\bar{r}r\bar{g}g-\bar{g}r\bar{r}g+\bar{g}g\bar{r}r-\bar{r}g\bar{g}r+\bar{r}r\bar{b}b \\ &-\bar{b}r\bar{r}b+\bar{b}b\bar{r}r-\bar{r}b\bar{b}r+\bar{g}g\bar{b}b-\bar{b}g\bar{g}b \\ &+\bar{b}b\bar{g}g-\bar{g}b\bar{b}g) \,, \end{aligned} $

(16) and the coupled color sextet-antisextet clusters,
$ 6\otimes \bar{6} $ :$ \begin{aligned}[b] \chi^c_4 =\;& \frac{\sqrt{6}}{12}(2\bar{r}r\bar{r}r+2\bar{g}g\bar{g}g+2\bar{b}b\bar{b}b+\bar{r}r\bar{g}g+\bar{g}r\bar{r}g \\ &+\bar{g}g\bar{r}r+\bar{r}g\bar{g}r+\bar{r}r\bar{b}b+\bar{b}r\bar{r}b+\bar{b}b\bar{r}r \\ &+\bar{r}b\bar{b}r+\bar{g}g\bar{b}b+\bar{b}g\bar{g}b+\bar{b}b\bar{g}g+\bar{g}b\bar{b}g) \,. \end{aligned} $

(17) Meanwhile, the possible color-singlet wave functions of three K-type structures are given by
$ \chi^c_5 = \chi^c_2 \,, $

(18) $ \chi^c_6 = \chi^c_1 \,, $

(19) $ \begin{aligned}[b] \chi^c_7 =\;& \frac{1}{2\sqrt{6}}(\bar{r}b\bar{b}r+\bar{r}r\bar{b}b+\bar{g}b\bar{b}g+\bar{g}g\bar{b}b+\bar{r}g\bar{g}r+\bar{r}r\bar{g}g \\ &+\bar{b}b\bar{g}g+\bar{b}g\bar{g}b+\bar{g}g\bar{r}r+\bar{g}r\bar{r}g+\bar{b}b\bar{r}r+\bar{b}r\bar{r}b) \\ &+\frac{1}{\sqrt{6}}(\bar{r}r\bar{r}r+\bar{g}g\bar{g}g+\bar{b}b\bar{b}b) \,, \end{aligned} $

(20) $ \begin{aligned}[b] \chi^c_8 =\;& \frac{1}{2\sqrt{3}}(\bar{r}b\bar{b}r-\bar{r}r\bar{b}b+\bar{g}b\bar{b}g-\bar{g}g\bar{b}b+\bar{r}g\bar{g}r-\bar{r}r\bar{g}g \\ &-\bar{b}b\bar{g}g+\bar{b}g\bar{g}b-\bar{g}g\bar{r}r+\bar{g}r\bar{r}g-\bar{b}b\bar{r}r+\bar{b}r\bar{r}b) \,, \end{aligned} $

(21) $ \chi^c_9 = \chi^c_7 \,, $

(22) $ \chi^c_{10} = -\chi^c_8 \,. $

(23) For the flavor degree-of-freedom, both iso-scalar (
$ I=0 $ ) and iso-vector ($ I=1 $ ) channels of$ \bar{q}q\bar{s}Q \; (q=u,\,d; Q=c,\,b) $ tetraquarks should be considered. In particular, for meson-meson and part of K-type (Fig. 1(d) and (e)) configurations, the flavor wave functions, which are denoted as$ \chi^{f_1}_{I, M_I} $ , are$ \chi_{0,0}^{f_1} = -\frac{1}{\sqrt{2}}(\bar{u}u\bar{s}Q+\bar{d}d\bar{s}Q) \,, $

(24) $ \chi_{1,0}^{f_1} = \frac{1}{\sqrt{2}}(-\bar{u}u\bar{s}Q+\bar{d}d\bar{s}Q) \,. $

(25) Additionally, using similar notations
$ \chi^{f_2}_{I, M_I} $ and$ \chi^{f_3}_{I, M_I} $ , where the superscripts$ 2 $ and$ 3 $ denote symmetry and antisymmetry properties between the$ \bar{q}\bar{s} $ -pair, respectively, the wave functions of diquark-antidiquark and K-type (Fig. 1(f)) structures are given by$ \chi_{0,0}^{f_2} = -\frac{1}{2}(\bar{u}u\bar{s}Q+\bar{s}u\bar{u}Q+\bar{d}d\bar{s}Q+\bar{s}d\bar{d}Q) \,, $

(26) $ \chi_{0,0}^{f_3} = +\frac{1}{2}(-\bar{u}u\bar{s}Q+\bar{s}u\bar{u}Q-\bar{d}d\bar{s}Q+\bar{s}d\bar{d}Q) \,, $

(27) $ \chi_{1,0}^{f_2} = +\frac{1}{2}(-\bar{u}u\bar{s}Q-\bar{s}u\bar{u}Q+\bar{d}d\bar{s}Q+\bar{s}d\bar{d}Q) \,, $

(28) $ \chi_{1,0}^{f_3} = +\frac{1}{2}(-\bar{u}u\bar{s}Q+\bar{s}u\bar{u}Q+\bar{d}d\bar{s}Q-\bar{s}d\bar{d}Q) \,. $

(29) Herein, the third component of the isospin,
$ M_I $ , is fixed to zero for simplicity, and this is because there is no flavor-dependent interaction in the Hamiltonian that can distinguish the third component of the isospin I.Now, let us consider the S-wave ground states with spin (S) ranging from
$ 0 $ to$ 2 $ . Therefore, the spin wave functions,$ \chi^{\sigma_i}_{S, M_S} $ , are given by ($ M_S $ can be set to be equal to S without losing generality):$ \chi_{0,0}^{\sigma_{u_1}}(4) = \chi^\sigma_{00}\chi^\sigma_{00} \,, $

(30) $ \chi_{0,0}^{\sigma_{u_2}}(4) = \frac{1}{\sqrt{3}}(\chi^\sigma_{11}\chi^\sigma_{1,-1}-\chi^\sigma_{10}\chi^\sigma_{10}+\chi^\sigma_{1,-1}\chi^\sigma_{11}) \,, $

(31) $ \begin{aligned}[b] \chi_{0,0}^{\sigma_{u_3}}(4) =\;& \frac{1}{\sqrt{2}}\big((\sqrt{\frac{2}{3}}\chi^\sigma_{11}\chi^\sigma_{\frac{1}{2}, -\frac{1}{2}}-\sqrt{\frac{1}{3}}\chi^\sigma_{10}\chi^\sigma_{\frac{1}{2}, \frac{1}{2}})\chi^\sigma_{\frac{1}{2}, -\frac{1}{2}} \\ &-(\sqrt{\frac{1}{3}}\chi^\sigma_{10}\chi^\sigma_{\frac{1}{2}, -\frac{1}{2}}-\sqrt{\frac{2}{3}}\chi^\sigma_{1, -1}\chi^\sigma_{\frac{1}{2}, \frac{1}{2}})\chi^\sigma_{\frac{1}{2}, \frac{1}{2}}\big) \,, \end{aligned} $

(32) $ \chi_{0,0}^{\sigma_{u_4}}(4) = \frac{1}{\sqrt{2}}(\chi^\sigma_{00}\chi^\sigma_{\frac{1}{2}, \frac{1}{2}}\chi^\sigma_{\frac{1}{2}, -\frac{1}{2}}-\chi^\sigma_{00}\chi^\sigma_{\frac{1}{2}, -\frac{1}{2}}\chi^\sigma_{\frac{1}{2}, \frac{1}{2}}) \,, $

(33) for
$ (S,M_S)=(0,0) $ , by$ \chi_{1,1}^{\sigma_{w_1}}(4) = \chi^\sigma_{00}\chi^\sigma_{11} \,, $

(34) $ \chi_{1,1}^{\sigma_{w_2}}(4) = \chi^\sigma_{11}\chi^\sigma_{00} \,, $

(35) $ \chi_{1,1}^{\sigma_{w_3}}(4) = \frac{1}{\sqrt{2}} (\chi^\sigma_{11} \chi^\sigma_{10}-\chi^\sigma_{10} \chi^\sigma_{11}) \,, $

(36) $ \begin{aligned}[b] \chi_{1,1}^{\sigma_{w_4}}(4) =\;& \sqrt{\frac{3}{4}}\chi^\sigma_{11}\chi^\sigma_{\frac{1}{2}, \frac{1}{2}}\chi^\sigma_{\frac{1}{2}, -\frac{1}{2}}-\sqrt{\frac{1}{12}}\chi^\sigma_{11}\chi^\sigma_{\frac{1}{2}, -\frac{1}{2}}\chi^\sigma_{\frac{1}{2}, \frac{1}{2}} \\ &-\sqrt{\frac{1}{6}}\chi^\sigma_{10}\chi^\sigma_{\frac{1}{2}, \frac{1}{2}}\chi^\sigma_{\frac{1}{2}, \frac{1}{2}} \,, \end{aligned} $

(37) $ \chi_{1,1}^{\sigma_{w_5}}(4) = (\sqrt{\frac{2}{3}}\chi^\sigma_{11}\chi^\sigma_{\frac{1}{2}, -\frac{1}{2}}-\sqrt{\frac{1}{3}}\chi^\sigma_{10}\chi^\sigma_{\frac{1}{2}, \frac{1}{2}})\chi^\sigma_{\frac{1}{2}, \frac{1}{2}} \,, $

(38) $ \chi_{1,1}^{\sigma_{w_6}}(4) = \chi^\sigma_{00}\chi^\sigma_{\frac{1}{2}, \frac{1}{2}}\chi^\sigma_{\frac{1}{2}, \frac{1}{2}} \,, $

(39) for
$ (S,M_S)=(1,1) $ , and by$ \begin{array}{*{20}{l}} \chi_{2,2}^{\sigma_{1}}(4) &= \chi^\sigma_{11}\chi^\sigma_{11} \,, \end{array} $

(40) for
$(S,\;M_S)=(2,\;2)$ . The superscripts$u_1,\;u_2,\ldots,u_4$ and$w_1, \; w_2,\ldots,w_6$ determine the spin wave function for each configuration of the$ \bar{q}q\bar{s}Q $ tetraquark system, their values are listed in Table 3. Furthermore, the expressions above are obtained by considering the coupling between two sub-clusters whose spin wave functions are given by trivial SU(2) algebra, and the necessary basis is expressed asDi-meson Diquark-antidiquark $ K_1 $ 

$ K_2 $ 

$ K_3 $ 

$ u_1 $ 

1 3 $ u_2 $ 

2 4 $ u_3 $ 

5 7 9 $ u_4 $ 

6 8 10 $ w_1 $ 

1 4 $ w_2 $ 

2 5 $ w_3 $ 

3 6 $ w_4 $ 

7 10 13 $ w_5 $ 

8 11 14 $ w_6 $ 

9 12 15 Table 3. Values of the superscripts
$ u_1,\ldots,u_4 $ and$ w_1,\ldots,w_6 $ that specify the spin wave function for each configuration of$ \bar{q}q\bar{s}Q \;\; (q=u,\,d;\, Q=c,\,b) $ tetraquark systems.$ \chi^\sigma_{00} = \frac{1}{\sqrt{2}}(\chi^\sigma_{\frac{1}{2}, \frac{1}{2}} \chi^\sigma_{\frac{1}{2}, -\frac{1}{2}}-\chi^\sigma_{\frac{1}{2}, -\frac{1}{2}} \chi^\sigma_{\frac{1}{2}, \frac{1}{2}}) \,, $

(41) $ \chi^\sigma_{11} = \chi^\sigma_{\frac{1}{2}, \frac{1}{2}} \chi^\sigma_{\frac{1}{2}, \frac{1}{2}} \,, $

(42) $ \chi^\sigma_{1,-1} = \chi^\sigma_{\frac{1}{2}, -\frac{1}{2}} \chi^\sigma_{\frac{1}{2}, -\frac{1}{2}} \,, $

(43) $ \chi^\sigma_{10} = \frac{1}{\sqrt{2}}(\chi^\sigma_{\frac{1}{2}, \frac{1}{2}} \chi^\sigma_{\frac{1}{2}, -\frac{1}{2}}+\chi^\sigma_{\frac{1}{2}, -\frac{1}{2}} \chi^\sigma_{\frac{1}{2}, \frac{1}{2}}) \,. $

(44) The Rayleigh-Ritz variational principle, which is one of the most used tools to solve eigenvalue problems, is employed to solve the Schrödinger-like four-body system equation. Generally, within a complex-scaling theoretical framework, the spatial wave function is expressed as follows:
$ \begin{array}{*{20}{l}} \psi_{LM_L}= \left[ \left[ \phi_{n_1l_1}(\vec{\rho}{\rm e}^{{\rm i}\theta}\,) \phi_{n_2l_2}(\vec{\lambda}{\rm e}^{{\rm i}\theta}\,)\right]_{l} \phi_{n_3l_3}(\vec{R}{\rm e}^{{\rm i}\theta}\,) \right]_{L M_L} \,, \end{array} $

(45) where the internal Jacobi coordinates are defined as
$ \vec{\rho} = \vec{x}_1-\vec{x}_{2(4)} \,, $

(46) $ \vec{\lambda} = \vec{x}_3 - \vec{x}_{4(2)} \,, $

(47) $ \vec{R} = \frac{m_1 \vec{x}_1 + m_{2(4)} \vec{x}_{2(4)}}{m_1+m_{2(4)}}- \frac{m_3 \vec{x}_3 + m_{4(2)} \vec{x}_{4(2)}}{m_3+m_{4(2)}} \,, $

(48) for the meson-meson configurations of Figs. 1
$(\rm a)$ and$(\rm b)$ ; and as$ \vec{\rho} = \vec{x}_1-\vec{x}_3 \,, $

(49) $ \vec{\lambda} = \vec{x}_2 - \vec{x}_4 \,, $

(50) $ \vec{R} = \frac{m_1 \vec{x}_1 + m_3 \vec{x}_3}{m_1+m_3}- \frac{m_2 \vec{x}_2 + m_4 \vec{x}_4}{m_2+m_4} \,, $

(51) for the diquark-antidiquark structure of Fig. 1
$(\rm c)$ . The remaining K-type configurations shown in Fig. 1$(\rm d)$ to$(\rm f)$ are ($ i, j, k, l $ take values according to panels$(\rm d)$ to$(\rm f)$ of Fig. 1):$ \vec{\rho} = \vec{x}_i-\vec{x}_j \,, $

(52) $ \vec{\lambda} = \vec{x}_k- \frac{m_i \vec{x}_i + m_j \vec{x}_j}{m_i+m_j} \,, $

(53) $ \vec{R} = \vec{x}_l- \frac{m_i \vec{x}_i + m_j \vec{x}_j+m_k \vec{x}_k}{m_i+m_j+m_k} \,. $

(54) It is obvious now that the center-of-mass kinetic term
$ T_\text{CM} $ can be completely eliminated for a non-relativistic system defined in any of the above sets of relative motion coordinates.The basis expansion of the genuine wave function of Eq. (45) is a crucial aspect in the Rayleigh-Ritz variational method. By employing the GEM [39], which has proven to be efficient in solving the bound-state problem of multi-body systems, we expand all the spatial wave functions corresponding to the four relative motions with Gaussian basis functions, whose sizes are obtained using geometric progression. Hence, the form of orbital wave functions, ϕ, in Eq. (45) for a S-wave tetraquark system is simply expressed as
$ \begin{array}{*{20}{l}} & \phi_{nlm}(\vec{r}{\rm e}^{{\rm i}\theta}\,) = \sqrt{1/4\pi} \, N_{nl} \, (r{\rm e}^{{\rm i}\theta})^{l} \, {\rm e}^{-\nu_{n} (r{\rm e}^{i\theta})^2} \,. \end{array} $

(55) Finally, the complete wave function, which fulfills the Pauli principle, is written as
$ \begin{aligned}[b] \Psi_{J M_J, I} &= \sum\limits_{i, j, k} c_{ijk} \Psi_{J M_J, I, i, j, k} \\ &=\sum\limits_{i, j, k} c_{ijk} {\cal A} \left[ \left[ \psi_{L M_L} \chi^{\sigma_i}_{S M_S}(4) \right]_{J M_J} \chi^{f_j}_I \chi^{c}_k \right] \,, \end{aligned} $

(56) where
$ \cal{A} $ is the anti-symmetry operator of$ \bar{q}q\bar{s}Q $ tetraquark systems, which considers the use of$ S U(3)$ flavor symmetry. According to Fig. 1, it is defined as$ {\cal{A}} = 1-(13) \,. $

(57) This is necessary in our theoretical framework because the complete wave function of the four-quark system is constructed from two sub-clusters: meson-meson, diquark-antidiquark, and K-type configurations.
Because the anti-symmetry operator and Gaussian basis functions are employed, the different channels, which include meson-meson in singlet- and hidden-color arrangements, diquark-antidiquark, and K-type configurations, are not orthogonal to each other. This is inevitable in our theoretical framework; however, the off-diagonal matrix elements are very small numerically and negligible compared with the diagonal ones. Accordingly, a quantitative analysis of the inter-quark distances,
$ \begin{array}{*{20}{l}} r_{q\bar{q}} = \sqrt{\langle \Psi_{J M_J, I} \vert r^2_{q \bar{q}} \vert \Psi_{J M_J, I} \rangle} \,, \end{array} $

(58) and a qualitative survey of dominant components,
$ \begin{array}{*{20}{l}} C_p =\sum\limits_{i,j,k\in C_p} \langle c^l_{ijk} \Psi_{J M_J, I, ijk} \vert c^r_{ijk} \Psi_{J M_J, I, ijk} \rangle \,, \end{array} $

(59) can be conducted. In the formula above,
$ c^l_{ijk} $ and$ c^r_{ijk} $ are the left and right generalized eigenvectors, respectively, of the complete anti-symmetric complex wave-function. Additionally, note that only the real part of these complex quantities are discussed herein to gain insights into the nature of$ \bar{q}q\bar{s}Q $ tetraquarks.In the next section, which discusses the computed results on the
$ \bar{q}q\bar{s}Q $ tetraquarks, we first study the systems through a real-range analysis, viz., the rotated angle θ is equal to$ 0^{\circ} $ . In this case, when a complete coupled-channel calculation of matrix diagonalization is performed, possible resonant states are embedded in the continuum. However, we can employ the CSM, with appropriate non-zero values of θ, to disentangle bound, resonance, and scattering states in a complex energy plane. Accordingly, for solving manageable eigevalue problems, the artificial parameter of rotated angle is ranged form$ 0^\circ $ to$ 6^\circ $ . Meanwhile, with the cooperation of real- and complex-range computations, available exotic states, which are first obtained within a complex-range analysis and then can be identified among continuum states according to its mass in a real-range calculation, are further investigated by analyzing their dominant quark arrangements, sizes, and decay patterns. -
The S-wave
$ \bar{q}q\bar{s}Q \;\; (q=u,\,d;\,Q=c,\,b) $ tetraquarks are systematically studied by including meson-meson, diquark-antidiquark, and K-type configurations. Therefore, the total angular momentum, J, coincides with the total spin, S, and can have values of$ 0 $ ,$ 1 $ , and$ 2 $ . Thus, the parity of tetraquark system is positive. Furthermore, both the iso-scalar$ (I=0) $ and -vector$ (I=1) $ sectors of$ \bar{q}q\bar{s}Q $ tetraquarks are considered.Tables 4 to 27 list the calculated results of low-lying
$ \bar{q}q\bar{s}Q $ tetraquark states. In particular, real-range computations on the lowest-lying masses of each tetraquark system in the allowed$ I(J^P) $ quantum numbers are presented in Tables 4, 6, 8, 10, 12, 14, 16, 18, 20, 22, 24, and 26. Therein, the considered meson-meson, diquark-antidiquark, and K-type configurations are listed in the first column; if available, the experimental value of the non-interacting di-meson threshold is labeled in parentheses. In the second column, each channel is assigned with an index, which indicates a particular combination of spin ($ \chi_J^{\sigma_i} $ ), flavor ($ \chi_I^{f_j} $ ), and color ($ \chi_k^c $ ) wave functions, which are shown explicitly in the third column. The theoretical mass calculated in each channel is shown in the fourth column, and the coupled result for each type of configuration is presented in the last one. The last row of the table indicates the lowest-lying mass, which is obtained in a complete coupled-channel calculation within the real-range formalism.Channel Index $ \chi_J^{\sigma_i} $ ;

$ \chi_I^{f_j} $ ;

$ \chi_k^c $ 

$ [i; j; k] $ 

M Mixed $ (\eta D_s)^1 (2516) $ 

1 [1; 1; 1] $ 2678 $ 

$ (\omega D^*_s)^1 (2894) $ 

2 [2; 1; 1] $ 2811 $ 

$ (K D)^1 (2364) $ 

3 [1; 1; 1] $ 2378 $ 

$ (K^* D^*)^1 (2899) $ 

4 [2; 1; 1] $ 2924 $ 

$ 2378 $ 

$ (\eta D_s)^8 $ 

5 [1; 1; 2] $ 3293 $ 

$ (\omega D^*_s)^8 $ 

6 [2; 1; 2] $ 3107 $ 

$ (K D)^8 $ 

7 [1; 1; 2] $ 3169 $ 

$ (K^* D^*)^8 $ 

8 [2; 1; 2] $ 3186 $ 

$ 2932 $ 

$ (qc)(\bar{q}\bar{s}) $ 

9 [3; 2; 4] $ 3188 $ 

$ (qc)(\bar{q}\bar{s}) $ 

10 [3; 3; 3] $ 2913 $ 

$ (qc)^*(\bar{q}\bar{s})^* $ 

11 [4; 2; 3] $ 3186 $ 

$ (qc)^*(\bar{q}\bar{s})^* $ 

12 [4; 3; 4] $ 3074 $ 

$ 2777 $ 

$ K_1 $ 

13 [5; 1; 5] $ 3102 $ 

14 [6; 1; 5] $ 3286 $ 

15 [5; 1; 6] $ 3066 $ 

16 [6; 1; 6] $ 3071 $ 

$ 2961 $ 

$ K_2 $ 

17 [7; 1; 7] $ 3094 $ 

18 [8; 1; 7] $ 3228 $ 

19 [7; 1; 8] $ 3207 $ 

20 [8; 1; 8] $ 3080 $ 

$ 2850 $ 

$ K_3 $ 

21 [9; 2; 10] $ 2920 $ 

22 [9; 3; 9] $ 3071 $ 

23 [10; 2; 9] $ 3190 $ 

24 [10; 3; 10] $ 2920 $ 

$ 2723 $ 

Complete coupled-channels: $ 2378 $ 

Table 4. Lowest-lying
$ \bar{q}q\bar{s}c $ tetraquark states with$ I(J^P)= $ $ 0(0^+) $ calculated within the real range formulation of the chiral quark model. The allowed meson-meson, diquark-antidiquark, and K-type configurations are listed in the first column; when possible, the experimental value of the non-interacting meson-meson threshold is labeled in parentheses. Each channel is assigned an index in the second column; it reflects a particular combination of spin ($ \chi_J^{\sigma_i} $ ), flavor ($ \chi_I^{f_j} $ ), and color ($ \chi_k^c $ ) wave functions, which are shown explicitly in the third column. The theoretical mass obtained in each channel is shown in the fourth column, and the coupled result for each type of configuration is presented in the fifth column. When a complete coupled-channel calculation is performed, the last row of the table indicates the calculated lowest-lying mass (unit: MeV).Channel Index $ \chi_J^{\sigma_i} $ ;

$ \chi_I^{f_j} $ ;

$ \chi_k^c $ 

$ [i; j; k] $ 

M Mixed $ (\eta D^*_s)^1 (2660) $ 

1 [1; 1; 1] $ 2804 $ 

$ (\omega D_s)^1 (2750) $ 

2 [2; 1; 1] $ 2685 $ 

$ (\omega D^*_s)^1 (2894) $ 

3 [3; 1; 1] $ 2811 $ 

$ (K D^*)^1 (2501) $ 

4 [1; 1; 1] $ 2498 $ 

$ (K^* D)^1 (2762) $ 

5 [2; 1; 1] $ 2804 $ 

$ (K^* D^*)^1 (2899) $ 

6 [3; 1; 1] $ 2924 $ 

$ 2498 $ 

$ (\eta D^*_s)^8 $ 

7 [1; 1; 2] $ 3296 $ 

$ (\omega D_s)^8 $ 

8 [2; 1; 2] $ 3179 $ 

$ (\omega D^*_s)^8 $ 

9 [3; 1; 2] $ 3147 $ 

$ (K D^*)^8 $ 

10 [1; 1; 2] $ 3174 $ 

$ (K^* D)^8 $ 

11 [2; 1; 2] $ 3191 $ 

$ (K^* D^*)^8 $ 

12 [3; 1; 2] $ 3192 $ 

$ 2897 $ 

$ (qc)(\bar{q}\bar{s})^* $ 

13 [4; 2; 4] $ 3181 $ 

$ (qc)(\bar{q}\bar{s})^* $ 

14 [4; 3; 3] $ 2949 $ 

$ (qc)^*(\bar{q}\bar{s}) $ 

15 [5; 2; 3] $ 3159 $ 

$ (qc)^*(\bar{q}\bar{s}) $ 

16 [5; 3; 4] $ 3162 $ 

$ (qc)^*(\bar{q}\bar{s})^* $ 

17 [6; 2; 3] $ 3169 $ 

$ (qc)^*(\bar{q}\bar{s})^* $ 

18 [6; 3; 4] $ 3094 $ 

$ 2852 $ 

$ K_1 $ 

19 [7; 1; 5] $ 3178 $ 

20 [8; 1; 5] $ 3121 $ 

21 [9; 1; 5] $ 3291 $ 

22 [7; 1; 6] $ 3044 $ 

23 [8; 1; 6] $ 3053 $ 

24 [9; 1; 6] $ 3113 $ 

$ 3005 $ 

$ K_2 $ 

25 [10; 1; 7] $ 3109 $ 

26 [11; 1; 7] $ 3186 $ 

27 [12; 1; 7] $ 3187 $ 

28 [10; 1; 8] $ 3045 $ 

29 [11; 1; 8] $ 3213 $ 

30 [12; 1; 8] $ 3187 $ 

$ 2956 $ 

$ K_3 $ 

31 [13; 2; 10] $ 3085 $ 

32 [13; 3; 9] $ 3083 $ 

33 [14; 2; 10] $ 3196 $ 

34 [14; 3; 9] $ 3112 $ 

35 [15; 2; 10] $ 3178 $ 

36 [15; 3; 9] $ 2946 $ 

$ 2787 $ 

Complete coupled-channels: $ 2498 $ 

Table 6. Lowest-lying
$ \bar{q}q\bar{s}c $ tetraquark states with$I(J^P)= $ $ 0(1^+)$ calculated within the real range formulation of the chiral quark model. The results are presented in the same manner as that in Table 4 (unit: MeV).Channel Index $ \chi_J^{\sigma_i} $ ;

$ \chi_I^{f_j} $ ;

$ \chi_k^c $ 

$ [i; j; k] $ 

M Mixed $ (\omega D^*_s)^1 (2894) $ 

1 [1; 1; 1] $ 2811 $ 

$ (K^* D^*)^1 (2899) $ 

2 [1; 1; 1] $ 2924 $ 

$ 2811 $ 

$ (\omega D^*_s)^8 $ 

3 [1; 1; 2] $ 3216 $ 

$ (K^* D^*)^8 $ 

4 [1; 1; 2] $ 3201 $ 

$ 3104 $ 

$ (qc)^*(\bar{q}\bar{s})^* $ 

5 [1; 2; 3] $ 3132 $ 

$ (qc)^*(\bar{q}\bar{s})^* $ 

6 [1; 3; 4] $ 3130 $ 

$ 3119 $ 

$ K_1 $ 

7 [1; 1; 5] $ 3200 $ 

8 [1; 1; 6] $ 3071 $ 

$ 3070 $ 

$ K_2 $ 

9 [1; 1; 7] $ 3156 $ 

10 [1; 1; 8] $ 3159 $ 

$ 3115 $ 

$ K_3 $ 

11 [1; 2; 10] $ 3119 $ 

12 [1; 3; 9] $ 3111 $ 

$ 3104 $ 

Complete coupled-channels: $ 2811 $ 

Table 8. Lowest-lying
$ \bar{q}q\bar{s}c $ tetraquark states with$I(J^P)= $ $ 0(2^+)$ calculated within the real range formulation of the chiral quark model. The results are presented in the same manner as that in Table 4 (unit: MeV).Channel Index $ \chi_J^{\sigma_i} $ ;

$ \chi_I^{f_j} $ ;

$ \chi_k^c $ 

$ [i; j; k] $ 

M Mixed $ (\pi D_s)^1 (2108) $ 

1 [1; 1; 1] $ 2138 $ 

$ (\rho D^*_s)^1 (2882) $ 

2 [2; 1; 1] $ 2887 $ 

$ (K D)^1 (2364) $ 

3 [1; 1; 1] $ 2378 $ 

$ (K^* D^*)^1 (2899) $ 

4 [2; 1; 1] $ 2924 $ 

$ 2138 $ 

$ (\pi D_s)^8 $ 

5 [1; 1; 2] $ 3177 $ 

$ (\rho D^*_s)^8 $ 

6 [2; 1; 2] $ 3167 $ 

$ (K D)^8 $ 

7 [1; 1; 2] $ 3169 $ 

$ (K^* D^*)^8 $ 

8 [2; 1; 2] $ 3105 $ 

$ 2894 $ 

$ (qc)(\bar{q}\bar{s}) $ 

9 [3; 2; 4] $ 3188 $ 

$ (qc)(\bar{q}\bar{s}) $ 

10 [3; 3; 3] $ 2913 $ 

$ (qc)^*(\bar{q}\bar{s})^* $ 

11 [4; 2; 3] $ 3084 $ 

$ (qc)^*(\bar{q}\bar{s})^* $ 

12 [4; 3; 4] $ 2956 $ 

$ 2772 $ 

$ K_1 $ 

13 [5; 1; 5] $ 3166 $ 

14 [6; 1; 5] $ 3167 $ 

15 [5; 1; 6] $ 3157 $ 

16 [6; 1; 6] $ 2475 $ 

$ 2460 $ 

$ K_2 $ 

17 [7; 1; 7] $ 2972 $ 

18 [8; 1; 7] $ 3228 $ 

19 [7; 1; 8] $ 3105 $ 

20 [8; 1; 8] $ 3080 $ 

$ 2826 $ 

$ K_3 $ 

21 [9; 2; 10] $ 3062 $ 

22 [9; 3; 9] $ 2891 $ 

23 [10; 2; 9] $ 3190 $ 

24 [10; 3; 10] $ 2920 $ 

$ 2700 $ 

Complete coupled-channels: $ 2138 $ 

Table 10. Lowest-lying
$ \bar{q}q\bar{s}c $ tetraquark states with$ I(J^P)=1(0^+) $ calculated within the real range formulation of the chiral quark model. The results are presented in the same manner as that in Table 4 (unit: MeV).Channel Index $ \chi_J^{\sigma_i} $ ;

$ \chi_I^{f_j} $ ;

$ \chi_k^c $ 

$ [i; j; k] $ 

M Mixed $ (\pi D^*_s)^1 (2252) $ 

1 [1; 1; 1] $ 2264 $ 

$ (\rho D_s)^1 (2738) $ 

2 [2; 1; 1] $ 2761 $ 

$ (\rho D^*_s)^1 (2882) $ 

3 [3; 1; 1] $ 2887 $ 

$ (K D^*)^1 (2501) $ 

4 [1; 1; 1] $ 2498 $ 

$ (K^* D)^1 (2762) $ 

5 [2; 1; 1] $ 2804 $ 

$ (K^* D^*)^1 (2899) $ 

6 [3; 1; 1] $ 2924 $ 

$ 2264 $ 

$ (\pi D^*_s)^8 $ 

7 [1; 1; 2] $ 3181 $ 

$ (\rho D_s)^8 $ 

8 [2; 1; 2] $ 3231 $ 

$ (\rho D^*_s)^8 $ 

9 [3; 1; 2] $ 3203 $ 

$ (K D^*)^8 $ 

10 [1; 1; 2] $ 3174 $ 

$ (K^* D)^8 $ 

11 [2; 1; 2] $ 3191 $ 

$ (K^* D^*)^8 $ 

12 [3; 1; 2] $ 3153 $ 

$ 2951 $ 

$ (qc)(\bar{q}\bar{s})^* $ 

13 [4; 2; 4] $ 3181 $ 

$ (qc)(\bar{q}\bar{s})^* $ 

14 [4; 3; 3] $ 2949 $ 

$ (qc)^*(\bar{q}\bar{s}) $ 

15 [5; 2; 3] $ 3159 $ 

$ (qc)^*(\bar{q}\bar{s}) $ 

16 [5; 3; 4] $ 3162 $ 

$ (qc)^*(\bar{q}\bar{s})^* $ 

17 [6; 2; 3] $ 3119 $ 

$ (qc)^*(\bar{q}\bar{s})^* $ 

18 [6; 3; 4] $ 3041 $ 

$ 2871 $ 

$ K_1 $ 

19 [7; 1; 5] $ 3233 $ 

20 [8; 1; 5] $ 3183 $ 

21 [9; 1; 5] $ 3173 $ 

22 [7; 1; 6] $ 3134 $ 

23 [8; 1; 6] $ 3144 $ 

24 [9; 1; 6] $ 2518 $ 

$ 2509 $ 

$ K_2 $ 

25 [10; 1; 7] $ 3158 $ 

26 [11; 1; 7] $ 3081 $ 

27 [12; 1; 7] $ 3187 $ 

28 [10; 1; 8] $ 3105 $ 

29 [11; 1; 8] $ 3110 $ 

30 [12; 1; 8] $ 3187 $ 

$ 2972 $ 

$ K_3 $ 

31 [13; 2; 10] $ 3149 $ 

32 [13; 3; 9] $ 3148 $ 

33 [14; 2; 10] $ 3069 $ 

34 [14; 3; 9] $ 2942 $ 

35 [15; 2; 10] $ 3178 $ 

36 [15; 3; 9] $ 2946 $ 

$ 2791 $ 

Complete coupled-channels: $ 2264 $ 

Table 12. Lowest-lying
$ \bar{q}q\bar{s}c $ tetraquark states with$ I(J^P)=1(1^+) $ calculated within the real range formulation of the chiral quark model. The results are presented in the same manner as that in Table 4 (unit: MeV).Channel Index $ \chi_J^{\sigma_i} $ ;

$ \chi_I^{f_j} $ ;

$ \chi_k^c $ 

$ [i; j; k] $ 

M Mixed $ (\rho D^*_s)^1 (2882) $ 

1 [1; 1; 1] $ 2887 $ 

$ (K^* D^*)^1 (2899) $ 

2 [1; 1; 1] $ 2924 $ 

$ 2887 $ 

$ (\rho D^*_s)^8 $ 

3 [1; 1; 2] $ 3265 $ 

$ (K^* D^*)^8 $ 

4 [1; 1; 2] $ 3235 $ 

$ 3140 $ 

$ (qc)^*(\bar{q}\bar{s})^* $ 

5 [1; 2; 3] $ 3181 $ 

$ (qc)^*(\bar{q}\bar{s})^* $ 

6 [1; 3; 4] $ 3174 $ 

$ 3166 $ 

$ K_1 $ 

7 [1; 1; 5] $ 3253 $ 

8 [1; 1; 6] $ 3161 $ 

$ 3160 $ 

$ K_2 $ 

9 [1; 1; 7] $ 3202 $ 

10 [1; 1; 8] $ 3210 $ 

$ 3166 $ 

$ K_3 $ 

11 [1; 2; 10] $ 3180 $ 

12 [1; 3; 9] $ 3174 $ 

$ 3167 $ 

Complete coupled-channels: $ 2887 $ 

Table 14. Lowest-lying
$ \bar{q}q\bar{s}c $ tetraquark states with$I(J^P)= $ $ 1(2^+)$ calculated within the real range formulation of the chiral quark model. The results are presented in the same manner as that in Table 4 (unit: MeV).Channel Index $ \chi_J^{\sigma_i} $ ;

$ \chi_I^{f_j} $ ;

$ \chi_k^c $ 

$ [i; j; k] $ 

M Mixed $ (\eta B_s)^1 (5915) $ 

1 [1; 1; 1] $ 6044 $ 

$ (\omega B^*_s)^1 (6197) $ 

2 [2; 1; 1] $ 6096 $ 

$ (K B)^1 (5774) $ 

3 [1; 1; 1] $ 5759 $ 

$ (K^* B^*)^1 (6217) $ 

4 [2; 1; 1] $ 6226 $ 

$ 5759 $ 

$ (\eta B_s)^8 $ 

5 [1; 1; 2] $ 6613 $ 

$ (\omega B^*_s)^8 $ 

6 [2; 1; 2] $ 6440 $ 

$ (K B)^8 $ 

7 [1; 1; 2] $ 6361 $ 

$ (K^* B^*)^8 $ 

8 [2; 1; 2] $ 6498 $ 

$ 6292 $ 

$ (qb)(\bar{q}\bar{s}) $ 

9 [3; 2; 4] $ 6501 $ 

$ (qb)(\bar{q}\bar{s}) $ 

10 [3; 3; 3] $ 6226 $ 

$ (qb)^*(\bar{q}\bar{s})^* $ 

11 [4; 2; 3] $ 6486 $ 

$ (qb)^*(\bar{q}\bar{s})^* $ 

12 [4; 3; 4] $ 6414 $ 

$ 6113 $ 

$ K_1 $ 

13 [5; 1; 5] $ 6403 $ 

14 [6; 1; 5] $ 6585 $ 

15 [5; 1; 6] $ 6358 $ 

16 [6; 1; 6] $ 6387 $ 

$ 6281 $ 

$ K_2 $ 

17 [7; 1; 7] $ 6427 $ 

18 [8; 1; 7] $ 6531 $ 

19 [7; 1; 8] $ 6520 $ 

20 [8; 1; 8] $ 6411 $ 

$ 6210 $ 

$ K_3 $ 

21 [9; 2; 10] $ 6485 $ 

22 [9; 3; 9] $ 6382 $ 

23 [10; 2; 9] $ 6478 $ 

24 [10; 3; 10] $ 6221 $ 

$ 6038 $ 

Complete coupled-channels: $ 5759 $ 

Table 16. Lowest-lying
$ \bar{q}q\bar{s}b $ tetraquark states with$I(J^P)= $ $ 0(0^+)$ calculated within the real range formulation of the chiral quark model. The results are presented in the same manner as that in Table 4 (unit: MeV).Channel Index $ \chi_J^{\sigma_i} $ ;

$ \chi_I^{f_j} $ ;

$ \chi_k^c $ 

$ [i; j; k] $ 

M Mixed $ (\eta B^*_s)^1 (5963) $ 

1 [1; 1; 1] $ 6089 $ 

$ (\omega B_s)^1 (6149) $ 

2 [2; 1; 1] $ 6051 $ 

$ (\omega B^*_s)^1 (6197) $ 

3 [3; 1; 1] $ 6096 $ 

$ (K B^*)^1 (5819) $ 

4 [1; 1; 1] $ 5800 $ 

$ (K^* B)^1 (6172) $ 

5 [2; 1; 1] $ 6185 $ 

$ (K^* B^*)^1 (6217) $ 

6 [3; 1; 1] $ 6226 $ 

$ 5800 $ 

$ (\eta B^*_s)^8 $ 

7 [1; 1; 2] $ 6614 $ 

$ (\omega B_s)^8 $ 

8 [2; 1; 2] $ 6496 $ 

$ (\omega B^*_s)^8 $ 

9 [3; 1; 2] $ 6470 $ 

$ (K B^*)^8 $ 

10 [1; 1; 2] $ 6361 $ 

$ (K^* B)^8 $ 

11 [2; 1; 2] $ 6360 $ 

$ (K^* B^*)^8 $ 

12 [3; 1; 2] $ 6497 $ 

$ 6315 $ 

$ (qb)(\bar{q}\bar{s})^* $ 

13 [4; 2; 4] $ 6498 $ 

$ (qb)(\bar{q}\bar{s})^* $ 

14 [4; 3; 3] $ 6239 $ 

$ (qb)^*(\bar{q}\bar{s}) $ 

15 [5; 2; 3] $ 6474 $ 

$ (qb)^*(\bar{q}\bar{s}) $ 

16 [5; 3; 4] $ 6473 $ 

$ (qb)^*(\bar{q}\bar{s})^* $ 

17 [6; 2; 3] $ 6466 $ 

$ (qb)^*(\bar{q}\bar{s})^* $ 

18 [6; 3; 4] $ 6421 $ 

$ 6140 $ 

$ K_1 $ 

19 [7; 1; 5] $ 6480 $ 

20 [8; 1; 5] $ 6410 $ 

21 [9; 1; 5] $ 6588 $ 

22 [7; 1; 6] $ 6351 $ 

23 [8; 1; 6] $ 6352 $ 

24 [9; 1; 6] $ 6405 $ 

$ 6299 $ 

$ K_2 $ 

25 [10; 1; 7] $ 6420 $ 

26 [11; 1; 7] $ 6502 $ 

27 [12; 1; 7] $ 6491 $ 

28 [10; 1; 8] $ 6353 $ 

29 [11; 1; 8] $ 6521 $ 

30 [12; 1; 8] $ 6512 $ 

$ 6254 $ 

$ K_3 $ 

31 [13; 2; 10] $ 6389 $ 

32 [13; 3; 9] $ 6386 $ 

33 [14; 2; 10] $ 6488 $ 

34 [14; 3; 9] $ 6398 $ 

35 [15; 2; 10] $ 6474 $ 

36 [15; 3; 9] $ 6231 $ 

$ 6063 $ 

Complete coupled-channels: $ 5800 $ 

Table 18. Lowest-lying
$ \bar{q}q\bar{s}b $ tetraquark states with$I(J^P)= $ $ 0(1^+)$ calculated within the real range formulation of the chiral quark model. The results are presented in the same manner as that in Table 4 (unit: MeV).Channel Index $ \chi_J^{\sigma_i} $ ;

$ \chi_I^{f_j} $ ;

$ \chi_k^c $ 

$ [i; j; k] $ 

M Mixed $ (\omega B^*_s)^1 (6197) $ 

1 [1; 1; 1] $ 6096 $ 

$ (K^* B^*)^1 (6217) $ 

2 [1; 1; 1] $ 6226 $ 

$ 6096 $ 

$ (\omega B^*_s)^8 $ 

3 [1; 1; 2] $ 6524 $ 

$ (K^* B^*)^8 $ 

4 [1; 1; 2] $ 6497 $ 

$ 6411 $ 

$ (qb)^*(\bar{q}\bar{s})^* $ 

5 [1; 2; 3] $ 6422 $ 

$ (qb)^*(\bar{q}\bar{s})^* $ 

6 [1; 3; 4] $ 6435 $ 

$ 6413 $ 

$ K_1 $ 

7 [1; 1; 5] $ 6489 $ 

8 [1; 1; 6] $ 6363 $ 

$ 6362 $ 

$ K_2 $ 

9 [1; 1; 7] $ 6455 $ 

10 [1; 1; 8] $ 6458 $ 

$ 6400 $ 

$ K_3 $ 

11 [1; 2; 10] $ 6403 $ 

12 [1; 3; 9] $ 6397 $ 

$ 6387 $ 

Complete coupled-channels: $ 6096 $ 

Table 20. Lowest-lying
$ \bar{q}q\bar{s}b $ tetraquark states with$I(J^P)= $ $ 0(2^+)$ calculated within the real range formulation of the chiral quark model. The results are presented in the same manner as that in Table 4 (unit: MeV).Channel Index $ \chi_J^{\sigma_i} $ ;

$ \chi_I^{f_j} $ ;

$ \chi_k^c $ 

$ [i; j; k] $ 

M Mixed $ (\pi B_s)^1 (5507) $ 

1 [1; 1; 1] $ 5504 $ 

$ (\rho B^*_s)^1 (6185) $ 

2 [2; 1; 1] $ 6172 $ 

$ (K B)^1 (5774) $ 

3 [1; 1; 1] $ 5759 $ 

$ (K^* B^*)^1 (6217) $ 

4 [2; 1; 1] $ 6226 $ 

$ 5504 $ 

$ (\pi B_s)^8 $ 

5 [1; 1; 2] $ 6494 $ 

$ (\rho B^*_s)^8 $ 

6 [2; 1; 2] $ 6499 $ 

$ (K B)^8 $ 

7 [1; 1; 2] $ 6361 $ 

$ (K^* B^*)^8 $ 

8 [2; 1; 2] $ 6420 $ 

$ 6243 $ 

$ (qb)(\bar{q}\bar{s}) $ 

9 [3; 2; 4] $ 6501 $ 

$ (qb)(\bar{q}\bar{s}) $ 

10 [3; 3; 3] $ 6226 $ 

$ (qb)^*(\bar{q}\bar{s})^* $ 

11 [4; 2; 3] $ 6298 $ 

$ (qb)^*(\bar{q}\bar{s})^* $ 

12 [4; 3; 4] $ 6302 $ 

$ 6123 $ 

$ K_1 $ 

13 [5; 1; 5] $ 6468 $ 

14 [6; 1; 5] $ 6463 $ 

15 [5; 1; 6] $ 6449 $ 

16 [6; 1; 6] $ 5792 $ 

$ 5780 $ 

$ K_2 $ 

17 [7; 1; 7] $ 6315 $ 

18 [8; 1; 7] $ 6531 $ 

19 [7; 1; 8] $ 6417 $ 

20 [8; 1; 8] $ 6411 $ 

$ 6200 $ 

$ K_3 $ 

21 [9; 2; 10] $ 6355 $ 

22 [9; 3; 9] $ 6203 $ 

23 [10; 2; 9] $ 6478 $ 

24 [10; 3; 10] $ 6221 $ 

$ 6029 $ 

Complete coupled-channels: $ 5504 $ 

Table 22. Lowest-lying
$ \bar{q}q\bar{s}b $ tetraquark states with$I(J^P)= $ $ 1(0^+)$ calculated within the real range formulation of the chiral quark model. The results are presented in the same manner as that in Table 4 (unit: MeV).Channel Index $ \chi_J^{\sigma_i} $ ;

$ \chi_I^{f_j} $ ;

$ \chi_k^c $ 

$ [i; j; k] $ 

M Mixed $ (\pi B^*_s)^1 (5555) $ 

1 [1; 1; 1] $ 5549 $ 

$ (\rho B_s)^1 (6137) $ 

2 [2; 1; 1] $ 6127 $ 

$ (\rho B^*_s)^1 (6185) $ 

3 [3; 1; 1] $ 6172 $ 

$ (K B^*)^1 (5819) $ 

4 [1; 1; 1] $ 5800 $ 

$ (K^* B)^1 (6172) $ 

5 [2; 1; 1] $ 6185 $ 

$ (K^* B^*)^1 (6217) $ 

6 [3; 1; 1] $ 6226 $ 

$ 5549 $ 

$ (\pi B^*_s)^8 $ 

7 [1; 1; 2] $ 6496 $ 

$ (\rho B_s)^8 $ 

8 [2; 1; 2] $ 6550 $ 

$ (\rho B^*_s)^8 $ 

9 [3; 1; 2] $ 6526 $ 

$ (K B^*)^8 $ 

10 [1; 1; 2] $ 6361 $ 

$ (K^* B)^8 $ 

11 [2; 1; 2] $ 6360 $ 

$ (K^* B^*)^8 $ 

12 [3; 1; 2] $ 6460 $ 

$ 6275 $ 

$ (qb)(\bar{q}\bar{s})^* $ 

13 [4; 2; 4] $ 6498 $ 

$ (qb)(\bar{q}\bar{s})^* $ 

14 [4; 3; 3] $ 6239 $ 

$ (qb)^*(\bar{q}\bar{s}) $ 

15 [5; 2; 3] $ 6474 $ 

$ (qb)^*(\bar{q}\bar{s}) $ 

16 [5; 3; 4] $ 6473 $ 

$ (qb)^*(\bar{q}\bar{s})^* $ 

17 [6; 2; 3] $ 6415 $ 

$ (qb)^*(\bar{q}\bar{s})^* $ 

18 [6; 3; 4] $ 6370 $ 

$ 6159 $ 

$ K_1 $ 

19 [7; 1; 5] $ 6536 $ 

20 [8; 1; 5] $ 6474 $ 

21 [9; 1; 5] $ 6466 $ 

22 [7; 1; 6] $ 6442 $ 

23 [8; 1; 6] $ 6443 $ 

24 [9; 1; 6] $ 5809 $ 

$ 5800 $ 

$ K_2 $ 

25 [10; 1; 7] $ 6468 $ 

26 [11; 1; 7] $ 6405 $ 

27 [12; 1; 7] $ 6491 $ 

28 [10; 1; 8] $ 6415 $ 

29 [11; 1; 8] $ 6418 $ 

30 [12; 1; 8] $ 6512 $ 

$ 6261 $ 

$ K_3 $ 

31 [13; 2; 10] $ 6454 $ 

32 [13; 3; 9] $ 6451 $ 

33 [14; 2; 10] $ 6358 $ 

34 [14; 3; 9] $ 6224 $ 

35 [15; 2; 10] $ 6474 $ 

36 [15; 3; 9] $ 6231 $ 

$ 6065 $ 

Complete coupled-channels: $ 5549 $ 

Table 24. Lowest-lying
$ \bar{q}q\bar{s}b $ tetraquark states with$I(J^P)= $ $ 1(1^+)$ calculated within the real range formulation of the chiral quark model. The results are presented in the same manner as that in Table 4 (unit: MeV).Channel Index $ \chi_J^{\sigma_i} $ ;

$ \chi_I^{f_j} $ ;

$ \chi_k^c $ 

$ [i; j; k] $ 

M Mixed $ (\rho B^*_s)^1 (6185) $ 

1 [1; 1; 1] $ 6172 $ 

$ (K^* B^*)^1 (6217) $ 

2 [1; 1; 1] $ 6226 $ 

$ 6172 $ 

$ (\rho B^*_s)^8 $ 

3 [1; 1; 2] $ 6575 $ 

$ (K^* B^*)^8 $ 

4 [1; 1; 2] $ 6531 $ 

$ 6448 $ 

$ (qb)^*(\bar{q}\bar{s})^* $ 

5 [1; 2; 3] $ 6472 $ 

$ (qb)^*(\bar{q}\bar{s})^* $ 

6 [1; 3; 4] $ 6479 $ 

$ 6462 $ 

$ K_1 $ 

7 [1; 1; 5] $ 6544 $ 

8 [1; 1; 6] $ 6453 $ 

$ 6452 $ 

$ K_2 $ 

9 [1; 1; 7] $ 6500 $ 

10 [1; 1; 8] $ 6510 $ 

$ 6451 $ 

$ K_3 $ 

11 [1; 2; 10] $ 6466 $ 

12 [1; 3; 9] $ 6462 $ 

$ 6452 $ 

Complete coupled-channels: $ 6172 $ 

Table 26. Lowest-lying
$ \bar{q}q\bar{s}b $ tetraquark states with$I(J^P)= $ $ 1(2^+)$ calculated within the real range formulation of the chiral quark model. The results are presented in the same manner as that in Table 4 (unit: MeV).Resonance Structure $6301+{\rm i}0.8$ 

$ r_{q\bar{q}}:1.03 $ ;

$ r_{\bar{q}\bar{q}}:1.08 $ ;

$ r_{b\bar{q}}:0.95 $ ;

$ r_{qb}:1.00 $ 

S: 15.1%; H: 11.5%; $ Di $ : 13.9%; K: 59.5%

$6399+{\rm i}2.6$ 

$ r_{q\bar{q}}:1.16 $ ;

$ r_{\bar{q}\bar{q}}:1.14 $ ;

$ r_{b\bar{q}}:0.79 $ ;

$ r_{qb}:1.02 $ 

S: 15.2%; H: 15.9%; $ Di $ : 11.8%; K: 57.1%

$6654+{\rm i}5.3$ 

$ r_{q\bar{q}}:1.48 $ ;

$ r_{\bar{q}\bar{q}}:1.50 $ ;

$ r_{b\bar{q}}:1.34 $ ;

$ r_{qb}:1.43 $ 

S: 10.4%; H: 15.4%; $ Di $ : 27.8%; K: 46.4%

$6740+{\rm i}10.0$ 

$ r_{q\bar{q}}:1.48 $ ;

$ r_{\bar{q}\bar{q}}:1.42 $ ;

$ r_{b\bar{q}}:1.16 $ ;

$ r_{qb}:1.32 $ 

S: 4.9%; H: 7.3%; $ Di $ : 41.6%; K: 46.2%

Table 27. Compositeness of exotic resonances obtained in a complete coupled-channel calculation in the
$ 1(2^+) $ state of the$ \bar{q}q\bar{s}b $ tetraquark. The results are presented in the same manner as that in Table 5.In a further step, a complete coupled-channel calculation is performed using the CSM in each
$ I(J^P) \;\; \bar{q}q\bar{s}Q $ tetraquark system. Figures 2 to 13 show the distribution of complex eigenenergies, and therein, the obtained resonance states are indicated inside circles. Several insights about the nature of these resonances are given by calculating their interquark sizes and dominant components; correspondingly, results are listed among Tables 5, 7, 9, 11, 13, 15, 17, 19, 21, 23, 25 and 27. In particular, because the$S U(3)$ flavor symmetry is considered for the$ \bar{q}q\bar{s}Q $ tetraquark systems, four types of quark distances, which are$ r_{q\bar{q}} $ ,$ r_{\bar{q}\bar{q}} $ ,$ r_{c\bar{q}} $ , and$ r_{qc} \;\; (q=u,\,d,\,s) $ , are calculated. Finally, a summary of our most salient results is presented in Table 28.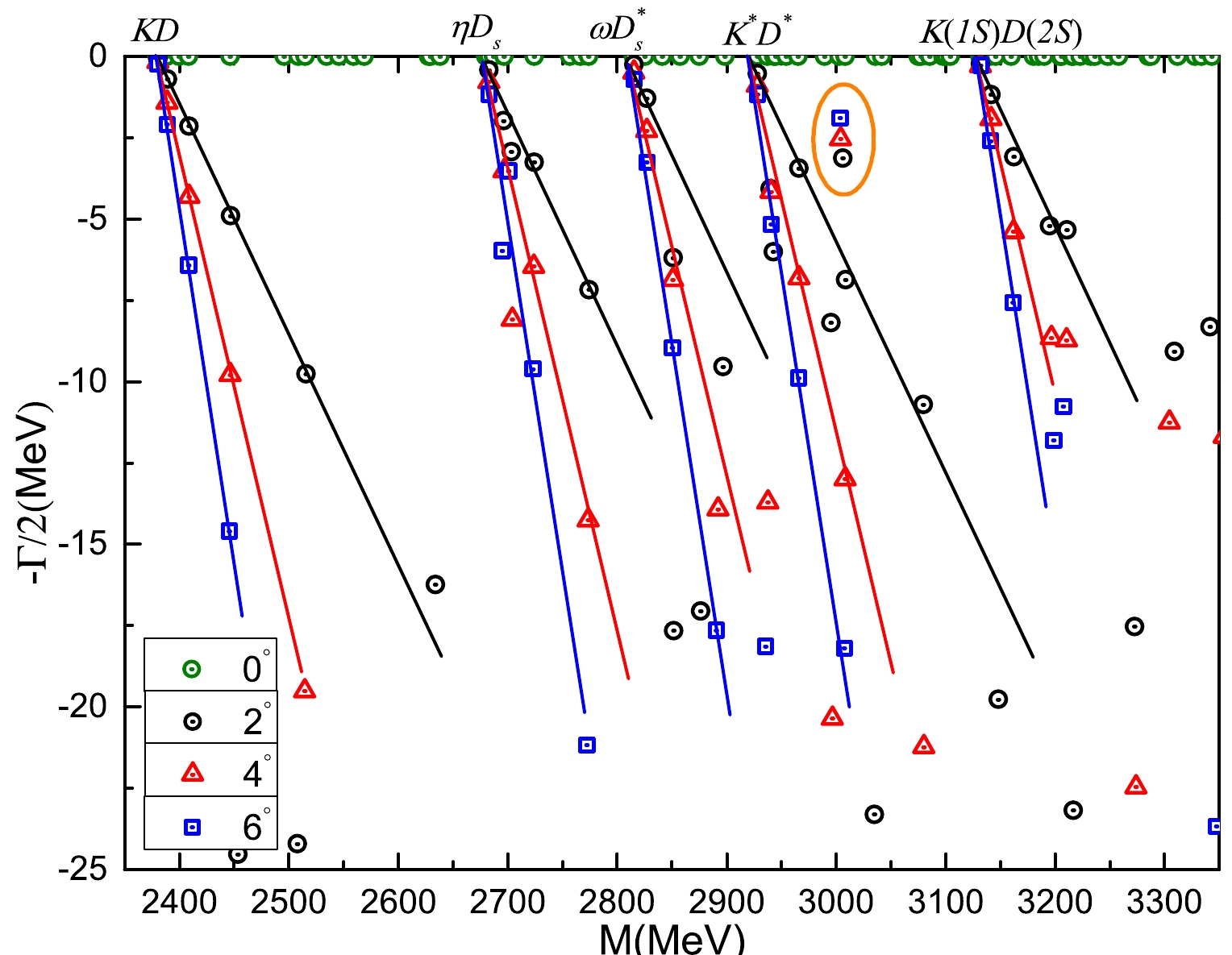
Figure 2. (color online) Complete coupled-channel calculation of the
$ \bar{q}q\bar{s}c $ tetraquark system with$ I(J^P)=0(0^+) $ quantum numbers.
Figure 13. (color online) Complete coupled-channel calculation of the
$ \bar{q}q\bar{s}b $ tetraquark system with$ I(J^P)=1(2^+) $ quantum numbers.Resonance Structure $3006+{\rm i}6.3$ 

$ r_{q\bar{q}}:1.64 $ ;

$ r_{\bar{q}\bar{q}}:2.23 $ ;

$ r_{c\bar{q}}:1.70 $ ;

$ r_{qc}:2.19 $ 

S: 21.3%; H: 9.1%; $ Di $ : 13.1%; K: 56.5%

Table 5. Compositeness of the exotic resonance obtained in a complete coupled-channel calculation in the
$ 0(0^+) $ state of the$ \bar{q}q\bar{s}c $ tetraquark. In particular, the first column shows the resonance pole labeled by$M+{\rm i}\Gamma$ (unit: MeV); the second one shows the distance between any two quarks or quark-antiquark ($ q=u,d,s $ ) pair (unit: fm) and the component of resonance state (S: di-meson structure in color-singlet channel; H: di-meson structure in the hidden-color channel;$ Di $ : diquark-antiquark configuration; K: K-type configuration).Resonance Structure $3119+{\rm i}19.8$ 

$ r_{q\bar{q}}:1.41 $ ;

$ r_{\bar{q}\bar{q}}:1.63 $ ;

$ r_{c\bar{q}}:1.03 $ ;

$ r_{qc}:1.61 $ 

S: 8.5%; H: 18.7%; $ Di $ : 29.6%; K: 43.2%

$3292+{\rm i}13.1$ 

$ r_{q\bar{q}}:1.14 $ ;

$ r_{\bar{q}\bar{q}}:1.13 $ ;

$ r_{c\bar{q}}:1.14 $ ;

$ r_{qc}:1.37 $ 

S: 9.6%; H: 13.9%; $ Di $ : 34.5%; K: 42.0%

$3346+{\rm i}22.0$ 

$ r_{q\bar{q}}:1.54 $ ;

$ r_{\bar{q}\bar{q}}:1.75 $ ;

$ r_{c\bar{q}}:1.49 $ ;

$ r_{qc}:1.64 $ 

S: 6.7%; H: 8.8%; $ Di $ : 34.7%; K: 49.8%

Table 7. Compositeness of exotic resonances obtained in a complete coupled-channel calculation in the
$ 0(1^+) $ state of the$ \bar{q}q\bar{s}c $ tetraquark. The results are presented in the same manner as that in Table 5.Resonance Structure $2965+{\rm i}0.5$ 

$ r_{q\bar{q}}:1.18 $ ;

$ r_{\bar{q}\bar{q}}:1.19 $ ;

$ r_{c\bar{q}}:0.86 $ ;

$ r_{qc}:1.11 $ 

S: 18.9%; H: 8.1%; $ Di $ : 27.8%; K: 45.2%

$3026+{\rm i}3.8$ 

$ r_{q\bar{q}}:1.40 $ ;

$ r_{\bar{q}\bar{q}}:1.50 $ ;

$ r_{c\bar{q}}:1.02 $ ;

$ r_{qc}:1.43 $ 

S: 15.4%; H: 12.6%; $ Di $ : 7.3%; K: 64.7%

$3344+{\rm i}3.3$ 

$ r_{q\bar{q}}:1.46 $ ;

$ r_{\bar{q}\bar{q}}:1.54 $ ;

$ r_{c\bar{q}}:1.38 $ ;

$ r_{qc}:1.46 $ 

S: 13.4%; H: 20.2%; $ Di $ : 18.5%; K: 47.9%

Table 9. Compositeness of exotic resonances obtained in a complete coupled-channel calculation in the
$ 0(2^+) $ state of the$ \bar{q}q\bar{s}c $ tetraquark. The results are presented in the same manner as that in Table 5.Resonance Structure $2770+{\rm i}1.5$ 

$ r_{q\bar{q}}:1.05 $ ;

$ r_{\bar{q}\bar{q}}:1.80 $ ;

$ r_{c\bar{q}}:1.59 $ ;

$ r_{qc}:1.75 $ 

S: 15.6%; H: 19.2%; $ Di $ : 30.3%; K: 34.9%

Table 11. Compositeness of the exotic resonance obtained in a complete coupled-channel calculation in the
$ 1(0^+) $ state of the$ \bar{q}q\bar{s}c $ tetraquark. The results are presented in the same manner as that in Table 5.Resonance Structure $2470+{\rm i}1.0$ 

$ r_{q\bar{q}}:0.92 $ ;

$ r_{\bar{q}\bar{q}}:0.95 $ ;

$ r_{c\bar{q}}:0.88 $ ;

$ r_{qc}:0.79 $ 

S: 26.5%; H: 4.0%; $ Di $ : 13.5%; K: 56.0%

$3134+{\rm i}2.0$ 

$ r_{q\bar{q}}:1.79 $ ;

$ r_{\bar{q}\bar{q}}:1.87 $ ;

$ r_{c\bar{q}}:1.92 $ ;

$ r_{qc}:1.61 $ 

S: 16.1%; H: 18.0%; $ Di $ : 16.6%; K: 49.3%

Table 13. Compositeness of exotic resonances obtained in a complete coupled-channel calculation in the
$ 1(1^+) $ state of the$ \bar{q}q\bar{s}c $ tetraquark. The results are presented in the same manner as that in Table 5.Resonance Structure $3031+{\rm i}0.7$ 

$ r_{q\bar{q}}:1.02 $ ;

$ r_{\bar{q}\bar{q}}:1.07 $ ;

$ r_{c\bar{q}}:0.94 $ ;

$ r_{qc}:1.00 $ 

S: 16.4%; H: 14.1%; $ Di $ : 27.0%; K: 42.5%

$3105+{\rm i}3.7$ 

$ r_{q\bar{q}}:1.26 $ ;

$ r_{\bar{q}\bar{q}}:1.33 $ ;

$ r_{c\bar{q}}:0.99 $ ;

$ r_{qc}:1.26 $ 

S: 4.0%; H: 21.6%; $ Di $ : 28.0%; K: 46.4%

$3373+{\rm i}4.2$ 

$ r_{q\bar{q}}:1.51 $ ;

$ r_{\bar{q}\bar{q}}:1.61 $ ;

$ r_{c\bar{q}}:1.45 $ ;

$ r_{qc}:1.55 $ 

S: 8.0%; H: 10.6%; $ Di $ : 26.7%; K: 54.7%

$3455+{\rm i}12.9$ 

$ r_{q\bar{q}}:1.68 $ ;

$ r_{\bar{q}\bar{q}}:1.54 $ ;

$ r_{c\bar{q}}:1.30 $ ;

$ r_{qc}:1.45 $ 

S: 6.6%; H: 9.8%; $ Di $ : 39.4%; K: 44.2%

Table 15. Compositeness of exotic resonances obtained in a complete coupled-channel calculation in the
$ 1(2^+) $ state of$ \bar{q}q\bar{s}c $ tetraquark. The results are presented in the same manner as that in Table 5.Resonance Structure $6011+{\rm i}9.0$ 

$ r_{q\bar{q}}:1.54 $ ;

$ r_{\bar{q}\bar{q}}:1.53 $ ;

$ r_{b\bar{q}}:0.66 $ ;

$ r_{qb}:1.48 $ 

S: 19.5%; H: 7.5%; $ Di $ : 14.4%; K: 58.6%

$6323+{\rm i}4.0$ 

$ r_{q\bar{q}}:1.16 $ ;

$ r_{\bar{q}\bar{q}}:1.24 $ ;

$ r_{b\bar{q}}:0.94 $ ;

$ r_{qb}:1.18 $ 

S: 16.9%; H: 7.6%; $ Di $ : 21.9%; K: 53.6%

$6397+{\rm i}13.8$ 

$ r_{q\bar{q}}:0.96 $ ;

$ r_{\bar{q}\bar{q}}:1.81 $ ;

$ r_{b\bar{q}}:1.74 $ ;

$ r_{qb}:1.76 $ 

S: 7.8%; H: 17.6%; $ Di $ : 25.4%; K: 49.2%

$6643+{\rm i}23.4$ 

$ r_{q\bar{q}}:1.35 $ ;

$ r_{\bar{q}\bar{q}}:1.61 $ ;

$ r_{b\bar{q}}:1.31 $ ;

$ r_{qb}:1.52 $ 

S: 13.1%; H: 21.4%; $ Di $ : 31.1%; K: 34.4%

$6678+{\rm i}16.0$ 

$ r_{q\bar{q}}:1.54 $ ;

$ r_{\bar{q}\bar{q}}:1.60 $ ;

$ r_{b\bar{q}}:1.33 $ ;

$ r_{qb}:1.52 $ 

S: 17.9%; H: 27.8%; $ Di $ : 21.2%; K: 33.1%

Table 17. Compositeness of exotic resonances obtained in a complete coupled-channel calculation in the
$ 0(0^+) $ state of the$ \bar{q}q\bar{s}b $ tetraquark. The results are presented in the same manner as that in Table 5.Resonance Structure $6031+{\rm i}12.0$ 

$ r_{q\bar{q}}:1.85 $ ;

$ r_{\bar{q}\bar{q}}:1.87 $ ;

$ r_{b\bar{q}}:0.73 $ ;

$ r_{qb}:1.81 $ 

S: 21.3%; H: 10.1%; $ Di $ : 16.6%; K: 52.0%

$6298+{\rm i}16.0$ 

$ r_{q\bar{q}}:1.95 $ ;

$ r_{\bar{q}\bar{q}}:1.94 $ ;

$ r_{b\bar{q}}:0.91 $ ;

$ r_{qb}:1.86 $ 

S: 27.4%; H: 10.6%; $ Di $ : 10.2%; K: 51.8%

$6413+{\rm i}20.6$ 

$ r_{q\bar{q}}:1.22 $ ;

$ r_{\bar{q}\bar{q}}:1.55 $ ;

$ r_{b\bar{q}}:0.80 $ ;

$ r_{qb}:1.60 $ 

S: 16.6%; H: 17.3%; $ Di $ : 21.9%; K: 44.2%

$6607+{\rm i}4.2$ 

$ r_{q\bar{q}}:1.56 $ ;

$ r_{\bar{q}\bar{q}}:1.84 $ ;

$ r_{b\bar{q}}:1.70 $ ;

$ r_{qb}:1.75 $ 

S: 17.9%; H: 25.2%; $ Di $ : 12.6%; K: 44.3%

$6652+{\rm i}7.7$ 

$ r_{q\bar{q}}:1.61 $ ;

$ r_{\bar{q}\bar{q}}:1.93 $ ;

$ r_{b\bar{q}}:1.67 $ ;

$ r_{qb}:1.78 $ 

S: 14.8%; H: 20.6%; $ Di $ : 27.4%; K: 37.2%

Table 19. Compositeness of exotic resonances obtained in a complete coupled-channel calculation in the
$ 0(1^+) $ state of the$ \bar{q}q\bar{s}b $ tetraquark. The results are presented in the same manner as that in Table 5.Resonance Structure $6239+{\rm i}0.8$ 

$ r_{q\bar{q}}:1.45 $ ;

$ r_{\bar{q}\bar{q}}:1.50 $ ;

$ r_{b\bar{q}}:0.93 $ ;

$ r_{qb}:1.43 $ 

S: 19.1%; H: 10.0%; $ Di $ : 12.8%; K: 58.1%

$6314+{\rm i}3.5$ 

$ r_{q\bar{q}}:1.53 $ ;

$ r_{\bar{q}\bar{q}}:1.52 $ ;

$ r_{b\bar{q}}:0.80 $ ;

$ r_{qb}:1.44 $ 

S: 22.4%; H: 6.4%; $ Di $ : 3.5%; K: 67.7%

$6619+{\rm i}4.0$ 

$ r_{q\bar{q}}:1.42 $ ;

$ r_{\bar{q}\bar{q}}:1.44 $ ;

$ r_{b\bar{q}}:1.27 $ ;

$ r_{qb}:1.34 $ 

S: 17.3%; H: 25.6%; $ Di $ : 14.0%; K: 43.1%

$6664+{\rm i}6.9$ 

$ r_{q\bar{q}}:1.32 $ ;

$ r_{\bar{q}\bar{q}}:1.53 $ ;

$ r_{b\bar{q}}:1.34 $ ;

$ r_{qb}:1.41 $ 

S: 12.1%; H: 18.0%; $ Di $ : 32.2%; K: 37.7%

Table 21. Compositeness of exotic resonances obtained in a complete coupled-channel calculation in the
$ 0(2^+) $ state of the$ \bar{q}q\bar{s}b $ tetraquark. The results are presented in the same manner as that in Table 5.Resonance Structure $6080+{\rm i}2.5$ 

$ r_{q\bar{q}}:0.95 $ ;

$ r_{\bar{q}\bar{q}}:1.37 $ ;

$ r_{b\bar{q}}:1.24 $ ;

$ r_{qb}:1.27 $ 

S: 18.3%; H: 10.6%; $ Di $ : 12.8%; K: 58.3%

$6149+{\rm i}1.6$ 

$ r_{q\bar{q}}:1.09 $ ;

$ r_{\bar{q}\bar{q}}:1.13 $ ;

$ r_{b\bar{q}}:1.10 $ ;

$ r_{qb}:1.00 $ 

S: 18.5%; H: 10.5%; $ Di $ : 8.5%; K: 62.5%

Table 23. Compositeness of exotic resonances obtained in a complete coupled-channel calculation in the
$ 1(0^+) $ state of the$ \bar{q}q\bar{s}b $ tetraquark. The results are presented in the same manner as that in Table 5.Resonance Structure $5764+{\rm i}0.4$ 

$ r_{q\bar{q}}:0.89 $ ;

$ r_{\bar{q}\bar{q}}:0.89 $ ;

$ r_{b\bar{q}}:0.78 $ ;

$ r_{qb}:0.69 $ 

S: 29.6%; H: 2.4%; $ Di $ : 9.2%; K: 58.8%

$6103+{\rm i}10.3$ 

$ r_{q\bar{q}}:0.93 $ ;

$ r_{\bar{q}\bar{q}}:1.73 $ ;

$ r_{b\bar{q}}:1.58 $ ;

$ r_{qb}:1.66 $ 

S: 17.6%; H: 13.1%; $ Di $ : 23.2%; K: 46.1%

$6308+{\rm i}8.4$ 

$ r_{q\bar{q}}:1.17 $ ;

$ r_{\bar{q}\bar{q}}:1.65 $ ;

$ r_{b\bar{q}}:1.40 $ ;

$ r_{qb}:1.37 $ 

S: 11.4%; H: 6.1%; $ Di $ : 11.7%; K: 70.8%

$6413+{\rm i}3.8$ 

$ r_{q\bar{q}}:1.50 $ ;

$ r_{\bar{q}\bar{q}}:1.63 $ ;

$ r_{b\bar{q}}:1.55 $ ;

$ r_{qb}:1.40 $ 

S: 15.6%; H: 19.8%; $ Di $ : 27.3%; K: 37.3%

Table 25. Compositeness of exotic resonances obtained in a complete coupled-channel calculation in the
$ 1(1^+) $ state of the$ \bar{q}q\bar{s}b $ tetraquark. The results are presented in the same manner as that in Table 5.$ \bar{q}q\bar{s}c $ tetraquarks

$ I(J^P) $ 

Dominant Component Theoretical resonance $ 0(0^+) $ 

$ \omega D^*_s (10{\text{%}})+K^* D^*(11{\text{%}})+Di(13{\text{%}})+K(57{\text{%}}) $ 

$3006+{\rm i}6.3$ 

$ 0(1^+) $ 

$ H(19{\text{%}})+Di(30{\text{%}})+K(43{\text{%}}) $ 

$3119+{\rm i}19.8$ 

$ Di(35{\text{%}})+K(42{\text{%}}) $ 

$3292+{\rm i}13.1$ 

$ Di(35{\text{%}})+K(50{\text{%}}) $ 

$ 3346+{\rm i}22.0 $ 

Continued on next page Table 28. Summary of resonance structures found in
$ \bar{q}q\bar{s}Q \;\; (q=u,\,d;\,Q=c,\,b) $ tetraquark systems. The first column shows the isospin, total spin, and parity of each singularity. The second column refers to the dominant configuration components; H: hidden color,$ Di $ : diquark-antidiquark, and K: K-type. Theoretical resonances are presented with the notation$E=M+{\rm i}\Gamma$ in the last column (unit: MeV).To explain our delicate compromise between obtaining reliable results and reasonable times of computation, let us explain our method of obtaining results when we perform a fully-coupled channel computation using the CSM. First, the bound, resonance, and scattering states are complex values distributed in a two dimensional plane, we can observe in the figures below that rotations from
$ 0^\circ $ to$ 6^\circ $ enable us to reasonably distinguish all bound, resonance, and scattering states when the imaginary part of the complex energy is lower than$ \approx15\;\text{MeV} $ . Second, regardless of how clear the figure may be, we follow the behavior of each energy point with respect to the rotated angle from zero to six degrees; thus, we can distinguish wether they belong to a particular meson-meson threshold or can be something else. In the second case, we perform some extra internal assessments before claiming that the state may be a resonance (there is no doubt when an energy point is a bound state because it is localized in the real axis and does not move with respect the angle). Third, we require a black-circle ($ 2^\circ $ ), red triangle ($ 4^\circ $ ), and blue square ($ 6^\circ $ ) leaving the meson-meson lines and forming a cluster to attract our attention; subsequently, we make some extra assessments related with the evolution of the energy point with respect the theta parameter and its connection with the$ 0^\circ $ case to finally claim that it is actually a resonance.Now, we describe in detail our theoretical findings for each sector of
$ \bar{q}q\bar{s}Q $ tetraquarks. -
Several resonances whose masses range from
$ 2.8 $ to$ 3.5 $ GeV are obtained in this tetraquark sector. Each iso-scalar and -vector sectors with total spin and parity$ J^P=0^+ $ ,$ 1^+ $ , and$ 2^+ $ are discussed individually below.The
$ \mathit{\boldsymbol{I(J^P)=0(0^+)}} $ sector: Four meson-meson configurations,$ \eta D_s $ ,$ \omega D^*_s $ ,$ K D $ and$ K^* D^* $ in both color-singlet and -octet channels, four diquark-antidiquark structures, along with three K-type configurations are individually calculated in Table 4. The lowest channel is the color-singlet state of$ K D $ , whose theoretical mass is$ 2378 $ MeV, the other three meson-meson configurations with the same color channel are in an energy region of$ 2.6 $ to$ 3.0 $ GeV. No bound state is found. Additionally, the single channel calculations are performed in each exotic structure. The hidden-color channels of di-meson configurations are generally located in the$ 3.1-3.3 $ GeV interval. This result also holds for the diquark-antidiquark and K-type structures, although the lowest masses of$ (qc)(\bar{q}\bar{s}) $ and$ K_3 $ -type channels are$ 2.9 $ GeV.After partially coupled-channel computations are performed in six configurations listed in Table 4, only the scattering state of
$ K D $ and several color resonances, which masses are in an energy region of$ 2.7-2.9 $ GeV, are obtained. Meanwhile, the lowest-lying mass of$ 2378 $ MeV for a$ K D $ scattering state remains even in the complete coupled-channel calculation.Figure 2 presents the distribution of complex energies for the
$ \bar{q}q\bar{s}c $ tetraquark in the$ 0(0^+) $ channel calculated using the CSM for fully coupled channels. In particular, within a mass region of$ 2.35 $ to$ 3.35 $ GeV, five scattering states that include the ground states of$ K D $ ,$ \eta D_s $ ,$ \omega D^*_s $ ,$ K^* D^* $ , and the radial excitation of$ K(1S) D(2S) $ are well presented. However, in addition to the vast majority of scattering dots, one stable resonance pole is found and circled. The mass and width are$ 3006 $ and$ 6.3 $ MeV, respectively.Table 5 shows the compositeness of the resonance state. First, it is a loosely-bound structure with quark-antiquark distances of
$ \sim 1.6 $ and$ \sim 2.2 $ fm for the$ qc $ and$ \bar{q}\bar{q} $ pairs, respectively. Moreover, a strong coupling exists among singlet-, hidden-color, diquark-antidiquark, and K-type channels. The golden decays for this resonance are$ \omega D^*_s $ and$ K^* D^* $ , which are the dominant components (21.3%) of the color-singlet channels.The
$ \mathit{\boldsymbol{I(J^P)=0(1^+)}} $ sector: 36 channels contribute to this case, and results in real-range calculations are listed in Table 6. First, the lowest mass,$ 2498 $ MeV, in a single channel computation is the theoretical threshold value of$ K D^* $ . The other di-meson channels, which include$ \eta D^*_s $ ,$ \omega D^{(*)}_s $ , and$ K^* D^{(*)} $ , are generally located in$ 2.7-2.9 $ GeV. All these states have a scattering nature. Six channels are also included in each exotic configuration. By referencing the calculated data on each channel, we find that the lowest masses in the hidden-color, diquark-antidiquark, and K-type configurations are all within$ 3.0-3.3 $ GeV. Furthermore, color resonances with structures of diquark-antidiquark and$ K_3 $ -type are still obtained at approximately$ 2.95 $ GeV.In a further step, the lowest coupled-channel masses within each considered configuration are
$ 2.50 $ ,$ 2.90 $ ,$ 2.85 $ ,$ 3.00 $ ,$ 2.96 $ , and$ 2.79 $ GeV, respectively. These results indicate that the coupling effect is significantly weak in color-singlet channels, but it becomes stronger in other configurations. However, the bound state is still unavailable even in a complete coupled-channel scenario.To find a possible resonance state in an excited energy region of
$ 2.5-3.4 $ GeV, the fully coupled-channel calculation is further performed using the CSM, and results are plotted in a complex energy plane in Fig. 3. Therein, seven meson-meson scattering states are generally presented. They are ground states of$ K^{(*)} D^{(*)} $ ,$ \omega D^{(*)}_s $ , and$ \eta D^*_s $ and the first radial excited state of$ K(1S) D^*(2S) $ . However, three stable poles are obtained within the radial excited energy region, and their complex energies are$3119+{\rm i}19.8$ ,$3292+{\rm i}13.1$ , and$3346+{\rm i}22$ MeV, respectively.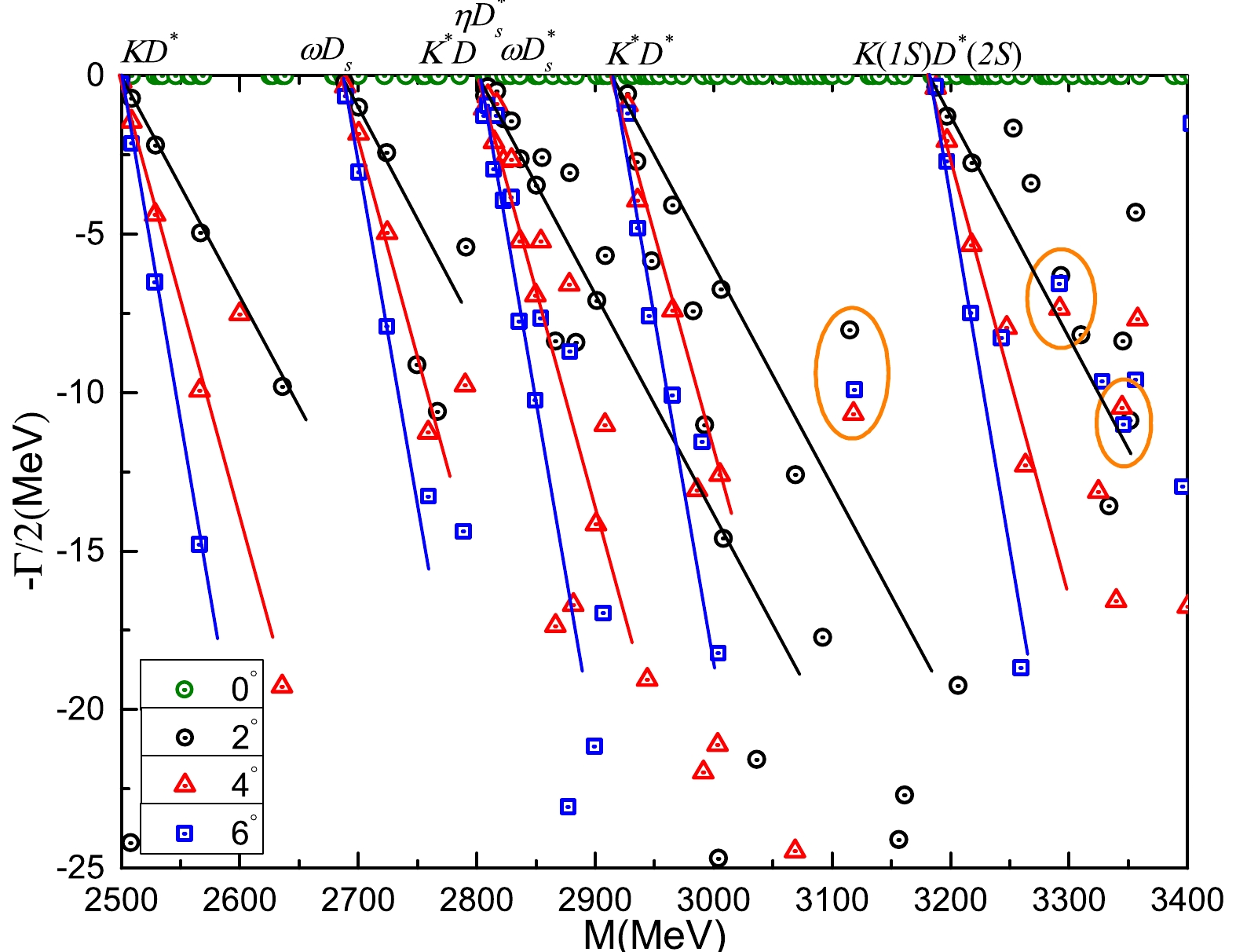
Figure 3. (color online) Complete coupled-channel calculation of the
$ \bar{q}q\bar{s}c $ tetraquark system with$ I(J^P)=0(1^+) $ .Table 7 shows particular features of the three resonances. First, their dominant components are all of exotic color structure,
$ i. e. $ , the hidden-color, diquark-antidiquark, and K-type configurations. Moreover, the coupling among these three sectors is strong. The color resonances are also confirmed by calculating their sizes, with internal quark distances of about$ 1.1-1.7 $ fm. These resonances are expected to be experimentally studied in the$ K^{(*)} D^{(*)} $ golden decay channels.The
$ \mathit{\boldsymbol{I(J^P)=0(2^+)}} $ state: Two meson-meson channels,$ \omega D^*_s $ and$ K^* D^* $ , should be considered in the highest spin state. Table 8 shows that their lowest masses are simply the theoretical threshold values; hence, no bound state is found. Meanwhile, ten other channels of exotic structures are generally located in a mass region of$ 3.1-3.2 $ GeV. When coupled-channel calculations are performed in each specific configuration, color resonances are located at$ \sim 3.1 $ GeV, and the scattering state of$ \omega D^*_s $ , which is the lowest-lying channel, remains at$ 2.8 $ GeV. This extremely weak coupling effect also holds for the complete coupled-channel study.Nevertheless, three narrow resonances are obtained in a complex analysis on the fully coupled-channel computation. Figure 4 shows the two scattering states of
$ \omega D^*_s $ and$ K^* D^* $ within$ 2.8-3.4 $ GeV. Moreover, three stable poles against the descending cut lines occur when the rotated angle is varied from$ 0^\circ $ to$ 6^\circ $ .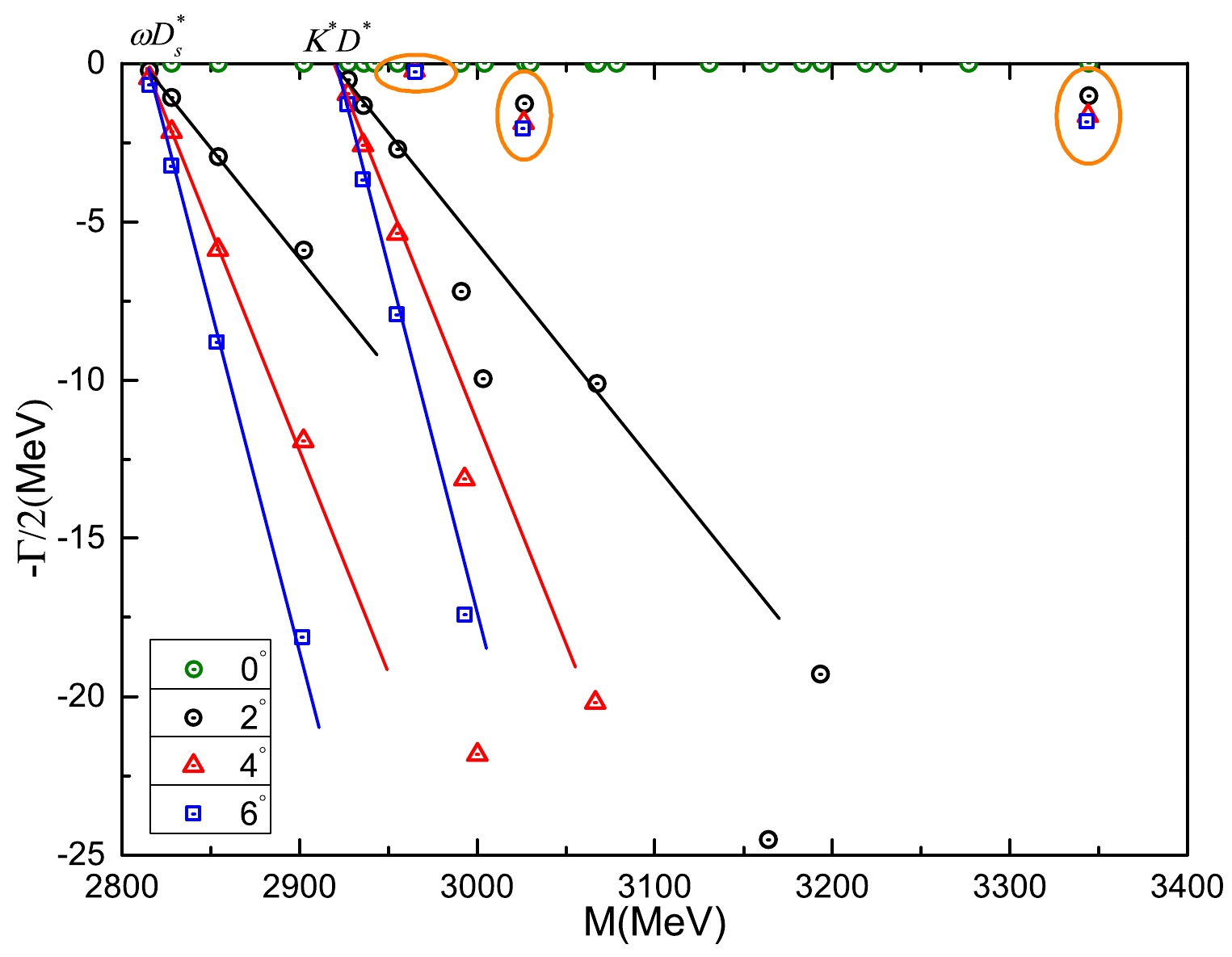
Figure 4. (color online) Complete coupled-channel calculation of the
$ \bar{q}q\bar{s}c $ tetraquark system with$ I(J^P)=0(2^+) $ quantum numbers.The naturalness of these narrow resonances can be inferred from Table 9. In particular, the complex energies of these resonances are
$2965+{\rm i}0.5$ ,$3026+{\rm i}3.8$ , and$3344+{\rm i}3.3$ MeV, respectively. Compact structures are dominant when referring to their interquark sizes, which are about$ 1.4 $ fm. Furthermore, strong couplings exist among the color-singlet, hidden-color, diquark-antidiquark, and K-type configurations of these states. Because the singlet-color component are$ (\sim 7\%) $ of$ \omega D^*_s $ and$ K^* D^* $ for these resonances, they can be experimentally investigated in any of the mentioned two-body strong decay processes.The
$ \mathit{\boldsymbol{I(J^P)=1(0^+)}} $ sector: This case is similar to the$ 0(0^+) $ channel, i.e., 24 channels are investigated, as shown in Table 10. First,$ \pi D_s $ ,$ \rho D^*_s $ ,$ K D $ , and$ K^* D^* $ channels in both singlet- and hidden-color configurations are calculated. The lowest-lying state is the$ \pi D_s $ scattering state with a theoretical threshold value of$ 2138 $ MeV. Moreover, the other three meson-meson structures in color-singlet channels are also unbound, and the four hidden-color channels are generally located at$ 3.17 $ GeV. For the single channel computations of diquark-antidiquark and K-type configurations, the lowest masses are generally distributed within an energy region of$ 2.9-3.2 $ GeV, except for a$ K_1 $ -type channel with mass at$ 2475 $ MeV.We do not find bound states when partially and fully coupled-channel calculations, in the real-range approximation, are performed. The complete coupled-channels study, in the complex range formulation, delivers six continuum states of
$ \pi D_s $ ,$ K^{(*)} D^{(*)} $ ,$ \rho D^*_s $ ,$ \pi(1S) D_s(2S) $ , and$ K(1S) D(2S) $ , which are clearly presented in the$ 2.1-3.2 $ GeV energy region of Fig. 5. Additionally, one stable resonance is obtained and circled in Fig. 5; its complex energy is predicted to be$2.8+{\rm i}0.002$ GeV. This state should be tentatively assigned to the experimentally observed$ T_{c\bar{s}}(2900) $ state [3, 4]; however, its theoretical width is smaller than the experimental one. Thus, we cannot be optimistic in the assignment. Note that only two-body strong decay processes are considered, and the other channels not considered herein must contribute to its total decay width.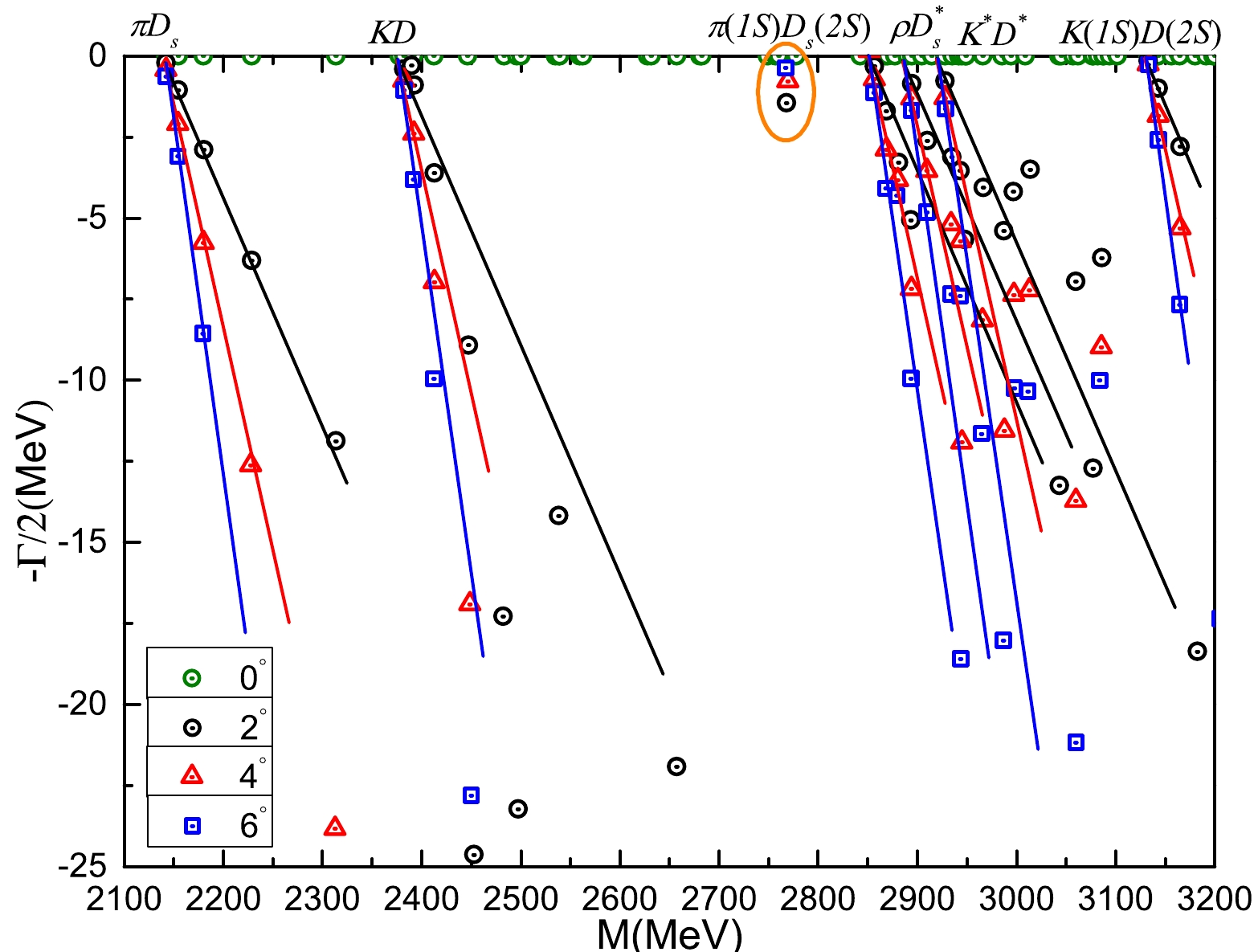
Figure 5. (color online) Complete coupled-channel calculation of the
$ \bar{q}q\bar{s}c $ tetraquark system with$ I(J^P)=1(0^+) $ quantum numbers.Table 11 lists the interquark distances and wavefunction components of the predicted exotic resonance. Its size is approximately
$ 1.6 $ fm, and a strong coupling exists among the four considered configurations, i.e., the color-singlet, hidden-color, diquark-antidiquark, and K-type structures. The color-singlet channels of$ \pi D_s $ and$ K D $ are comparable (~8%); hence, they are suggested to be the golden decay channels.The
$ \mathit{\boldsymbol{I(J^P)=1(1^+)}} $ sector: Among the six channels of the considered$ \bar{q}q\bar{s}c $ tetraquark configurations, which include singlet-, hidden- color, diquark-antidiquark, and K-type structures, the lowest-lying state is the$ \pi D^*_s $ scattering state with a theoretical threshold at$ 2264 $ MeV. Meanwhile, this unbound nature remains unchanged in coupled-channel computations. The other five di-meson configurations in the color-singlet channels, which are$ \rho D^{(*)}_s $ and$ K^{(*)} D^{(*)} $ , are also unbound. The masses of exotic color channels are generally located in the energy region of$ 2.9-3.2 $ GeV, except for a$ K_1 $ -type channel whose calculated mass is$ 2518 $ MeV. When coupled-channel calculations are performed in each of these structures, the lowest masses of hidden-color, diquark-antidiquark,$ K_1 $ ,$ K_2 $ , and$ K_3 $ channels are$ 2951 $ ,$ 2871 $ ,$ 2509 $ ,$ 2972 $ , and$ 2791 $ MeV, respectively. Although bound states are unavailable, the mentioned excited states obtained in each exotic color configuration may be good candidates of color resonances for the$ \bar{q}q\bar{s}c $ tetraquark system.Furthermore, Fig. 6 shows the distribution of complex energies in the fully coupled-channel calculation using the CSM. In particular, the top panel presents six scattering states, which have been discussed above. Within
$ 2.25-3.15 $ GeV, two stable poles are obtained, and they are indicated within circles. The lower resonance pole is at$2470+{\rm i}1$ MeV, whereas the higher one is at$3134+{\rm i}2$ MeV. Finally, the bottom panel of Fig. 6 is an enlarged part of$ 2.85-3.00 $ GeV. Therein, no stable resonance is found, and only three scattering states of$ \rho D^*_s $ ,$\pi(1S) D^*_s(2S)$ , and$ K^* D^* $ are presented.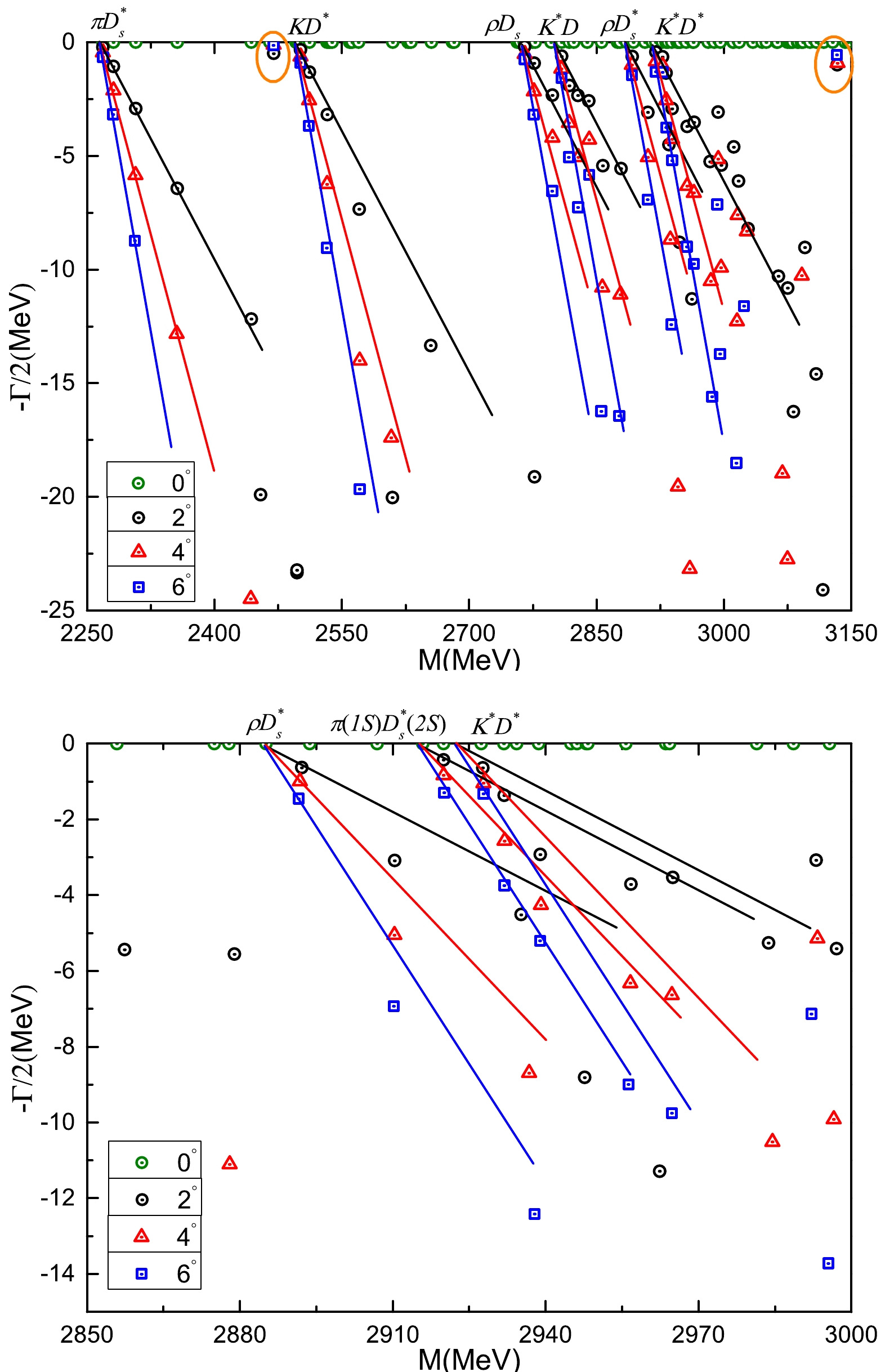
Figure 6. (color online) Complete coupled-channel calculation of the
$ \bar{q}q\bar{s}c $ tetraquark system with$ I(J^P)=1(1^+) $ quantum numbers. In particular, the bottom panel shows enlarged parts of dense energy region from$ 2.85\,\text{GeV} $ to$ 3.00\,\text{GeV} $ .Some insights into the nature of the narrow resonances can be found in Table 13. In particular, a compact
$ \bar{q}q\bar{s}c $ tetraquark structure is predicted for the lower resonance, and its size is about$ 0.9 $ fm. The coupling is strong among color-singlet (27%), diquark-antidiquark (14%), and K-type (56%) channels. Moreover, the dominant meson-meson decay channel is$ \pi D^*_s $ , which is expected to be confirmed in future experiments. In contrast, the higher resonance is a loosely-bound structure with a size of$ \sim 1.8 $ fm. The ratios between the components are similar to those at a lower resonance. This suggests that this state should be further studied using high energy experiments in the$ \pi D^*_s $ and$ K^* D^* $ decay channels.The
$ \mathit{\boldsymbol{I(J^P)=1(2^+)}} $ sector: Twelve channels are considered in the highest spin and isospin tetraquark state, and our results are listed in Table 14. First, the bound states are not found in either single-channel or coupled-channel cases. The lowest channel is$ \rho D^*_s $ with a theoretical threshold value of$ 2887 $ MeV, and another di-meson structure$ K^* D^* $ in the color-singlet channel is at$ 2924 $ MeV. The masses of other channels with exotic configurations are generally in an energy region of$ 3.1-3.2 $ GeV, and each of the lowest coupled mass in one specific structure is$ \sim 3.16 $ GeV.The complete coupled-channel calculation using CSM is shown in Fig. 7. In particular, two scattering states of
$ \rho D^*_s $ and$ K^* D^* $ are well presented within$ 2.8-3.5 $ GeV. However, four stable poles occur above the threshold lines. Table 15 shows the resonance masses, widths, and wavefunction configurations. Moreover, we can infer the compact tetraquark structure, with a size of approximately$ 1.0-1.6 $ fm, for the four resonances at$3031+{\rm i}0.7$ ,$3105+{\rm i}3.7$ ,$3373+{\rm i}4.2$ , and$3455+{\rm i}12.9$ MeV, respectively. Additionally, strong couplings exist among the singlet-, hidden-color, diquark-antidiquark, and K-type channels for these resonances. Both$ \rho D^*_s $ and$ K^* D^* $ appear to be golden decay channels. -
Three spin-parity states,
$ J^P=0^+ $ ,$ 1^+ $ , and$ 2^+ $ , with isospins of$ I=0 $ and$ 1 $ , are investigated for the$ \bar{q}q\bar{s}b $ tetraquark system. Several narrow resonances are obtained in each$ I(J^P) $ quantum number. The details of the calculation and the related discussion are presented in the following.The
$ \mathit{\boldsymbol{I(J^P)=0(0^+)}} $ sector: Table 16 shows that 24 channels are investigated in this case. First, concerning the four color-singlet channels, which include$ \eta B_s $ ,$ \omega B^*_s $ ,$ K B $ , and$ K^* B^* $ , the lowest mass is$ 5759 $ MeV. This is the theoretical threshold value of$ K B $ , and the other channels are also unbound. Furthermore, the lowest-lying channels of hidden-color, diquark-antidiquark, and K-type configurations are generally located in a mass region of$ 6.2-6.6 $ GeV. When coupled-channel computations are performed for each type of structure, the scattering nature of$ K B $ channel in di-meson structure is still obtained. Moreover, possible color resonances in$ K_3 $ and diquark-antidiquark structures are obtained at$ 6.04 $ and$ 6.11 $ GeV, respectively. The lowest masses of other three configurations are$ \sim 6.2 $ GeV. Finally, in a real-range computation, which is performed by including all of the above channels, the lowest mass of the$ \bar{q}q\bar{s}b $ tetraquark system remains at the$ K B $ theoretical threshold,$ 5759 $ MeV.In the next step, the complete coupled-channel case is studied in a complex-range formulation. Figure 8 shows the distribution of complex energies within
$ 5.7-6.7 $ GeV. In particular, five scattering states, which are the ground states of$ K B $ ,$ \eta B_s $ ,$ \omega B^*_s $ , and$ K^* B^* $ , and the first radial excitation of$ K(1S) B(2S) $ , are well presented. Nevertheless, five resonances are also found and circled in Fig. 8; their complex energies are$6011+{\rm i}9$ ,$6323+{\rm i}4$ ,$6397+{\rm i}13.8$ ,$6643+{\rm i}23.4$ , and$6678+{\rm i}16$ MeV, respectively.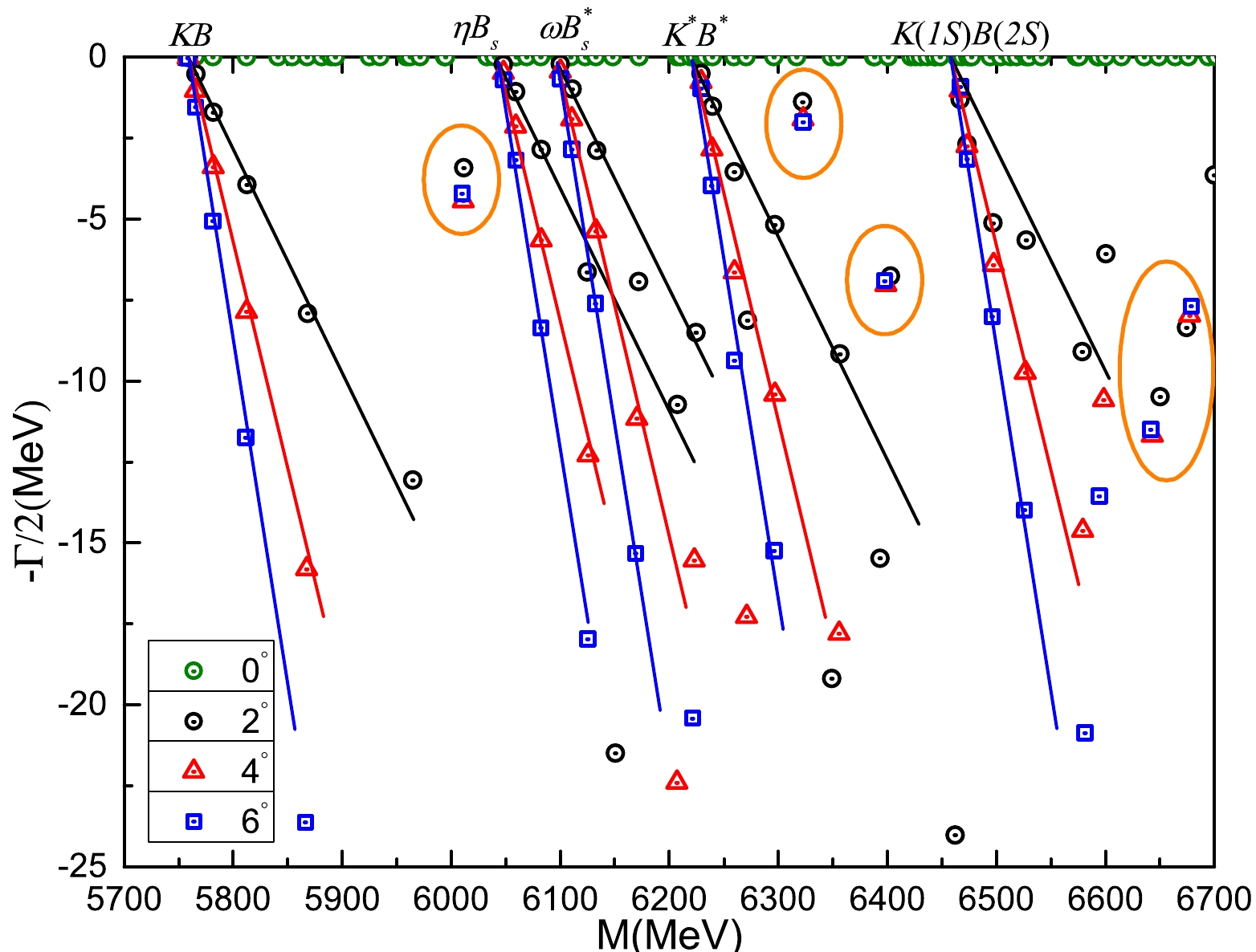
Figure 8. (color online) Complete coupled-channel calculation of the
$ \bar{q}q\bar{s}b $ tetraquark system with$ I(J^P)=0(0^+) $ quantum numbers.The details of the properties of such resonances are listed in Table 17. First, strong couplings exist among the singlet-, hidden-color, diquark-antidiquark, and K-type channels. Second, their sizes are less than
$ 1.9 $ fm. In particular, the two lower resonances have sizes within$ 1.1-1.5 $ fm, and the other three are extended to approximately$ 1.3-1.8 $ fm. Third, for the lowest resonance at$ 6.0 $ GeV, the golden decay channel is the$ K B $ channel; the$ \omega B^*_s $ and$ K^* B^* $ channels are dominant meson-meson components for the two resonances at$ 6.3 $ GeV, whereas the remaining two resonances at$ 6.6 $ GeV can be confirmed in$ K B $ and$ K^* B^* $ channels.The
$ \mathit{\boldsymbol{I(J^P)=0(1^+)}} $ sector: Table 18 summarizes our results in the real-range formalism. First, the$ \eta B^*_s $ ,$ \omega B^{(*)}_s $ , and$ K^{(*)} B^{(*)} $ channels in both color-singlet and -octet cases are considered. The lowest mass is$ 5.8 $ GeV, which is the$ K B^* $ theoretical threshold value. Moreover, no bound states in the other meson-meson channels are found, and the six hidden-color channels are generally located within the energy range of$ 6.3-6.6 $ GeV. This energy region is also shared by the diquark-antidiquark and three K-type channels. In partially coupled-channel calculations, the coupling effect is weak in singlet- and hidden-color channels, their lowest masses are$ 5.80 $ and$ 6.32 $ GeV, respectively. Therefore, the bound state is still unavailable. Although strong couplings exist in diquark-antidiquark and three K-type channels, and the mass shift for the lowest-lying channel is$ 50-170 $ MeV, the bound state is again not obtained. This result also holds for the fully coupled-channel calculation.In a further complex analysis of the complete coupled-channel, five resonances are obtained, and they are indicated in Fig. 9. We can observe, in addition to the seven scattering states of
$ K^{(*)} B^{(*)} $ ,$ \omega B^{(*)}_s $ , and$ \eta B^*_s $ in the energy region of$ 5.8-6.7 $ GeV, the five stable poles circled, with complex energies given by$6031+{\rm i}12$ ,$6298+{\rm i}16$ ,$6413+{\rm i}20.6$ ,$6607+{\rm i}4.2$ , and$6652+{\rm i}7.7$ MeV, respectively.
Figure 9. (color online) Complete coupled-channel calculation of the
$ \bar{q}q\bar{s}b $ tetraquark system with$ I(J^P)=0(1^+) $ quantum numbers.Table 19 lists the calculated properties of the resonances to elucidate their nature. First, the strong coupling effects of different tetraquark configurations are reflected. Meanwhile, these resonances have sizes of about
$ 1.6-1.9 $ fm, except for the one at$ 6.4 $ GeV, whose size is less than$ 1.6 $ fm. Finally, the lowest resonance at$ 6.03 $ GeV is suggested to be experimentally studied in the$ K B^* $ decay channel. The$ K^* B $ and$ K^* B^* $ are dominant two-body strong decay channels for the$ 6.29 $ and$ 6.41 $ GeV resonances, whereas the$ K B^* $ is the golden channel for the remaining two resonances at$ 6.6 $ GeV.The
$ \mathit{\boldsymbol{I(J^P)=0(2^+)}} $ sector:$ \omega B^*_s $ and$ K^* B^* $ in both color-singlet and hidden-color channels, along with two diquark-antidiquark, and six K-type channels are considered in this case. First, the bound state is not found in single- and coupled-channel computations. The lowest-lying channel is the$ \omega B^*_s $ scattering state. Additionally, other channels with exotic color structures are located in$ 6.36-6.52 $ GeV. When a coupled-channel calculation is performed in each specific configuration, their lowest mass is$ \sim 6.4 $ GeV.Furthermore, Fig. 10 presents results in the fully coupled-channel case using the CSM. Therein, scattering states of
$ \omega B^*_s $ ,$ K^* B^* $ , and$ \omega(1S) B^*_s(2S) $ are fully shown; additionally, four narrow resonances are also found. Table 21 presents their resonance parameters:$6239+{\rm i}0.8$ ,$6314+{\rm i}3.5$ ,$6619+{\rm i}4$ , and$6664+{\rm i}6.9$ MeV, respectively. Moreover, the size of these four resonances is approximately$ 1.4 $ fm; the proportions of singlet-, hidden-color, diquark-antidiquark, and K-type channels are comparable. They can be further confirmed experimentally in$ \omega B^*_s $ and$ K^* B^* $ channels.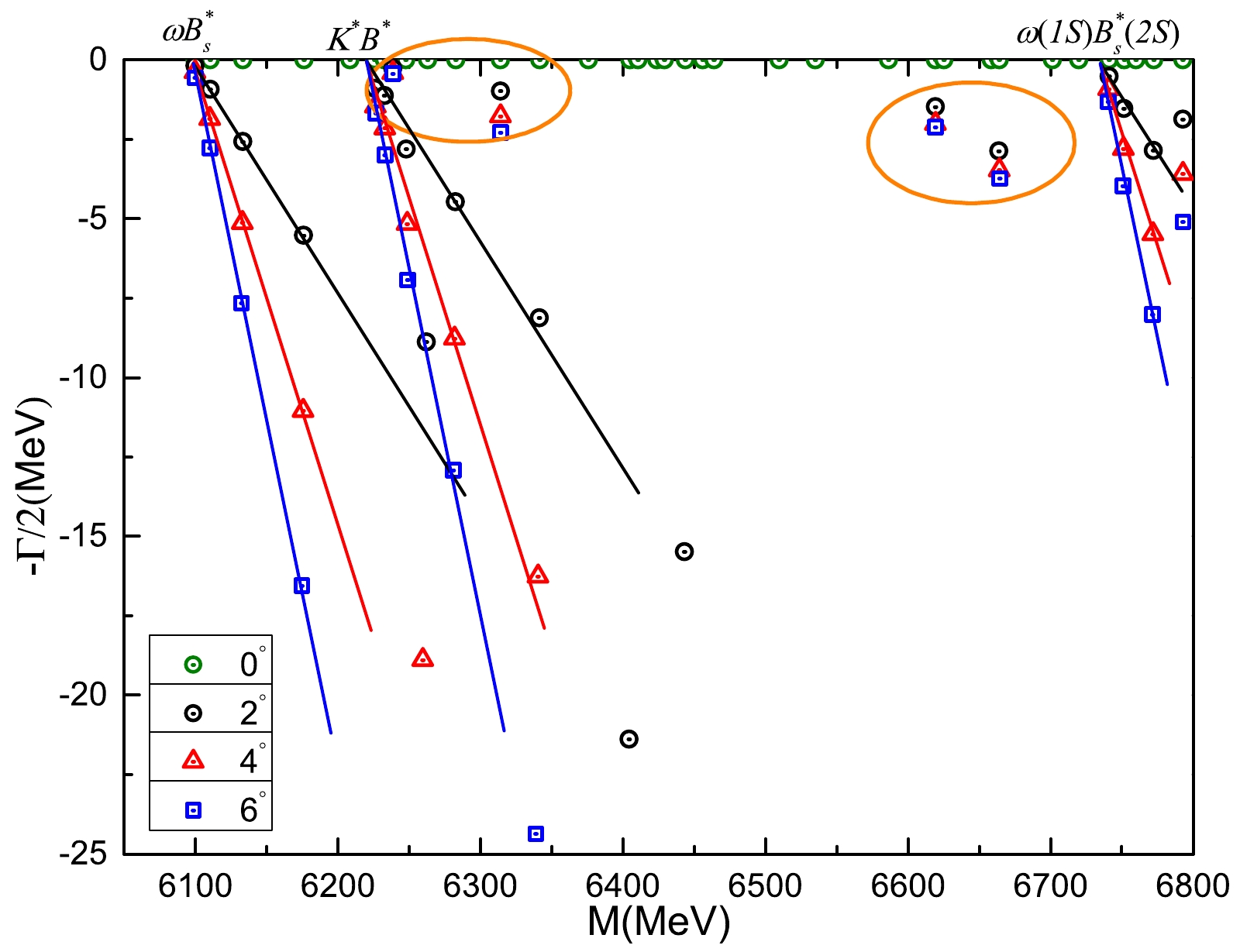
Figure 10. (color online) Complete coupled-channel calculation of the
$ \bar{q}q\bar{s}b $ tetraquark system with$ I(J^P)=0(2^+) $ quantum numbers.The
$ \mathit{\boldsymbol{I(J^P)=1(0^+)}} $ sector: First, all channels listed in Table 22 are investigated in the real-range computations, and no bound state is obtained. The lowest-lying scattering state is$ \pi B_s $ with a theoretical threshold value of$ 5504 $ MeV. The other three di-meson scattering states are$ \rho B^*_s $ ,$ K B $ , and$ K^* B^* $ . Furthermore, the masses of these four meson-meson structures in hidden-color channels are approximately$ 6.4 $ GeV; the values are similar for the$ K_1 $ channels, except for one at$ 5.79 $ GeV. For the diquark-antidiquark,$ K_2 $ , and$ K_3 $ channels, they are generally located at$ 6.2-6.5 $ GeV. In coupled-channel studies, which include six partial and one complete channels calculations, strong and weak coupling effects are both presented. In particular, channel couplings in the hidden-color, diquark-antidiquark,$ K_2 $ , and$ K_3 $ configurations are strong, and they exhibit$ 100-170 $ MeV mass shifts. Their lowest masses are$ 6.24 $ ,$ 6.12 $ ,$ 6.20 $ , and$ 6.03 $ GeV, respectively. However, the coupling is weak in color-singlet,$ K_1 $ , and fully-coupled channels calculations. Accordingly, the scattering nature of$ \pi B_s $ remains unchanged.In a further step, the complex-range study is conducted on the complete coupled-channel case. Six scattering states are plotted in Fig. 11, and they are the ground states of
$ \pi B_s $ ,$ K B $ ,$ \rho B^*_s $ , and$ K^* B^* $ and the first radial excitations of$ \pi(1S) B_s(2S) $ and$ K(1S) B(2S) $ . Moreover, within an energy region of$ 5.5-6.5 $ GeV, two narrow resonance poles are obtained.
Figure 11. (color online) Complete coupled-channel calculation of the
$ \bar{q}q\bar{s}b $ tetraquark system with$ I(J^P)=1(0^+) $ quantum numbers.The compositeness of these resonances is listed in Table 23. First, the complex energies of the two resonances are expressed as
$6080+{\rm i}2.5$ and$6149+{\rm i}1.6$ MeV, respectively. Meanwhile, they are compact$ \bar{q}q\bar{s}b $ tetraquark structures, whose sizes are$ \sim 1.2 $ fm. The couplings among color-singlet, -octet, diquark-antidiquark, and K-type channels are strong. Both$ \pi B_s $ and$ K B $ are their golden channels to be discovered.The
$ \mathit{\boldsymbol{I(J^P)=1(1^+)}} $ sector: Table 24 lists 36 channels under consideration for this quantum state. In the meson-meson color-singlet channels,$ \pi B^*_s $ ,$ \rho B^{(*)}_s $ , and$ K^{(*)} B^{(*)} $ are calculated. The lowest channel is the scattering state of$ \pi B^*_s $ , and its mass is a theoretical threshold value of$ 5549 $ MeV. Moreover, the scattering nature of$ \pi B^*_s $ channel remains in partially and fully coupled-channel calculations. Other channels are also unbound. The channels in the other five structures, which are hidden-color, diquark-antidiquark, and K-types configurations, are generally located in an energy region of$ 6.2-6.5 $ GeV, except for a$ K_1 $ channel at$ 5.8 $ GeV. Additionally, when coupled-channel calculations are considered in each of these five structures, a weak coupling effect is obtained in$ K_1 $ channels, and the lowest coupled mass is still$ 5.8 $ GeV. In contrast, strong coupling effects exist in other configurations. Nevertheless, they are still unstable excited states within$ 6.06-6.28 $ GeV.In the complete coupled-channel computation using CSM, the distribution of complex energies is plotted in Fig. 12. Particularly, within an energy region of
$5.55- 6.45$ GeV in the top panel of Fig. 12, the scattering states of$ \pi B^*_s $ ,$ K B^* $ ,$ \rho B_s $ , and$ K^* B^* $ are well presented. Furthermore, dense distributions of energy dots occur at approximately$ 6.2 $ GeV; hence, an enlarged part from$ 6.12 $ to$ 6.22 $ GeV is plotted in the bottom panel. Therein, four scattering states of$ \rho B^{(*)}_s $ ,$ K^* B $ , and$ \pi(1S) B^*_s(2S) $ are shown.
Figure 12. (color online) Top panel: Complete coupled-channel calculation of the
$ \bar{q}q\bar{s}b $ tetraquark system with$ I(J^P)=1(1^+) $ quantum numbers. In particular, the bottom panel shows enlarged parts of dense energy region from$ 6.12\,\text{GeV} $ to$ 6.22\,\text{GeV} $ .In addition to the obtained continuum states, four resonances are found in complex plane. Table 25 summarizes their calculated results. First, the four stable poles are
$5764+{\rm i}0.4$ ,$6103+{\rm i}10.3$ ,$6308+{\rm i}8.4$ , and$6413+{\rm i}3.8$ MeV, respectively. Moreover, color-singlet, diquark-antidiquark, and K-type channels couplings are strong for the resonances. A compact structure, with a size of approximately$ 0.8 $ fm, is obtained for the lowest resonance at$ 5.76 $ GeV, and the dominant meson-meson component is$ \pi B^*_s $ . However, the other three resonances are loose structures with a size of$ \sim 1.6 $ fm. The golden channels of the second resonance at$ 6.1 $ GeV are$ \pi B^*_s $ and$ K B^* $ , whereas$ \pi B^*_s $ ,$ K^* B $ , and$ K^* B^* $ channels are suggested to be the dominant di-meson components for the other two higher resonances at$ 6.3 $ and$ 6.4 $ GeV, respectively.The
$ \mathit{\boldsymbol{I(J^P)=1(2^+)}} $ sector: Twelve channels listed in Table 26 are studied for the highest spin and isospin state of the$ \bar{q}q\bar{s}b $ tetraquark. First, the bound states are not found in three types of calculations: single, partially coupled, and fully coupled-channels. The lowest scattering state is$ \rho B^*_s $ , with a theoretical threshold value of$ 6172 $ MeV; another one is the$ 6226 $ MeV threshold value of the$ K^* B^* $ channel. Furthermore, the masses of channels in five exotic color structures are generally located in the energy region of$ 6.4-6.5 $ GeV. The lowest mass obtained within a coupled-channel calculation for each specific configuration is always located at$ \sim 6.45 $ GeV.Figure 13 shows the distribution of complex energies in the complete coupled-channel computation using the CSM. Within the
$ 6.15-6.80 $ GeV energy region, the$ \rho B^*_s $ and$ K^* B^* $ scattering states are clearly presented. Meanwhile, four stable resonance poles are also obtained, and they are circled in the complex plane.Table 27 lists the properties of these resonances. In particular, their complex energies are
$6301+{\rm i}0.8$ ,$6399+ {\rm i}2.6$ ,$6654+{\rm i}5.3$ , and$6740+{\rm i}10$ MeV, respectively. The couplings among color-singlet, -octet, diquark-antidiquark, and K-type channels are strong for the first three resonances. However, only a strong coupling exists between diquark-antidiquark and K-type channels for the highest resonance. Moreover, the compact$ \bar{q}q\bar{s}b $ tetraquark structure is obtained for the four resonances because their sizes are less than$ 1.5 $ fm. Finally, both$ \rho B^*_s $ and$ K^* B^* $ are the dominant meson-meson components of these exotic states. -
The S-wave
$ \bar{q}q\bar{s}Q \;\; (q=u,\,d;\,Q=c,\,b) $ tetraquarks with spin-parities of$ J^P=0^+ $ ,$ 1^+ $ , and$ 2^+ $ and isospins of$ I=0 $ and$ 1 $ are systematically investigated under a chiral quark model formalism. Furthermore, the color-singlet, -octet meson-meson configurations, diquark-antidiquark arrangements with their allowed color triplet-antitriplet and sextet-antisextet channels, and K-type configurations are considered. The four-body bound and resonant states are determined using a highly efficient numerical approach: the Gaussian expansion method (GEM) supplemented with a complex-scaling analysis (CSM). Three types of computations are generally presented: single, partially-coupled, and fully-coupled channels.Table 28 summarizes our theoretical findings for the
$ \bar{q}q\bar{s}c $ and$ \bar{q}q\bar{s}b $ tetraquark systems. The first column shows the quantum numbers$ I(J^P) $ , the second one expresses the dominant configurations, and the third one lists the complex eigenenergies.Table 28-continued from previous page $ 0(2^+) $ 

$ \omega D^*_s (12{\text{%}})+K^* D^*(7{\text{%}})+Di(28{\text{%}})+K(45{\text{%}}) $ 

$ 2965+{\rm i}0.5 $ 

$ \omega D^*_s (8{\text{%}})+K^* D^*(7{\text{%}})+H(13{\text{%}})+K(65{\text{%}}) $ 

$ 3026+{\rm i}3.8 $ 

$ H(20{\text{%}})+Di(19{\text{%}})+K(48{\text{%}}) $ 

$ 3344+{\rm i}3.3 $ 

$ 1(0^+) $ 

$ H(19{\text{%}})+Di(30{\text{%}})+K(35{\text{%}}) $ 

$ 2770+{\rm i}1.5 $ 

$ 1(1^+) $ 

$ \pi D^*_s (24{\text{%}})+Di(14{\text{%}})+K(56{\text{%}}) $ 

$ 2470+{\rm i}1.0 $ 

$ K^* D^* (10{\text{%}})+H(18{\text{%}})+Di(17{\text{%}})+K(49{\text{%}}) $ 

$ 3134+{\rm i}2.0 $ 

$ 1(2^+) $ 

$ Di(27{\text{%}})+K(43{\text{%}}) $ 

$ 3031+{\rm i}0.7 $ 

$ H(22{\text{%}})+Di(28{\text{%}})+K(46{\text{%}}) $ 

$ 3105+{\rm i}3.7 $ 

$ Di(27{\text{%}})+K(55{\text{%}}) $ 

$ 3373+{\rm i}4.2 $ 

$ Di(39{\text{%}})+K(44{\text{%}}) $ 

$ 3455+{\rm i}12.9 $ 

$ \bar{q}q\bar{s}b $ tetraquarks

$ I(J^P) $ 

Dominant Component Theoretical resonance $ 0(0^+) $ 

$ K B (17{\text{%}})+Di(14{\text{%}})+K(59{\text{%}}) $ 

$ 6011+{\rm i}9.0 $ 

$ K^* B^* (10{\text{%}})+Di(22{\text{%}})+K(54{\text{%}}) $ 

$ 6323+{\rm i}4.0 $ 

$ H(18{\text{%}})+Di(25{\text{%}})+K(49{\text{%}}) $ 

$ 6397+{\rm i}13.8 $ 

$ H(21{\text{%}})+Di(31{\text{%}})+K(34{\text{%}}) $ 

$ 6643+{\rm i}23.4 $ 

$ H(28{\text{%}})+Di(21{\text{%}})+K(33{\text{%}}) $ 

$ 6678+{\rm i}16.0 $ 

$ 0(1^+) $ 

$ K B^* (17{\text{%}})+Di(17{\text{%}})+K(52{\text{%}}) $ 

$ 6031+{\rm i}12.0 $ 

$ K^* B (10{\text{%}})+K^* B^* (12{\text{%}})+K(52{\text{%}}) $ 

$ 6298+{\rm i}16.0 $ 

$ Di(22{\text{%}})+K(44{\text{%}}) $ 

$ 6413+{\rm i}20.6 $ 

$ K B^* (11{\text{%}})+H(25{\text{%}})+K(44{\text{%}}) $ 

$ 6607+{\rm i}4.2 $ 

$ H(21{\text{%}})+Di(27{\text{%}})+K(37{\text{%}}) $ 

$ 6652+{\rm i}7.7 $ 

$ 0(2^+) $ 

$ \omega B^*_s (9{\text{%}})+K^* B^*(10{\text{%}})+Di(13{\text{%}})+K(58{\text{%}}) $ 

$ 6239+{\rm i}0.8 $ 

$ \omega B^*_s (15{\text{%}})+K^* B^*(7{\text{%}})+K(68{\text{%}}) $ 

$ 6314+{\rm i}3.5 $ 

$ \omega B^*_s (11{\text{%}})+K^* B^*(7{\text{%}})+H(26{\text{%}})+K(43{\text{%}}) $ 

$ 6619+{\rm i}4.0 $ 

$ H(18{\text{%}})+Di(32{\text{%}})+K(38{\text{%}}) $ 

$ 6664+{\rm i}6.9 $ 

$ 1(0^+) $ 

$ \pi B_s (7{\text{%}})+K B(8{\text{%}})+Di(13{\text{%}})+K(58{\text{%}}) $ 

$ 6080+{\rm i}2.5 $ 

$ \pi B_s (8{\text{%}})+K B(5{\text{%}})+H(11{\text{%}})+K(63{\text{%}}) $ 

$ 6149+{\rm i}1.6 $ 

$ 1(1^+) $ 

$ \pi B^*_s (27{\text{%}})+K(59{\text{%}}) $ 

$ 5764+{\rm i}0.4 $ 

$ Di(23{\text{%}})+K(46{\text{%}}) $ 

$ 6103+{\rm i}10.3 $ 

$ \pi B^*_s (4{\text{%}})+K^* B^* (5{\text{%}})+K(71{\text{%}}) $ 

$ 6308+{\rm i}8.4 $ 

$ \pi B^*_s (6{\text{%}})+K^* B^*(4{\text{%}})+Di(27{\text{%}})+K(37{\text{%}}) $ 

$ 6413+{\rm i}3.8 $ 

$ 1(2^+) $ 

$ \rho B^*_s (6{\text{%}})+K^* B^* (9{\text{%}})+Di(14{\text{%}})+K(60{\text{%}}) $ 

$ 6301+{\rm i}0.8 $ 

$ \rho B^*_s (13{\text{%}})+H(16{\text{%}})+K(57{\text{%}}) $ 

$ 6399+{\rm i}2.6 $ 

$ Di(28{\text{%}})+K(46{\text{%}}) $ 

$ 6654+{\rm i}5.3 $ 

$ Di(42{\text{%}})+K(46{\text{%}}) $ 

$ 6740+{\rm i}10.0 $ 

All identified exotic states are expected to be confirmed in future high-energy particle and nuclear experiments.
The ${ {\bar{\boldsymbol q}\boldsymbol q\bar{\boldsymbol s}\boldsymbol Q} \;\; {(\boldsymbol q {\bf =}\boldsymbol u,\,\boldsymbol d;\,\boldsymbol Q{\bf =}\boldsymbol c,\,\boldsymbol b)} }$ tetraquark system in a chiral quark model
- Received Date: 2024-01-10
- Available Online: 2024-07-15
Abstract: The S-wave







 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF









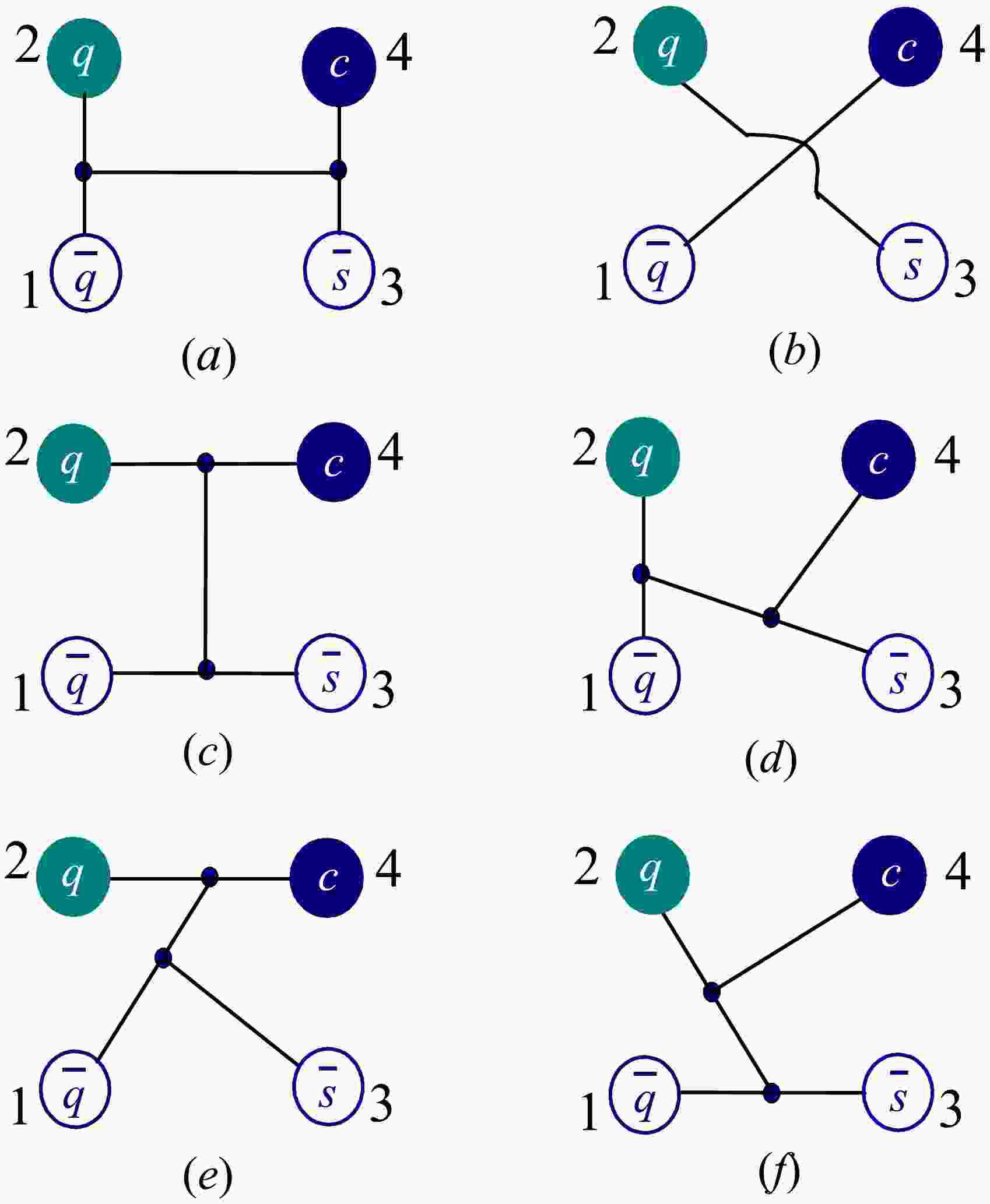










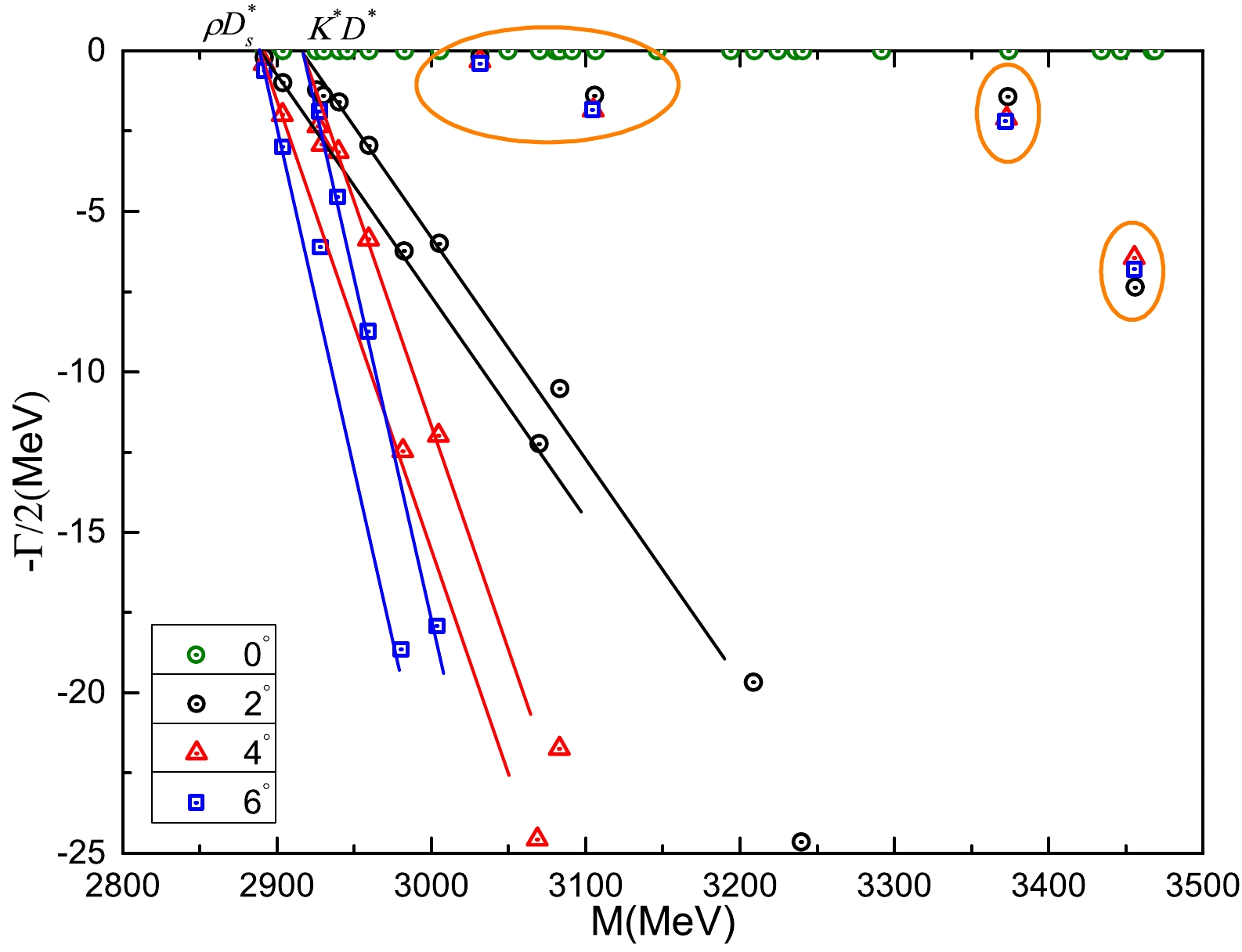



 DownLoad:
DownLoad: