-
Flavor physics plays a pivotal role not only in testing the Standard Model (SM) parameters but also in tracing the signatures of New Physics (NP). Most efforts in this area focus on the detailed study of B meson decays as they are rich in phenomenology. Furthermore, from the NP point of view, the rare decays of B meson, in particular the decays induced by flavor changing neutral current (FCNC) transitions
$ b\to q $ with$ q=d,s $ , are of significant interest, as these decays are loop suppressed and only allowed via the GIM mechanism in the SM [1]. Specifically, the observables involved in the rare radiative decay$ b\to q\gamma $ and rare semileptonic decay$ b\to q\ell^{+}\ell^{-} $ allow the exploration of the structure of NP. Semileptonic decays, which involve the$ b\to s $ current, have been analyzed rigorously in the past and have shown discrepancies from the SM predictions both in the lepton flavor-dependent (LFD) and lepton flavor-independent observables, defined as lepton flavor universality (LFU) ratios.Among LFD (
$ b\to s\mu\mu $ ) observables, deviations are observed from SM predictions in the branching fractions of$ B\to K\mu^{+}\mu^{-} $ [2],$ B\to K^{\ast}\mu^{+}\mu^{-} $ [2−4], and$ B_{s}\to\phi\mu^{+}\mu^{-} $ [5, 6] decays. The observed branching fractions suggest lower values when compared to their SM predictions. Furthermore, in$ B^{0}\to K^{\ast 0}\mu^{+}\mu^{-} $ decay, the angular observable,$ P_{5}^{\prime} $ , has shown mismatch from the SM values [7, 8]. Interestingly, global fits predict the NP effects present in the LFD observables involving$ b\to s\mu\mu $ transition. Following this, NP effects in different complementary decay modes, driven by the same quark level transition$ b\to s\mu\mu $ , such as$ B\to K_{1}\mu^{+}\mu^{-} $ [9−12],$ B\to K_{2}^{\ast}\mu^{+}\mu^{-} $ [13, 14],$ B_s\to f_{2}^{\prime}\mu^{+}\mu^{-} $ [14, 15], and$ B_{c}\to D_{s}^{(\ast)}\mu^{+}\mu^{-} $ [16−19], have been investigated in model-independent approach and specific NP models.LFU ratios in
$ b\to s $ sector have been measured by LHCb collaboration [20−22], defined as$R_{K^{(\ast)}}= \dfrac{{\cal{B}}(B\to K^{(\ast)}\mu^{+}\mu^{-})}{{\cal{B}}(B\to K^{(\ast)}e^{+}e^{-})}$ , in different$ q^{2} $ bins, and their analysis showed a$ 3\sigma $ deviation from the SM prediction. A similar analysis has been performed by BELLE collaboration [23, 24], for the same ratios$ R_{K^{(\ast)}} $ in the$ q^{2}\in (1-6) $ $ {\rm{GeV}}^{2} $ bin, and it shows consistency with the SM predictions but with large experimental uncertainties. Furthermore, the recent measurements of ratios$ R_{K^{(\ast)}} $ in the low and central$ q^{2} $ region of the spectrum by LHCb collaboration [25, 26] have shown an agreement with the SM predictions.Apart from the LFU violation in
$ R_{K^{(\ast)}} $ , LFU violation has also been examined in flavor changing charged current (FCCC) semileptonic$ B\to D^{(\ast)}\ell\nu_{\ell} $ decay mode via ratios$R_{D^{(\ast)}}=\dfrac{{\cal{B}}(B\to D^{(\ast)}\tau^{+}\nu_{\tau})}{{\cal{B}}(B\to D^{(\ast)}\ell\nu_{\ell})}$ with$ (\ell=e, \mu) $ [27−30]. However, the recent analysis of$ R_{D^{\ast}} $ and$ R_{D} $ , via LHCb [31] and Belle collaboration [32], shows a good agreement with the SM predictions. To draw any conclusion regarding the status of NP, the other sectors must be exploited.In this study, we consider FCNC processes governed by
$ b\to d\ell^{+}\ell^{-} $ transitions, as these modes are CKM suppressed when compared to those of$ b\to s\ell^{+}\ell^{-} $ transitions. The typical branching ratios that belong to$ b\to d\ell^{+}\ell^{-} $ processes are of the order$ 10^{-8} $ , and hence the measurements of these modes are considered to be challenging. To date, only the branching ratios of rare$ b\to d\mu^{+}\mu^{-} $ and$ b\to d\gamma $ decays have been measured, and the observed decay modes are (i)$ B^{+}\to\pi^{+}\mu^{+}\mu^{-} $ [33] (ii)$ B^{0}\to\mu^{+}\mu^{-} $ [34] (iii)$ B^{0}_{s}\to K^{\ast 0}\mu^{+}\mu^{-} $ [35] (iv)$ B\to X_{d}\gamma $ [36, 37]. In previous studies, researchers used data sets to extract information on NP Wilson coefficients from the global fit analysis; see Refs. [38−41]. Furthermore, the experimental data can be used to extract the information on the Wilson coefficients of different NP models such as family non-universal$ Z^{\prime} $ Model [42, 43], supersymmetric models [44], and Two Higgs doublet Models [45].The goal of our study is to use the family non-universal
$ Z^{\prime} $ effective Hamiltonian and perform the four-fold angular analysis of$ B\to\rho(\to\pi\pi)\mu\mu $ and$ B\to a_{1}(\to\rho_{\parallel,\perp}\pi)\mu\mu $ decays. For$ B\to\rho $ decay, we use the fit results for simplified series expansion (SSE) coefficients in the fit to Light Cone Sum Rules (LCSR) form factors [46], and for the$ B\to a_{1} $ decay, we use the perturbative QCD (pQCD) form factors [47]. Both the decays are analyzed in the low$ q^{2} $ region of the spectrum. For the decay channel$ \rho\to\pi\pi $ , the probability is 100%, whereas for the decays$ a_{1}\to\rho_{\parallel}\pi $ and$ a_{1}\to\rho_{\perp}\pi $ , the probability is 17% and 43%, respectively. In our study, we choose the values of Wilson coefficients from [43] and provide the predictions of different physical observables such as differential branching fractions, forward-backward asymmetry, longitudinal helicity fraction of$ \rho $ and$ a_{1} $ mesons, and the individual normalized angular observables within the SM and two scenarios of the family non-universal$ Z^{\prime} $ model.The organization of this study is as follows. In section II, we present the theoretical framework, which includes effective Hamiltonian of the family non-universal
$ Z^{\prime} $ model for$ b\to d\mu^{+}\mu^{-} $ transition, where the basis operators remain the same as that of the SM. Then, we express the matrix elements for$ B\to\rho\mu^{+}\mu^{-} $ and$ B\to a_{1}\mu^{+}\mu^{-} $ decays in terms of form factors. Furthermore, using the helicity formalism, we derive the four-fold angular decay distribution of$ B\to\rho(\to\pi\pi)\mu\mu $ and$ B\to a_{1}(\to\rho_{\parallel,\perp}\pi)\mu\mu $ decays, which contains the angular coefficients given in terms of the helicity amplitudes. These angular coefficients are then used to construct various physical observables along with the normalized angular coefficients. In section III, we present numerical analysis of the physical observables in the SM and family non-universal$ Z^{\prime} $ model, and finally, section IV, summarizes our study. -
In this section, we provide the effective electroweak (EW) Hamiltonian approach [48, 49], where the SM heavy degrees of freedom, such as
$W^{\pm},\; Z^{0}$ gauge bosons and the top quark are integrated. The effective Hamiltonian is then used to calculate the full angular distribution of$ B\to\rho(770)(\to\pi\pi)\mu^{+}\mu^{-} $ and$ B\to a_{1}(1260) (\to\rho_{\parallel, \perp}\pi)\mu^{+}\mu^{-} $ decays. Using the form of four-fold angular distribution, we extract$ q^{2} $ dependent angular coefficients, which are further used to analyze the signatures of the family non-universal$ Z^{\prime} $ model. -
A family non-universal
$ Z^{\prime} $ gauge boson can be naturally derived in many extensions of the SM. The simplest way to incorporate the$ Z^{\prime} $ gauge boson is by incorporating extra$ U^{\prime}(1) $ gauge symmetry. The model was formulated by Langacker and Plümacher [50]. One of the features of this model is that FCNC transitions can be induced at tree level due to the non-diagonal chiral coupling matrix. The signatures of$ Z^{\prime} $ gauge boson arise in two different ways.(i) By introducing new Wilson coefficients only and the basis of operators remains the same as that of SM.
(ii) In other approach, new Wilson coefficients and new operators are added to the SM effective Hamiltonian.
In this study, we will analyze the family non-universal
$ Z^{\prime} $ model using the aforementioned B meson decays, and the NP in this model arises due to the modification of the Wilson coefficients$ C^{{\rm{eff}}}_{9} $ and$ C_{10} $ while the structure of the operators remains the same as that of SM. Wilson coefficients can be modified due to the off-diagonal couplings of quarks as well as leptons with$ Z^{\prime} $ gauge boson. The current due to extra$ U^{\prime}(1) $ gauge symmetry in the SM eigenstate basis can be expressed as [50, 51]$ J_{\mu}=\sum\limits_{i,j} \bar{\psi}_{i}\gamma_{\mu}[\epsilon_{\psi_{L_{ij}}}P_{L}+\epsilon_{\psi_{R_{ij}}}P_{R}]\psi_{j}, $

(1) where the summation runs overall quarks and leptons field
$ \psi_{ij} $ ,$ P_{R,L}=\dfrac{1}{2}(1\pm\gamma^{5}) $ are the right-handed and left-handed projectors, and$ \epsilon_{\psi_{R,L}} $ indicates the chiral couplings of the new gauge boson.As already discussed, in the family non-universal
$ Z^{\prime} $ model, FCNC transition arises at the tree level if the chiral coupling matrices$ \epsilon_{\psi R, L} $ are non- diagonal. However, if the couplings of$ Z^{\prime} $ gauge bosons are diagonal but non-universal, flavor-changing couplings are generated via fermion mixing. The fermion Yukawa matrix$ h_{\psi} $ can be diagonalized in the weak eigenstate basis through CKM unitary matrices$ V^{\psi}_{R, L} $ and can be expressed as$ h_{\psi,diag}=V^{\psi}_{R}h_{\psi}V^{\dagger\psi}_{L}. $

(2) Hence, the chiral
$ Z^{\prime} $ couplings in the fermion mass eigenstates can be expressed as [51]$ B^{\psi_{L}}_{ij}=(V^{\psi}_{L}\epsilon_{\psi_{L}}V^{\dagger\psi}_{L})_{ij}, B^{\psi_{R}}_{ij}=(V^{\psi}_{R}\epsilon_{\psi_{R}}V^{\dagger\psi}_{R})_{ij}. $

(3) In Eq. (3), the non-vanishing quark coupling matrices
$ B^{\psi_{L, R}}_{ij} $ represent the signature of the NP. Furthermore, two decades ago it was shown that the flavor non-universal$ Z^{\prime} $ model can be used to improve the precision of electroweak data [52].At quark level, decay
$ B\to M\mu^{+}\mu^{-} $ ($ M=\rho(770), a_{1}(1260) $ ) modes are governed by$ b\to d $ transitions. Hence, FCNC Lagrangian due to$ Z^{\prime} $ model can be expressed as [43]$ {\cal{L}}^{Z^{\prime}}_{{\rm{FCNC}}}=-g^{\prime}(B^{L}_{db}\bar{d}_{L}\gamma_{\mu}b_{L}+B^{R}_{db}\bar{d}_{R}\gamma_{\mu}b_{R})Z^{\prime\mu}+{\rm h.c.}, $

(4) where
$ g^{\prime} $ denotes the gauge coupling associated with$ U^{\prime}(1) $ gauge group. -
The signatures of
$ Z^{\prime} $ gauge boson can be analyzed through the decay modes of B mesons within the framework of SM low energy effective field theory. As mentioned earlier that in this framework, the heavy degrees of freedom, including the new particles, are integrated (Wilson coefficients), and the effective Hamiltonian appears in terms of four Fermi operators as well as the Wilson coefficients.The effective Hamiltonian for modes
$ B\to M\mu^{+}\mu^{-} $ ($ M=\rho(770),a_{1}(1260) $ ) in the SM can be expressed as$\begin{aligned}[b] H^{{\rm{SM}}}_{{\rm{eff}}}=\;&-\frac{4G_{F}\alpha}{\sqrt{2}}V_{tb}V^{\ast}_{td}\Bigg[\sum\limits_{i=1}^{10}C_{i}O_{i}-\lambda_{u}\{C_{1}[O^{u}_{1}-O_{1}] \\&+C_{2}[O^{u}_{2}-O_{2}]\}\Bigg], \end{aligned}$

(5) where
$ G_{F} $ denotes the Fermi coupling constant,$ V_{ij} $ , and$ \lambda_{u}=\dfrac{V_{ub}V^{\ast}_{ud}}{V_{tb}V^{\ast}_{td}} $ represents the corresponding CKM factors and their ratios. The explicit form of the four fermion operators that contribute to the said process in the SM can be expressed as$ \begin{aligned}[b] O_{7\gamma} =\;&\frac{e}{16\pi ^{2}}m_{b}\left( \bar{d}\sigma _{\mu \nu }P_{R}b\right) F^{\mu \nu }\,, \\ O_{9} =\;&\frac{e^{2}}{16\pi ^{2}}(\bar{d}\gamma _{\mu }P_{L}b)(\bar{\ell}\gamma^{\mu }\ell)\,, \\ O_{10} =\;&\frac{e^{2}}{16\pi ^{2}}(\bar{d}\gamma _{\mu }P_{L}b)(\bar{\ell} \gamma ^{\mu }\gamma _{5} \ell)\,, \end{aligned} $

(6) where
$ F^{\mu\nu} $ denotes the electromagnetic field strength tensor, e denotes an electromagnetic coupling constant, and$ m_{b} $ appears in the electromagnetic dipole operator expression is the running b quark mass in$ \overline{{\rm{MS}}} $ scheme.In Eq. (5),
$ C_{i}(\mu) $ denotes the Wilson coefficients at the energy scale$ \mu $ . The form of$ C_{7}^{{\rm{eff}}}(q^{2}) $ and$ C_{9}^{{\rm{eff}}}(q^{2}) $ Wilson coefficients [39, 53−57], which contain the factorizable contributions from current-current, QCD penguins, and chromomagnetic dipole operators$ O_{1-6,8} $ , are provided in Appendix A.At the quark level, decays
$ B\to M\mu^{+}\mu^{-} $ are governed by$ b\to d\mu^{+}\mu^{-} $ transition, and in the framework of SM its amplitude can be expressed as$ \begin{aligned}[b]& {\cal{M}}^{{\rm{SM}}}\left(b \rightarrow d \mu^+ \mu^-\right) \\=\;&\frac{G_F \alpha V_{t b} V_{t d}^*}{2 \sqrt{2} \pi}\left\{C_9^{e f f}\left\langle M(k, \epsilon)\left|\bar{d} \gamma^\mu\left(1-\gamma^5\right) b\right| B(p)\right\rangle \bar{\ell} \gamma_\mu \ell\right. \\ & +C_{10}^{{\rm{SM}}}\left\langle M(k, \epsilon)\left|\bar{d} \gamma^\mu\left(1-\gamma^5\right) b\right| B(p)\right\rangle \bar{\ell} \gamma_\mu \gamma_5 \ell \\ & \left.-\frac{2 m_b}{q^2} C_7^{{\rm{eff}}}\left\langle M(k, \epsilon)\left|\bar{d} i \sigma^{\mu \nu} q_\nu\left(1+\gamma^5\right) b\right| B(p)\right\rangle \bar{\ell} \gamma_\mu \ell\right\}, \end{aligned} $

(7) As discussed above in the family non-universal
$ Z^{\prime} $ model, the FCNC transition arises at the tree level. Hence, by ignoring$ Z-Z^{\prime} $ mixing and assuming that the couplings of right-handed quark flavors with$ Z^{\prime} $ boson are diagonal [58−60], the effective Hamiltonian for$ b\to d\mu^{+}\mu^{-} $ transition in the family non-universal$ Z^{\prime} $ model can be expressed in a straightforward manner as$\begin{aligned}[b] {\cal{H}}^{Z^{\prime}}_{{\rm{eff}}}=\;&-\frac{2G_{F}}{\sqrt{2}}V_{tb}V^{\ast}_{td}\Bigg[-\frac{B^{L}_{db}B^{L}_{\ell\ell}}{V_{tb}V^{\ast}_{td}} (\bar{d}b)_{V-A}(\bar{\ell}\ell)_{V-A}\\&-\frac{B^{L}_{db}B^{R}_{\ell\ell}}{V_{tb}V^{\ast}_{td}}(\bar{d}b)_{V-A}(\bar{\ell}\ell)_{V+A}\Bigg], \end{aligned}$

(8) where
$B^{L}_{db}=|B^{L}_{db}| {\rm e}^{-{\rm i}\phi_{db}}$ represents the left-handed coupling of quarks with$ Z^{\prime} $ gauge boson and$ \phi_{db} $ denotes the new CP-violating phase, which is not present in the SM. In condensed notation, Eq. (8) can be expressed as$ {\cal{H}}^{Z^{\prime}}_{{\rm{eff}}}=-\frac{4G_{F}}{\sqrt{2}}V_{tb}V^{\ast}_{td}\left[\Lambda_{db}C^{Z^{\prime}}_{9}O_{9} +\Lambda_{db}C^{Z^{\prime}}_{10}O_{10}\right], $

(9) where
$ \Lambda_{db}=\frac{4\pi {\rm e}^{-{\rm i}\phi_{db}}}{\alpha V_{tb}V^{\ast}_{td}}, $

(10) $ C^{Z^{\prime}}_{9}=|B^{L}_{db}|S_{LR}; C^{Z^{\prime}}_{10}=|B^{L}_{db}|D_{LR}, $

(11) and
$ S_{LR}=B^{L}_{\ell\ell}+B^{R}_{\ell\ell},\quad D_{LR}=B^{L}_{\ell\ell}-B^{R}_{\ell\ell}. $

(12) In Eq. (12),
$ S_{LR} $ and$ D_{LR} $ constitutes the couplings of new$ Z^{\prime} $ gauge boson with left and right-handed leptons. The total amplitude for the decay$ B\to M\mu^{+}\mu^{-} $ in terms of SM and in the$ Z^{\prime} $ model can be expressed as$ \begin{aligned}[b]& {{\cal{M}}^{{\rm{tot}}}(b\to d\ell^+\ell^-)}\\=\;&{\frac{G_{F}\alpha V_{tb}V_{td}^{\ast}}{2\sqrt{2}\pi}\left\{C_{9}^{{\rm{tot}}}\langle M(k,\epsilon)|\bar d\gamma^{\mu}(1-\gamma^{5})b|B(p)\rangle \bar{\ell}\gamma_{\mu}\ell\right.}\\ &{+C_{10}^{{\rm{tot}}}\langle M(k,\epsilon)|\bar d\gamma^{\mu}(1-\gamma^{5})b|B(p)\rangle\bar{\ell}\gamma_{\mu}\gamma_{5}\ell}\\ &{\left.-\frac{2m_{b}}{q^{2}}C_{7}^{{\rm{eff}}}\langle M(k,\epsilon)|\bar{d}i\sigma^{\mu\nu}q_{\nu}(1+\gamma^{5})b|B(p)\rangle\bar{\ell}\gamma_{\mu}\ell\right\}\,, } \end{aligned} $

(13) where
$ {\cal{M}}^{{\rm{tot}}}={\cal{M}}^{{\rm{SM}}}+{\cal{M}}^{{\rm{ZP}}}, $

(14) and
$ C_{9}^{{\rm{tot}}}=C_{9}^{{\rm{eff}}}+\Lambda_{db} C^{Z^{\prime}}_{9},\quad C_{10}^{{\rm{tot}}}=C_{10}^{{\rm{SM}}}+\Lambda_{db} C^{Z^{\prime}}_{10}. $

(15) Furthermore, the amplitude for decays
$ B\to M\ell^{+}\ell^{-} $ in the framework of SM and in the family non-universal$ Z^{\prime} $ model can be expressed as$\begin{aligned}[b]& {\cal{M}}^{{\rm{tot}}}\left(B\to M\ell^+\ell^-\right)\\=\;&\frac{G_{F}\alpha}{2\sqrt{2}\pi}V_{tb}V^{\ast}_{td}\left\{T^{1,M}_{\mu}(\bar{\ell}\gamma^{\mu}\ell) +T^{2,M}_{\mu}(\bar{\ell}\gamma^{\mu}\gamma_{5}\ell)\right\},\end{aligned} $

(16) where
$\begin{aligned}[b] T^{1,M}_{\mu}=\;&C_{9}^{{\rm{tot}}}\langle M(k,\varepsilon)|\bar s\gamma_{\mu}(1-\gamma_{5})b|B(p)\rangle\\& -\frac{2m_{b}}{q^{2}}C_{7}^{{\rm{eff}}} \langle M(k,\varepsilon)|\bar s {\rm i}\sigma_{\mu\nu}q^{\nu}(1+\gamma_{5})b|B(p)\rangle,\end{aligned} $

(17) $ T^{2,M}_{\mu}=C_{10}^{{\rm{tot}}}\langle M(k,\varepsilon)|\bar s\gamma_{\mu}(1-\gamma_{5})b|B(p)\rangle, $

(18) where
$ T^{i,M}_{\mu} $ ,$ i=(1,2) $ and contain the matrix elements of$ B\to M $ . -
The form factors for
$ B\to\rho $ and$ B\to a_{1} $ decays can be expressed in terms of Lorentz invariant form factors as$ \langle \rho(k,\overline\epsilon)\left\vert \bar{s}\gamma _{\mu }b\right\vert B(p)\rangle =\frac{2\epsilon_{\mu\nu\alpha\beta}} {m_{B}+m_{\rho}}\overline\epsilon^{\,\ast\nu}p^{\alpha}k^{\beta}V(q^{2}), $

(19) $ \begin{aligned}[b]& {\langle \rho(k,\overline\epsilon)\left\vert \bar{s}\gamma_{\mu}\gamma_{5}b\right\vert B(p)\rangle }\\=\;&{{ i}\left(m_{B}+m_{\rho}\right)g_{\mu\nu}\overline\epsilon^{\,\ast\nu}A_{1}(q^{2})} \\ &{-{ i}P_{\mu}(\overline\epsilon^{\ast}\cdot q)\frac{A_{2}(q^{2})}{\left(m_{B}+m_{\rho}\right)}}\\ &{-{ i}\frac{2m_{\rho}}{q^{2}}q_{\mu}(\overline\epsilon^{\,\ast}\cdot q) \left[A_{3}(q^{2})-A_{0}(q^{2})\right],} \end{aligned} $

(20) where
$ P_{\mu}=p_{\mu}+k_{\mu} $ ,$ q_{\mu}=p_{\mu}-k_{\mu} $ , and$ A_3(0)=A_0(0) $ . We used$ \epsilon_{0123}=+1 $ convention throughout the study. The additional tensor form factors are expressed as$ \langle \rho(k,\overline\epsilon)\left\vert \bar{s}i\sigma _{\mu \nu }q^{\nu }b\right\vert B(p)\rangle =-2\epsilon _{\mu\nu\alpha\beta}\overline\epsilon^{\,\ast\nu}p^{\alpha}k^{\beta}T_{1}(q^{2}), $

(21) $ \begin{aligned}[b]& {\langle \rho(k,\overline\epsilon )\left\vert \bar{s}{i}\sigma _{\mu \nu }q^{\nu}\gamma_{5}b\right\vert B(p)\rangle}\\=\; &{{i}\left[\left(m^2_{B}-m^2_{\rho}\right)g_{\mu\nu}\overline\epsilon^{\,\ast\nu}\right.}\\ &{\left.-(\overline\epsilon^{\,\ast }\cdot q)P_{\mu}\right]T_{2}(q^{2})+{i}(\overline\epsilon^{\,\ast}\cdot q)}\\ &{\times\left[q_{\mu}-\frac{q^{2}}{m^2_{B}-m^2_{\rho}}P_{\mu} \right]T_{3}(q^{2}).} \end{aligned} $

(22) The relations between the form factors in [46], and the form factors provided in the aforementioned matrix elements are
$ \begin{aligned}[b] A_{12}(q^{2})=\;&\frac{\left(m_{B}+m_{\rho}\right)^2(m^2_{B}-m^2_{\rho}-q^2) A_{1}(q^{2})-\lambda A_{2}(q^{2})}{16m_{B}m^2_{\rho}\left(m_{B}+m_{\rho}\right)}, \\ T_{23}(q^{2})=\;&\frac{\left(m^2_{B}-m^2_{\rho}\right)(m^2_{B}+3m^2_{\rho}-q^2) T_{2}(q^{2})-\lambda T_{3}(q^{2})}{8m_{B}m^2_{\rho}\left(m_{B}-m_{\rho}\right)}. \end{aligned} $

(23) and
$ \begin{aligned}[b] {\langle a_{1}(k,\overline\epsilon)|V_{\mu}|B(p)\rangle}=\;&-\overline\epsilon^{\ast}_{\mu}(m_{B}+m_{a_{1}})V_{1}(q^{2})\\&+ (p+k)_{\mu}(\overline\epsilon^{\ast}.q)\frac{V_{2}(q^{2})}{m_{B}+m_{a_{1}}}\\ &+q_{\mu}(\overline\epsilon^{\ast}.q)\frac{2m_{a_{1}}}{q^{2}}[V_{3}(q^{2})-V_{0}(q^{2})]\,, \end{aligned}$

(24) $ \langle a_{1}(k,\overline\epsilon)|A_{\mu}|B(p)\rangle=\frac{2{i}\epsilon_{\mu\nu\alpha\beta}}{m_{B}+m_{a_{1}}} \overline\epsilon^{\ast\nu}p^{\alpha}k^{\beta}A(q^{2})\,, $

(25) where
$ V^{\mu}=\bar d\gamma^{\mu}b $ and$ A^{\mu}=\bar d\gamma^{\mu}\gamma^{5}b $ are the vector and axial vector currents, respectively, and$ \overline\epsilon^{\ast\nu} $ denotes the polarization vectors of the axial vector meson. The relation for the vector form factor$ V_{3}(q^{2}) $ , provided in Eq. (24), can be expressed as$ \begin{aligned}[b]& V_{3}(q^{2})=\frac{m_{B}+m_{a_{1}}}{2m_{a_{1}}}V_{1}(q^{2})-\frac{m_{B}-m_{a_{1}}}{2m_{a_{1}}}V_{2}(q^{2})\,, \\& V_{3}(0)=V_{0}(0)\,. \end{aligned} $

(26) $ \begin{aligned}[b]& {\langle a_{1}(k,\overline\epsilon)|\bar di\sigma_{\mu\nu}q^{\nu}b|B(p)\rangle}\\=\;&{[(m_{B}^{2}-m_{a_{1}}^{2})\overline\epsilon^{\ast}_{\mu} -(\overline\epsilon^{\ast}.q)(p+k)_{\mu}]T_{2}(q^{2})}\\ &{+(\overline\epsilon^{\ast}.q)\left[q_{\mu}-\frac{q^{2}}{m^2_{B}-m^2_{a_{1}}}(p+k)_{\mu}\right]T_{3}(q^{2})\,,} \end{aligned} $

(27) $ \langle a_{1}(k,\overline\epsilon)|\bar d {i}\sigma_{\mu\nu}q^{\nu}\gamma^{5}b|B(p)\rangle=2{i}\epsilon_{\mu\nu\alpha\beta}\overline\epsilon^{\ast\nu}p^{\alpha}k^{\beta}T_{1}(q^{2})\,. $

(28) -
To calculate the angular distribution of the four-fold
$ B\to\rho (\to\pi\pi)\mu^{+}\mu^{-} $ and$ B\to a_{1}(\to\rho_{\parallel, \perp}\pi)\mu^{+}\mu^{-} $ decays, we use the helicity formalism and follow [61]. The kinematics of the four-fold decays under consideration are shown in Fig. 1. The completeness and orthogonality properties of helicity basis can expressed as follows:
Figure 1. (color online) Kinematics of
$ B\rightarrow \rho(\rightarrow \pi\pi)l^{+}l^{-} $ and$ B\rightarrow a_{1}(\rightarrow \rho\pi)l^{+}l^{-} $ decays.$ \varepsilon^{\ast\alpha}(n)\varepsilon_{\alpha}(l)=g_{nl}, \quad \sum\limits_{n, l=t, +, -, 0}\varepsilon^{\ast\alpha}(n)\varepsilon^{\beta}(l)g_{nl}=g^{\alpha\beta}, $

(29) with
$ g_{nl}={\rm{diag}}(+, -, -, -) $ . From the completeness relation provided in Eq. (29), the contraction of leptonic tensors$ L^{(k)\alpha\beta} $ and hadronic tensors$ H^{ij}_{\alpha\beta}=T^{i,M}_{\alpha}\overline{T}^{\,j,M}_{\beta} $ $ (i, j=1, 2) $ can be expressed as$ L^{(k)\alpha\beta}H^{ij}_{\alpha\beta}=\sum\limits_{n, n^{\prime}, l, l^{\prime}}L^{(k)}_{nl}g_{nn^{\prime}}g_{ll^{\prime}}H^{ij}_{n^{\prime}l^{\prime}}, $

(30) where leptonic and hadronic tensors can be expressed in the helicity basis as follows:
$ L^{(k)}_{nl}=\varepsilon^{\alpha}(n)\varepsilon^{\ast\beta}(l)L^{(k)}_{\alpha\beta},\quad H^{ij}_{nl}=\varepsilon^{\ast\alpha}(n)\varepsilon^{\beta}(l)H^{ij}_{\alpha\beta}. $

(31) Leptonic and hadronic tensors shown in Eq. (31) can be evaluated in two different frames of reference. The lepton tensor
$ L^{(k)}_{nl} $ is evaluated in the dimuon center of mass (CM) frame, and the hadronic tensor$ H^{ij}_{nl} $ is evaluated in the rest frame of B meson. For the above mentioned decays, the hadronic tensor can be expressed as follows,$ \begin{aligned}[b] {H^{ij}_{nl}}\;&{=\left(\varepsilon^{\ast\alpha}(n)T^{i,M}_{\alpha}\right)\cdot\left(\overline{\varepsilon^{\ast\beta}(l)T^{j,M}_{\beta}}\right)} \\ &{=\left(\varepsilon^{\ast\alpha}(n)\overline\epsilon^{\ast\mu}(r)T^{i,M}_{\alpha,\mu}\right)\cdot\left(\overline{\varepsilon^{\ast\beta}(l) \overline\epsilon^{\ast\nu}(s)T^{j,M}_{\beta,\nu}}\right)\delta_{rs}}\\ & \equiv H^{i,M}_n \,\overline{H}^{\, j,M_l}_l. \end{aligned} $

(32) -
The explicit expressions of the helicity amplitudes for
$ B\to\rho $ and$ B\to a_{1} $ are as follows:$ \begin{aligned}[b] H^{1, \rho}_t=\;&-{i}\sqrt{\frac{\lambda}{q^2}}C_{9}^{{\rm{tot}}}A_0(q^{2}), \\ H^{2, \rho}_t=\;&-{i}\sqrt{\frac{\lambda}{q^2}}C_{10}^{{\rm{tot}}}A_0(q^{2}), \\ H^{1, \rho}_{\pm}=\;&-{i}\left(m^2_{B}-m^2_{\rho}\right)\left[C_{9}^{{\rm{tot}}} \frac{A_{1}(q^{2})}{\left(m_{B}-m_{\rho}\right)}\right. \left. +\frac{2m_{b}}{q^{2}}C_{7}^{{\rm{eff}}}T_{2}(q^{2})\right]\\ & \pm {i}\sqrt{\lambda}\left[C_{9}^{{\rm{tot}}} \frac{V(q^{2})}{\left(m_{B}+m_{\rho}\right)}+\frac{2m_{b}}{q^{2}}C_{7}^{{\rm{eff}}}T_{1}(q^{2})\right], \\ H^{2, \rho}_{\pm}=\;&- {i} C_{10}^{{\rm{tot}}}\left(m_{B}+m_{\rho}\right) A_{1}(q^{2})\pm { i}\sqrt{\lambda}C_{10}^{{\rm{tot}}} \frac{V(q^{2})}{\left(m_{B}+m_{\rho}\right)}, \\ H^{1, \rho}_0=\;&-\frac{8{i}m_{B}m_{\rho}}{\sqrt{q^2}}\left[C_{9}^{{\rm{tot}}} A_{12}(q^{2})+m_b C_{7}^{{\rm{eff}}}\frac{T_{23}(q^{2})}{m_{B}+m_{\rho}}\right], \\ H^{2, \rho}_0=\;&-\frac{8{i}m_{B} m_{\rho}}{\sqrt{q^2}}\left[C_{10}^{{\rm{tot}}} A_{12}(q^{2})\right]. \end{aligned} $

(33) and
$ \begin{aligned}[b]H^{1,a_{1}}_t=\;&-\sqrt{\frac{\lambda}{q^2}}C_{9}^{{\rm{tot}}}V_0(q^{2}), \\ H^{2,a_{1}}_t=\;&-\sqrt{\frac{\lambda}{q^2}}C_{10}^{{\rm{tot}}}V_0(q^{2}), \\ H^{1,a_{1}}_{\pm}=\;&-\left(m^2_{B}-m^2_{a_{1}}\right)\left[C_{9}^{{\rm{tot}}} \frac{V_{1}(q^{2})}{\left(m_{B}-m_{a_{1}}\right)}+\frac{2m_{b}}{q^{2}}C_{7}^{{\rm{eff}}}T_{2}(q^{2})\right] \\&\pm \sqrt{\lambda}\left[C_{9}^{{\rm{tot}}} \frac{A(q^{2})}{\left(m_{B}+m_{a_{1}}\right)}+\frac{2m_{b}}{q^{2}}C_{7}^{{\rm{eff}}}T_{1}(q^{2})\right], \\ H^{2,a_{1}}_{\pm}=\;&-C_{10}^{{\rm{tot}}}\left(m_{B}+m_{a_{1}}\right) V_{1}(q^{2})\pm \sqrt{\lambda}C_{10}^{{\rm{tot}}} \frac{A(q^{2})}{\left(m_{B}+m_{a_{1}}\right)}, \\ H^{1,a_{1}}_0=\;&-\frac{1}{2m_{a_{1}}\sqrt{q^2}}\left[C_{9}^{{\rm{tot}}} \left\{(m^2_{B}-m^2_{a_{1}}-q^2)\left(m_{B}+m_{a_{1}}\right)V_{1}(q^{2})\right.\right. \\ &-\frac{\lambda}{m_{B}+m_{a_{1}}}V_{2}(q^{2})\Big\}+2m_b C_{7}^{{\rm{eff}}}\\& \times \Big\{(m^2_{B}+3m^2_{a_{1}}-q^2)T_{2}(q^{2}) -\frac{\lambda}{m^2_{B}-m^2_{a_{1}}}T_{3}(q^{2})\Big\} \Bigg], \\H^{2, a_1}_0=\;&-\frac{1}{2m_{a_{1}}\sqrt{q^2}}C_{10}^{{\rm{tot}}} \Bigg[(m^2_{B}-m^2_{a_{1}}-q^2)\left(m_{B}+m_{a_{1}}\right)V_{1}(q^{2})\\&-\frac{\lambda}{m_{B}+m_{a_{1}}}V_{2}(q^{2})\Bigg]. \end{aligned} $

(34) -
The four-fold decay distribution depends on the square of the dilepton invariant mass
$ q^{2} $ , angles$ \theta_{\ell} $ ,$ \theta_{V} $ , and$ \phi $ as shown in Fig. 1. For$ B\to\rho $ decay mode, the four-fold distribution can be expressed as$ \begin{aligned}[b]&{\frac{{\rm d}^4\Gamma\left(B\to\rho\,(\to \pi\pi)\mu^+\mu^-\right)}{{\rm d} q^2 \ {\rm d}\cos{\theta_{l}} \ {\rm d}\cos {\theta}_{V} \ {\rm d}\phi}} \\=\;&{ \frac{9}{32 \pi} {\cal{B}}(\rho\to \pi\pi)} \\ &{\times\left[I^{\rho}_{1s}\sin^2\theta_{V}+I^{\rho}_{1c}\cos^2\theta_{V}\right.}\\ &{+\left(I^{\rho}_{2s}\sin^2\theta_{V}+I^{\rho}_{2c}\cos^2\theta_{V}\right)\cos{2\theta_{l}}} \\ &{+\left(I^{\rho}_{6s}\sin^2\theta_{V}+I^{\rho}_{6c}\cos^2\theta_{V}\right)\cos{\theta_{l}}}\\ &{+\left(I^{\rho}_{3}\cos{2\phi} +I^{\rho}_{9}\sin{2\phi}\right)\sin^2\theta_{V}\sin^2\theta_{l}} \\ &{+\left(I^{\rho}_{4}\cos{\phi}+I^{\rho}_{8}\sin{\phi}\right)\sin2\theta_{V}\sin2\theta_{l}} \\ &{\left.+\left(I^{\rho}_{5}\cos{\phi}+I^{\rho}_{7}\sin{\phi}\right)\sin2\theta_{V}\sin\theta_{l}\right].}\end{aligned} $

(35) The explicit expressions of
$ I^{\rho}_{n\lambda} $ in terms of the helicity amplitudes are obtained as$ \begin{aligned}[b]{I^{\rho}_{1s}} =\;&{ \frac{(2+\beta_l^2)}{2}N^2\left(|H_+^1|^2+|H_+^2|^2+|H_-^1|^2+|H_-^2|^2\right)} \\&{+\frac{4m_l^2}{q^2}N^2\left(|H_+^1|^2-|H_+^2|^2+|H_-^1|^2-|H_-^2|^2\right),} \end{aligned} $

(36) $ \begin{aligned}[b]I^{\rho}_{1c} =\;& 2N^2\left(|H_0^1|^2+|H_0^2|^2\right)\\&+\frac{8m_l^2}{q^2}N^2\left(|H_0^1|^2-|H_0^2|^2+2|H_t^2|^2\right),\end{aligned} $

(37) $ I^{\rho}_{2s} = \frac{\beta_l^2}{2}N^2\left(|H_+^1|^2+|H_+^2|^2+|H_-^1|^2+|H_-^2|^2\right), $

(38) $ I^{\rho}_{2c} = -2\beta_l^2N^2\left(|H_0^1|^2+|H_0^2|^2\right), $

(39) $ I^{\rho}_{3}=-2\beta_l^2N^2\left[{\cal{R}}e\left(H_+^{1}H_-^{1\ast}+H_+^{2}H_-^{2\ast}\right)\right], $

(40) $\begin{aligned}[b] I^{\rho}_{4}=\;&\beta_l^2N^2\Big[{\cal{R}}e\left(H_+^{1}H_0^{1\ast}+H_-^{1}H_0^{1\ast}\right) \\&+{\cal{R}}e\left(H_+^{2}H_0^{2\ast}+H_-^{2}H_0^{2\ast}\right)\Big],\end{aligned} $

(41) $\begin{aligned}[b] I^{\rho}_{5}=\;&-2\beta_lN^2\Big[{\cal{R}}e\left(H_+^{1}H_0^{2\ast}-H_-^{1}H_0^{2\ast}\right) \\&+{\cal{R}}e\left(H_+^{2}H_0^{1\ast}-H_-^{2}H_0^{1\ast}\right)\Big], \end{aligned}$

(42) $ I^{\rho}_{6s}=-4\beta_lN^2\left[{\cal{R}}e\left(H_+^{1}H_+^{2\ast}-H_-^{1}H_-^{2\ast}\right)\right], $

(43) $ I^{\rho}_{6c}=0, $

(44) $\begin{aligned}[b] I^{\rho}_{7}=\;&-2\beta_lN^2\Big[{\cal{I}}m\left(H_0^{1}H_+^{2\ast}+H_0^{1}H_-^{2\ast}\right) \\&+{\cal{I}}m\left(H_0^{2}H_+^{1\ast}+H_0^{2}H_-^{1\ast}\right)\Big], \end{aligned}$

(45) $\begin{aligned}[b] I^{\rho}_{8}=\;&\beta_l^2N^2\Big[{\cal{I}}m\left(H_0^{1}H_+^{1\ast}-H_0^{1}H_-^{1\ast}\right) \\&+{\cal{I}}m\left(H_0^{2}H_+^{2\ast}-H_0^{2}H_-^{2\ast}\right)\Big],\end{aligned} $

(46) $ I^{\rho}_{9}=2\beta_l^2N^2\left[{\cal{I}}m\left(H_+^{1}H_-^{1\ast}+H_+^{2}H_-^{2\ast}\right)\right], $

(47) For the decay
$ B\to a_{1}\,(\to \rho_{\|(\perp)}\pi)\mu^+\mu^- $ , the four fold distribution can be expressed as$ \begin{aligned}[b]&{\frac{{\rm d}^4\Gamma\left(B\to a_{1}\,(\to \rho_{\|(\perp)}\pi)\mu^+\mu^-\right)}{{\rm d} q^2 \ {\rm d}\cos{\theta_{l}} \ {\rm d}\cos {\theta}_{V} \ {\rm d}\phi}}\\ =\;&{ \frac{9}{32 \pi} {\cal{B}}(a_{1}\to \rho_{\|(\perp)}\pi) } \\ &{\times\left[I^{a_{1}}_{1s,\|(\perp)}\sin^2\theta_{V}+I^{a_{1}}_{1c,\|(\perp)}\cos^2\theta_{V}\right.}\\ &{+\left(I^{a_{1}}_{2s,\|(\perp)}\sin^2\theta_{V}+I^{a_{1}}_{2c,\|(\perp)}\cos^2\theta_{V}\right)\cos{2\theta_{l}}} \\ &{+\left(I^{a_{1}}_{6s,\|(\perp)}\sin^2\theta_{V}+I^{a_{1}}_{6c,\|(\perp)}\cos^2\theta_{V}\right)\cos{\theta_{l}}}\\ &{+\left(I^{a_{1}}_{3,\|(\perp)}\cos{2\phi} +I^{a_{1}}_{9,\|(\perp)}\sin{2\phi}\right)\sin^2\theta_{V}\sin^2\theta_{l}} \\ &{+\left(I^{a_{1}}_{4,\|(\perp)}\cos{\phi}+I^{a_{1}}_{8,\|(\perp)}\sin{\phi}\right)\sin2\theta_{V}\sin2\theta_{l}} \\ &{\left.+\left(I^{a_{1}}_{5,\|(\perp)}\cos{\phi}+I^{a_{1}}_{7,\|(\perp)}\sin{\phi}\right)\sin2\theta_{V}\sin\theta_{l}\right].}\end{aligned} $

(48) where
$ I^{a_{1}}_{n\lambda,\|} $ and$ I_{n\lambda,\perp}^{a_{1}} $ are the angular coefficients. The explicit expressions of$ I^{a_{1}}_{n\lambda,\|} $ in terms of the helicity amplitudes can be expressed as$ \begin{aligned}[b] {I^{a_{1}}_{1s,\|} }=\;&{ \frac{(2+\beta_l^2)}{2}N^2\left(|H_+^1|^2+|H_+^2|^2+|H_-^1|^2+|H_-^2|^2\right) }\\&{+\frac{4m_l^2}{q^2}N^2\left(|H_+^1|^2-|H_+^2|^2+|H_-^1|^2-|H_-^2|^2\right),}\end{aligned} $

(49) $\begin{aligned}[b] I^{a_{1}}_{1c,\|} =\;& 2N^2\left(|H_0^1|^2+|H_0^2|^2\right)\\&+\frac{8m_l^2}{q^2}N^2\left(|H_0^1|^2-|H_0^2|^2+2|H_t^2|^2\right),\end{aligned} $

(50) $ I^{a_{1}}_{2s,\|} = \frac{\beta_l^2}{2}N^2\left(|H_+^1|^2+|H_+^2|^2+|H_-^1|^2+|H_-^2|^2\right), $

(51) $ I^{a_{1}}_{2c,\|} = -2\beta_l^2N^2\left(|H_0^1|^2+|H_0^2|^2\right), $

(52) $ I^{a_{1}}_{3,\|}=-2\beta_l^2N^2\left[{\cal{R}}e\left(H_+^{1}H_-^{1\ast}+H_+^{2}H_-^{2\ast}\right)\right], $

(53) $\begin{aligned}[b] I^{a_{1}}_{4,\|}=\;&\beta_l^2N^2\Big[{\cal{R}}e\left(H_+^{1}H_0^{1\ast}+H_-^{1}H_0^{1\ast}\right) \\&+{\cal{R}}e\left(H_+^{2}H_0^{2\ast}+H_-^{2}H_0^{2\ast}\right)\Big],\end{aligned} $

(54) $\begin{aligned}[b] I^{a_{1}}_{5,\|}=\;&-2\beta_lN^2\Big[{\cal{R}}e\left(H_+^{1}H_0^{2\ast}-H_-^{1}H_0^{2\ast}\right) \\&+{\cal{R}}e\left(H_+^{2}H_0^{1\ast}-H_-^{2}H_0^{1\ast}\right)\Big],\end{aligned} $

(55) $ I^{a_{1}}_{6s,\|}=-4\beta_lN^2\left[{\cal{R}}e\left(H_+^{1}H_+^{2\ast}-H_-^{1}H_-^{2\ast}\right)\right], $

(56) $ I^{a_{1}}_{6c,\|}=0, $

(57) $\begin{aligned}[b] I^{a_{1}}_{7,\|}=\;&-2\beta_lN^2\Big[{\cal{I}}m\left(H_0^{1}H_+^{2\ast}+H_0^{1}H_-^{2\ast}\right) \\&+{\cal{I}}m\left(H_0^{2}H_+^{1\ast}+H_0^{2}H_-^{1\ast}\right)\Big],\end{aligned} $

(58) $\begin{aligned}[b] I^{a_{1}}_{8,\|}=\;&\beta_l^2N^2\Big[{\cal{I}}m\left(H_0^{1}H_+^{1\ast}-H_0^{1}H_-^{1\ast}\right) \\&+{\cal{I}}m\left(H_0^{2}H_+^{2\ast}-H_0^{2}H_-^{2\ast}\right)\Big],\end{aligned} $

(59) $ I^{a_{1}}_{9,\|}=2\beta_l^2N^2\left[{\cal{I}}m\left(H_+^{1}H_-^{1\ast}+H_+^{2}H_-^{2\ast}\right)\right], $

(60) whereas the expressions of
$ I_{n\lambda,\perp}^{a_{1}} $ in terms of the helicity amplitudes are expressed as$\begin{aligned}[b] {I^{a_{1}}_{1s,\perp}} =\;&\frac{(2+\beta_l^2)}{4}N^2\left(|H_+^1|^2+|H_+^2|^2+|H_-^1|^2+|H_-^2|^2\right)\\&+\left(|H_0^1|^2+|H_0^2|^2\right)\\ &+\frac{2m_l^2}{q^2}N^2\Big[\left(|H_+^1|^2-|H_+^2|^2+|H_-^1|^2-|H_-^2|^2\right) \\&+2\left(|H_0^1|^2-|H_0^2|^2+2|H_t^2|^2\right)\Big], \end{aligned} $

(61) $ \begin{aligned}[b]{I^{a_{1}}_{1c,\perp}}=\; &{ \frac{(2+\beta_l^2)}{2}N^2\left(|H_+^1|^2+|H_+^2|^2+|H_-^1|^2+|H_-^2|^2\right)} \\ &{+\frac{4m_l^2}{q^2}N^2\left(|H_+^1|^2-|H_+^2|^2+|H_-^1|^2-|H_-^2|^2\right),} \end{aligned} $

(62) $\begin{aligned}[b] I^{a_{1}}_{2s,\perp} =\;& -\beta_l^2N^2\Big[\left(|H_0^1|^2+|H_0^2|^2\right)\\&-\frac{1}{4}\left(|H_+^1|^2+|H_+^2|^2+|H_-^1|^2+|H_-^2|^2\right)\Big] ,\end{aligned} $

(63) $ I^{a_{1}}_{2c,\perp} = \frac{\beta_l^2}{2}N^2\left(|H_+^1|^2+|H_+^2|^2+|H_-^1|^2+|H_-^2|^2\right), $

(64) $ I^{a_{1}}_{3,\perp}=\beta_l^2N^2\left[{\cal{R}}e\left(H_+^{1}H_-^{1\ast}+H_+^{2}H_-^{2\ast}\right)\right], $

(65) $ \begin{aligned}[b]I^{a_{1}}_{4,\perp}=\;&-\frac{\beta_l^2}{2}N^2\Big[{\cal{R}}e\left(H_+^{1}H_0^{1\ast}+H_-^{1}H_0^{1\ast}\right)\\& +{\cal{R}}e\left(H_+^{2}H_0^{2\ast}+H_-^{2}H_0^{2\ast}\right)\Big],\end{aligned} $

(66) $\begin{aligned}[b] I^{a_{1}}_{5,\perp}=\;&\beta_lN^2\Big[{\cal{R}}e\left(H_+^{1}H_0^{2\ast}-H_-^{1}H_0^{2\ast}\right) \\&+{\cal{R}}e\left(H_+^{2}H_0^{1\ast}-H_-^{2}H_0^{1\ast}\right)\Big],\end{aligned} $

(67) $ I^{a_{1}}_{6s,\perp}=-2\beta_lN^2\left[{\cal{R}}e\left(H_+^{1}H_+^{2\ast}-H_-^{1}H_-^{2\ast}\right)\right], $

(68) $ I^{a_{1}}_{6c,\perp}=-4\beta_lN^2\left[{\cal{R}}e\left(H_+^{1}H_+^{2\ast}-H_-^{1}H_-^{2\ast}\right)\right], $

(69) $\begin{aligned}[b] I^{a_{1}}_{7,\perp}=\;&\beta_lN^2\Big[{\cal{I}}m\left(H_0^{1}H_+^{2\ast}+H_0^{1}H_-^{2\ast}\right) \\&+{\cal{I}}m\left(H_0^{2}H_+^{1\ast}+H_0^{2}H_-^{1\ast}\right)\Big],\end{aligned} $

(70) $\begin{aligned}[b] I^{a_{1}}_{8,\perp}=\;&-\frac{\beta_l^2}{2}N^2\Big[{\cal{I}}m\left(H_0^{1}H_+^{1\ast}-H_0^{1}H_-^{1\ast}\right)\\& +{\cal{I}}m\left(H_0^{2}H_+^{2\ast}-H_0^{2}H_-^{2\ast}\right)\Big],\end{aligned} $

(71) $ I^{a_{1}}_{9,\perp}=-\beta_l^2N^2\left[{\cal{I}}m\left(H_+^{1}H_-^{1\ast}+H_+^{2}H_-^{2\ast}\right)\right], $

(72) where
$ N=V_{tb}V^{\ast}_{td}\left[\frac{G_{F}^2\alpha^2}{3.2^{10} \pi^5 m_{B}^{3}} q^2\sqrt{\lambda}\beta_l\right]^{1/2}, $

(73) with
$ \lambda\equiv \lambda(m^2_{B}, m^2_{M}, q^2) $ and$ \beta_l=\sqrt{1-4m_l^2/q^2} $ . -
In this section, we provide the expressions of the physical observables, such as the differential decay rate, lepton forward-backward asymmetry, longitudinal helicity fraction of
$ \rho $ , and the normalized angular observables$ \langle I^{\rho}_{n\lambda}\rangle $ , for$ B\to\rho(\to\pi\pi)\mu^{+}\mu^{-} $ decay.(i) Differential decay rate: From the full angular distribution Eq. (35), integration over
$ \cos\theta_l = [-1, 1] $ ,$ \cos\theta_V = [-1, 1] $ , and$ \phi = [0,2\pi] $ yields the$ q^{2} $ dependent differential decay rate expression, which in terms of the angular coefficients is as follows:$ \frac{{\rm d}\Gamma \left(B\to\rho(\to \pi\pi)\mu^+\mu^-\right)}{{\rm d} q^{2}}={\cal{B}}(\rho\to \pi\pi)\frac{1}{4}(3I^{\rho}_{1c}+6I^{\rho}_{1s}-I^{\rho}_{2c}-2I^{\rho}_{2s}). $

(74) (ii) Lepton forward-backward asymmetry: From the full angular distribution Eq. (35), the integration over
$ \cos\theta_V = [-1, 1] $ and$ \phi = [0,2\pi] $ gives the double differential decay rate$\left(\dfrac{{\rm d}^{2}\Gamma}{{\rm d}q^{2}{\rm d}\cos\theta_{\ell}}\right)$ . The lepton forward-backward asymmetry corresponding to$ \theta_\ell $ is$A_{{\rm{FB}}} = (F-B)/ (F+B)$ , where F and B are the forward and backward hemispheres, respectively. The forward-backward asymmetry for$ B\to\rho\mu^{+}\mu^{-} $ decay can be obtained by integrating$\dfrac{{\rm d}^{2}\Gamma}{{\rm d}q^{2}{\rm d}\cos\theta_{\ell}}$ , and it can be defined as$ A_{{\rm{FB}}}^{\rho}(q^{2})=\frac{\displaystyle\int_{0}^{1}\dfrac{{\rm d}^{2}\Gamma}{{\rm d}q^{2}{\rm d}\cos\theta_{\ell}}{\rm d}\cos\theta_{\ell}-\int_{-1}^{0}\dfrac{{\rm d}^{2}\Gamma}{{\rm d}q^{2}{\rm d}\cos\theta_{\ell}}{\rm d}\cos\theta_{\ell}}{\displaystyle\int_{-1}^{1}\dfrac{{\rm d}^{2}\Gamma}{{\rm d}q^{2}{\rm d}\cos\theta_{\ell}}{\rm d}\cos\theta_{\ell}}. $

(75) In terms of angular coefficients
$ I's $ , the lepton forward-backward asymmetry for$ B\to\rho(\to\pi\pi)\mu^{+}\mu^{-} $ as a function of$ q^{2} $ can be expressed as$ A_{{\rm{FB}}}^{\rho}(q^{2})=\frac{6I^{\rho}_{6s}}{2(3I^{\rho}_{1c}+6I^{\rho}_{1s}-I^{\rho}_{2c}-2I^{\rho}_{2s})}. $

(76) (iii) Longitudinal helicity fraction: Based on the full angular distribution Eq. (35), the integration over
$ \cos\theta_l = [-1, 1] $ and$ \phi = [0,2\pi] $ leads to the double differential decay rate$\left(\dfrac{{\rm d}^{2}\Gamma}{{\rm d}q^{2}{\rm d}\cos\theta_{V}}\right)$ . The longitudinal helicity fraction of the decay$ B\to \rho(\to \pi\pi)\mu^{+}\mu^{-} $ , when$ \rho $ meson is longitudinally polarized, can be defined as$ f_{L}^{\rho}(q^{2})=\frac{\displaystyle\int_{-1}^{1}\dfrac{{\rm d}^{2}\Gamma}{{\rm d}q^{2}{\rm d}\cos\theta_{V}}\left(\frac{5}{2}\cos^2\theta_V-\frac{1}{2}\right) {\rm d}\cos\theta_{V}}{{{\rm d}\Gamma \left(B\to\rho(\to \pi\pi)\mu^+\mu^-\right)}/{{\rm d}q^{2}}}. $

(77) In terms of the angular coefficients
$ I's(q^{2}) $ , the longitudinal helicity fraction for the decay$ B\to \rho(\to \pi\pi)\mu^{+}\mu^{-} $ can be expressed as$ f_{L}^{\rho}(q^{2})=\frac{3I^{\rho}_{1c}-I^{\rho}_{2c}}{3I^{\rho}_{1c}+6I^{\rho}_{1s}-I^{\rho}_{2c}-2I^{\rho}_{2s}}. $

(78) (iv) Normalized angular observables:
$ \langle I_{n\lambda}^{\rho}\rangle=\frac{{\cal{B}}(\rho\to \pi\pi)I_{n\lambda}^{\rho}}{{\rm d}\Gamma \left(B\to\rho(\to \pi\pi)\mu^+\mu^-\right)/{\rm d}q^{2}}. $

(79) (v) Binned normalized angular observables:
$ \langle I_{n\lambda}^{\rho}\rangle_{\left[q^{2}_{{\rm{min}}},q^{2}_{{\rm{max}}}\right]}=\frac{\displaystyle\int^{q^{2}_{{\rm{max}}}}_{q^{2}_{{\rm{min}}}}{\cal{B}}(\rho\to \pi\pi)I_{n\lambda}^{\rho}\,{\rm d}q^2}{\displaystyle\int^{q^{2}_{{\rm{max}}}}_{q^{2}_{{\rm{min}}}}({\rm d}\Gamma \left(B\to\rho(\to \pi\pi)\mu^+\mu^-\right)/{\rm d}q^{2}){\rm d}q^2}. $

(80) -
The formulas of physical observables for
$B\to a_{1}(\to\rho_{||,\perp}\pi)\mu^{+}\mu^{-}$ decay can be expressed as(i) Differential decay rates:
$ \begin{aligned}[b]&\frac{{\rm d}\Gamma \left(B\to a_{1}(\to\rho_{||}\pi)\mu^+\mu^-\right)}{{\rm d}q^{2}}\\=\;&{\cal{B}}(a_{1}\to\rho_{||}\pi)\frac{1}{4}(3I^{a_{1}}_{1c,||}+6I^{a_{1}}_{1s,||}-I^{a_{1}}_{2c,||}-2I^{a_{1}}_{2s,||}).\end{aligned} $

(81) $ \begin{aligned}[b]&\frac{{\rm d}\Gamma \left(B\to a_{1}(\to\rho_{\perp}\pi)\mu^+\mu^-\right)}{{\rm d}q^{2}}\\=\;&{\cal{B}}(a_{1}\to\rho_{\perp}\pi)\frac{1}{4}(3I^{a_{1}}_{1c,\perp}+6I^{a_{1}}_{1s,\perp}-I^{a_{1}}_{2c,\perp}-2I^{a_{1}}_{2s,\perp}). \end{aligned} $

(82) $ \begin{aligned}[b]&\frac{{\rm d}\Gamma \left(B\to a_{1}(\to\rho\pi)\mu^+\mu^-\right)}{{\rm d}q^{2}}\\=\;& \frac{{\rm d}\Gamma \left(B\to a_{1}(\to\rho_{||}\pi)\mu^+\mu^-\right)}{{\rm d}q^{2}}\\&+\frac{{\rm d}\Gamma \left(B\to a_{1}(\to\rho_{\perp}\pi)\mu^+\mu^-\right)}{{\rm d}q^{2}}. \end{aligned} $

(83) (ii) Lepton forward-backward asymmetry: Based on the full angular distribution Eq. (48), the integration over
$ \cos\theta_V = [-1, 1] $ and$ \phi = [0,2\pi] $ provides the double differential decay rate$\left(\dfrac{{\rm d}^{2}\Gamma_{\parallel(\perp)}}{{\rm d}q^{2}{\rm d}\cos\theta_{\ell}}\right)$ , where$\Gamma_{\parallel(\perp)}\equiv \Gamma (B \to a_{1}(\to \rho_{\parallel(\perp)}\pi)\mu^{+}\mu^{-})$ . The lepton forward-backward asymmetry corresponding to$ \theta_\ell $ can be obtained from these polarized double differential decay rates as$\begin{aligned}[b] A_{{\rm{FB}}}^{a_{1}}\left(q^2\right) \equiv\;& \Bigg[\int_{0}^1 {\rm d}\cos\theta_\ell\frac{{\rm d}^2\Gamma_{\parallel(\perp)}}{{\rm d}q^2 {\rm d}\cos\theta_{\ell}}\\&-\int_{-1}^0 {\rm d}\cos\theta_\ell\frac{{\rm d}^2\Gamma_{\parallel(\perp)}}{{\rm d} q^2 {\rm d}\cos\theta_{\ell}}\Bigg]\bigg/{\frac{{\rm d}\Gamma_{\parallel(\perp)}}{{\rm d} q^2}}, \end{aligned}$

(84) which in terms of the angular coefficient functions can be expressed as
$ \begin{aligned}[b]A_{{\rm{FB}}}^{a_{1}}(q^{2})=\;&\frac{3\left(I^{a_{1}}_{6c,\perp}+2I^{a_{1}}_{6s,\perp}\right)}{2(3I^{a_{1}}_{1c,\perp}+6I^{a_{1}}_{1s,\perp}-I^{a_{1}}_{2c,\perp}-2I^{a_{1}}_{2s,\perp})}\\=\;&\frac{6I^{a_{1}}_{6s,||}}{2(3I^{a_{1}}_{1c,||}+6I^{a_{1}}_{1s,||}-I^{a_{1}}_{2c,||}-2I^{a_{1}}_{2s,||})}.\end{aligned} $

(85) (iii) Longitudinal helicity fraction: Based on the full angular distribution Eq. (48), the integration over
$\cos\theta_l = [-1, 1]$ and$ \phi = [0,2\pi] $ leads to the double differential decay rate$\left(\dfrac{{\rm d}^{2}\Gamma_{\parallel(\perp)}}{{\rm d}q^{2}{\rm d}\cos\theta_{V}}\right)$ . The longitudinal helicity fraction of decay$ B\to a_{1}\mu^{+}\mu^{-} $ , when$ a_{1} $ meson is longitudinally polarized, can be defined as$\begin{aligned}[b] f_{L}^{a_1}(q^{2})=\;&\frac{\displaystyle\int_{-1}^{1}\dfrac{{\rm d}^{2}\Gamma_{||}}{{\rm d}q^{2}{\rm d}\cos\theta_{V}}\left(\dfrac{5}{2}\cos^2\theta_V-\dfrac{1}{2}\right){\rm d}\cos\theta_{V}}{{{\rm d}\Gamma \left(B\to a_{1}(\to\rho_{||}\pi)\mu^+\mu^-\right)}/{{\rm d}q^{2}}}\\=\;&\frac{\displaystyle\int_{-1}^{1}\dfrac{{\rm d}^{2} \Gamma_{\perp}}{{\rm d}q^{2}{\rm d}\cos\theta_{V}}\left(2-5\cos^2\theta_V\right){\rm d}\cos\theta_{V}}{{{\rm d}\Gamma \left(B\to a_{1}(\to\rho_{\perp}\pi)\mu^+\mu^-\right)}/{{\rm d}q^{2}}},\end{aligned} $

(86) which in terms of the angular coefficient functions is given by
$\begin{aligned}[b] f_{L}^{a_1}(q^{2})=\;&\frac{3I^{a_{1}}_{1c,||}-I^{a_{1}}_{2c,||}}{3I^{a_{1}}_{1c,||}+6I^{a_{1}}_{1s,||}-I^{a_{1}}_{2c,||}-2I^{a_{1}}_{2s,||}}\\=\;&\frac{(6I^{a_{1}}_{1s,\perp}-2I^{a_{1}}_{2s,\perp})-(3I^{a_{1}}_{1c,\perp}-I^{a_{1}}_{2c,\perp})}{3I^{a_{1}}_{1c,\perp}+6I^{a_{1}}_{1s,\perp}-I^{a_{1}}_{2c,\perp}-2I^{a_{1}}_{2s,\perp}}.\end{aligned} $

(87) (iv) Normalized angular observables: We introduce the normalized angular observables:
$ \langle \widehat{I}^{a_{1}}_{n\lambda, \parallel\left(\perp\right)}\rangle = \frac{{\cal{B}}(a_{1}\to \rho_{\|(\perp)}\pi)I^{a_1}_{n\lambda,\parallel\left(\perp\right)}}{{\rm d}\Gamma\left(B\to a_{1}(\to\rho\pi)\mu^+\mu^-\right)/{\rm d}q^2}. $

(88) (v) Binned normalized angular observables:
$ \langle \widehat{I}^{a_{1}}_{n\lambda, \parallel\left(\perp\right)}\rangle_{\left[q^{2}_{{\rm{min}}},q^{2}_{{\rm{max}}}\right]}=\frac{\int^{q^{2}_{{\rm{max}}}}_{q^{2}_{{\rm{min}}}}{\cal{B}}(a_{1}\to \rho_{\|(\perp)}\pi)I^{a_1}_{n\lambda,\parallel\left(\perp\right)}\,{\rm d}q^2}{\int^{q^{2}_{{\rm{max}}}}_{q^{2}_{{\rm{min}}}}({\rm d}\Gamma\left(B\to a_{1}(\to\rho\pi)\mu^+\mu^-\right)/{\rm d}q^2){\rm d}q^2}. $

(89) To compute the branching ratios
$ {\cal{B}}(a_{1}\to \rho_{\|(\perp)}\pi) $ provided in Eq. (89), one needs the amplitude of the decay, whose expression is as follows [62]:$ \langle\rho(p_{\rho},\eta)\pi(p_{\pi})|a_{1}(k,\overline\epsilon)\rangle=g_{1}(\overline\epsilon.\eta)(k.p_{\rho})+g_{2}(\overline\epsilon.p_{\rho})(k.\eta), $

(90) where
$ g_{1},g_{2} $ are strong coupling constants, and$ \overline\epsilon $ and$ \eta $ are the polarizations of$ a_{1} $ and$ \rho $ mesons, respectively.The form of
$ {\cal{B}}(a_{1}\to \rho_{\|(\perp)}\pi) $ for longitudinal and transverse$ \rho $ meson can be expressed as$ {\cal{B}}(a_{1}\to \rho_{\|(\perp)}\pi)=\frac{1}{\Gamma_{a_{1}}}\frac{|\vec{p}_{\rho}|}{24\pi m^{2}_{a_{1}}}\Gamma_{\|(\perp)}, $

(91) where
$ |\vec{p}_{\rho}|=\dfrac{1}{2m_{a_{1}}}\sqrt{\lambda(m^{2}_{a_{1}},m^{2}_{\rho},m^{2}_{\pi})} $ , and$ \Gamma_{\|}=\frac{m^{2}_{a_{1}}}{m^{2}_{\rho}}[(m^{2}_{\rho}+|\vec{p}_{\rho}|^{2})g_{1}+|\vec{p}_{\rho}|^{2}g_{2}]^{2}, $

(92) $ \Gamma_{\perp}=2g^{2}_{1}m^{2}_{a_{1}}|\vec{p}_{\rho}|^{2}\left(1+\frac{m^{2}_{\rho}}{|\vec{p}_{\rho}|^{2}}\right). $

(93) Coupling constants
$ g_{1} $ and$ g_{2} $ can be related through the amplitude provided in [63]:$ \langle\rho(p_{\rho},\eta)\pi(p_{\pi})|a_{1}(k,\overline\epsilon)\rangle=-\frac{2\lambda_{a_{1}\rho\pi}}{m_{\rho}m_{a_{1}}}\left[(k.p_{\rho})(\overline\epsilon.\eta)-(\overline\epsilon.p_{\rho})(k.\eta)\right] $

(94) Comparing Eq. (90), and (94) gives
$g_{1}=-g_{2}=\dfrac{2\lambda_{a_{1}\rho\pi}}{m_{\rho}m_{a_{1}}}$ . Using the numerical values of masses from [64],$ {\cal{B}}(a_{1}\to \rho_{\|}\pi)=17.2 $ % and$ {\cal{B}}(a_{1}\to \rho_{\perp}\pi)=43 $ % can be obtained. These values of branching ratios are used to analyze the above mentioned physical observables. -
To analyze the signatures of family non-universal
$ Z^{\prime} $ gauge boson in the observables that belong to$ B\to (\rho,a_{1})\mu^{+}\mu^{-} $ decays, we use input parameters such as hadronic transition form factors, which are calculated in the framework of light cone sum rules (LCSR) for the case of$ B\to\rho(\pi\pi)\mu^{+}\mu^{-} $ decay [46] and in perturbative QCD (pQCD) approach for the case of$ B\to a_{1}(\to\rho\pi)\mu^{+}\mu^{-} $ decay [47].The combined fit of the simplified series expansion (SSE) parametrization to LCSR results for
$ B\to \rho(\pi\pi)\mu^{+}\mu^{-} $ are as follows [46]:$ z(t)=\frac{\sqrt{t_+-t}-\sqrt{t_+-t_{0}}}{\sqrt{t_+-t}+\sqrt{t_+-t_{0}}}, $

(95) where
$ t_{\pm}\equiv (m_{B}\pm m_{\rho})^{2} $ and$ t_{0}\equiv t_{+}\left(1-\sqrt{1-\dfrac{t_{-}}{t_{+}}}\right) $ . We can write the expressions of the transition form factors for the decay$ B\to\rho $ as$ F_{i}(q^{2})=P_{i}(q^{2})\sum\limits_{k}\alpha^{i}_{k}[z(q^{2})-z(0)]^{k}, $

(96) where
$ P_{i}(q^{2})=\dfrac{1}{(1-\frac{q^{2}}{m^{2}_{R,i}})} $ is a simple pole corresponding to the first resonance in the spectrum. The resonance masses and the fit results for the SSE expansion coefficients in the fit to the LCSR computation for the decay$ B\to\rho $ are presented in Table 1 and Table 2.$ F_{i} $ 

$ J^{P} $ 

$ m^{b\to d}_{R,i} $ 

$ A_{0} $ 

$ 0^{-} $ 

$ 5.279 $ 

$ T_{1},V $ 

$ 1^{-} $ 

$ 5.325 $ 

$ T_{2},T_{23},A_{1},A_{12} $ 

$ 1^{+} $ 

$ 5.274 $ 

Table 1. Masses of resonances of quantum numbers
$ J^{P} $ as represented for the parametrization of form factors$ F_{i} $ for$ b\to d $ transition.$ A_{0} $ 

$ A_{1} $ 

$ A_{12} $ 

V $ T_{1} $ 

$ T_{2} $ 

$ T_{23} $ 

$ \alpha_{0} $ 

$ 0.36\pm 0.04 $ 

$ 0.26\pm 0.03 $ 

$ 0.30\pm 0.03 $ 

$ 0.33\pm 0.03 $ 

$ 0.27\pm 0.03 $ 

$ 0.27\pm 0.03 $ 

$ 0.75\pm 0.08 $ 

$ \alpha_{1} $ 

$ -0.83\pm 0.20 $ 

$ 0.39\pm 0.14 $ 

$ 0.76\pm 0.20 $ 

$ -0.86\pm 0.18 $ 

$ -0.74\pm 0.14 $ 

$ 0.47\pm 0.13 $ 

$ 1.90\pm 0.43 $ 

$ \alpha_{2} $ 

$ 1.33\pm 1.05 $ 

$ 0.16\pm 0.41 $ 

$ 0.46\pm 0.76 $ 

$ 1.80\pm 0.97 $ 

$ 1.45\pm 0.77 $ 

$ 0.58\pm 0.46 $ 

$ 2.93\pm 1.81 $ 

Table 2. Fit results for the SSE expansion coefficients in the fit to the LCSR computation for decay
$ B\to\rho $ [46].For
$ B\to a_{1} $ decay, the transition form factors were calculated in the framework of pQCD approach. The form factors that are involved in$ B\to a_{1} $ decay can be parametrized in the whole kinematical$ q^{2} $ region as follows [47]:$ F(q^{2})=\frac{F(0)}{1-a(q^{2}/m^{2}_{B})+b(q^{2}/m^{2}_{B})^{2}}. $

(97) The numerical results for
$ B\to a_{1} $ decay at$ q^{2}=0 $ , in the pQCD approach, are presented in Table 3. The numerical values of Wilson coefficients in the SM, evaluated at the renormalization scale$ \mu\sim m_{b} $ [65], are presented in Table 4. To analyze the normalized angular observables and other observables, such as differential branching ratios, forward-backward asymmetry, longitudinally polarized final state vector and axial vector mesons in$ B\to\rho(\to\pi\pi)\mu^{+}\mu^{-} $ and$ B\to a_{1}(\to\rho_{\perp,\parallel})\mu^{+}\mu^{-} $ decays, respectively, in the framework of the family non-universal$ Z^{\prime} $ model, the numerical values of$ Z^{\prime} $ model parameters are listed in Table 5.A $ V_{0} $ 

$ V_{1} $ 

$ V_{2} $ 

$ T_{1} $ 

$ T_{2} $ 

$ T_{3} $ 

$ F(0) $ 

$ 0.26^{+0.09}_{-0.09} $ 

$ 0.34^{+0.16}_{-0.17} $ 

$ 0.43^{+0.15}_{-0.15} $ 

$ 0.13^{+0.03}_{-0.04} $ 

$ 0.34^{+0.13}_{-0.13} $ 

$ 0.34^{+0.13}_{-0.13} $ 

$ 0.37^{+0.17}_{-0.12} $ 

a $ 1.72^{+0.05}_{-0.05} $ 

$ 1.73^{+0.05}_{-0.06} $ 

$ 0.75^{+0.05}_{-0.05} $ 

– $ 1.60^{+0.06}_{-0.05} $ 

$ 0.71^{+0.07}_{-0.05} $ 

$ 1.60^{+0.06}_{-0.05} $ 

b $ 0.66^{+0.07}_{-0.06} $ 

$ 0.66^{+0.06}_{-0.08} $ 

$ -0.12^{+0.05}_{-0.02} $ 

– $ 0.53^{+0.06}_{-0.04} $ 

$ -0.16^{+0.03}_{-0.02} $ 

$ 0.53^{+0.06}_{-0.04} $ 

Table 3. Numerical values of transition form factors for
$ B\to a_{1} $ decay at$ q^{2}=0 $ , and the fitted parameters a and b [47].$ C_{1} $ 

$ C_{2} $ 

$ C_{3} $ 

$ C_{4} $ 

$ C_{5} $ 

$ C_{6} $ 

$ C_{7} $ 

$ C_{8} $ 

$ C_{9} $ 

$ C_{10} $ 

$ -0.294 $ 

$ 1.017 $ 

$ -0.0059 $ 

$ -0.087 $ 

$ 0.0004 $ 

$ 0.0011 $ 

$ -0.324 $ 

$ -0.176 $ 

$ 4.114 $ 

$ -4.193 $ 

Table 4. Numerical values of the SM Wilson coefficients up to NNLL accuracy, evaluated at the renormalization scale
$ \mu\sim m_{b} $ [65].Scenarios $ |B^{L}_{db}|\times 10^{-3} $ 

$ \phi_{db} $ in degrees

$ S_{LR} $ 

$ D_{LR} $ 

S1 $ 0.16\pm 0.08 $ 

$ -33\pm 45 $ 

$ 0.08 $ 

$ -0.02 $ 

S2 $ 0.12\pm 0.03 $ 

$ -23\pm 21 $ 

$ 0.08 $ 

$ -0.02 $ 

Table 5. Numerical values of lepton and quark coupling in
$ Z^{\prime} $ model are acquired from Ref. [43].To gauge the effects of family non-universal
$ Z^{\prime} $ model in the aforementioned physical observables, the numerical values of coupling$ B^{L}_{db} $ and weak phase$ \phi_{db} $ presented in Table 5 are fixed, and they are constrained from$ B^{0}_{q}-\bar{B}^{0}_{q} $ mixing [66]. The scenarios S1(S2) listed in Table 5 represents the constraints from UTfit collaboration on parameters$ C_{B_{q}} $ and$ \phi_{B_{q}} $ [67]. The explicit form of$ C_{B_{q}} $ and$ \phi_{B_{q}} $ can be expressed as$ C_{B_{q}} {\rm e}^{2{\rm i}\phi_{B_{q}}}\equiv\frac{\langle B_{q}|H^{{\rm{full}}}_{{\rm{eff}}}|\bar{B}_{q}\rangle}{\langle B_{q}|H^{{\rm{SM}}}_{{\rm{eff}}}|\bar{B}_{q}\rangle} . $

(98) Using the maximum allowed values of the coupling constants provided in Table 5, the numerical values of the non-universal family
$ Z^{\prime} $ model Wilson coefficients$ C_{9}^{Z^{\prime}} $ and$ C_{10}^{Z^{\prime}} $ in scenarios S1 and S2 are presented in Table 6.S1 S2 $ C_{9}^{Z^{\prime}} $ 

0.0000192 0.000012 $ C_{10}^{Z^{\prime}} $ 

$ -4.8\times 10^{-6} $ 

$ -3.0\times 10^{-6} $ 

Table 6. Numerical values of family non-universal
$ Z^{\prime} $ model Wilson coefficients$ C_{9}^{Z^{\prime}} $ and$ C_{10}^{Z^{\prime}} $ in scenarios S1 and S2. -
In this section, we present our phenomenological analysis of the family non-universal
$ Z^{\prime} $ model via physical observables constructed from the combination of different angular coefficients such as the differential branching ratios$\left({\rm d}{\cal{B}}/{\rm d}q^{2}\right)$ , lepton forward-backward asymmetry$ (A_{{\rm{FB}}}) $ , longitudinal polarization fraction$ (f_L) $ of$ \rho $ and$ a_{1} $ mesons, in the$ B\to\rho(\to\pi\pi)\mu^{+}\mu^{-} $ and$ B\to a_{1}(\to\rho_{\parallel, \perp})\mu^{+}\mu^{-} $ decays, respectively. The predicted numerical values of these observables, in different$ q^2 $ bins, for the SM as well as for the two different scenarios of family non-universal$ Z^{\prime} $ model are provided in Tables 7−14 of Appendix B. The listed errors in these tables originate mainly from the uncertainties of the form factors. Furthermore, in Figs. 2−3, we plot the aforementioned physical observables as a function of$ q^{2} $ . The following are our predictions regarding the physical observables.$ q^{2}= 0.1 - 1.0 $ GeV2

Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho\mu^{+}\mu^{-}) \times 10^{+9} $ 

$ 1.889^{+0.339}_{-0.339} $ 

$ 2.410^{+0.497}_{-0.497} $ 

$ 2.176^{+0.430}_{-0.430} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho(\to \pi\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 1.889^{+0.339}_{-0.339} $ 

$ 2.410^{+0.497}_{-0.497} $ 

$ 2.176^{+0.430}_{-0.430} $ 

$ A_{{\rm{FB}}}^{\rho} $ 

$ 0.077^{+0.002}_{-0.002} $ 

$ 0.037^{+0.001}_{-0.001} $ 

$ 0.049^{+0.001}_{-0.001} $ 

$ f_{L}^{\rho} $ 

$ 0.510^{+0.005}_{-0.005} $ 

$ 0.717^{+0.005}_{-0.005} $ 

$ 0.652^{+0.006}_{-0.006} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 11.1295^{+7.3804}_{-7.3804} $ 

$ 19.208^{+12.684}_{-12.684} $ 

$ 15.772^{+1.042}_{-1.042} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\parallel}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 3.1905^{+2.1157}_{-2.1157} $ 

$ 5.506^{+3.636}_{-3.636} $ 

$ 4.521^{+2.989}_{-2989} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\perp}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 7.7907^{+5.1663}_{-5.1663} $ 

$ 13.446^{+8.879}_{-8.879} $ 

$ 11.040^{+7.3001}_{-7.3001} $ 

$ A_{{\rm{FB}}}^{a_{1}} $ 

$ 0.0347^{+0.0005}_{-0.0005} $ 

$ 0.0135^{+0.00005}_{-0.00005} $ 

$ 0.0191^{+0.0002}_{-0.0002} $ 

$ f_{L}^{a_{1}} $ 

$ 0.8022^{+0.0036}_{-0.0036} $ 

$ 0.7952^{+0.0034}_{-0.0034} $ 

$ 0.7910^{+0.0038}_{-0.0038} $ 

Table 7. Predictions of observables in the decay
$ B\to\rho(\to \pi\pi)\mu^{+}\mu^{-} $ , such as differential branching ratios,$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho\mu^{+}\mu^{-}) $ ,$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho(\to \pi\pi)\mu^{+}\mu^{-}) $ , lepton forward-backward asymmetry$ A_{{\rm{FB}}}^{\rho} $ , and longitudinal helicity fraction$ f_{L}^{\rho} $ , and in the decay$ B\to a_{1}(\to\rho\pi)\mu^{+}\mu^{-} $ , such as differential branching ratios$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}\mu^{+}\mu^{-}) $ ,$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}}(B\to a_{1}(\to\rho_{\parallel}\pi)\mu^{+}\mu^{-}) $ ,$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\perp}\pi)\mu^{+}\mu^{-}) $ , lepton forward-backward asymmetry$ A_{{\rm{FB}}}^{a_{1}} $ , and longitudinal helicity fraction$ f_{L}^{a_{1}} $ , in$ q^2 = 0.1 - 1.0 $ GeV2 bin, for the SM as well as the NP scenarios (S1, S2) of$ Z^{\prime} $ model listed in Table 5. The errors presented mainly arise from the uncertainties of the form factors.$ q^{2}= 1.0 - 2.0 $ GeV2

Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho\mu^{+}\mu^{-}) \times 10^{+9} $ 

$ 0.856^{+0.166}_{-0.166} $ 

$ 1.462^{+0.279}_{-0.279} $ 

$ 1.188^{+0.275}_{-0.275} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho(\to \pi\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 0.856^{+0.166}_{-0.166} $ 

$ 1.462^{+0.279}_{-0.279} $ 

$ 1.188^{+0.275}_{-0.275} $ 

$ A_{\text{FB}}^{\rho} $ 

$ 0.100^{+0.002}_{-0.002} $ 

$ 0.015^{+0.001}_{-0.001} $ 

$ 0.035^{+0.001}_{-0.001} $ 

$ f_{L}^{\rho} $ 

$ 0.840^{+0.001}_{-0.001} $ 

$ 0.968^{+0.001}_{-0.001} $ 

$ 0.943^{+0.001}_{-0.001} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 8.1566^{+5.3824}_{-5.3824} $ 

$ 15.5392^{+10.2335}_{-10.2335} $ 

$ 12.330^{+8.125}_{-8.125} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\parallel}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 2.3382^{+1.5430}_{-1.5430} $ 

$ 4.4546^{+2.9336}_{-2.9336} $ 

$ 3.534^{+2.329}_{-2.329} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\perp}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 5.7096^{+3.7677}_{-3.7677} $ 

$ 10.8774^{+7.1634}_{-7.1634} $ 

$ 8.629^{+5.687}_{-5.687} $ 

$ A_{\text{FB}}^{a_{1}} $ 

$ 0.0357^{+0.0004}_{-0.0004} $ 

$ 0.0075^{+0.0006}_{-0.0006} $ 

$ 0.0140^{+0.0006}_{-0.0006} $ 

$ f_{L}^{a_{1}} $ 

$ 0.9479^{+0.0008}_{-0.0008} $ 

$ 0.9533^{+0.0062}_{-0.0062} $ 

$ 0.9492^{+0.0011}_{-0.0011} $ 

Table 8. Same as that in Table 7 but for
$ q^2 = 1.0 - 2.0 $ GeV2 bin.$ q^{2}= 2.0 - 3.0 $ GeV2

Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho\mu^{+}\mu^{-}) \times 10^{+9} $ 

$ 0.789^{+0.146}_{-0.146} $ 

$ 1.443^{+0.266}_{-0.266} $ 

$ 1.147^{+0.212}_{-0.212} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho(\to \pi\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 0.789^{+0.146}_{-0.146} $ 

$ 1.443^{+0.266}_{-0.266} $ 

$ 1.147^{+0.212}_{-0.212} $ 

$ A_{\text{FB}}^{\rho} $ 

$ 0.048^{+0.002}_{-0.002} $ 

$ 0.033^{+0.001}_{-0.001} $ 

$ 0.019^{+0.001}_{-0.001} $ 

$ f_{L}^{\rho} $ 

$ 0.880^{+0.001}_{-0.001} $ 

$ 0.949^{+0.000}_{-0.000} $ 

$ 0.942^{+0.000}_{-0.000} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 7.5818^{+4.9957}_{-4.9957} $ 

$ 14.6876^{+9.6632}_{-9.6632} $ 

$ 11.570^{+7.618}_{-7.618} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\parallel}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 2.1735^{+1.4321}_{-1.4321} $ 

$ 4.2105^{+2.7701}_{-2.7701} $ 

$ 3.317^{+2.1837}_{-2.1837} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\perp}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 5.3073^{+3.4970}_{-3.4970} $ 

$ 10.2813^{+6.7642}_{-6.7642} $ 

$ 8.0399^{+5.3320}_{-5.3320} $ 

$ A_{\text{FB}}^{a_{1}} $ 

$ 0.0230^{+0.0014}_{-0.0014} $ 

$ -0.0036^{+0.0012}_{-0.0012} $ 

$ 0.0015^{+0.0014}_{-0.0014} $ 

$ f_{L}^{a_{1}} $ 

$ 0.9484^{+0.0012}_{-0.0012} $ 

$ 0.9584^{+0.0088}_{-0.0088} $ 

$ 0.9539^{+0.0010}_{-0.0010} $ 

Table 9. Same as that in Table 7 but for
$ q^2 = 2.0 - 3.0 $ GeV2 bin.$ q^{2}= 3.0 - 4.0 $ GeV2

Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho\mu^{+}\mu^{-}) \times 10^{+9} $ 

$ 0.797^{+0.143}_{-0.143} $ 

$ 1.503^{+0.269}_{-0.269} $ 

$ 1.212^{+0.212}_{-0.212} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho(\to \pi\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 0.797^{+0.143}_{-0.143} $ 

$ 1.503^{+0.269}_{-0.269} $ 

$ 1.212^{+0.212}_{-0.212} $ 

$ A_{\text{FB}}^{\rho} $ 

$ -0.012^{+0.002}_{-0.002} $ 

$ -0.078^{+0.001}_{-0.001} $ 

$ -0.072^{+0.002}_{-0.002} $ 

$ f_{L}^{\rho} $ 

$ 0.8501^{+0.0003}_{-0.0003} $ 

$ 0.893^{+0.0005}_{-0.0005} $ 

$ 0.8931^{+0.0004}_{-0.0004} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 7.2480^{+4.7714}_{-4.7714} $ 

$ 14.1509^{+9.3042}_{-9.3042} $ 

$ 11.600^{+7.618}_{-7.618} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\parallel}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 2.0778^{+1.3678}_{-1.3678} $ 

$ 4.0566^{+2.6672}_{-2.6672} $ 

$ 3.184^{+2.090}_{-2.090} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\perp}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 5.0736^{+3.3400}_{-3.3400} $ 

$ 9.9056^{+6.5129}_{-6.5129} $ 

$ 7.775^{+5.115}_{-5.115} $ 

$ A_{\text{FB}}^{a_{1}} $ 

$ 0.0079^{+0.0023}_{-0.0023} $ 

$ -0.0157^{+0.0017}_{-0.0017} $ 

$ 0.0125^{+0.0020}_{-0.0020} $ 

$ f_{L}^{a_{1}} $ 

$ 0.9264^{+0.0028}_{-0.0028} $ 

$ 0.9384^{+0.0093}_{-0.0093} $ 

$ 0.9337^{+0.0027}_{-0.0027} $ 

Table 10. Same as that in Table 7 but for
$ q^2 = 3.0 - 4.0 $ GeV2 bin.$ q^{2}= 4.0 - 5.0 $ GeV2

Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho\mu^{+}\mu^{-}) \times 10^{+9} $ 

$ 0.828^{+0.144}_{-0.144} $ 

$ 1.587^{+0.276}_{-0.276} $ 

$ 1.242^{+0.216}_{-0.216} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho(\to \pi\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 0.828^{+0.144}_{-0.144} $ 

$ 1.587^{+0.276}_{-0.276} $ 

$ 1.242^{+0.216}_{-0.216} $ 

$ A_{\text{FB}}^{\rho} $ 

$ -0.068^{+0.002}_{-0.002} $ 

$ -0.117^{+0.002}_{-0.002} $ 

$ -0.119^{+0.001}_{-0.001} $ 

$ f_{L}^{\rho} $ 

$ 0.8101^{+0.0002}_{-0.0002} $ 

$ 0.8315^{+0.0006}_{-0.0006} $ 

$ 0.8352^{+0.0005}_{-0.0005} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 6.9869^{+4.5967}_{-4.5967} $ 

$ 13.7062^{+9.0082}_{-9.0082} $ 

$ 11.110^{+7.307}_{-7.307} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\parallel}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 2.0029^{+1.3177}_{-1.3177} $ 

$ 3.9291^{+2.5823}_{-2.5823} $ 

$ 3.076^{+2.023}_{-2.023} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\perp}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 4.8908^{+3.2177}_{-3.2177} $ 

$ 9.5944^{+6.3057}_{-6.3057} $ 

$ 7.512^{+4.939}_{-4.939} $ 

$ A_{\text{FB}}^{a_{1}} $ 

$ -0.0083^{+0.0031}_{-0.0031} $ 

$ -0.0284^{+0.0022}_{-0.0022} $ 

$ -0.0271^{+0.0026}_{-0.0026} $ 

$ f_{L}^{a_{1}} $ 

$ 0.8957^{+0.0040}_{-0.0040} $ 

$ 0.9087^{+0.0090}_{-0.0090} $ 

$ 0.9040^{+0.0040}_{-0.0040} $ 

Table 11. Same as that in Table 7 but for
$ q^2 = 4.0 - 5.0 $ GeV2 bin.$ q^{2}= 5.0 - 6.0 $ GeV2

Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho\mu^{+}\mu^{-}) \times 10^{+9} $ 

$ 0.868^{+0.147}_{-0.147} $ 

$ 1.682^{+0.285}_{-0.285} $ 

$ 1.312^{+0.220}_{-0.220} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho(\to \pi\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 0.868^{+0.147}_{-0.147} $ 

$ 1.682^{+0.285}_{-0.285} $ 

$ 1.312^{+0.220}_{-0.220} $ 

$ A_{\text{FB}}^{\rho} $ 

$ -0.119^{+0.002}_{-0.002} $ 

$ -0.152^{+0.001}_{-0.001} $ 

$ -0.161^{+0.001}_{-0.001} $ 

$ f_{L}^{\rho} $ 

$ 0.7601^{+0.0002}_{-0.0002} $ 

$ 0.7735^{+0.0006}_{-0.0006} $ 

$ 0.7786^{+0.0006}_{-0.0006} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 6.7521^{+4.4407}_{-4.4407} $ 

$ 13.2899^{+8.7329}_{-8.7329} $ 

$ 10.700^{+7.056}_{-7.056} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\parallel}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 1.9356^{+1.2730}_{-1.2730} $ 

$ 3.8098^{+2.5034}_{-2.5034} $ 

$ 2.977^{+1.893}_{-1.893} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\perp}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 4.7265^{+3.1085}_{-3.1085} $ 

$ 9.3029^{+6.1130}_{-6.1130} $ 

$ 7.269^{+4.778}_{-4.778} $ 

$ A_{\text{FB}}^{a_{1}} $ 

$ -0.0252^{+0.0037}_{-0.0037} $ 

$ -0.0415^{+0.0025}_{-0.0025} $ 

$ -0.0423^{+0.0030}_{-0.0030} $ 

$ f_{L}^{a_{1}} $ 

$ 0.8599^{+0.0049}_{-0.0049} $ 

$ 0.8732^{+0.0084}_{-0.0084} $ 

$ 0.8687^{+0.0050}_{-0.0050} $ 

Table 12. Same as that in Table 7 but for
$ q^2 = 5.0 - 6.0 $ GeV2 bin.$ q^{2}= 6.0 - 7.0 $ GeV2

Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho\mu^{+}\mu^{-}) \times 10^{+9} $ 

$ 0.912^{+0.150}_{-0.150} $ 

$ 1.781^{+0.294}_{-0.294} $ 

$ 1.386^{+0.285}_{-0.285} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho(\to \pi\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 0.912^{+0.150}_{-0.150} $ 

$ 1.781^{+0.294}_{-0.294} $ 

$ 1.386^{+0.285}_{-0.285} $ 

$ A_{\text{FB}}^{\rho} $ 

$ -0.164^{+0.002}_{-0.002} $ 

$ -0.181^{+0.001}_{-0.001} $ 

$ -0.197^{+0.002}_{-0.002} $ 

$ f_{L}^{\rho} $ 

$ 0.7101^{+0.0003}_{-0.0003} $ 

$ 0.7207^{+0.0006}_{-0.0006} $ 

$ 0.7263^{+0.0006}_{-0.0006} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 6.5244^{+4.2905}_{-4.2905} $ 

$ 12.8742^{+8.4599}_{-8.4599} $ 

$ 10.390^{+6.827}_{-6.827} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\parallel}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 1.8703^{+1.2300}_{-1.2300} $ 

$ 3.6906^{+2.4252}_{-2.4252} $ 

$ 2.879^{+1.893}_{-1.893} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\perp}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 4.5671^{+3.0034}_{-3.0034} $ 

$ 9.0120^{+5.9220}_{-5.9220} $ 

$ 7.030^{+4.621}_{-4.621} $ 

$ A_{\text{FB}}^{a_{1}} $ 

$ -0.0425^{+0.0042}_{-0.0042} $ 

$ -0.0548^{+0.0028}_{-0.0028} $ 

$ -0.0578^{+0.0033}_{-0.0033} $ 

$ f_{L}^{a_{1}} $ 

$ 0.8204^{+0.0056}_{-0.0056} $ 

$ 0.8336^{+0.0076}_{-0.0076} $ 

$ 0.8292^{+0.0058}_{-0.0058} $ 

Table 13. Same as that in Table 7 but for
$ q^2 = 6.0 - 7.0 $ GeV2 bin.$ q^{2}= 7.0 - 8.0 $ GeV2

Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho\mu^{+}\mu^{-}) \times 10^{+9} $ 

$ 0.959^{+0.150}_{-0.150} $ 

$ 1.882^{+0.303}_{-0.303} $ 

$ 1.463^{+0.235}_{-0.235} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to\rho(\to \pi\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 0.959^{+0.150}_{-0.150} $ 

$ 1.882^{+0.303}_{-0.303} $ 

$ 1.463^{+0.235}_{-0.235} $ 

$ A_{{\rm{FB}}}^{\rho} $ 

$ -0.202^{+0.003}_{-0.003} $ 

$ -0.207^{+0.002}_{-0.002} $ 

$ -0.228^{+0.002}_{-0.002} $ 

$ f_{L}^{\rho} $ 

$ 0.6602^{+0.0004}_{-0.0004} $ 

$ 0.6733^{+0.0006}_{-0.0006} $ 

$ 0.6790^{+0.0006}_{-0.0006} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 6.2930^{+4.1389}_{-4.1389} $ 

$ 12.4428^{+8.1785}_{-8.1785} $ 

$ 10.004^{+6.622}_{-6.622} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\parallel}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 1.8040^{+1.1865}_{-1.1865} $ 

$ 3.5669^{+2.3445}_{-2.3445} $ 

$ 2.778^{+1.827}_{-1.827} $ 

$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} (B\to a_{1}(\to \rho_{\perp}\pi)\mu^{+}\mu^{-})\times 10^{+9} $ 

$ 4.4051^{+2.8972}_{-2.8972} $ 

$ 8.7100^{+5.7249}_{-5.7249} $ 

$ 6.786^{+4.461}_{-4.461} $ 

$ A_{{\rm{FB}}}^{a_{1}} $ 

$ -0.0600^{+0.0046}_{-0.0046} $ 

$ -0.0681^{+0.0029}_{-0.0029} $ 

$ -0.0734^{+0.0035}_{-0.0035} $ 

$ f_{L}^{a_{1}} $ 

$ 0.7778^{+0.0059}_{-0.0059} $ 

$ 0.7905^{+0.0068}_{-0.0068} $ 

$ 0.7864^{+0.0029}_{-0.0029} $ 

Table 14. Same as that in Table 7 but for
$ q^2 = 7.0 - 8.0 $ GeV2 bin.
Figure 2. (color online) Differential branching ratio of decays (a)
$ B\to\rho(\to\pi\pi)\mu^{+}\mu^{-} $ , (b)$ B\to a_{1}(\to\rho\pi)\mu^{+}\mu^{-} $ , (c)$ B\to a_{1}(\to\rho_{\parallel}\pi)\mu^{+}\mu^{-} $ , and (d)$ B\to a_{1}(\to\rho_{\perp}\pi)\mu^{+}\mu^{-} $ in the SM and two scenarios of the family non-universal$ Z^{\prime} $ model.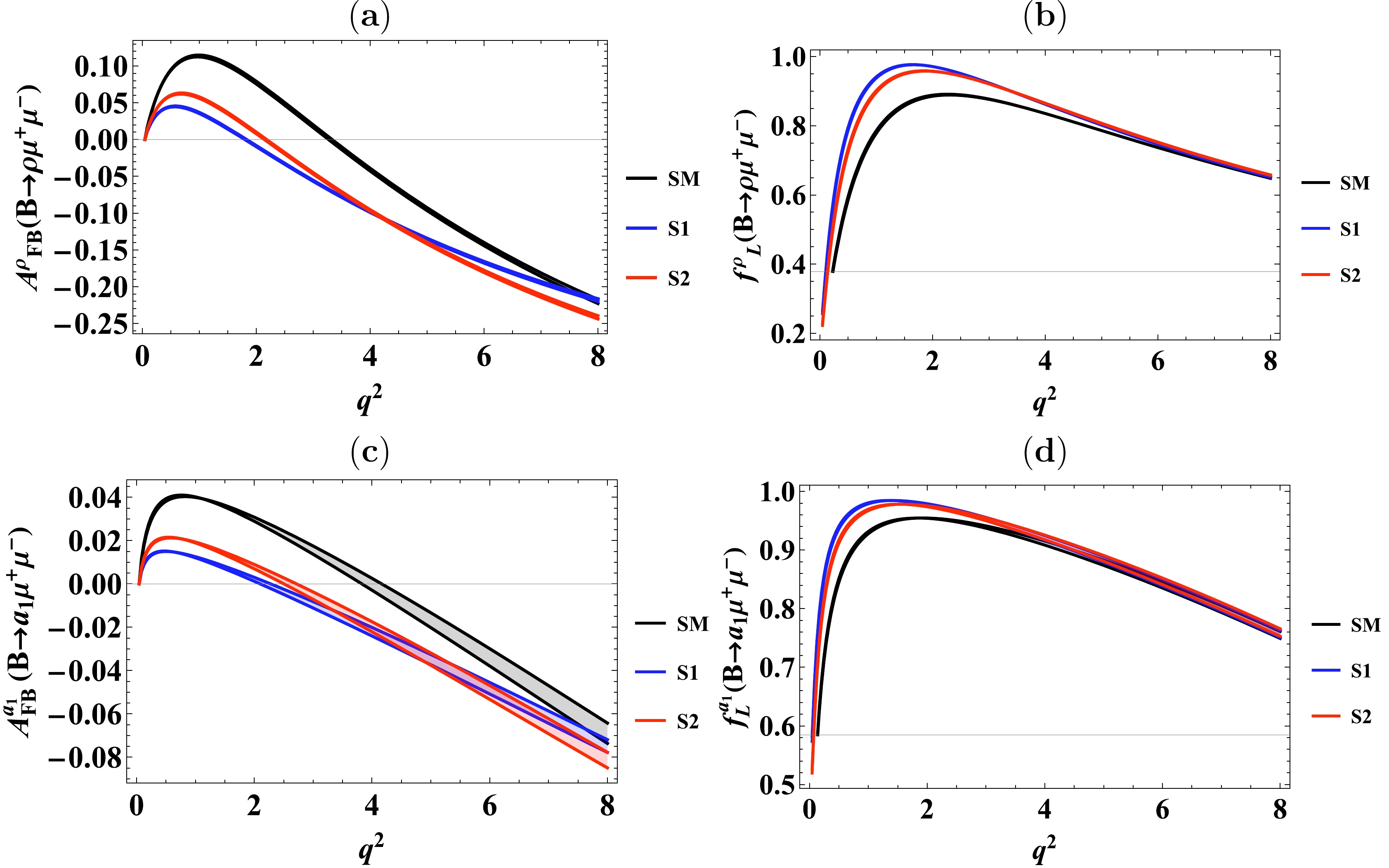
Figure 3. (color online) (a) Lepton forward-backward asymmetry
$ A_{{\rm{FB}}}^{\rho} $ and (b) longitudinal polarization fraction of$ \rho $ meson$ f_{L}^{\rho} $ for the$ B\to\rho\mu^{+}\mu^{-} $ decay, along with (c) Lepton forward-backward asymmetry$ A_{{\rm{FB}}}^{a_1} $ and (d) longitudinal polarization fraction of$ a_1 $ meson$ f_{L}^{a_1} $ for the$ B\to a_{1}\mu^{+}\mu^{-} $ decay, in the SM and two scenarios of the family non-universal$ Z^{\prime} $ model.● Figures 2(a), 2(b), 2(c), and 2(d) depict the differential branching ratios
$ \frac{{\rm d}{\cal{B}}}{{\rm d}q^{2}} $ of$ B\to\rho(\to\pi\pi)\mu^{+}\mu^{-} $ ,$ B\to a_{1} (\to\rho\pi)\mu^{+}\mu^{-} $ ,$ B\to a_{1}(\to\rho_{\parallel}\pi)\mu^{+}\mu^{-} $ , and$ B\to a_{1}(\to\rho_{\perp}\pi)\mu^{+}\mu^{-} $ decays, respectively, in the framework of the SM and scenarios S1 and S2 of the family non-universal$ Z^{\prime} $ model. Figure 2(a) indicates that, after including uncertainties of the form factors, the predictions of differential branching ratio in two scenarios of the family non-universal$ Z^{\prime} $ model deviate from the SM predictions such that they show a tendency towards higher values of differential branching ratios as compared to the SM expectations, which is more dominant in Scenario S1. In Figs. 2(b), 2(c), and 2(d), our results show similar trend of higher values of differential branching ratios in two scenarios of the family non-universal$ Z^{\prime} $ model. However, in this case SM predictions largely overlap with the scenarios of the family non-universal$ Z^{\prime} $ model due to the larger uncertainties originating from the form factors. At very low$ q^2 < 1 $ GeV2, these differential branching ratios are dominated by the SM magnetic dipole Wilson coefficient$ C_{7} $ . Hence, at$ q^2\to 0 $ , the results of differential branching ratios indicate singularity corresponding to photon pole.● Figure 3 represents the lepton forward-backward asymmetry and longitudinally polarized final state mesons as a function of
$ q^{2} $ in the framework of the SM and family non-universal$ Z^{\prime} $ model for$ B\to\rho\mu^{+}\mu^{-} $ and$B\to a_{1}\mu^{+}\mu^{-}$ decays. Figures 3(a) and Fig. 3(c) show the zero position of the$ A_{{\rm{FB}}}(q^{2}) $ for$ B\to\rho\mu^{+}\mu^{-} $ and$ B\to a_{1}\mu^{+}\mu^{-} $ decays in the framework of SM and two scenarios S1 and S2 of family non-universal$ Z^{\prime} $ model, respectively. Both scenarios S1 and S2 of the family non-universal$ Z^{\prime} $ are shifted towards left when compared to the SM prediction. Hence, the zero crossing of$ A_{{\rm{FB}}}^{\rho}(q^{2}) $ and$ A_{{\rm{FB}}}^{a_1}(q^{2}) $ for both scenarios is distinguishable from the SM expectation. Furthermore, overall$ q^2 $ predictions of$ A_{{\rm{FB}}}^{\rho}(q^{2}) $ and$ A_{{\rm{FB}}}^{a_1}(q^{2}) $ in S1 and S2 scenarios for the entire$ q^2 $ range show discrimination from the SM results. In Fig. 3(b), we plot the longitudinal helicity fraction$ f_{L}^{\rho} $ for the decay$ B\to\rho\mu^{+}\mu^{-} $ in the SM framework and the two scenarios S1 and S2 of the family non universal$ Z^{\prime} $ model. The predictions in S1 and S2 scenarios clearly show a departure from the SM result in the region$ q^{2}=(0.1-5) $ GeV2. However, for the case of$B\to a_1\mu^{+}\mu^{-} $ decay, longitudinal helicity fraction$f_L^{a_1} $ shows deviation from the SM result in region$q^2=(0.1-3)~{\rm GeV^2}$ , only as shown in Fig. 3(d). Based on the values of the NP Wilson coefficients reported in Table 6, it is evident that the SM Wilson coefficients become more pronounced deviations in the presence of scenario S1 when compared to scenario S2, which also reflects in the overall results of observables such that scenario S2 appears closer to the SM. -
In this section, we present the effects of the family non-universal
$ Z^{\prime} $ model on the separate normalized angular coefficients such as$ \langle I^{\rho}_{n\lambda}\rangle $ , in the$ B\to\rho(\to\pi\pi)\mu^{+}\mu^{-} $ decay, and$ \langle \widehat{I}^{a_{1}}_{n\lambda,\parallel}\rangle $ ,$ \langle \widehat{I}^{a_{1}}_{n\lambda,\perp}\rangle $ , in the$ B\to a_{1}(\to\rho_{\parallel, \perp})\mu^{+}\mu^{-} $ decay. The predicted numerical values of these observables, in different$ q^2 $ bins, for the SM as well as for the two different scenarios of family non-universal$ Z^{\prime} $ model are provided in Tables 15−38 of Appendix B. The listed errors in these tables originate mainly from the uncertainties of the form factors. Furthermore, we display the results of normalized angular observables as a function of$ q^{2} $ in Figs. 4−6.
Figure 5. (color online) Angular observables
$ \langle \widehat{I}^{a_1}_{1s,\parallel}\rangle,\langle \widehat{I}^{a_1}_{1c.\parallel}\rangle,\langle \widehat{I}^{a_1}_{2s,\parallel}\rangle,\langle \widehat{I}^{a_1}_{2c,\parallel}\rangle,\langle \widehat{I}^{a_1}_{3,\parallel}\rangle,\langle \widehat{I}^{a_1}_{4,\parallel}\rangle,\langle \widehat{I}^{a_1}_{5,\parallel}\rangle $ , and$ \langle \widehat{I}^{a_1}_{6s,\parallel}\rangle $ for the decay$ B\to a_{1}(\to\rho_{\parallel}\pi)\mu^{+}\mu^{-} $ in the SM and two scenarios of the family non-universal$ Z^{\prime} $ model.$ q^{2}= 0.1 - 1.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle I^{\rho}_{1s}\rangle $ 

$ 0.3524^{+0.0043}_{-0.0043} $ 

$ 0.2056^{+0.0039}_{-0.0039} $ 

$ 0.2533^{+0.0042}_{-0.0042} $ 

$ \langle I^{\rho}_{1c}\rangle $ 

$ 0.5455^{+0.0060}_{-0.0060} $ 

$ 0.7569^{+0.0056}_{-0.0056} $ 

$ 0.6882^{+0.0060}_{-0.0060} $ 

$ \langle I^{\rho}_{2s}\rangle $ 

$ 0.0908^{+0.0011}_{-0.0011} $ 

$ 0.0517^{+0.0009}_{-0.0009} $ 

$ 0.0641^{+0.0011}_{-0.0011} $ 

$ \langle I^{\rho}_{2c}\rangle $ 

$ -0.4308^{+0.0055}_{-0.0055} $ 

$ -0.4035^{+0.0054}_{-0.0054} $ 

$ -0.4134^{+0.0055}_{-0.0055} $ 

$ \langle I^{\rho}_{3}\rangle $ 

$ 0.0008^{+0.0003}_{-0.0003} $ 

$ 0.0012^{+0.0005}_{-0.0005} $ 

$ 0.0012^{+0.0005}_{-0.0005} $ 

$ \langle I^{\rho}_{4}\rangle $ 

$ -0.1193^{+0.0003}_{-0.0003} $ 

$ -0.1354^{+0.0003}_{-0.0003} $ 

$ -0.1371^{+0.0003}_{-0.0003} $ 

$ \langle I^{\rho}_{5}\rangle $ 

$ 0.0024^{+0.0002}_{-0.0002} $ 

$ 0.0013^{+0.00005}_{-0.00005} $ 

$ 0.0021^{+0.0001}_{-0.0001} $ 

$ \langle I^{\rho}_{6s}\rangle $ 

$ 0.1028^{+0.0003}_{-0.0003} $ 

$ 0.0639^{+0.0002}_{-0.0002} $ 

$ 0.0918^{+0.0002}_{-0.0002} $ 

$ \langle I^{\rho}_{6c}\rangle $ 

0 0 0 Table 15. Predictions of averaged values of angular observables for the
$ B\to\rho(\to \pi\pi)\mu^{+}\mu^{-} $ decay, in$ q^2 = 0.1 - 1.0 $ GeV2 bin, for the SM as well as NP scenarios (S1, S2) of the$ Z^{\prime} $ model listed in Table 5. The errors presented mainly arise from the uncertainties of the form factors.$ q^{2}= 1.0 - 2.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle I^{\rho}_{1s}\rangle $ 

$ 0.1122^{+0.0022}_{-0.0022} $ 

$ 0.0238^{+0.0006}_{-0.0006} $ 

$ 0.0434^{+0.0011}_{-0.0011} $ 

$ \langle I^{\rho}_{1c}\rangle $ 

$ 0.8629^{+0.0029}_{-0.0029} $ 

$ 0.9830^{+0.0008}_{-0.0008} $ 

$ 0.9572^{+0.0014}_{-0.0014} $ 

$ \langle I^{\rho}_{2s}\rangle $ 

$ 0.0363^{+0.0007}_{-0.0007} $ 

$ 0.0078^{+0.0002}_{-0.0002} $ 

$ 0.0139^{+0.0003}_{-0.0003} $ 

$ \langle I^{\rho}_{2c}\rangle $ 

$ -0.8108^{+0.0031}_{-0.0031} $ 

$ -0.8350^{+0.0033}_{-0.0033} $ 

$ -0.8125^{+0.0032}_{-0.0032} $ 

$ \langle I^{\rho}_{3}\rangle $ 

$ -0.0003^{+0.0001}_{-0.0001} $ 

$ 0.0013^{+0.0005}_{-0.0005} $ 

$ 0.0002^{+0.0001}_{-0.0001} $ 

$ \langle I^{\rho}_{4}\rangle $ 

$ -0.0213^{+0.0003}_{-0.0003} $ 

$ -0.0030^{+0.0006}_{-0.0006} $ 

$ -0.0150^{+0.0004}_{-0.0004} $ 

$ \langle I^{\rho}_{5}\rangle $ 

$ -0.0124^{+0.0004}_{-0.0004} $ 

$ -0.0037^{+0.0011}_{-0.0011} $ 

$ -0.0090^{+0.0003}_{-0.0003} $ 

$ \langle I^{\rho}_{6s}\rangle $ 

$ 0.1335^{+0.0022}_{-0.0022} $ 

$ 0.0863^{+0.0016}_{-0.0016} $ 

$ 0.1257^{+0.0020}_{-0.0020} $ 

$ \langle I^{\rho}_{6c}\rangle $ 

0 0 0 Table 16. Same as that in Table 15 but for
$ q^2 = 1.0 - 2.0 $ GeV2 bin.$ q^{2}= 2.0 - 3.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle I^{\rho}_{1s}\rangle $ 

$ 0.0854^{+0.0008}_{-0.0008} $ 

$ 0.0380^{+0.0002}_{-0.0002} $ 

$ 0.0334^{+0.0003}_{-0.0003} $ 

$ \langle I^{\rho}_{1c}\rangle $ 

$ 0.8940^{+0.0011}_{-0.0011} $ 

$ 0.9579^{+0.0003}_{-0.0003} $ 

$ 0.9506^{+0.0001}_{-0.0001} $ 

$ \langle I^{\rho}_{2s}\rangle $ 

$ 0.0284^{+0.0003}_{-0.0003} $ 

$ 0.0125^{+0.0001}_{-0.0001} $ 

$ 0.0144^{+0.0001}_{-0.0001} $ 

$ \langle I^{\rho}_{2c}\rangle $ 

$ -0.8623^{+0.0012}_{-0.0012} $ 

$ -0.9200^{+0.0010}_{-0.0010} $ 

$ -0.8775^{+0.0012}_{-0.0012} $ 

$ \langle I^{\rho}_{3}\rangle $ 

$ -0.0032^{+0.0012}_{-0.0012} $ 

$ -0.0032^{+0.0012}_{-0.0012} $ 

$ -0.0029^{+0.0011}_{-0.0011} $ 

$ \langle I^{\rho}_{4}\rangle $ 

$ 0.0737^{+0.0015}_{-0.0015} $ 

$ 0.0941^{+0.0020}_{-0.0020} $ 

$ 0.0830^{+0.0018}_{-0.0018} $ 

$ \langle I^{\rho}_{5}\rangle $ 

$ -0.0227^{+0.0006}_{-0.0006} $ 

$ -0.0071^{+0.0001}_{-0.0001} $ 

$ -0.0158^{+0.0004}_{-0.0004} $ 

$ \langle I^{\rho}_{6s}\rangle $ 

$ 0.0644^{+0.0025}_{-0.0025} $ 

$ 0.0290^{+0.0019}_{-0.0019} $ 

$ 0.0612^{+0.0024}_{-0.0024} $ 

$ \langle I^{\rho}_{6c}\rangle $ 

0 0 0 Table 17. Same as that in Table 15 but for
$ q^2 = 2.0 - 3.0 $ GeV2 bin.$ q^{2}= 3.0 - 4.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle I^{\rho}_{1s}\rangle $ 

$ 0.1072^{+0.0002}_{-0.0002} $ 

$ 0.0804^{+0.0004}_{-0.0004} $ 

$ 0.0801^{+0.0003}_{-0.0003} $ 

$ \langle I^{\rho}_{1c}\rangle $ 

$ 0.8624^{+0.0003}_{-0.0003} $ 

$ 0.8984^{+0.0005}_{-0.0005} $ 

$ 0.8988^{+0.0004}_{-0.0004} $ 

$ \langle I^{\rho}_{2s}\rangle $ 

$ 0.0357^{+0.00009}_{-0.00009} $ 

$ 0.0265^{+0.00001}_{-0.00001} $ 

$ 0.0266^{+0.00009}_{-0.00009} $ 

$ \langle I^{\rho}_{2c}\rangle $ 

$ -0.8408^{+0.0004}_{-0.0004} $ 

$ -0.9098^{+0.00008}_{-0.00008} $ 

$ -0.8611^{+0.0003}_{-0.0003} $ 

$ \langle I^{\rho}_{3}\rangle $ 

$ -0.0070^{+0.0024}_{-0.0024} $ 

$ -0.0070^{+0.0021}_{-0.0021} $ 

$ -0.0067^{+0.0023}_{-0.0023} $ 

$ \langle I^{\rho}_{4}\rangle $ 

$ 0.1364^{+0.0025}_{-0.0025} $ 

$ 0.1535^{+0.0029}_{-0.0029} $ 

$ 0.1452^{+0.0027}_{-0.0027} $ 

$ \langle I^{\rho}_{5}\rangle $ 

$ -0.0288^{+0.0007}_{-0.0007} $ 

$ -0.0092^{+0.0002}_{-0.0002} $ 

$ -0.0197^{+0.0004}_{-0.0004} $ 

$ \langle I^{\rho}_{6s}\rangle $ 

$ -0.0159^{+0.0025}_{-0.0025} $ 

$ -0.0405^{+0.0020}_{-0.0020} $ 

$ -0.0167^{+0.0025}_{-0.0025} $ 

$ \langle I^{\rho}_{6c}\rangle $ 

0 0 0 Table 18. Same as that in Table 15 but for
$ q^2 = 3.0 - 4.0 $ GeV2 bin.$ q^{2}= 4.0 - 5.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle I^{\rho}_{1s}\rangle $ 

$ 0.1421^{+0.00002}_{-0.00002} $ 

$ 0.1260^{+0.0004}_{-0.0004} $ 

$ 0.1235^{+0.0004}_{-0.0004} $ 

$ \langle I^{\rho}_{1c}\rangle $ 

$ 0.8145^{+0.00003}_{-0.00003} $ 

$ 0.8356^{+0.0005}_{-0.0005} $ 

$ 0.8393^{+0.0005}_{-0.0005} $ 

$ \langle I^{\rho}_{2s}\rangle $ 

$ 0.0473^{+0.000005}_{-0.000005} $ 

$ 0.0417^{+0.0001}_{-0.0001} $ 

$ 0.0409^{+0.00001}_{-0.00001} $ 

$ \langle I^{\rho}_{2c}\rangle $ 

$ -0.7987^{+0.00001}_{-0.00001} $ 

$ -0.8690^{+0.0005}_{-0.0005} $ 

$ -0.8202^{+0.0001}_{-0.0001} $ 

$ \langle I^{\rho}_{3}\rangle $ 

$ -0.0113^{+0.0036}_{-0.0036} $ 

$ -0.0174^{+0.0030}_{-0.0030} $ 

$ -0.0111^{+0.0035}_{-0.0035} $ 

$ \langle I^{\rho}_{4}\rangle $ 

$ 0.1786^{+0.0032}_{-0.0032} $ 

$ 0.1922^{+0.0035}_{-0.0035} $ 

$ 0.1861^{+0.0035}_{-0.0035} $ 

$ \langle I^{\rho}_{5}\rangle $ 

$ -0.0323^{+0.0007}_{-0.0007} $ 

$ -0.0106^{+0.0002}_{-0.0002} $ 

$ -0.0219^{+0.0005}_{-0.0005} $ 

$ \langle I^{\rho}_{6s}\rangle $ 

$ -0.0914^{+0.0026}_{-0.0026} $ 

$ -0.1070^{+0.0020}_{-0.0020} $ 

$ -0.0910^{+0.0025}_{-0.0025} $ 

$ \langle I^{\rho}_{6c}\rangle $ 

0 0 0 Table 19. Same as that in Table 15 but for
$ q^2 = 4.0 - 5.0 $ GeV2 bin.$ q^{2}= 5.0 - 6.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle I^{\rho}_{1s}\rangle $ 

$ 0.1792^{+0.0002}_{-0.0002} $ 

$ 0.1667^{+0.0004}_{-0.0004} $ 

$ 0.1659^{+0.0004}_{-0.0004} $ 

$ \langle I^{\rho}_{1c}\rangle $ 

$ 0.7640^{+0.0002}_{-0.0002} $ 

$ 0.7767^{+0.0006}_{-0.0006} $ 

$ 0.7818^{+0.0006}_{-0.0006} $ 

$ \langle I^{\rho}_{2s}\rangle $ 

$ 0.0596^{+0.0001}_{-0.0001} $ 

$ 0.0561^{+0.0001}_{-0.0001} $ 

$ 0.0550^{+0.0001}_{-0.0001} $ 

$ \langle I^{\rho}_{2c}\rangle $ 

$ -0.7520^{+0.0002}_{-0.0002} $ 

$ -0.8191^{+0.0007}_{-0.0007} $ 

$ -0.7730^{+0.0004}_{-0.0004} $ 

$ \langle I^{\rho}_{3}\rangle $ 

$ -0.0163^{+0.0048}_{-0.0048} $ 

$ -0.0164^{+0.0048}_{-0.0049} $ 

$ -0.0161^{+0.0047}_{-0.0047} $ 

$ \langle I^{\rho}_{4}\rangle $ 

$ 0.2078^{+0.0036}_{-0.0036} $ 

$ 0.2185^{+0.0039}_{-0.0039} $ 

$ 0.2140^{+0.0031}_{-0.0031} $ 

$ \langle I^{\rho}_{5}\rangle $ 

$ -0.0343^{+0.0008}_{-0.0008} $ 

$ -0.0116^{+0.0002}_{-0.0002} $ 

$ -0.0231^{+0.0005}_{-0.0005} $ 

$ \langle I^{\rho}_{6s}\rangle $ 

$ -0.1589^{+0.0028}_{-0.0028} $ 

$ -0.1668^{+0.0022}_{-0.0022} $ 

$ -0.1578^{+0.0027}_{-0.0027} $ 

$ \langle I^{\rho}_{6c}\rangle $ 

0 0 0 Table 20. Same as that in Table 15 but for
$ q^2 = 5.0 - 6.0 $ GeV2 bin.$ q^{2}= 6.0 - 7.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle I^{\rho}_{1s}\rangle $ 

$ 0.2151^{+0.0003}_{-0.0003} $ 

$ 0.2093^{+0.0005}_{-0.0005} $ 

$ 0.2051^{+0.0005}_{-0.0005} $ 

$ \langle I^{\rho}_{1c}\rangle $ 

$ 0.7156^{+0.0004}_{-0.0004} $ 

$ 0.7232^{+0.0006}_{-0.0006} $ 

$ 0.7288^{+0.00056}_{-0.0006} $ 

$ \langle I^{\rho}_{2s}\rangle $ 

$ 0.0715^{+0.0001}_{-0.0001} $ 

$ 0.0693^{+0.0001}_{-0.0001} $ 

$ 0.0680^{+0.0002}_{-0.0002} $ 

$ \langle I^{\rho}_{2c}\rangle $ 

$ -0.7062^{+0.0004}_{-0.0004} $ 

$ -0.7685^{+0.0009}_{-0.0009} $ 

$ -0.7259^{+0.0005}_{-0.0005} $ 

$ \langle I^{\rho}_{3}\rangle $ 

$ -0.0218^{+0.0058}_{-0.0058} $ 

$ -0.0220^{+0.0058}_{-0.0058} $ 

$ -0.0216^{+0.0057}_{-0.0057} $ 

$ \langle I^{\rho}_{4}\rangle $ 

$ 0.2286^{+0.0040}_{-0.0040} $ 

$ 0.2371^{+0.0042}_{-0.0042} $ 

$ 0.2338^{+0.0041}_{-0.0041} $ 

$ \langle I^{\rho}_{5}\rangle $ 

$ -0.0354^{+0.0008}_{-0.0008} $ 

$ -0.0122^{+0.0003}_{-0.0003} $ 

$ -0.0239^{+0.0006}_{-0.0006} $ 

$ \langle I^{\rho}_{6s}\rangle $ 

$ -0.2182^{+0.0031}_{-0.0031} $ 

$ -0.2192^{+0.0025}_{-0.0025} $ 

$ -0.2166^{+0.0030}_{-0.0030} $ 

$ \langle I^{\rho}_{6c}\rangle $ 

0 0 0 Table 21. Same as that in Table 15 but for
$ q^2 = 6.0 - 7.0 $ GeV2 bin.$ q^{2}= 7.0 - 8.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle I^{\rho}_{1s}\rangle $ 

$ 0.2483^{+0.0003}_{-0.0003} $ 

$ 0.2448^{+0.0005}_{-0.0005} $ 

$ 0.2406^{+0.0005}_{-0.0005} $ 

$ \langle I^{\rho}_{1c}\rangle $ 

$ 0.6708^{+0.0004}_{-0.0004} $ 

$ 0.6753^{+0.0006}_{-0.0006} $ 

$ 0.6820^{+0.0007}_{-0.0007} $ 

$ \langle I^{\rho}_{2s}\rangle $ 

$ 0.0826^{+0.0001}_{-0.0001} $ 

$ 0.0811^{+0.0002}_{-0.0002} $ 

$ 0.0798^{+0.0002}_{-0.0002} $ 

$ \langle I^{\rho}_{2c}\rangle $ 

$ -0.6632^{+0.0005}_{-0.0005} $ 

$ -0.7230^{+0.0009}_{-0.0009} $ 

$ -0.6815^{+0.0006}_{-0.0006} $ 

$ \langle I^{\rho}_{3}\rangle $ 

$ -0.0280^{+0.0068}_{-0.0068} $ 

$ -0.0282^{+0.0065}_{-0.0065} $ 

$ -0.0278^{+0.0067}_{-0.0067} $ 

$ \langle I^{\rho}_{4}\rangle $ 

$ 0.2437^{+0.0042}_{-0.0042} $ 

$ 0.2504^{+0.0044}_{-0.0044} $ 

$ 0.2480^{+0.0043}_{-0.0043} $ 

$ \langle I^{\rho}_{5}\rangle $ 

$ -0.0357^{+0.0009}_{-0.0009} $ 

$ -0.0126^{+0.0003}_{-0.0003} $ 

$ -0.0239^{+0.0006}_{-0.0006} $ 

$ \langle I^{\rho}_{6s}\rangle $ 

$ -0.2701^{+0.0035}_{-0.0035} $ 

$ -0.2650^{+0.0029}_{-0.0029} $ 

$ -0.2681^{+0.0034}_{-0.0034} $ 

$ \langle I^{\rho}_{6c}\rangle $ 

0 0 0 Table 22. Same as that in Table 15 but for
$ q^2 = 7.0 - 8.0 $ GeV2 bin.$ q^{2}= 0.1 - 1.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\parallel}\rangle $ 

$ 0.0414^{+0.0007}_{-0.0007} $ 

$ 0.0190^{+0.0006}_{-0.0006} $ 

$ 0.0251^{+0.0006}_{-0.0006} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\parallel}\rangle $ 

$ 0.2390^{+0.0009}_{-0.0009} $ 

$ 0.2737^{+0.0007}_{-0.0007} $ 

$ 0.2643^{+0.0008}_{-0.0008} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\parallel}\rangle $ 

$ 0.0107^{+0.0002}_{-0.0002} $ 

$ 0.0048^{+0.0001}_{-0.0001} $ 

$ 0.0064^{+0.0002}_{-0.0002} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\parallel}\rangle $ 

$ -0.2027^{+0.0015}_{-0.0015} $ 

$ -0.2211^{+0.0008}_{-0.0008} $ 

$ -0.2159^{+0.0010}_{-0.0010} $ 

$ \langle\widehat{I}^{a_{1}}_{3,\parallel}\rangle $ 

$ 0.0027^{+0.00001}_{-0.00001} $ 

$ 0.0024^{+0.00003}_{-0.00003} $ 

$ 0.0026^{+0.000002}_{-0.000002} $ 

$ \langle\widehat{I}^{a_{1}}_{4,\parallel}\rangle $ 

$ -0.0218^{+0.0007}_{-0.0007} $ 

$ -0.0183^{+0.0007}_{-0.0007} $ 

$ -0.0207^{+0.0007}_{-0.0007} $ 

$ \langle\widehat{I}^{a_{1}}_{5,\parallel}\rangle $ 

$ 0.0639^{+0.0002}_{-0.001} $ 

$ 0.0265^{+0.0003}_{-0.0003} $ 

$ 0.0366^{+0.0003}_{-0.0003} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\parallel}\rangle $ 

$ 0.0133^{+0.0002}_{-0.0002} $ 

$ 0.0051^{+0.00002}_{-0.00002} $ 

$ 0.0073^{+0.0001}_{-0.0001} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\parallel}\rangle $ 

0 0 0 Table 23. Predictions of averaged values of angular observables for the
$ B\to a_{1}(\to\rho_{\parallel}\pi)\mu^{+}\mu^{-} $ decay, in$ q^2 = 0.1 - 1.0 $ GeV2 bin, for the SM as well as the NP scenarios (S1, S2) of the$ Z^{\prime} $ model listed in Table 5. The errors presented mainly arise from the uncertainties of the form factors.$ q^{2}= 1.0 - 2.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\parallel}\rangle $ 

$ 0.0112^{+0.0002}_{-0.0002} $ 

$ 0.0038^{+0.00003}_{-0.00003} $ 

$ 0.0052^{+0.0004}_{-0.0004} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\parallel}\rangle $ 

$ 0.2743^{+0.0002}_{-0.0002} $ 

$ 0.2855^{+0.00007}_{-0.00007} $ 

$ 0.2533^{+0.0001}_{-0.0001} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\parallel}\rangle $ 

$ 0.0036^{+0.00005}_{-0.00005} $ 

$ 0.0012^{+0.00001}_{-0.00001} $ 

$ 0.0017^{+0.00001}_{-0.00001} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\parallel}\rangle $ 

$ -0.2640^{+0.0005}_{-0.0005} $ 

$ -0.2702^{+0.00004}_{-0.00004} $ 

$ -0.2692^{+0.0002}_{-0.0002} $ 

$ \langle\widehat{I}^{a_{1}}_{3\parallel}\rangle $ 

$ 0.0004^{+0.0001}_{-0.0001} $ 

$ -0.0002^{+0.0002}_{-0.0002} $ 

$ 0.0016^{+0.0002}_{-0.0002} $ 

$ \langle\widehat{I}^{a_{1}}_{4\parallel}\rangle $ 

$ 0.0103^{+0.0012}_{-0.0012} $ 

$ 0.0135^{+0.0011}_{-0.0011} $ 

$ 0.0116^{+0.0011}_{-0.0011} $ 

$ \langle\widehat{I}^{a_{1}}_{5\parallel}\rangle $ 

$ 0.0408^{+0.0008}_{-0.0008} $ 

$ 0.0124^{+0.0005}_{-0.0005} $ 

$ 0.0193^{+0.0007}_{-0.0007} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\parallel}\rangle $ 

$ 0.0136^{+0.0002}_{-0.0002} $ 

$ 0.0029^{+0.0002}_{-0.0002} $ 

$ 0.0053^{+0.0002}_{-0.0002} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\parallel}\rangle $ 

0 0 0 Table 24. Same as that in Table 23 but for
$ q^2 = 1.0 - 2.0 $ GeV2 bin.$ q^{2}= 2.0 - 3.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\parallel}\rangle $ 

$ 0.0111^{+0.0003}_{-0.0003} $ 

$ 0.0072^{+0.0004}_{-0.0004} $ 

$ 0.0076^{+0.0004}_{-0.0004} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\parallel}\rangle $ 

$ 0.2733^{+0.0004}_{-0.0004} $ 

$ 0.2792^{+0.0006}_{-0.0006} $ 

$ 0.2786^{+0.0005}_{-0.0005} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\parallel}\rangle $ 

$ 0.0037^{+0.0001}_{-0.0001} $ 

$ 0.0024^{+0.0001}_{-0.0001} $ 

$ 0.0025^{+0.0001}_{-0.0001} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\parallel}\rangle $ 

$ -0.2675^{+0.0002}_{-0.0002} $ 

$ -0.2705^{+0.0005}_{-0.0005} $ 

$ -0.2705^{+0.0004}_{-0.0004} $ 

$ \langle \widehat{I}^{a_{1}}_{3\parallel}\rangle $ 

$ -0.0035^{+0.0003}_{-0.0003} $ 

$ -0.0040^{+0.0003}_{-0.0003} $ 

$ -0.0037^{+0.0003}_{-0.0003} $ 

$ \langle\widehat{I}^{a_{1}}_{4\parallel}\rangle $ 

$ 0.0305^{+0.0014}_{-0.0014} $ 

$ 0.0329^{+0.0012}_{0.0012} $ 

$ 0.0314^{+0.0013}_{-0.0013} $ 

$ \langle\widehat{I}^{a_{1}}_{5\parallel}\rangle $ 

$ 0.0255^{+0.0011}_{-0.0011} $ 

$ 0.0043^{+0.0006}_{-0.0006} $ 

$ 0.0090^{+0.0007}_{-0.0007} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\parallel}\rangle $ 

$ 0.0088^{+0.0005}_{-0.0005} $ 

$ -0.0014^{+0.0005}_{-0.0005} $ 

$ 0.0001^{+0.0001}_{-0.0001} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\parallel}\rangle $ 

0 0 0 Table 25. Same as that in Table 23 but for
$ q^2 = 2.0 - 3.0 $ GeV2 bin.$ q^{2}= 3.0 - 4.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\parallel}\rangle $ 

$ 0.0158^{+0.0006}_{-0.0006} $ 

$ 0.0135^{+0.0007}_{-0.0007} $ 

$ 0.0134^{+0.0007}_{-0.0007} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\parallel}\rangle $ 

$ 0.2666^{+0.0008}_{-0.0008} $ 

$ 0.2702^{+0.0010}_{-0.0010} $ 

$ 0.2702^{+0.0001}_{-0.0001} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\parallel}\rangle $ 

$ 0.0053^{+0.0002}_{-0.0002} $ 

$ 0.0044^{+0.0002}_{-0.0002} $ 

$ 0.0044^{+0.0002}_{-0.0002} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\parallel}\rangle $ 

$ -0.2625^{+0.0007}_{-0.0007} $ 

$ -0.2642^{+0.0009}_{-0.0009} $ 

$ -0.2647^{+0.0009}_{-0.0009} $ 

$ \langle\widehat{I}^{a_{1}}_{3\parallel}\rangle $ 

$ -0.0076^{+0.0004}_{-0.0004} $ 

$ -0.0082^{+0.0004}_{-0.0004} $ 

$ -0.0078^{+0.0003}_{-0.0003} $ 

$ \langle\widehat{I}^{a_{1}}_{4\parallel}\rangle $ 

$ 0.0448^{+0.0014}_{-0.0014} $ 

$ 0.0467^{+0.0012}_{-0.0012} $ 

$ 0.0455^{+0.0013}_{-0.0013} $ 

$ \langle\widehat{I}^{a_{1}}_{5\parallel}\rangle $ 

$ 0.0155^{+0.0012}_{-0.0012} $ 

$ -0.0011^{+0.0007}_{-0.0007} $ 

$ 0.0021^{+0.0008}_{-0.0008} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\parallel}\rangle $ 

$ 0.0030^{+0.0009}_{-0.0009} $ 

$ 0.0060^{+0.0007}_{-0.0007} $ 

$ 0.0048^{+0.0008}_{-0.0008} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\parallel}\rangle $ 

0 0 0 Table 26. Same as that in Table 23 but for
$ q^2 = 3.0 - 4.0 $ GeV2 bin.$ q^{2}= 4.0 - 5.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\parallel}\rangle $ 

$ 0.0224^{+0.0009}_{-0.0009} $ 

$ 0.0209^{+0.0010}_{-0.0010} $ 

$ 0.0206^{+0.0009}_{-0.0009} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\parallel}\rangle $ 

$ 0.2575^{+0.0012}_{-0.0012} $ 

$ 0.2599^{+0.0013}_{-0.0013} $ 

$ 0.2603^{+0.0010}_{-0.0010} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\parallel}\rangle $ 

$ 0.0075^{+0.0003}_{-0.0003} $ 

$ 0.0069^{+0.0003}_{-0.0003} $ 

$ 0.0068^{+0.0003}_{-0.0003} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\parallel}\rangle $ 

$ -0.2545^{+0.0010}_{-0.0010} $ 

$ -0.2554^{+0.0012}_{-0.0012} $ 

$ -0.2561^{+0.0012}_{-0.0012} $ 

$ \langle\widehat{I}^{a_{1}}_{3\parallel}\rangle $ 

$ -0.0121^{+0.0005}_{-0.0005} $ 

$ -0.0126^{+0.0005}_{-0.0005} $ 

$ -0.0123^{+0.0005}_{-0.0005} $ 

$ \langle\widehat{I}^{a_{1}}_{4\parallel}\rangle $ 

$ 0.0561^{+0.0013}_{-0.0013} $ 

$ 0.0576^{+0.0012}_{-0.0012} $ 

$ 0.0567^{+0.0012}_{-0.0012} $ 

$ \langle\widehat{I}^{a_{1}}_{5\parallel}\rangle $ 

$ 0.0082^{+0.0013}_{-0.0013} $ 

$ -0.0051^{+0.0007}_{-0.0007} $ 

$ 0.0029^{+0.0009}_{-0.0009} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\parallel}\rangle $ 

$ -0.0032^{+0.0012}_{-0.0012} $ 

$ -0.0109^{+0.0008}_{-0.0008} $ 

$ -0.0104^{+0.0001}_{-0.0001} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\parallel}\rangle $ 

0 0 0 Table 27. Same as that in Table 23 but for
$ q^2 = 4.0 - 5.0 $ GeV2 bin.$ q^{2}= 5.0 - 6.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\parallel}\rangle $ 

$ 0.0301^{+0.0011}_{-0.0011} $ 

$ 0.0291^{+0.0011}_{-0.0011} $ 

$ 0.0286^{+0.0013}_{-0.0013} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\parallel}\rangle $ 

$ 0.2471^{+0.0014}_{-0.0014} $ 

$ 0.2487^{+0.0015}_{-0.0015} $ 

$ 0.2493^{+0.0015}_{-0.0015} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\parallel}\rangle $ 

$ 0.0100^{+0.0004}_{-0.0004} $ 

$ 0.0096^{+0.0004}_{-0.0004} $ 

$ 0.0095^{+0.0038}_{-0.0038} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\parallel}\rangle $ 

$ -0.2448^{+0.0013}_{-0.0013} $ 

$ -0.2452^{+0.0015}_{-0.0015} $ 

$ -0.2460^{+0.0015}_{-0.0015} $ 

$ \langle\widehat{I}^{a_{1}}_{3\parallel}\rangle $ 

$ -0.0169^{+0.0006}_{-0.0006} $ 

$ -0.0174^{+0.0006}_{-0.0006} $ 

$ -0.0170^{+0.0006}_{-0.0006} $ 

$ \langle\widehat{I}^{a_{1}}_{4\parallel}\rangle $ 

$ 0.0655^{+0.0011}_{-0.0011} $ 

$ 0.0666^{+0.0010}_{-0.0010} $ 

$ 0.0659^{+0.0011}_{-0.0011} $ 

$ \langle\widehat{I}^{a_{1}}_{5\parallel}\rangle $ 

$ 0.0025^{+0.0013}_{-0.0013} $ 

$ -0.0082^{+0.0007}_{-0.0007} $ 

$ 0.0068^{+0.0009}_{-0.0009} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\parallel}\rangle $ 

$ -0.0096^{+0.0014}_{-0.0014} $ 

$ -0.0158^{+0.0010}_{-0.0010} $ 

$ -0.0162^{+0.0013}_{-0.0013} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\parallel}\rangle $ 

0 0 0 Table 28. Same as that in Table 23 but for
$ q^2 = 5.0 - 6.0 $ GeV2 bin.$ q^{2}= 6.0 - 7.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\parallel}\rangle $ 

$ 0.0386^{+0.0012}_{-0.0012} $ 

$ 0.0379^{+0.0013}_{-0.0013} $ 

$ 0.0374^{+0.0013}_{-0.0013} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\parallel}\rangle $ 

$ 0.2357^{+0.0016}_{-0.0016} $ 

$ 0.2368^{+0.0017}_{-0.0017} $ 

$ 0.2374^{+0.0017}_{-0.0017} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\parallel}\rangle $ 

$ 0.0128^{+0.0004}_{-0.0004} $ 

$ 0.0125^{+0.0004}_{-0.0004} $ 

$ 0.0124^{+0.00004}_{-0.00004} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\parallel}\rangle $ 

$ -0.2338^{+0.0015}_{-0.0015} $ 

$ -0.2340^{+0.0017}_{-0.0017} $ 

$ -0.2348^{+0.0016}_{-0.0016} $ 

$ \langle\widehat{I}^{a_{1}}_{3,\parallel}\rangle $ 

$ -0.0220^{+0.0006}_{-0.0006} $ 

$ -0.0224^{+0.0006}_{-0.0006} $ 

$ -0.0221^{+0.0007}_{-0.0007} $ 

$ \langle\widehat{I}^{a_{1}}_{4,\parallel}\rangle $ 

$ 0.0734^{+0.0010}_{-0.0010} $ 

$ 0.0743^{+0.0009}_{-0.0009} $ 

$ 0.0737^{+0.0012}_{-0.0012} $ 

$ \langle\widehat{I}^{a_{1}}_{5,\parallel}\rangle $ 

$ -0.0021^{+0.0012}_{-0.0012} $ 

$ -0.0106^{+0.0007}_{-0.0007} $ 

$ -0.0091^{+0.0008}_{-0.0008} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\parallel}\rangle $ 

$ -0.0162^{+0.0016}_{-0.0016} $ 

$ -0.0209^{+0.0011}_{-0.0011} $ 

$ -0.0220^{+0.0013}_{-0.0013} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\parallel}\rangle $ 

0 0 0 Table 29. Same as that in Table 23 but for
$ q^2 = 6.0 - 7.0 $ GeV2 bin.$ q^{2}= 7.0 - 8.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\parallel}\rangle $ 

$ 0.0478^{+0.0013}_{-0.0013} $ 

$ 0.0201^{+0.0008}_{-0.0008} $ 

$ 0.0467^{+0.0013}_{-0.0013} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\parallel}\rangle $ 

$ 0.2233^{+0.0017}_{-0.0017} $ 

$ 0.2241^{+0.0018}_{-0.0018} $ 

$ 0.2248^{+0.0018}_{-0.0018} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\parallel}\rangle $ 

$ 0.0159^{+0.0004}_{-0.0004} $ 

$ 0.0157^{+0.0004}_{-0.0004} $ 

$ 0.0155^{+0.0004}_{-0.0004} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\parallel}\rangle $ 

$ -0.2218^{+0.0016}_{-0.0016} $ 

$ -0.2218^{+0.0017}_{-0.0017} $ 

$ -0.2227^{+0.0018}_{-0.0018} $ 

$ \langle\widehat{I}^{a_{1}}_{3,\parallel}\rangle $ 

$ -0.0274^{+0.0006}_{-0.0006} $ 

$ -0.0278^{+0.0006}_{-0.0006} $ 

$ -0.0275^{+0.0006}_{-0.0006} $ 

$ \langle\widehat{I}^{a_{1}}_{4,\parallel}\rangle $ 

$ 0.0801^{+0.0008}_{-0.0008} $ 

$ 0.0808^{+0.0007}_{-0.0007} $ 

$ 0.0804^{+0.0007}_{-0.0007} $ 

$ \langle\widehat{I}^{a_{1}}_{5,\parallel}\rangle $ 

$ -0.0058^{+0.0012}_{-0.0012} $ 

$ -0.0126^{+0.0006}_{-0.0006} $ 

$ -0.0125^{+0.0008}_{-0.0008} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\parallel}\rangle $ 

$ -0.0229^{+0.0017}_{-0.0017} $ 

$ -0.0260^{+0.0011}_{-0.0011} $ 

$ -0.0226^{+0.0017}_{-0.0017} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\parallel}\rangle $ 

0 0 0 Table 30. Same as that in Table 23 but for
$ q^2 = 7.0 - 8.0 $ GeV2 bin.$ q^{2}= 0.1 - 1.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\perp}\rangle $ 

$ 0.3423^{+0.0002}_{-0.0002} $ 

$ 0.3574^{+0.0002}_{-0.0002} $ 

$ 0.3533^{+0.0002}_{-0.0002} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\perp}\rangle $ 

$ 0.1010^{+0.0018}_{-0.0018} $ 

$ 0.0465^{+0.0013}_{-0.0013} $ 

$ 0.0613^{+0.0092}_{-0.0092} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\perp}\rangle $ 

$ -0.2345^{+0.0020}_{-0.0020} $ 

$ -0.2641^{+0.0012}_{-0.0012} $ 

$ -0.2558^{+0.0015}_{-0.0015} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\perp}\rangle $ 

$ 0.0260^{+0.0005}_{-0.0005} $ 

$ 0.0117^{+0.0004}_{-0.0004} $ 

$ 0.0156^{+0.0004}_{-0.0004} $ 

$ \langle\widehat{I}^{a_{1}}_{3,\perp}\rangle $ 

$ 0.0008^{+0.00003}_{-0.00003} $ 

$ 0.0009^{+0.00003}_{-0.00003} $ 

$ 0.0001^{+0.00003}_{-0.00003} $ 

$ \langle\widehat{I}^{a_{1}}_{4,\perp}\rangle $ 

$ 0.0267^{+0.0008}_{-0.0008} $ 

$ 0.0224^{+0.0009}_{-0.0009} $ 

$ 0.0252^{+0.0009}_{-0.0009} $ 

$ \langle\widehat{I}^{a_{1}}_{5,\perp}\rangle $ 

$ -0.0780^{+0.0002}_{-0.0002} $ 

$ -0.0324^{+0.0003}_{-0.0003} $ 

$ -0.0447^{+0.0003}_{-0.0003} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\perp}\rangle $ 

$ 0.0162^{+0.0002}_{-0.0002} $ 

$ 0.0063^{+0.00002}_{-0.00002} $ 

$ 0.0089^{+0.0001}_{-0.0001} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\perp}\rangle $ 

$ 0.0324^{+0.0005}_{-0.0005} $ 

$ 0.0126^{+0.00005}_{-0.00005} $ 

$ 0.0178^{+0.0001}_{-0.0001} $ 

Table 31. Predictions of averaged values of angular observables for the
$ B\to a_{1}(\to\rho_{\perp}\pi)\mu^{+}\mu^{-} $ decay, in$ q^2 = 0.1 - 1.0 $ GeV2 bin, for the SM as well as the NP scenarios (S1, S2) of the$ Z^{\prime} $ model listed in Table 5. The errors presented mainly arise from the uncertainties of the form factors.$ q^{2}= 1.0 - 2.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\perp}\rangle $ 

$ 0.3486^{+0.00002}_{-0.00002} $ 

$ 0.3531^{+0.00005}_{-0.00005} $ 

$ 0.3533^{+0.00002}_{-0.00002} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\perp}\rangle $ 

$ 0.0272^{+0.0004}_{-0.0004} $ 

$ 0.0092^{+0.00008}_{-0.00008} $ 

$ 0.0126^{+0.0001}_{-0.0001} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\perp}\rangle $ 

$ -0.3179^{+0.0007}_{-0.0007} $ 

$ -0.3284^{+0.00003}_{-0.00003} $ 

$ -0.3266^{+0.0002}_{-0.0002} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\perp}\rangle $ 

$ 0.0088^{+0.0001}_{-0.0001} $ 

$ 0.0030^{+0.00003}_{-0.00003} $ 

$ 0.0041^{+0.0002}_{-0.0002} $ 

$ \langle\widehat{I}^{a_{1}}_{3,\perp}\rangle $ 

$ 0.0030^{+0.00009}_{-0.00009} $ 

$ 0.0031^{+0.00009}_{-0.00009} $ 

$ 0.0031^{+0.0008}_{-0.0008} $ 

$ \langle\widehat{I}^{a_{1}}_{4,\perp}\rangle $ 

$ -0.0126^{+0.0015}_{-0.0015} $ 

$ -0.0165^{+0.0014}_{-0.0014} $ 

$ -0.0141^{+0.0011}_{-0.0011} $ 

$ \langle\widehat{I}^{a_{1}}_{5,\perp}\rangle $ 

$ -0.0498^{+0.0010}_{-0.0010} $ 

$ -0.0151^{+0.0006}_{-0.0006} $ 

$ -0.0236^{+0.0008}_{-0.0008} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\perp}\rangle $ 

$ 0.0167^{+0.0002}_{-0.0002} $ 

$ 0.0035^{+0.0003}_{-0.0003} $ 

$ 0.0065^{+0.0003}_{-0.0003} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\perp}\rangle $ 

$ 0.0333^{+0.0004}_{-0.0004} $ 

$ 0.0070^{+0.0005}_{-0.0005} $ 

$ 0.0131^{+0.0006}_{-0.0006} $ 

Table 32. Same as that in Table 31 but for
$ q^2 = 1.0 - 2.0 $ GeV2 bin.$ q^{2}= 2.0 - 3.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\perp}\rangle $ 

$ 0.3473^{+0.0002}_{-0.0002} $ 

$ 0.3497^{+0.0002}_{-0.0002} $ 

$ 0.3494^{+0.0002}_{-0.0002} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\perp}\rangle $ 

$ 0.0271^{+0.0006}_{-0.0006} $ 

$ 0.0176^{+0.0010}_{-0.0010} $ 

$ 0.0185^{+0.0009}_{-0.0009} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\perp}\rangle $ 

$ -0.3220^{+0.0003}_{-0.0003} $ 

$ -0.3274^{+0.0008}_{-0.0008} $ 

$ -0.3272^{+0.0006}_{-0.0006} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\perp}\rangle $ 

$ 0.0090^{+0.0002}_{-0.0002} $ 

$ 0.0058^{+0.0003}_{-0.0003} $ 

$ 0.0061^{+0.0003}_{-0.0003} $ 

$ \langle\widehat{I}^{a_{1}}_{3,\perp}\rangle $ 

$ 0.0055^{+0.0001}_{-0.0001} $ 

$ 0.0055^{+0.0001}_{-0.0001} $ 

$ 0.0055^{+0.0001}_{-0.0001} $ 

$ \langle\widehat{I}^{a_{1}}_{4,\perp}\rangle $ 

$ -0.0372^{+0.0017}_{-0.0017} $ 

$ -0.0401^{+0.0015}_{-0.0015} $ 

$ -0.0383^{+0.0016}_{-0.0016} $ 

$ \langle\widehat{I}^{a_{1}}_{5,\perp}\rangle $ 

$ -0.0312^{+0.0013}_{-0.0013} $ 

$ -0.0052^{+0.0008}_{-0.0008} $ 

$ -0.0109^{+0.0009}_{-0.0009} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\perp}\rangle $ 

$ 0.0107^{+0.0007}_{-0.0007} $ 

$ -0.0017^{+0.0006}_{-0.0006} $ 

$ 0.0069^{+0.0006}_{-0.0006} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\perp}\rangle $ 

$ 0.0215^{+0.0013}_{-0.0013} $ 

$ -0.0034^{+0.0011}_{-0.0011} $ 

$ 0.0014^{+0.0013}_{-0.0013} $ 

Table 33. Same as that in Table 31 but for
$ q^2 = 2.0 - 3.0 $ GeV2 bin.$ q^{2}= 3.0 - 4.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\perp}\rangle $ 

$ 0.3448^{+0.0003}_{-0.0003} $ 

$ 0.3463^{+0.0003}_{-0.0003} $ 

$ 0.3462^{+0.0003}_{-0.0003} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\perp}\rangle $ 

$ 0.0386^{+0.0014}_{-0.0014} $ 

$ 0.0328^{+0.0018}_{-0.0018} $ 

$ 0.0326^{+0.0017}_{-0.0017} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\perp}\rangle $ 

$ -0.3141^{+0.0011}_{-0.0011} $ 

$ -0.3172^{+0.0014}_{-0.0014} $ 

$ -0.3178^{+0.0013}_{-0.0013} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\perp}\rangle $ 

$ 0.0128^{+0.0005}_{-0.0005} $ 

$ 0.0108^{+0.0006}_{-0.0006} $ 

$ 0.0108^{+0.0005}_{-0.0005} $ 

$ \langle\widehat{I}^{a_{1}}_{3,\perp}\rangle $ 

$ 0.0081^{+0.0002}_{-0.0002} $ 

$ 0.0081^{+0.0002}_{-0.0002} $ 

$ 0.0081^{+0.0002}_{-0.0002} $ 

$ \langle\widehat{I}^{a_{1}}_{4,\perp}\rangle $ 

$ -0.0547^{+0.0017}_{-0.0017} $ 

$ -0.0570^{+0.0015}_{-0.0015} $ 

$ -0.0556^{+0.0016}_{-0.0016} $ 

$ \langle\widehat{I}^{a_{1}}_{5,\perp}\rangle $ 

$ -0.0190^{+0.0015}_{-0.0015} $ 

$ -0.0013^{+0.0009}_{-0.0009} $ 

$ -0.0027^{+0.0011}_{-0.0011} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\perp}\rangle $ 

$ 0.0037^{+0.0011}_{-0.0011} $ 

$ -0.0073^{+0.0008}_{-0.0008} $ 

$ 0.0051^{+0.0009}_{-0.0009} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\perp}\rangle $ 

$ 0.0074^{+0.0022}_{-0.0022} $ 

$ -0.0147^{+0.0016}_{-0.0016} $ 

$ 0.0116^{+0.0019}_{-0.0019} $ 

Table 34. Same as that in Table 31 but for
$ q^2 = 3.0 - 4.0 $ GeV2 bin.$ q^{2}= 4.0 - 5.0 $ 

$\rm GeV^{2}$ 

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\perp}\rangle $ 

$ 0.3418^{+0.0004}_{-0.0004} $ 

$ 0.3428^{+0.0004}_{-0.0004} $ 

$ 0.3429^{+0.0005}_{-0.0005} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\perp}\rangle $ 

$ 0.0548^{+0.0021}_{-0.0021} $ 

$ 0.0510^{+0.0024}_{-0.0024} $ 

$ 0.0502^{+0.0023}_{-0.0023} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\perp}\rangle $ 

$ -0.3017^{+0.0016}_{-0.0016} $ 

$ -0.3034^{+0.0019}_{-0.0019} $ 

$ -0.3044^{+0.0019}_{-0.0019} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\perp}\rangle $ 

$ 0.0182^{+0.0007}_{-0.0007} $ 

$ 0.0168^{+0.0008}_{-0.0008} $ 

$ 0.0166^{+0.0008}_{-0.0008} $ 

$ \langle\widehat{I}^{a_{1}}_{3,\perp}\rangle $ 

$ 0.0109^{+0.0002}_{-0.0002} $ 

$ 0.0109^{+0.0002}_{-0.0002} $ 

$ 0.0109^{+0.0002}_{-0.0002} $ 

$ \langle\widehat{I}^{a_{1}}_{4,\perp}\rangle $ 

$ -0.0685^{+0.0016}_{-0.0016} $ 

$ -0.0703^{+0.0014}_{-0.0014} $ 

$ -0.0691^{+0.0014}_{-0.0014} $ 

$ \langle\widehat{I}^{a_{1}}_{5,\perp}\rangle $ 

$ -0.0100^{+0.0015}_{-0.0015} $ 

$ -0.0062^{+0.0009}_{-0.0009} $ 

$ -0.0035^{+0.0011}_{-0.0011} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\perp}\rangle $ 

$ -0.0039^{+0.0014}_{-0.0014} $ 

$ -0.0133^{+0.0010}_{-0.0010} $ 

$ -0.0127^{+0.0012}_{-0.0012} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\perp}\rangle $ 

$ -0.0078^{+0.0029}_{-0.0029} $ 

$ -0.0265^{+0.0020}_{-0.0020} $ 

$ -0.0253^{+0.0024}_{-0.0024} $ 

Table 35. Same as that in Table 31 but for
$ q^2 = 4.0 - 5.0 $ GeV2 bin.$ q^{2}= 5.0 - 6.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\perp}\rangle $ 

$ 0.3411^{+0.0005}_{-0.0005} $ 

$ 0.3392^{+0.0005}_{-0.0005} $ 

$ 0.3394^{+0.0005}_{-0.0005} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\perp}\rangle $ 

$ 0.0735^{+0.0026}_{-0.0026} $ 

$ 0.0710^{+0.0028}_{-0.0028} $ 

$ 0.0699^{+0.0028}_{-0.0028} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\perp}\rangle $ 

$ -0.2866^{+0.0021}_{-0.0021} $ 

$ -0.2877^{+0.0023}_{-0.0023} $ 

$ -0.2888^{+0.0023}_{-0.0023} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\perp}\rangle $ 

$ 0.0244^{+0.0009}_{-0.0009} $ 

$ 0.0235^{+0.0009}_{-0.0009} $ 

$ 0.0314^{+0.0009}_{-0.0009} $ 

$ \langle\widehat{I}^{a_{1}}_{3,\perp}\rangle $ 

$ 0.0139^{+0.0002}_{-0.0002} $ 

$ 0.0140^{+0.0002}_{-0.0002} $ 

$ 0.0140^{+0.0001}_{-0.0001} $ 

$ \langle\widehat{I}^{a_{1}}_{4,\perp}\rangle $ 

$ -0.0800^{+0.0014}_{-0.0014} $ 

$ -0.0814^{+0.0013}_{-0.0013} $ 

$ -0.0805^{+0.0013}_{-0.0013} $ 

$ \langle\widehat{I}^{a_{1}}_{5,\perp}\rangle $ 

$ -0.0030^{+0.0015}_{-0.0015} $ 

$ -0.0100^{+0.0009}_{-0.0009} $ 

$ -0.0083^{+0.0011}_{-0.0011} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\perp}\rangle $ 

$ -0.0118^{+0.0017}_{-0.0017} $ 

$ -0.0194^{+0.0012}_{-0.0012} $ 

$ -0.0197^{+0.0014}_{-0.0014} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\perp}\rangle $ 

$ -0.0235^{+0.0035}_{-0.0035} $ 

$ -0.0387^{+0.0024}_{-0.0024} $ 

$ -0.0395^{+0.0028}_{-0.0028} $ 

Table 36. Same as that in Table 31 but for
$ q^2 = 5.0 - 6.0 $ GeV2 bin.$ q^{2}= 6.0 - 7.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\perp}\rangle $ 

$ 0.3348^{+0.0005}_{-0.0005} $ 

$ 0.3354^{+0.0005}_{-0.0005} $ 

$ 0.3355^{+0.0005}_{-0.0005} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\perp}\rangle $ 

$ 0.0943^{+0.0029}_{-0.0029} $ 

$ 0.0926^{+0.0031}_{-0.0031} $ 

$ 0.0699^{+0.0028}_{-0.0028} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\perp}\rangle $ 

$ -0.2698^{+0.0023}_{-0.0023} $ 

$ -0.2703^{+0.0025}_{-0.0025} $ 

$ -0.2716^{+0.0025}_{-0.0025} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\perp}\rangle $ 

$ 0.0313^{+0.0010}_{-0.0010} $ 

$ 0.0306^{+0.0010}_{-0.0010} $ 

$ 0.0302^{+0.0010}_{-0.0010} $ 

$ \langle\widehat{I}^{a_{1}}_{3,\perp}\rangle $ 

$ 0.0173^{+0.0002}_{-0.0002} $ 

$ 0.0173^{+0.0002}_{-0.0002} $ 

$ 0.0174^{+0.0002}_{-0.0002} $ 

$ \langle\widehat{I}^{a_{1}}_{4,\perp}\rangle $ 

$ -0.0896^{+0.0012}_{-0.0012} $ 

$ -0.0907^{+0.0011}_{-0.0011} $ 

$ -0.0174^{+0.0001}_{-0.0013} $ 

$ \langle\widehat{I}^{a_{1}}_{5,\perp}\rangle $ 

$ -0.0026^{+0.0015}_{-0.0015} $ 

$ -0.0130^{+0.0008}_{-0.0008} $ 

$ -0.0122^{+0.0011}_{-0.0011} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\perp}\rangle $ 

$ -0.0198^{+0.0020}_{-0.0020} $ 

$ -0.0256^{+0.0013}_{-0.0013} $ 

$ -0.0270^{+0.0016}_{-0.0016} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\perp}\rangle $ 

$ -0.0397^{+0.0039}_{-0.0039} $ 

$ -0.0511^{+0.0026}_{-0.0026} $ 

$ -0.0539^{+0.0031}_{-0.0031} $ 

Table 37. Same as that in Table 31 but for
$ q^2 = 6.0 - 7.0 $ GeV2 bin.$ q^{2}= 7.0 - 8.0 $ GeV2

Angular Observables SM $ Z^{\prime} $ Scenario 1

$ Z^{\prime} $ Scenario 2

$ \langle\widehat{I}^{a_{1}}_{1s,\perp}\rangle $ 

$ 0.3310^{+0.0005}_{-0.001} $ 

$ 0.3314^{+0.0006}_{-0.0006} $ 

$ 0.3355^{+0.0005}_{-0.0005} $ 

$ \langle\widehat{I}^{a_{1}}_{1c,\perp}\rangle $ 

$ 0.1166^{+0.0031}_{-0.0031} $ 

$ 0.1155^{+0.0032}_{-0.0032} $ 

$ 0.1142^{+0.0033}_{-0.0033} $ 

$ \langle\widehat{I}^{a_{1}}_{2s,\perp}\rangle $ 

$ -0.2514^{+0.0025}_{-0.0025} $ 

$ -0.2517^{+0.0027}_{-0.0027} $ 

$ -0.2529^{+0.0025}_{-0.0025} $ 

$ \langle\widehat{I}^{a_{1}}_{2c,\perp}\rangle $ 

$ 0.0388^{+0.0010}_{-0.0010} $ 

$ 0.0383^{+0.0011}_{-0.0011} $ 

$ 0.03785^{+0.0011}_{-0.0011} $ 

$ \langle\widehat{I}^{a_{1}}_{3,\perp}\rangle $ 

$ 0.0210^{+0.0002}_{-0.0002} $ 

$ 0.0210^{+0.0001}_{-0.0001} $ 

$ 0.0211^{+0.0001}_{-0.0001} $ 

$ \langle\widehat{I}^{a_{1}}_{4,\perp}\rangle $ 

$ -0.0978^{+0.0010}_{-0.0010} $ 

$ -0.0987^{+0.0009}_{-0.0009} $ 

$ -0.0982^{+0.0009}_{-0.0009} $ 

$ \langle\widehat{I}^{a_{1}}_{5,\perp}\rangle $ 

$ 0.0071^{+0.0015}_{-0.0015} $ 

$ 0.0153^{+0.0008}_{-0.0008} $ 

$ 0.0152^{+0.0001}_{-0.0001} $ 

$ \langle\widehat{I}^{a_{1}}_{6s,\perp}\rangle $ 

$ -0.0280^{+0.0021}_{-0.0021} $ 

$ -0.0318^{+0.0014}_{-0.0014} $ 

$ -0.0343^{+0.0016}_{-0.0016} $ 

$ \langle\widehat{I}^{a_{1}}_{6c,\perp}\rangle $ 

$ -0.0560^{+0.0043}_{-0.0043} $ 

$ -0.0636^{+0.0027}_{-0.0027} $ 

$ -0.0685^{+0.0033}_{-0.0033} $ 

Table 38. Same as that in Table 31 but for
$ q^2 = 7.0 - 8.0 $ GeV2 bin.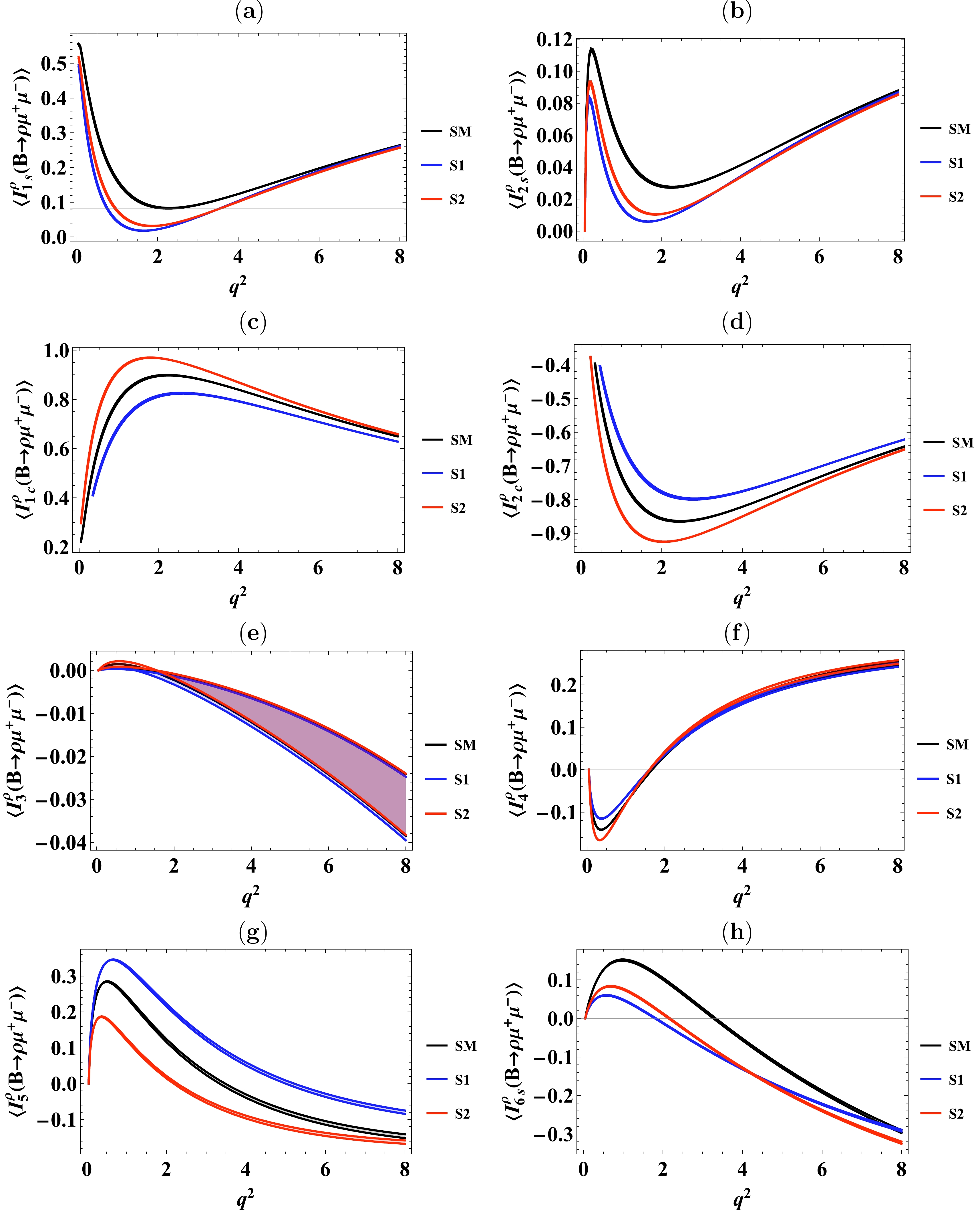
Figure 4. (color online) Angular observables
$ \langle I^{\rho}_{1s}\rangle, \langle I^{\rho}_{2s}\rangle, \langle I^{\rho}_{1c}\rangle, \langle I^{\rho}_{2c}\rangle, \langle I^{\rho}_{3}\rangle, \langle I^{\rho}_{4}\rangle, \langle I^{\rho}_{5}\rangle $ , and$ \langle I^{\rho}_{6s}\rangle $ for the decay$ B\to\rho(\to\pi\pi)\mu^{+}\mu^{-} $ in the SM and two scenarios of the family non-universal$ Z^{\prime} $ model.
Figure 6. (color online) Angular observables
$ \langle \widehat{I}^{a_{1}}_{1s,\perp}\rangle, \langle \widehat{I}^{a_{1}}_{1c,\perp}\rangle, \langle \widehat{I}^{a_{1}}_{2s,\perp}\rangle $ ,$ \langle \widehat{I}^{a_{1}}_{2c,\perp}\rangle $ ,$ \langle \widehat{I}^{a_1}_{3,\perp}\rangle,\langle \widehat{I}^{a_1}_{4,\perp}\rangle,\langle \widehat{I}^{a_1}_{5,\perp}\rangle $ , and$ \langle \widehat{I}^{a_1}_{6s,\perp}\rangle $ for the decay$ B\to a_{1}(\to\rho_{\perp}\pi)\mu^{+}\mu^{-} $ in the SM and two scenarios of the family non-universal$ Z^{\prime} $ model.● Figure 4 depicts the normalized angular observables
$ \langle I^{\rho}_{1s}\rangle, \langle I^{\rho}_{2s}\rangle, \langle I^{\rho}_{1c}\rangle, \langle I^{\rho}_{2c}\rangle, \langle I^{\rho}_{3}\rangle, \langle I^{\rho}_{4}\rangle, \langle I^{\rho}_{5}\rangle $ , and$ \langle I^{\rho}_{6s}\rangle $ for the$B\to\rho(\to \pi\pi)\mu^{+}\mu^{-}$ decay in the SM and two scenarios S1 and S2 of the family non universal$ Z^{\prime} $ model. Figs. 4(a)−4(d) show clear discrimination between the SM predictions and the two scenarios, S1 and S2, of the family non-universal$ Z^{\prime} $ model for the angular observables$ \langle I^{\rho}_{1s}\rangle,\langle I^{\rho}_{2s}\rangle,\langle I^{\rho}_{1c}\rangle $ , and$ \langle I^{\rho}_{2c}\rangle $ in the range of$ q^{2}=(0.1-7) $ $ {\rm{GeV}}^{2} $ . Furthermore, the angular observables$\langle I^{\rho}_{3}\rangle, \langle I^{\rho}_{4}\rangle, \langle I^{\rho}_{5}\rangle$ , and$ \langle I^{\rho}_{6s}\rangle $ are shown in Fig. 4(e)−4(h). For observables$ \langle I^{\rho}_{3}\rangle $ and$ \langle I^{\rho}_{4}\rangle $ shown in Fig. 4(e) and 4(f), the SM predictions and effects of the family non-universal$ Z^{\prime} $ model overlap each other. Hence, no discrimination can be observed for these observables, except for some region of$ q^2 $ near$ 0.5 $ $ {\rm{GeV}}^{2} $ for$ \langle I^{\rho}_{4}\rangle $ . For observables$ \langle I^{\rho}_{5}\rangle $ and$ \langle I^{\rho}_{6s}\rangle $ , the discrimination between the SM and two scenarios of the family non-universal$ Z^{\prime} $ model can be determined for almost the whole kinematical range of$ q^2 $ . Furthermore, the two scenarios remain distinguishable from each other in almost the entire$ q^2 $ range.● Figure 5 presents the normalized angular observables
$ \langle \widehat{I}^{a_1}_{1s,\parallel}\rangle,\langle \widehat{I}^{a_1}_{1c.\parallel}\rangle,\langle \widehat{I}^{a_1}_{2s,\parallel}\rangle,\langle \widehat{I}^{a_1}_{2c,\parallel}\rangle,\langle \widehat{I}^{a_1}_{3,\parallel}\rangle,\langle \widehat{I}^{a_1}_{4,\parallel}\rangle $ ,$ \langle \widehat{I}^{a_1}_{5,\parallel}\rangle $ , and$ \langle \widehat{I}^{a_1}_{6s,\parallel}\rangle $ for the$ B\to a_{1}(\to\rho_{\parallel}\pi)\mu^{+}\mu^{-} $ decay in the SM as well as in S1 and S2 of the family non universal$ Z^{\prime} $ model. Figs. 5(a)−5(d) show some discrimination between the SM predictions and two scenarios S1 and S2 of the family non-universal$ Z^{\prime} $ model for the angular observables$\langle \widehat{I}^{a_1}_{1s,\parallel}\rangle, \langle \widehat{I}^{a_1}_{1c.\parallel}\rangle,\langle \widehat{I}^{a_1}_{2s,\parallel}\rangle,\langle \widehat{I}^{a_1}_{2c,\parallel}\rangle$ in the range of$ q^{2}=(0.1-3) $ $ {\rm{GeV}}^{2} $ , whereas the two scenarios are not much distinct from each other. Furthermore, angular observables$ \langle \widehat{I}^{a_1}_{3,\parallel}\rangle,\langle \widehat{I}^{a_1}_{4,\parallel}\rangle $ ,$ \langle \widehat{I}^{a_1}_{5,\parallel}\rangle $ , and$ \langle \widehat{I}^{a_1}_{6s,\parallel}\rangle $ are shown in Fig. 5(e)−5(h). For observables$ \langle \widehat{I}^{a_1}_{3,\parallel}\rangle $ and$ \langle \widehat{I}^{a_1}_{4,\parallel}\rangle $ , no segregation is observed among the SM and the S1 and S2 scenarios of the family non-universal$ Z^{\prime} $ model as they are found to be largely overlapping each other as shown in Fig. 5(e) and 5(f). For observables$ \langle \widehat{I}^{a_1}_{5,\parallel}\rangle $ and$ \langle \widehat{I}^{a_1}_{6s,\parallel}\rangle $ , the discrimination between the SM and two scenarios of the family non-universal$ Z^{\prime} $ model can be found for almost the whole kinematical range of$ q^2 $ , whereas the two scenarios remain distinguishable from each other in the range of$ q^{2}=(0.1-4) $ $ {\rm{GeV}}^{2} $ and$ q^{2}=(0.1-2.5) $ $ {\rm{GeV}}^{2} $ , for$ \langle \widehat{I}^{a_1}_{5,\parallel}\rangle $ and$ \langle \widehat{I}^{a_1}_{6s,\parallel}\rangle $ , respectively, as shown in Fig. 5(g) and 5(h).● Figure 6 presents the normalized angular observables
$ \langle \widehat{I}^{a_1}_{1s,\perp}\rangle,\langle \widehat{I}^{a_1}_{1c.\perp}\rangle,\langle \widehat{I}^{a_1}_{2s,\perp}\rangle,\langle \widehat{I}^{a_1}_{2c,\perp}\rangle,\langle \widehat{I}^{a_1}_{3,\perp}\rangle $ ,$ \langle \widehat{I}^{a_1}_{4,\perp}\rangle $ ,$ \langle \widehat{I}^{a_1}_{5,\perp}\rangle $ , and$ \langle \widehat{I}^{a_1}_{6s,\perp}\rangle $ for the$ B\to a_{1}(\to\rho_{\perp}\pi)\mu^{+}\mu^{-} $ decay in the SM as well as in S1 and S2 of the family non universal$ Z^{\prime} $ model. Although the angular observable$ \langle \widehat{I}^{a_1}_{6c,\perp}\rangle $ is non-zero, we do not present its results as its$ q^2 $ behaviour is similar to that of$ \langle \widehat{I}^{a_1}_{6s,\perp}\rangle $ , based on Eqs. (68) and (69). For all angular observables, scenario S1 of the family non-universal$ Z^{\prime} $ model overlaps the SM error band, whereas scenario S2 remains distinct from the SM and scenario S1 in different ranges of$ q^{2} $ for different angular observables, as shown in Fig. 6(a)−6(h). -
Investigating B meson decays allows us to test SM parameters while exploring NP. Several exclusive semileptonic decays, involving both flavor-changing neutral current transitions and flavor-changing charged current transitions, exhibit notable deviations from SM predictions. Semileptonic decays, involving the
$ b\rightarrow s $ current, have been examined during recent years and showed deviations from the SM predictions. In this study, the FCNC processes governed by the$ b\rightarrow dl^{+}l^{-} $ transition were examined in the family non-universal$ Z^{\prime} $ model. The four-fold angular decay distributions of$ B\to\rho(\to\pi\pi)\mu^{+}\mu^{-} $ and$ B\to a_{1}(\to\rho_{\parallel, \perp}\pi)\mu^{+}\mu^{-} $ decays were derived using the helicity formalism. For both decays, various physical observables were extracted and examined in the SM and two scenarios of the family non-universal$ Z^{\prime} $ model.From the analysis, a significant difference was observed among the SM and NP predicted values of the studied physical observables and in the majority of the normalized angular coefficients for the
$ B\to\rho(\to\pi\pi)\mu^{+}\mu^{-} $ decay, while the overall effect of NP was less distinct in most of the observables for the case of the$B\to a_{1}(\to \rho_{\parallel, \perp}\pi)\mu^{+}\mu^{-}$ decay. For instance, in the case of the differential branching ratio, the SM and$ Z^{\prime} $ model scenarios were distinguishable for the$ B\to\rho(\to\pi\pi)\mu^{+}\mu^{-} $ decay, while no clear distinction was observed for the$ B\rightarrow a_{1}\mu^{+}\mu^{-} $ decay. In the case of the forward-backward asymmetry and longitudinal polarization fraction, the patterns of deviations reported were similar for both decays. However, a distinction among the two scenarios appeared for a larger range of$ q^{2} $ in the case of the$ B\to\rho(\to\pi\pi)\mu^{+}\mu^{-} $ decay in comparison with the$ B\rightarrow a_{1}\mu^{+}\mu^{-} $ decay. Similarly, most of the normalized angular observables for the$ B\to\rho(\to\pi\pi)\mu^{+}\mu^{-} $ decay showed a notable distinction between the SM and the S1 and S2 scenario predictions of the family non-universal$ Z^{\prime} $ model, whereas, except for some, most of the normalized angular observables for the$ B\to a_{1}(\to\rho_{\parallel, \perp}\pi)\mu^{+}\mu^{-} $ decay showed little or no distinction between the SM and NP scenarios in the family non-universal$ Z^{\prime} $ model. Rare$ b\to d \ell \ell $ decays can be examined at high luminosity flavor facilities such as the LHCb [68] and Belle II [69]. In the present study, measurements of various normalized angluar observables on the order of 1% relative to the branching ratio on the order of$ 10^{-9} $ at the$ 3\sigma $ level require approximately$ 10^{13} $ $ B\bar B $ pairs. Furthermore, for the integrated luminosity goal of 300 fb–1 in HL-LHC$ \sim 10^{14} ,$ $ b\bar b $ pairs are expected to be produced [68, 70]. Therefore, precise measurements of these observables in experiments conducted at the LHCb and future collider facilities seem feasible and will provide valuable supplementary data needed to elucidate the underlying characteristics of NP within$ b\rightarrow dl^{+}l^{-} $ decays. -
The explicit expressions used for Wilson coefficients are provided as follows [39, 53−57]:
$\begin{aligned}[b] {C_{7}^{{\rm{eff}}}(q^2)}=\;&C_{7}-\frac{1}{3}\left(C_{3}+\frac{4}{3}C_{4}+20C_{5}+\frac{80}{3}C_{6}\right) \\&-\frac{\alpha_{s}}{4\pi}\left[(C_{1}-6C_{2})F^{(7)}_{1,c}(q^2)+C_{8}F^{(7)}_{8}(q^2)\right] \\&-\frac{\alpha_{s}}{4\pi}\lambda^{(q)}_{u}\left(C_{1}-6C_{2}\right)\left(F^{7}_{1,c}-F^{7}_{1,u}\right),\\ C_{9}^{{\rm{eff}}}(q^2)=\;&C_{9}+\frac{4}{3}\left(C_{3}+\frac{16}{3}C_{5}+\frac{16}{9}C_{6}\right) \\&-h(0, q^2)\left(\frac{1}{2}C_{3}+\frac{2}{3}C_{4}+8C_{5}+\frac{32}{3}C_{6}\right) \\ &-h(m_{b}^{{\rm{pole}}}, q^2)\left(\frac{7}{2}C_{3}+\frac{2}{3}C_{4}+38C_{5}+\frac{32}{3}C_{6}\right)\\&+h(m_{c}^{{\rm{pole}}}, q^2) \left(\frac{4}{3}C_{1}+C_{2}+6C_{3}+60C_{5}\right)\\ &+\lambda^{(q)}_{u}\left[h(m_{c},q^{2})-h(0,q^{2})\right]\left(\frac{4}{3}C_{1}+C_{2}\right) \\&-\frac{\alpha_{s}}{4\pi}\left[C_{1}F^{(9)}_{1,c}(q^2)+C_{2}F^{(9)}_{2,c}(q^2)+C_{8}F^{(9)}_{8}(q^2)\right]\\&-\frac{\alpha_{s}}{4\pi}\lambda^{(q)}_{u}\left[C_{1}(F^{(9)}_{1,c}-F^{(9)}_{1,u})+C_{2}(F^{(9)}_{2,c}-F^{(9)}_{2,u})\right], \end{aligned} $

(A1) where functions
$ h(m_{q}^{{\rm{pole}}}, q^2) $ with$ q=c, b $ and functions$ F^{(7,9)}_{8}(q^2) $ are as defined in [54], while functions$ F^{(7,9)}_{1,c}(q^2) $ and$ F^{(7,9)}_{2,c}(q^2) $ are as defined in [56] for low$ q^{2} $ and in [57] for high$ q^{2} $ . The quark masses appearing in all these functions are defined in the pole scheme. -
In this appendix, we provide the SM as well as the family non-universal
$ Z^{\prime} $ model predictions of physical observables for varying$ q^2 $ bins.
Effects of family non-universal Z ′ model in angular observables of ${\boldsymbol B {\bf\to} (\boldsymbol\rho, {\boldsymbol a}_{\bf 1})\boldsymbol\mu^+\boldsymbol\mu^- }$ decays
- Received Date: 2024-03-09
- Available Online: 2024-10-15
Abstract: We present the angular distribution of the four-fold







 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF










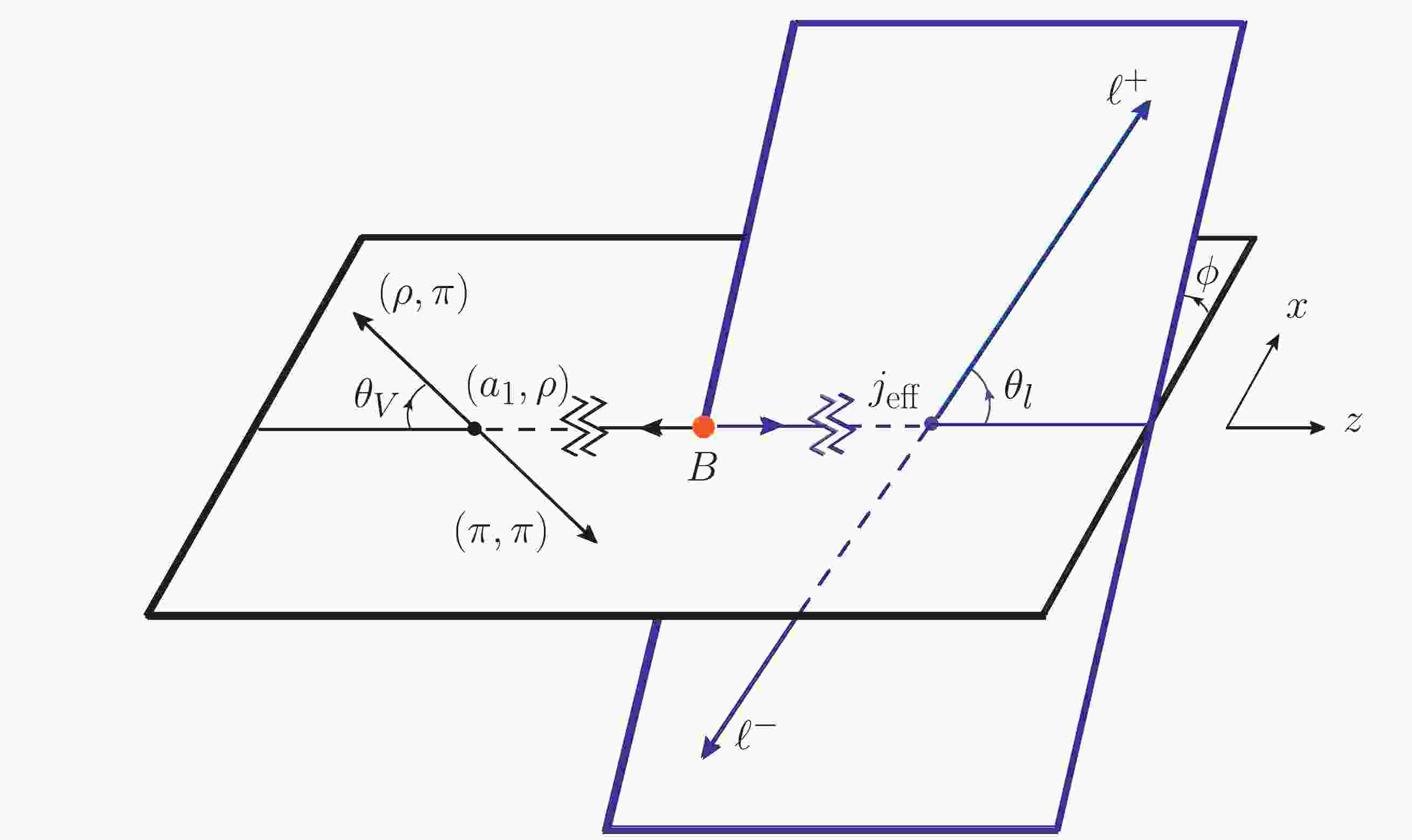













 DownLoad:
DownLoad: