Rotation effect of a fissile nucleus (ROT effect) for prompt γ-rays in binary fission of ²³⁵U by polarized neutrons of different energies

In 2005, at the high-flux neutron source ILL in Grenoble, the authors [1] discovered the effect of a rotation of the fissile nucleus in the angular distributions of α-particles from the ternary fission of ²³⁵U by cold polarized neutrons while studying the three-vector correlation (between the spin of the neutron causing fission, the direction of momentum of the light fission fragment, and the direction of α-particle emission). The observed effect manifested as a slight rotation of the angular distribution of α-particles from ternary fission relative to the axis of fragment emission, depending on the direction of the neutron spin.

In the classical model, this phenomenon was attributed to the collective rotation of the fissile nucleus at the moment of its rupture. The effect was subsequently named the "ROT effect" (derived from the word "rotation"). The experimental observation of this rotation effect of the fissile nucleus in the angular distribution of α-particles from ternary fission was enabled by the strong anisotropy of this distribution relative to the fission axis.

The authors [2, 3] demonstrated that the observed ROT effect in ternary fission is closely linked to the fission mechanism and is sensitive to the characteristics of collective transition states. The high sensitivity of the ROT effect to the parameters J and K (angular momentum and its projection onto the fission axis) was clearly shown through trajectory calculations [3]. These calculations, which are standard Monte Carlo simulations, involve event-by-event tracking of charged particle trajectories under the influence of a mutual Coulomb field and specified initial conditions. In this analysis, K values were treated as free parameters, varying from $ K = 0 $ to $ K = J $. By comparing the results of model calculations with experimental data, the authors [2, 3, 4] identified the most probable combinations of $ (J, K) $ for various fissioning nuclei.

Subsequent studies by the authors [5, 6, 7] investigated the effect of a rotation of the fissile nucleus in the angular distributions of prompt neutrons and γ-rays from the binary fission of ²³³U and ²³⁵U by cold polarized neutrons. These experiments were conducted at two facilities: the polarized neutron beam of the V-13 facility of the BER-II reactor (HMI, Berlin) and the polarized neutron beam of the MEPHISTO facility [8] of the FRM II reactor (TUM, Munich). The results revealed a similar effect in the emission of prompt γ-rays for both ²³⁵U and ²³³U. However, the observed effect was significantly smaller – approximately an order of magnitude – compared to the rotational effect detected in the emission of α-particles from ternary fission.

In recent years, our research group has also conducted a series of experiments to study the rotational effects of the fissile nucleus ²³⁶U in the $ ^{235}U(n,f) $ process, induced by monochromatic polarized neutrons with energies of 62 meV and 270 meV. These measurements were performed using the polarized neutron beam at the POLI instrument [9] of the FRM II reactor. To date, preliminary results of these measurements have been published in conference proceedings, though they exhibited slight variations among them [10, 11, 12, 13]. However, only the final results for the 62 meV neutron energy measurements have been formally published in [14].

This paper summarizes all previous studies on the ROT effects for prompt γ-rays emitted during the binary fission of ²³⁵U. For comparison, the rotation angle of the fission axis were determined for both cold neutrons (using processed data from the group [6]) and hot neutrons (0.27 eV), which were not included in the authors' earlier publications [5, 6, 7] or in our preliminary results. Additionally, the paper presents the relationship between the rotation angle of the fission axis and the energy of the neutrons inducing fission, providing an explanation for this relationship within the framework of a quasi-classical model.

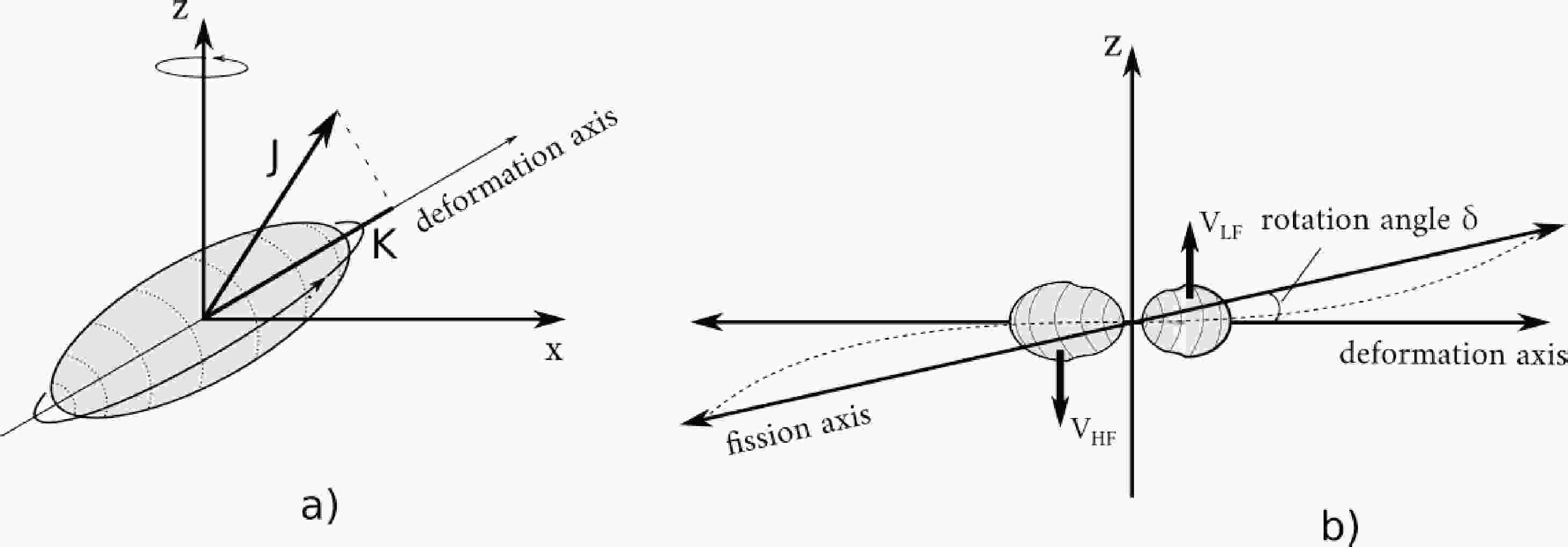
When a ²³⁵U nucleus in its ground state (spin $ I = \dfrac{7}{2} $) captures an s-wave neutron (with an orbital angular momentum of 0), a compound ²³⁶U nucleus is formed in states with spins $ J^{+} = I + \dfrac{1}{2} = 4 $ and $ J^{-} = I - \dfrac{1}{2} = 3 $. The excited states of this compound nucleus exhibit collective behavior, particularly rotation around an axis perpendicular to the deformation axis. If the ²³⁵U nucleus is unpolarized and the incident neutron beam is polarized ($ p_n $), the resulting compound nucleus becomes polarized. Consequently, the polarization of the compound nucleus gives rise to a rotation axis Z, around which the deformation axis (symmetry axis) of the nucleus rotates (see Fig. 1a). The plane of rotation may form a specific angle with the Z-axis.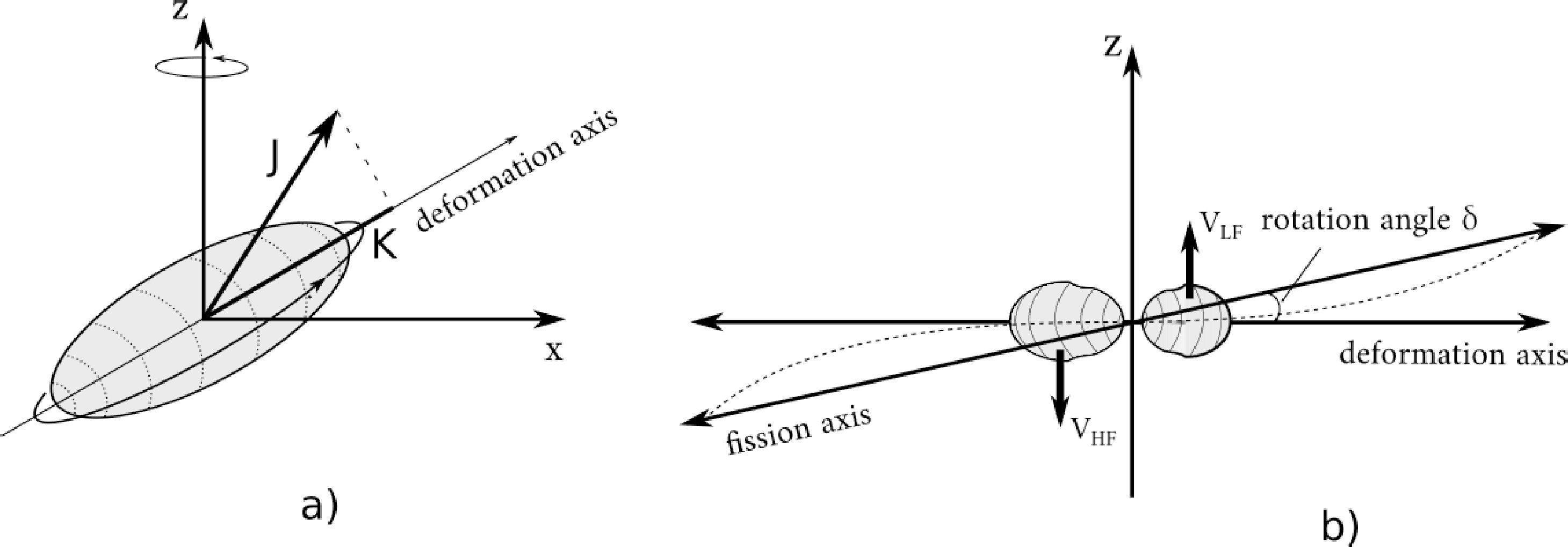

Figure 1.  Visualization of the rotation of a deformed nucleus: J – angular momentum, K – its projection onto the fission axis. b) Fission of a rotating nucleus: $ V_{LF} $, $ V_{HF} $ – tangential components of the fragment velocities at the initial moment of neck rupture.

Nuclei with transition state spins $ J^{\pm} = (I \pm \dfrac{1}{2}) $ exhibit distinct rotational speeds and directions [2]:

where ω is the angular speed of rotation, K is the projection of the angular momentum onto the fission axis, $ \Im $ is the moment of inertia around the Z axis in the scission configuration, $ \hbar $ is Planck's constant.

During the fission of such a rotating nucleus, the fission fragments acquire a tangential velocity component at the initial moment of neck rupture, directed perpendicular to the deformation axis of the fissile nucleus. As a result, instead of following a rectilinear trajectory due to Coulomb repulsion, the fragments follow a hyperbolic trajectory. This causes the emission axis of the fission fragments at infinity to deviate slightly from the deformation axis of the fissile nucleus at the time of neck rupture, forming a small angle δ (see Fig. 1b). Measuring this angle provides valuable information about the rotational speed of the nucleus.

The sign and magnitude of the rotation angle are determined by the ratio of the spin components' contributions to the fission cross-section, which itself depends on the energy of the incident neutron. Figure 2 shows the spin-separated fission cross-section for ²³⁶U* as a function of energy, calculated using the SAMMY computer code [15].

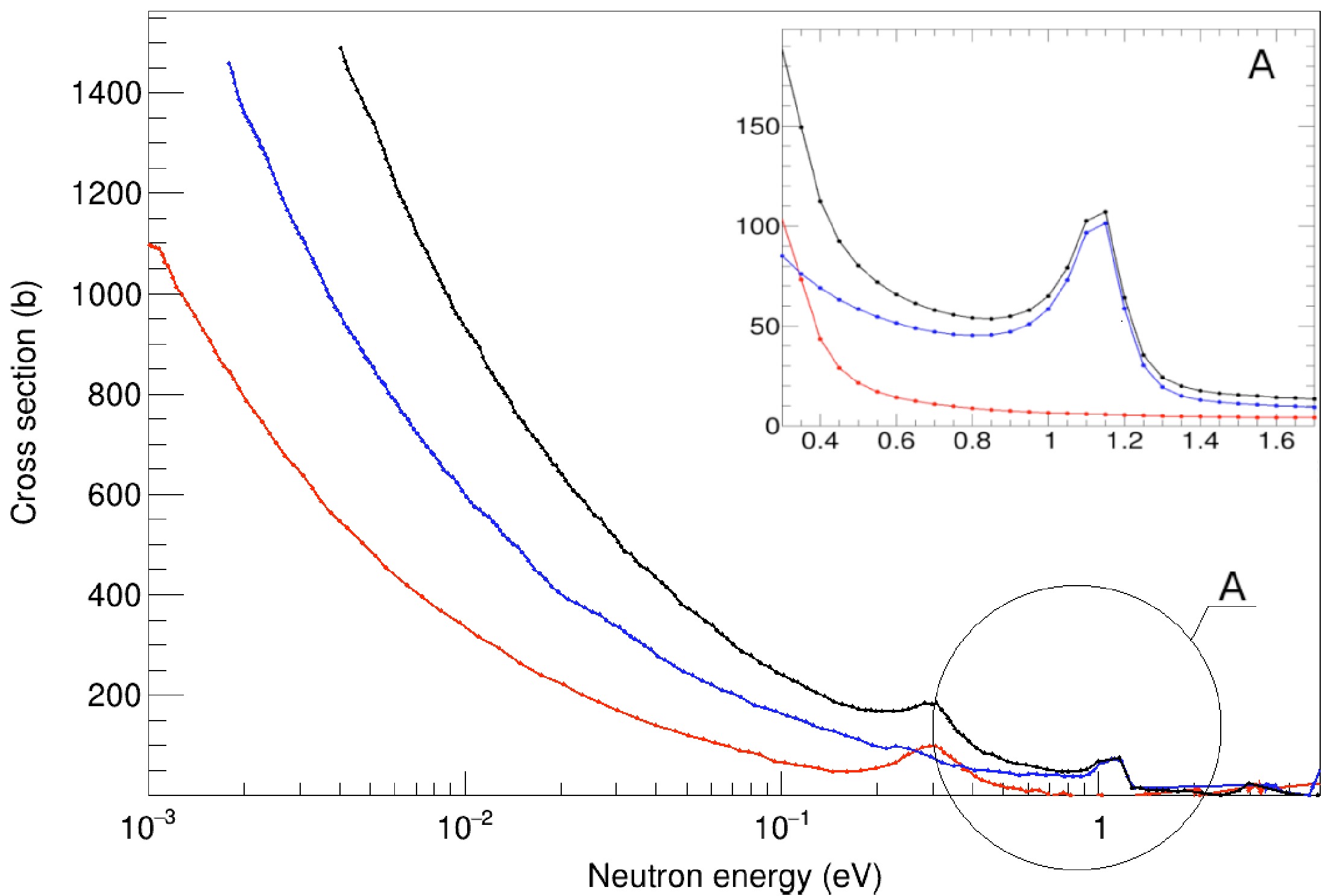
Figure 2.  (color online) Spin-separated fission cross sections for ²³⁶U* depending on the energy of the incident neutron. Red line corresponds to J = 3 spin, blue line to J = 4 spin, black line to the sum of both, respectively.

From this figure, it is clear that at low neutron energies, the fission channels are mixed, meaning both resonances contribute to the fission cross-section. However, the ratio of their contributions and the spin states of the compound nucleus remain undefined. In contrast, isolated resonances, such as the one at 1.14 eV (see Fig. 2), exhibit contributions from only a single spin state to the fission cross-section. Analyzing these isolated resonances provides a more reliable understanding of their physical nature, enabling the determination of the weights of the J and K channels.

In the experiment, the direction of fission fragment emission from the target is recorded. However, the orientation of the deformation axis at the moment of neck rupture is not directly observable. To determine this orientation, a characteristic marker is needed. Nuclear fission is typically accompanied by the emission of secondary particles, whose angular distributions are defined relative to the deformation axis of the nucleus at the moment of neck rupture. If these angular distributions are anisotropic, the secondary particles can serve as a reliable marker for reconstructing the deformation axis orientation.

The angular distribution of prompt γ-rays is also anisotropic [16, 17]. However, these γ-rays are emitted not at the moment of neck rupture but by excited fission fragments after the rotation of the fissile nucleus's deformation axis has already occurred. An explanation for how prompt γ-rays can exhibit the ROT effect was proposed by V.V. Novitsky [5], based on V.M. Strutinsky's hypothesis [18] regarding the alignment of fission fragment spins in a plane orthogonal to the fission axis. According to this model, at the moment of neck rupture, the spins of the excited fission fragments align in a plane orthogonal to the deformation axis connecting the centers of mass of the two fragments. This spin orientation remains unchanged even as the trajectories of the fragments deviate from the original direction of the deformation axis.

The experimental setup for the study of the ROT effect includes: a neutron polarizer, a neutron polarization analyzer, a neutron spin control system, a two-sided fissile target, a vacuum fission chamber, low-pressure multiwire proportional counters (LPMWPC) for detecting fission fragments (start and stop detectors), scintillation detectors, located around the target at different angles, for detecting neutrons and γ-rays accompanying fission, as well as a neutron beam monitor. A detailed description and performance evaluation of the setup, data acquisition electronics are given in [19, 20]. A schematic view of the detector part of the experimental setup is shown in Fig. 3.

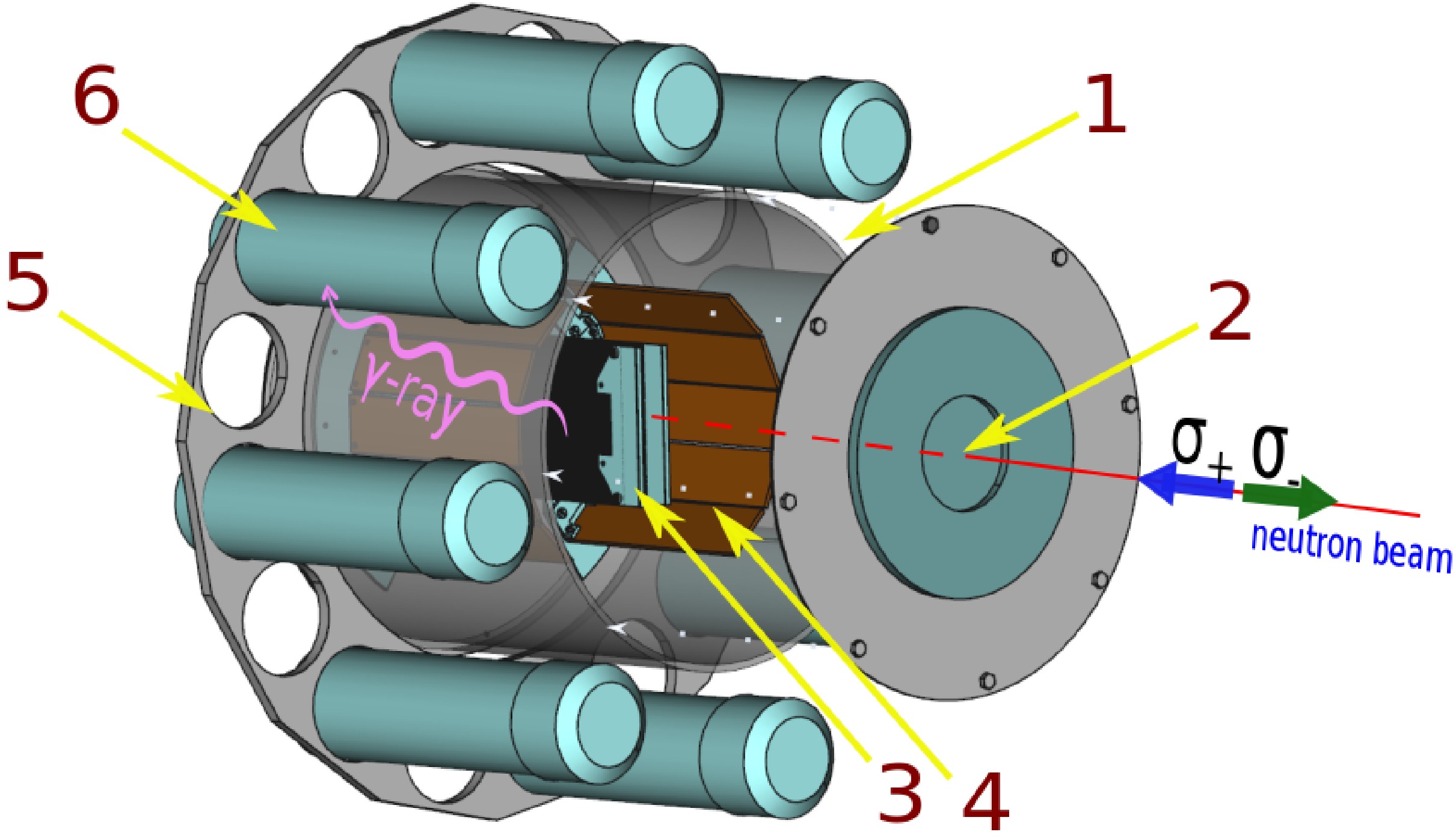
Figure 3.  (color online) Diagram of the detector part of the experimental setup: 1 – fission chamber, 2 – input Al window of the chamber, 3, 4 – fission fragment detectors based on position-sensitive low-pressure multi-wire proportional counters (start and stop detectors), 5 – detector holder, 6 – scintillation plastic detectors of γ-rays and neutrons. The fissile target is in the center, between the starting detectors. The scale of the figure is 1 to 10.

In the experiment, longitudinally polarized neutrons irradiate a ²³⁵U target located in the center of the fission chamber. Rotation of the deformation axis at the rupture point of the ²³⁵U nucleus leads to a shift of the fission axis. Due to the rotation of the system, the angle at which the γ-rays are registered with respect to the axis of fission fragment detection is not θ, but a new angle $ \theta^{'} $. This angle is equal to θ minus or plus δ, which is the rotation angle of the fission axis, depending on the spin of the polarized neutron used in the experiment:

However, this does not imply that the angular distributions of the particles rotate in the same manner as the fragments themselves. Instead, these shifts should be understood as arising from minor changes in the coordinate system used for measurements. Because the detectors remain fixed, the angular distributions of particles associated with the fragments in the two coordinate systems – corresponding to the opposite directions of neutron polarization – are shifted relative to each other.

Following the work [18], the rate of γ-rays counting without rotation of the nuclear system is as follows:

where A is the coefficient of anisotropy. The count rates corresponding to different spin states of the neutron can be written as:

By measuring the counting rate of coincidences of signals from γ-ray detectors and fragment detectors with respect to the polarization direction of the neutron beam, the counting asymmetry is determined:

where $ N^{+}(\theta^{'}) $ and $ N^{-}(\theta^{'}) $ is the number of coincidences of signals from γ-ray detectors and from fragment detectors, located at an angle θ to each other in the plane orthogonal to the axis of the longitudinally polarized neutron beam, measured at two opposite directions of the neutron beam polarization. Then the magnitude of the measured asymmetry is determined by the formula:

Thus, by measuring the asymmetry and determining the function of the angular distribution of γ-rays, it is possible to determine the angle by which the fragment trajectory is shifted, and, consequently, the angular velocity and direction of rotation of the fissile nucleus.

During the experiment, for each trigger event occurring when one of the fragment detectors coincided with one of the plastic detectors, three signal processing lines were recorded:

● the arrival time of the stop signal on the TDC;

● the amplitude of the pulses recorded using the ADC;

● the formed pulses from all detectors, as well as the current state of the neutron spin, which were registered on the counters.

The frequent (about 1 Hz) spin flip of the incident neutron beam was one of the main mechanisms used for suppressing instrumental asymmetry. The analysis of the experimental data was conducted using a computer program written in C++. This program allows calculating the asymmetry for any possible combination of angles between fission fragments and prompt γ-rays, as well as neutrons, and determining the rotation angle of the fissile nucleus before its breakup. The algorithm of the program is described in detail in [10]. The program records in an array the results of coincidences of pulses from eight independent plastic detectors with each pulse from ten segments of the stop detector. As a result, data on 16 different angles between the fragment detectors and γ/neutrons in the experiment is accumulated.

The ROT asymmetry was calculated from the experimental data using Eq. 4. The measurement error was calculated using the following formula:

The calculation results were recorded in a text file, and graphs were constructed based on them using the ROOT library.

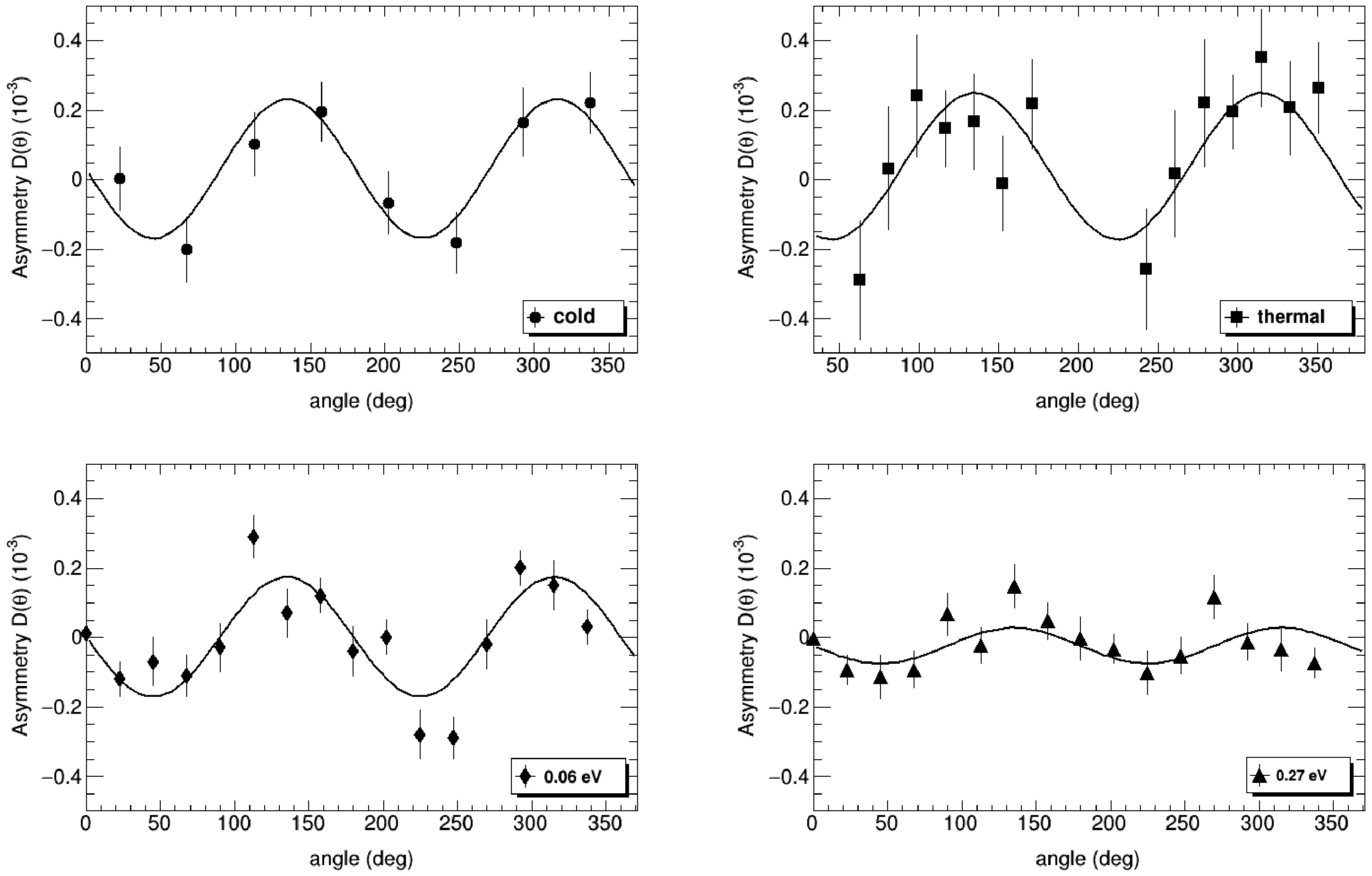
The updated results from all our measurements are compiled in Tab. 1 and depicted in Fig. 4. We also revisited and reanalyzed data from earlier studies conducted by the ITEP group [6], which investigated the rotation effect of a fissile nucleus in the angular distributions of prompt γ-rays of fission of ²³⁵U nuclei by polarized cold neutrons. However, that publication only provided the ROT-asymmetry coefficient values at an angle of 67.5° relative to the average axis of fragment and γ-ray detection. This angle was chosen by the authors because it was close to the maximum ROT-asymmetry. Notably, the sign of the effect measured in [6] was initially opposite to that observed in later experiments. Over time, the sign of the effect reported by the ITEP group reversed and eventually aligned with our findings [7]. In our latest experiments, we specifically determined the sign of the effect [14], which we consider reliable. Unfortunately, for the cold neutron measurements, no preserved information exists regarding the directions of the magnetic fields or the neutron spins in the region between the polarizer and the analyzer, making it impossible to resolve these discrepancies. In this paper, we assert that the sign of the effect for cold neutrons is consistent with our results. To provide a comprehensive overview of ROT asymmetry for γ-rays, Tab. 1 and Fig. 4 include updated data for cold neutrons alongside results for thermal neutrons from the PNPI group [21].

	Asymmetry parameter $ R_{\gamma} $, in units of 10−5
$ E_n $(eV)	Preliminary results	Corrected results
cold	20.9 ± 2.4 (for 67.5°) [6]	−20.0 ± 4.5
thermal		−21.1 ± 6.8 [21]
0.06	−12.5 ± 3.1 [13]	−17.3 ± 2.8 [14]
0.27	3.8 ± 2.8 [12]	−5.4 ± 2.5

Table 1.  ROT asymmetry parameters for prompt γ-rays of ²³⁵U fission.

Figure 4.  The asymmetry ratio $ D(\theta^{'}) $ as a function of the angle for prompt fission γ-rays. The solid line shows the result of approximation of the obtained angular dependence $ D(\theta^{'}) $ by the function $ F = R_{\gamma}sin(2\theta) $.

The ROT effect is explained by considering the preservation of the spin orientation of the primary fission fragment and the anisotropy of the gamma radiation generated by this spin in the center-of-mass frame of the fragment [21]. The γ-radiation anisotropy coefficient A for binary fission fragments of the ²³⁶U compound nucleus was measured in each experiment [22]. It is important to note that these measurements were conducted within the same γ-ray energy range and detector geometry used to study the ROT effect, ensuring that any potential effects related to the experimental setup's geometry were consistent in both cases. The measured angular distributions of prompt γ-rays were then approximated by Eq. 3, and the corresponding anisotropy coefficients were determined. By substituting these values into Eq. 6, we calculated the angle δ, which characterizes the rotation of the fission axis and, consequently, the angular shift in the gamma distribution. Table 2 presents the calculated values of the anisotropy coefficient A and the rotation angle δ for all experimental series, along with the result obtained by the PNPI group for thermal neutrons.

The study of the dependence of the ROT effect magnitude on the energy of neutrons causing the fission can help to understand the details of the fission mechanism, such as the transition of the angular momentum from the fissile nucleus to fission fragments.

At an energy of 1.14 eV, the magnitude of the ROT effect is expected to be higher than for cold neutrons. This expectation arises from Fig. 2, which demonstrates the resonant dominance of the J = 4 spin state at this particular energy. The lack of experimental data on the study of the ROT effect in this resonance is primarily due to the current unavailability of high-intensity sources of polarized neutrons with the required energy at the moment.

For cold neutrons, the $ \delta(J=4) / \delta(J=3) $ ratio is ≈1.8. Furthermore, according to Eq. 1 that the partial angular velocity of rotation of the state $ I + \frac{1}{2} $ is greater in absolute value the partial velocity of rotation in the opposite direction of the composite state $ I - \frac{1}{2} $. Consequently, a positive and significant value of the ROT effect is observed in the region of cold neutrons. The ratio $ \delta(J=4) / \delta(J=3) $ or polarized neutrons in the thermal region is approximately the same as in the region of cold neutrons. Therefore, the expected values of the ROT effect for thermal and cold neutrons should coincide, which are consistent with the experimental results within the experimental error (see Tab. 2 and Fig. 5).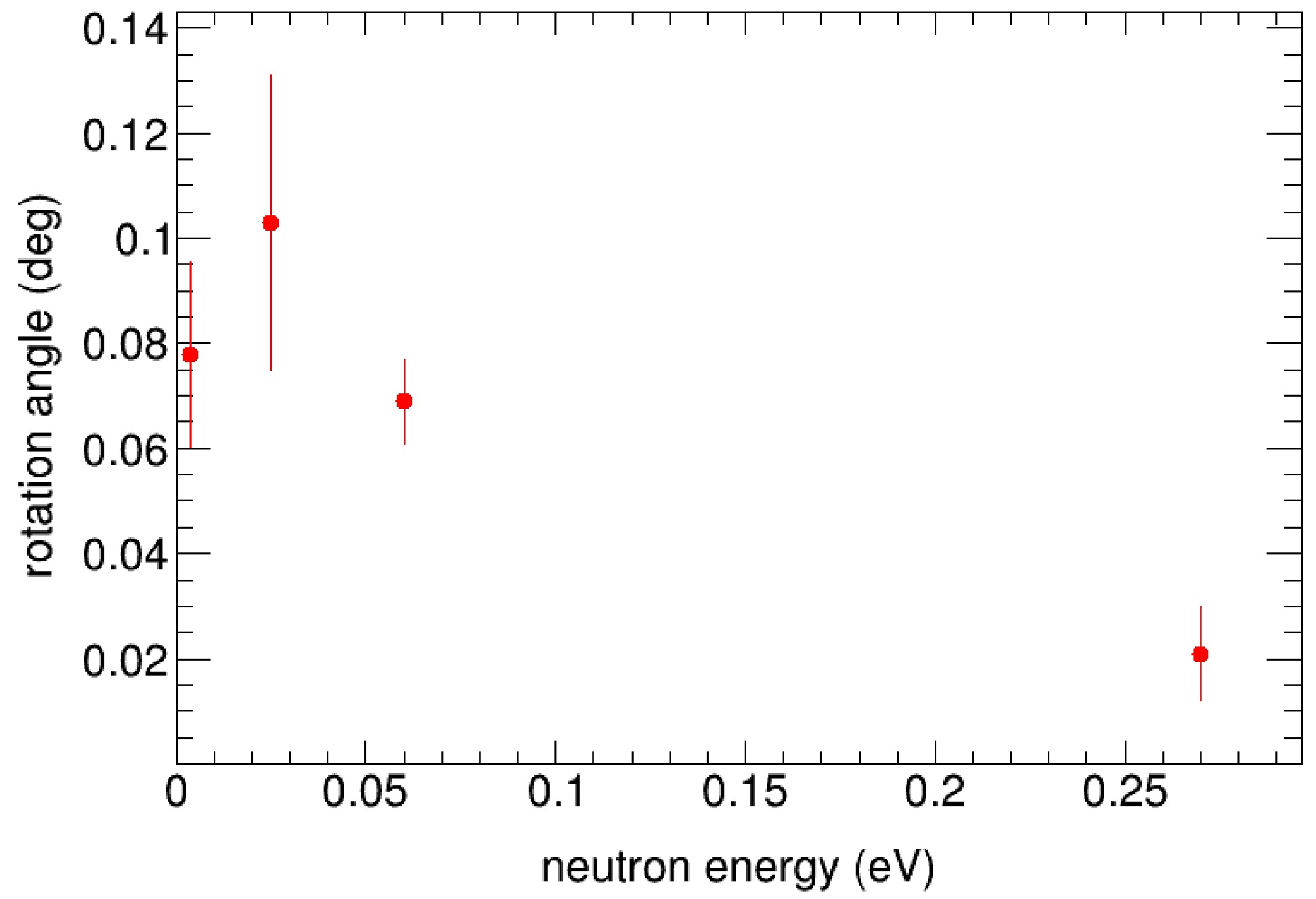

Figure 5.  (color online) Dependence of the rotation angle δ on the energy of neutrons causing the fission.

As the energy approaches 0.27 eV (3 resonance of ²³⁵U isotope), the ratios of the spin-dependent contributions of the fission cross section change. At this energy, the cross section with spin $ J = 3 $ prevails, albeit slightly. It can be assumed that the observed effect will change sign in this region. However, according to the calculations [4, 15], the rotation in the positive direction ($ J = 4 $) continues to dominate due to the much higher absolute value of the partial angular velocity compared to the rotation velocity in the opposite direction ($ J = 3 $). Consequently, a positive sign of the measured ROT effect was obtained. The magnitude of the effect was found to be 3.5 times lower than for thermal or cold neutrons, which also aligns with the statements given by the authors of [4, 15], who developed one of the most complete models of the ROT effect.

Experimentally measured rotation angles reveal that, in all compound nuclei formed by the capture of polarized neutrons with varying energies, the direction of rotation aligns with the direction of the neutron's initial spin. This suggests that the effective angular velocity in these cases is consistently positive. The differing absolute values of the rotation angles arise from various combinations of channels $ (J, K) $.

It is known that the spin state of a compound nucleus is influenced not only by the value of J but also by the projections of K, which determine the orientation of the spin along a given axis of symmetry. When the projection of K changes, both the shape of the nucleus and its energy levels are affected. These configurations can be understood through the shell model and the interactions between nucleons. These principles provide insight into how the shape and properties of the nucleus evolve with changes in the spin projection onto the axis of symmetry, thereby opening up new avenues for experiments in the field of nuclear physics.

The initial attempts to determine the projections of K based on the results of the ROT effect were made by the authors of [1, 3]. By comparing the results of model calculations with experimental data (the experimentally found value $ 2\Delta = 0.215^{\circ} $), the most probable combination $ (J, K) $ was found. The combinations $ (J, K) $ = (3, 2) and $ (J, K) $ = (4, 0) provided a significantly better fit for angular shifts as a function of the energies of the thernary particles and fragments involved. In [4], the authors attempted to correlate the K values obtained in this manner with the transition states of K identified in binary fission [23, 24, 25]. In these studies focusing on cold neutrons, the $ (J, K) $ combination of (4, 2) contributed 70%, while (4, 1) contributed 30%. For the state with J = 3, the contributions from $ (J, K) $ = (3, 0) and (3, 1) were 70% and 30%, respectively. These results, obtained using a multichannel multilevel fit, are more detailed than the data of the authors of [1, 3], who assumed that only one channel is effective at each level. Consequently, discrepancies exist in the estimates of K between binary and ternary fission. The authors of [4] note that due to the different values of K, it appears that the two modes (binary and ternary fission) at the saddle point proceed through different transition states.

The rotation angles of the fissile nucleus observed for α-particles in ternary fission and for prompt γ-rays in binary fission do not match. In [21], the authors reported a rotation angle of $ \delta = 0.183(2)^{\circ} $ for ternary fission of ²³⁵U, which is approximately 1.8 times greater than the angle measured for prompt γ-rays in the same study. However, the deviations in the fission axis for both binary and ternary fission share the same sign, aligning with the mechanism outlined in this work. The authors of [21] propose that the different rotation angles may result from varying moments of inertia. Near the moment of rupture, the composite system exhibits a more elongated configuration in binary fission compared to ternary fission.

In later studies [26], the authors suggest that the smaller rotation angle observed in binary fission could be attributed to the time required for the formation of fragment spin. This conclusion builds on Wilson's research regarding the generation of angular momentum in nuclear fission [27]. During this interval, the fragments can travel a certain distance apart, leading to an increase in the system's moment of inertia and a reduction in its rotational speed. In other words, the motion direction of the fission fragment, which results in the anisotropy of the gamma radiation distribution, does not align with its motion direction at the moment of nuclear rupture but instead corresponds to a later stage in the fission process.

It is anticipated that measuring the ROT effect at the 1.14 eV resonance will help clarify these assumptions. This resonance is notably pure, with minimal contributions from cross sections associated with states of the compound nucleus that have different possible spins. The experimental data obtained will be valuable for investigating the dynamics of the fission process, particularly near the scission point, and for developing a comprehensive quantum-mechanical model of fission, which is currently lacking.

In recent years, advancements in polarized spin filters ³He for neutrons, utilizing the SEOP optical pumping method, have led to the emergence of high-intensity polarized neutron sources with energies exceeding 1 eV [28, 29]. This development opens up new research opportunities. Recently, we discussed the possibility of conducting such measurements at the CSNS facilities with our Chinese colleagues, and we hope to carry out similar experiments there in the near future. Additionally, we propose measuring the ROT effect at the 1.14 eV resonance at the IBR-2M reactor in Dubna using thick targets that are opaque to fragments. The methodology for conducting this experiment was detailed in [30]. In these studies, we plan to reconstruct the trajectory of fragment motion from the angular distributions of prompt fission neutrons. Although the magnitude of the ROT effect for prompt gamma quanta in coincidence with neutrons will be smaller than that observed in coincidence with fragments, the higher statistical accuracy of the experimental results will enhance the reliability of our measurements.

In the conclusion of the article, it should be noted that the conducted studies on ROT effects for prompt γ-rays from the fission of ²³⁵U using polarized neutrons provide important experimental data that are highly significant for the theory of nuclear fission and the study of the dynamics of the fission process, especially near the break point. The article consolidates the results of measurements of ROT asymmetry and the rotation angles of the fissile nucleus of ²³⁵U for various neutron energies, including data from our group (for energies of 0.06 and 0.27 eV) and results from other research teams. It should be highlighted that this work first demonstrates the rotation angle of the nucleus for cold neutrons and for the first isolated resonance of ²³⁵U (0.27 eV). To calculate the rotation angle of the nucleus for cold neutrons, the old data from the authors of ITEP were reprocessed.

Moreover, the obtained values of ROT-asymmetry for the first isolated resonance indicate that the effect is significantly smaller compared to cold neutrons. Nevertheless, this result is important for testing proposed theoretical models and understanding the fission process as a whole. The theoretical calculations [23, 24] predicted such a reduction in the anisotropy coefficient for the isolated resonance at 0.27 eV for ²³⁵U, based on known contributions from J = 3 and J = 4 partial cross-sections for these nuclei, as well as from the values of the most probable K-channel for these spins, obtained from their work.

It is also worth emphasizing that the difference in the rotation angles of the fission axis during different types of fission (binary and ternary) may be the key to a deeper understanding of the fission mechanism. In the future, additional planned experiments, especially at the resonance of 1.14 eV, may clarify the correlations between the rotation angles and serve as a basis for more accurate theoretical calculations in this area.

We're grateful to S. Masalovich, V. Hutanu, and J. Klenke for their help in setting up and conducting the experiment.